Fractals on:
[Wikipedia]
[Google]
[Amazon]

 In
In 
 Starting in the 17th century with notions of recursion, fractals have moved through increasingly rigorous mathematical treatment to the study of
Starting in the 17th century with notions of recursion, fractals have moved through increasingly rigorous mathematical treatment to the study of
 The word "fractal" often has different connotations for the lay public as opposed to mathematicians, where the public is more likely to be familiar with
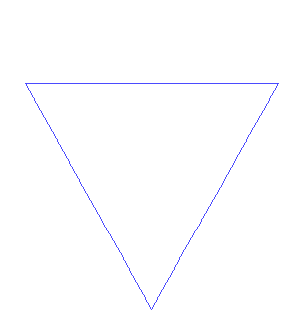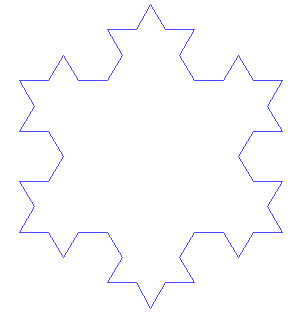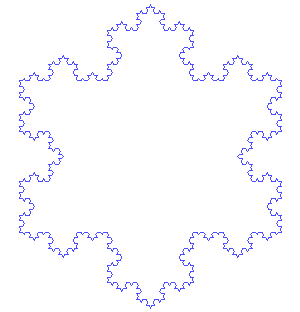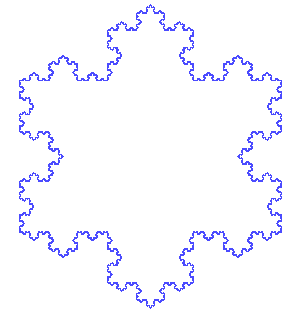
The word "fractal" often has different connotations for the lay public as opposed to mathematicians, where the public is more likely to be familiar with  This also leads to understanding a third feature, that fractals as mathematical equations are "nowhere differentiable". In a concrete sense, this means fractals cannot be measured in traditional ways. To elaborate, in trying to find the length of a wavy non-fractal curve, one could find straight segments of some measuring tool small enough to lay end to end over the waves, where the pieces could get small enough to be considered to conform to the curve in the normal manner of measuring with a tape measure. But in measuring an infinitely "wiggly" fractal curve such as the Koch snowflake, one would never find a small enough straight segment to conform to the curve, because the jagged pattern would always re-appear, at arbitrarily small scales, essentially pulling a little more of the tape measure into the total length measured each time one attempted to fit it tighter and tighter to the curve. The result is that one must need infinite tape to perfectly cover the entire curve, i.e. the snowflake has an infinite perimeter.
This also leads to understanding a third feature, that fractals as mathematical equations are "nowhere differentiable". In a concrete sense, this means fractals cannot be measured in traditional ways. To elaborate, in trying to find the length of a wavy non-fractal curve, one could find straight segments of some measuring tool small enough to lay end to end over the waves, where the pieces could get small enough to be considered to conform to the curve in the normal manner of measuring with a tape measure. But in measuring an infinitely "wiggly" fractal curve such as the Koch snowflake, one would never find a small enough straight segment to conform to the curve, because the jagged pattern would always re-appear, at arbitrarily small scales, essentially pulling a little more of the tape measure into the total length measured each time one attempted to fit it tighter and tighter to the curve. The result is that one must need infinite tape to perfectly cover the entire curve, i.e. the snowflake has an infinite perimeter.
 The history of fractals traces a path from chiefly theoretical studies to modern applications in computer graphics, with several notable people contributing canonical fractal forms along the way.
A common theme in traditional African architecture is the use of fractal scaling, whereby small parts of the structure tend to look similar to larger parts, such as a circular village made of circular houses.
According to Pickover, the mathematics behind fractals began to take shape in the 17th century when the mathematician and philosopher Gottfried Leibniz pondered recursive self-similarity (although he made the mistake of thinking that only the straight line was self-similar in this sense).
In his writings, Leibniz used the term "fractional exponents", but lamented that "Geometry" did not yet know of them. Indeed, according to various historical accounts, after that point few mathematicians tackled the issues and the work of those who did remained obscured largely because of resistance to such unfamiliar emerging concepts, which were sometimes referred to as mathematical "monsters". Thus, it was not until two centuries had passed that on July 18, 1872 Karl Weierstrass presented the first definition of a function with a graph that would today be considered a fractal, having the non-
The history of fractals traces a path from chiefly theoretical studies to modern applications in computer graphics, with several notable people contributing canonical fractal forms along the way.
A common theme in traditional African architecture is the use of fractal scaling, whereby small parts of the structure tend to look similar to larger parts, such as a circular village made of circular houses.
According to Pickover, the mathematics behind fractals began to take shape in the 17th century when the mathematician and philosopher Gottfried Leibniz pondered recursive self-similarity (although he made the mistake of thinking that only the straight line was self-similar in this sense).
In his writings, Leibniz used the term "fractional exponents", but lamented that "Geometry" did not yet know of them. Indeed, according to various historical accounts, after that point few mathematicians tackled the issues and the work of those who did remained obscured largely because of resistance to such unfamiliar emerging concepts, which were sometimes referred to as mathematical "monsters". Thus, it was not until two centuries had passed that on July 18, 1872 Karl Weierstrass presented the first definition of a function with a graph that would today be considered a fractal, having the non-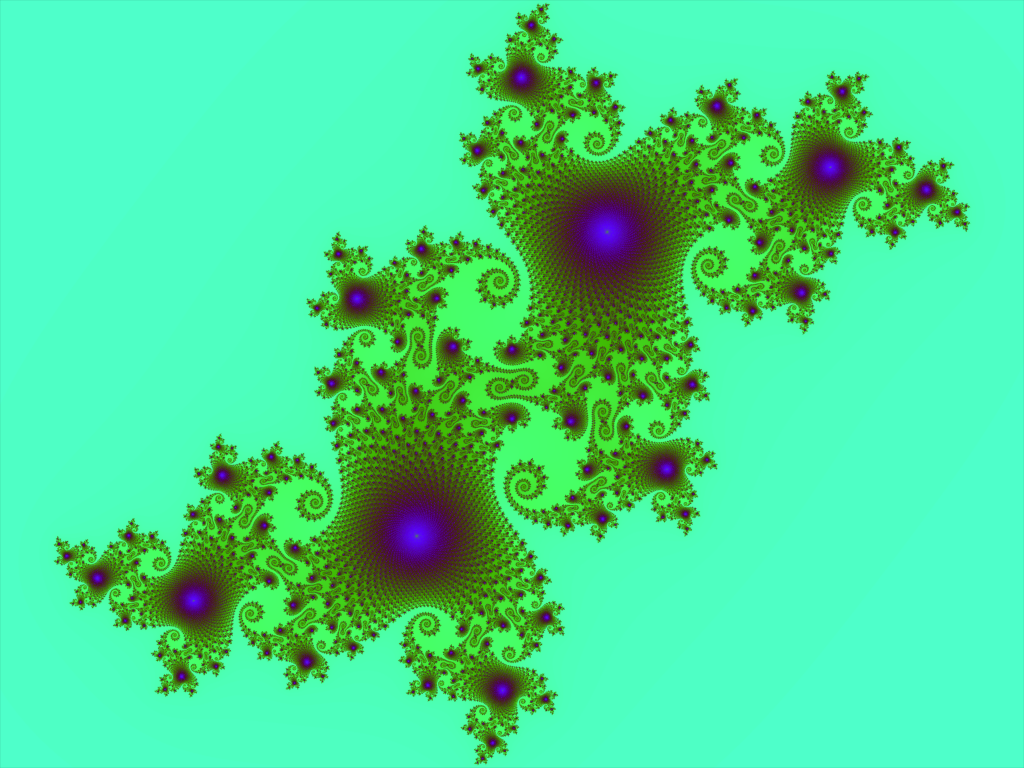
 One of the next milestones came in 1904, when
One of the next milestones came in 1904, when 
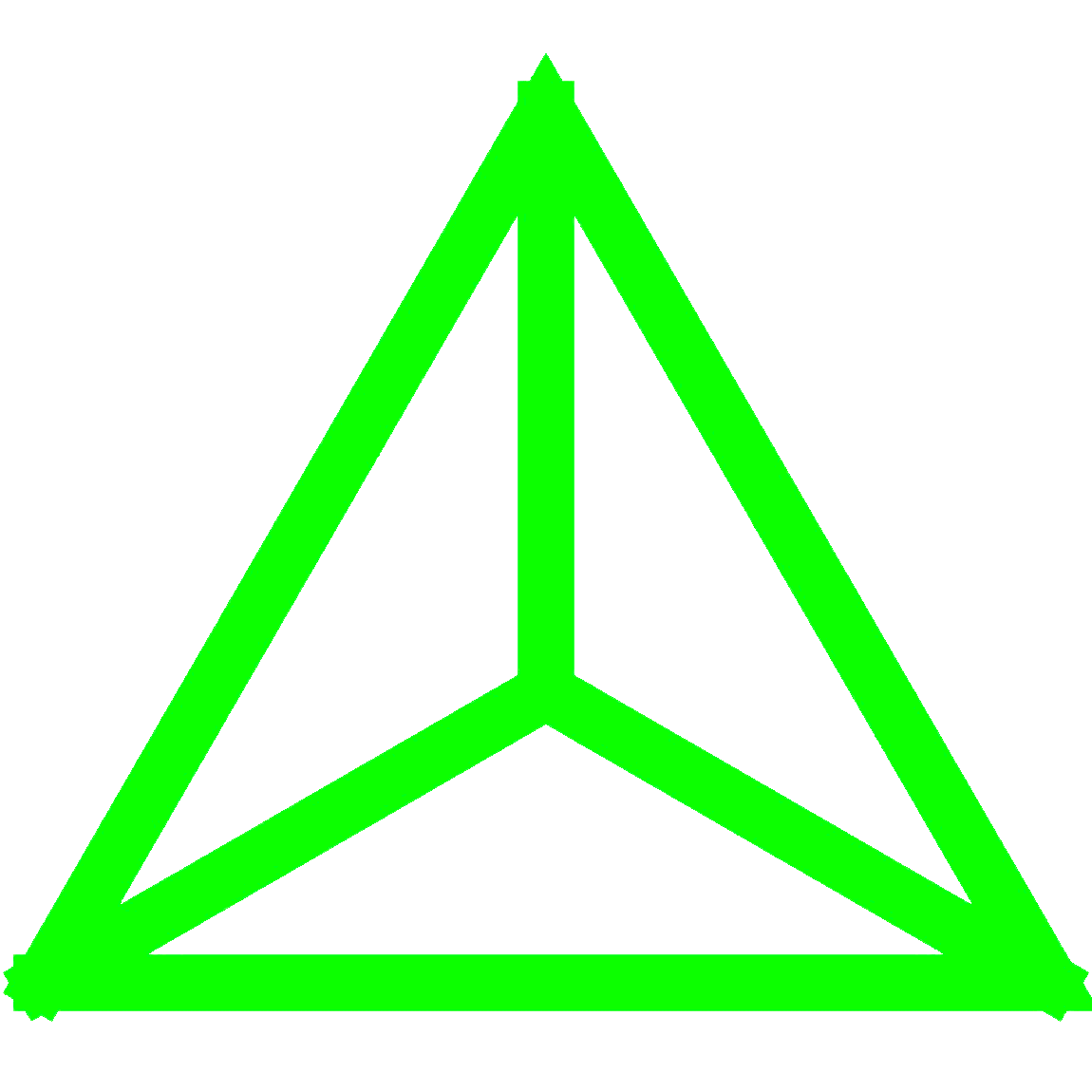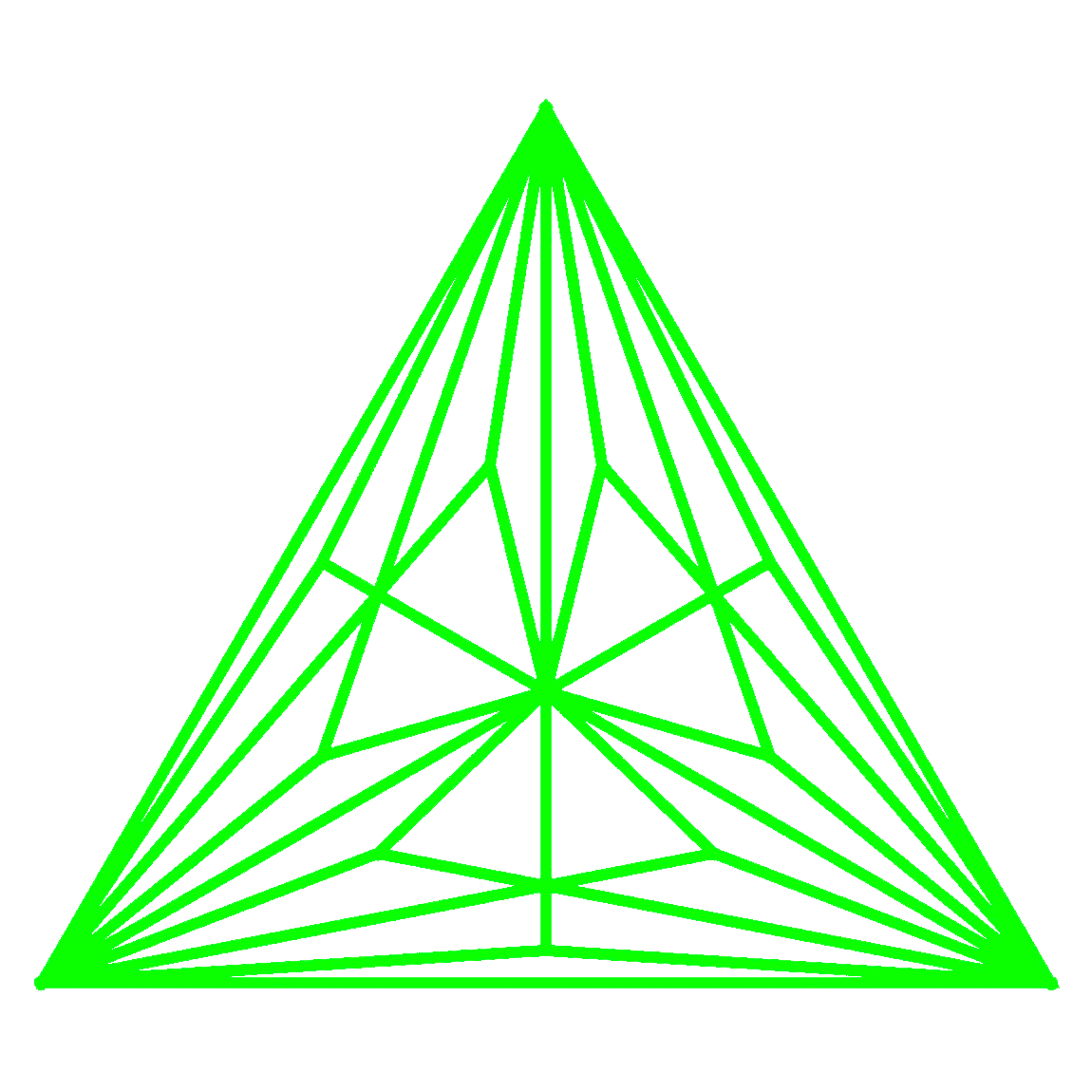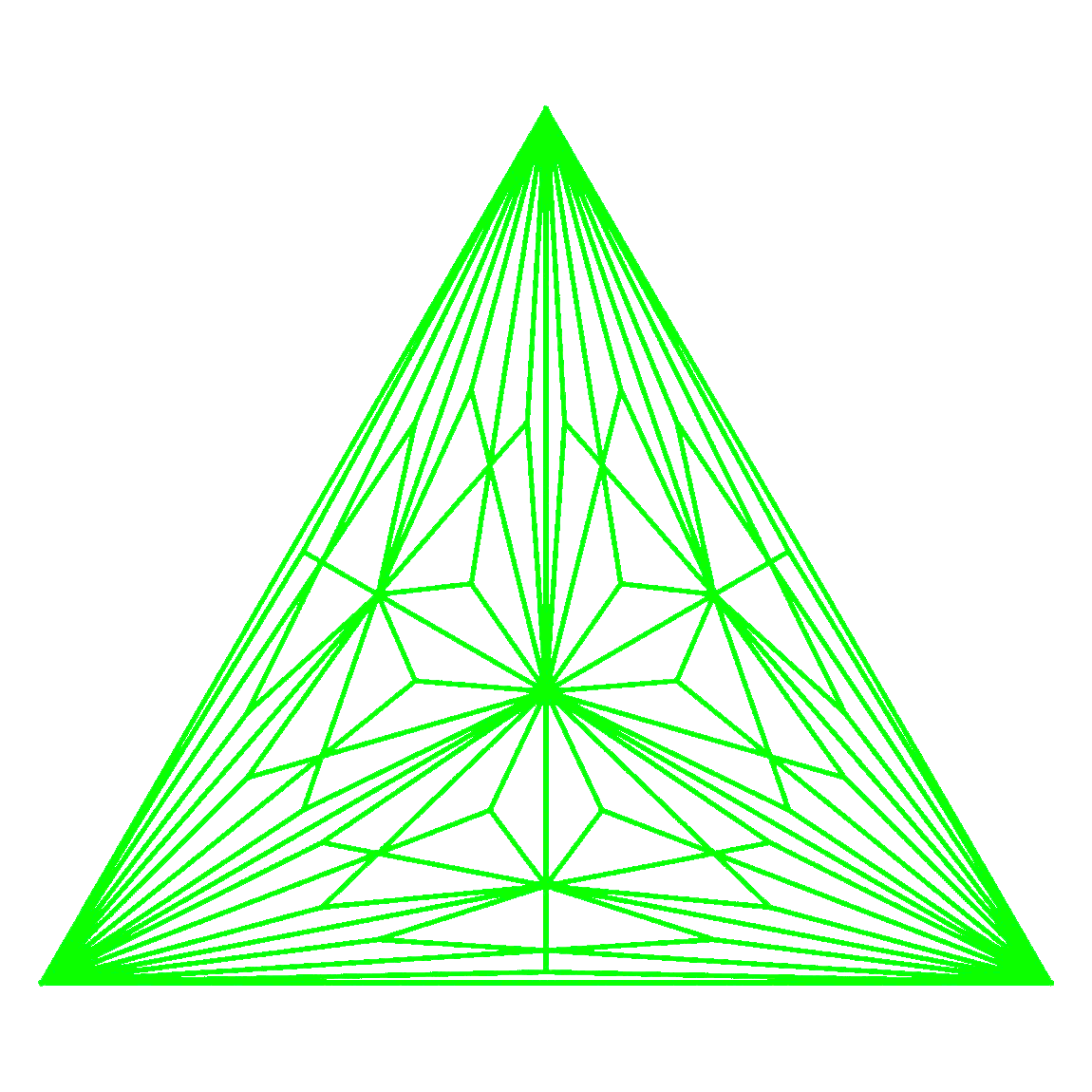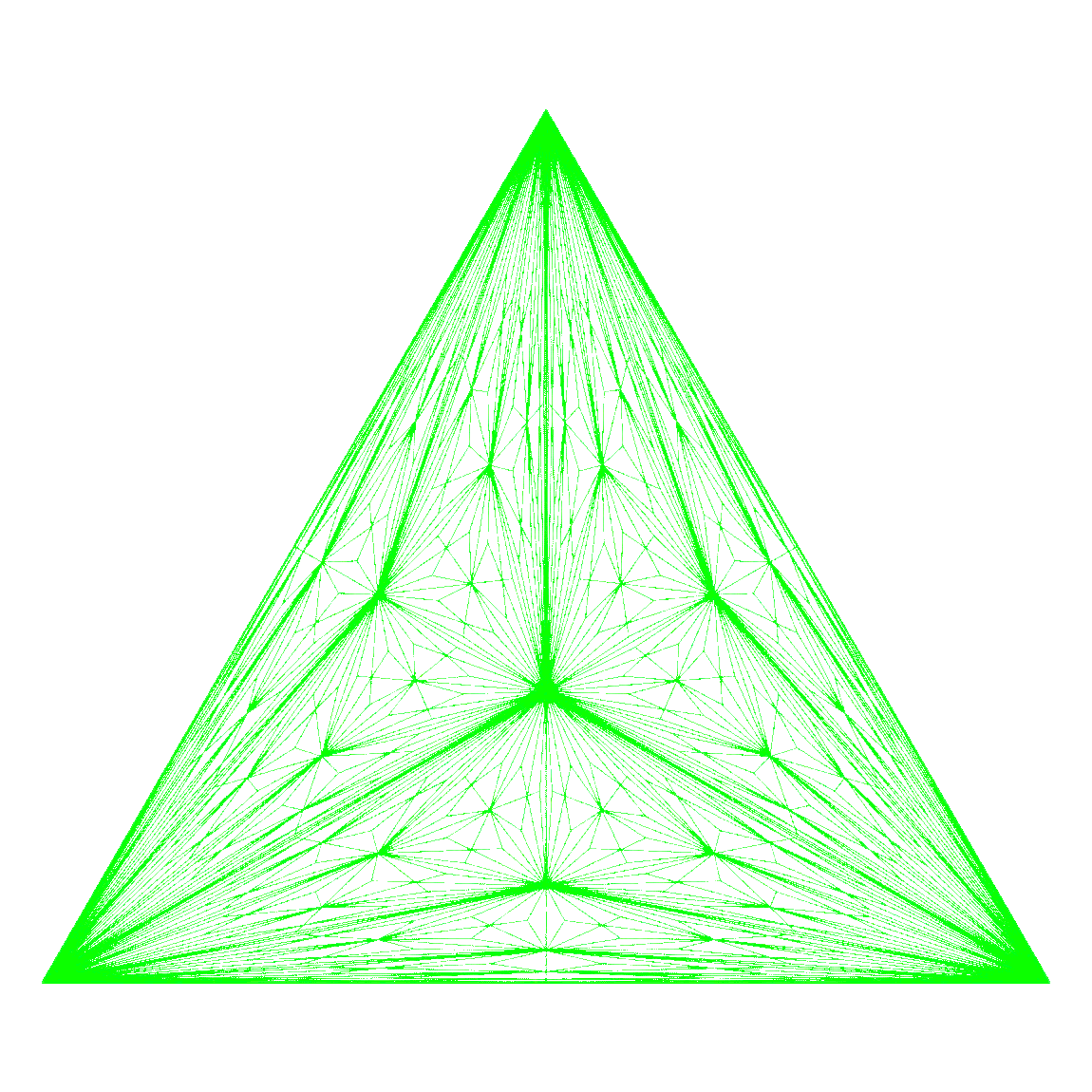
 Different researchers have postulated that without the aid of modern computer graphics, early investigators were limited to what they could depict in manual drawings, so lacked the means to visualize the beauty and appreciate some of the implications of many of the patterns they had discovered (the Julia set, for instance, could only be visualized through a few iterations as very simple drawings). That changed, however, in the 1960s, when
Different researchers have postulated that without the aid of modern computer graphics, early investigators were limited to what they could depict in manual drawings, so lacked the means to visualize the beauty and appreciate some of the implications of many of the patterns they had discovered (the Julia set, for instance, could only be visualized through a few iterations as very simple drawings). That changed, however, in the 1960s, when
 Images of fractals can be created by fractal generating programs. Because of the butterfly effect, a small change in a single variable can have an unpredictable outcome.
* ''
Images of fractals can be created by fractal generating programs. Because of the butterfly effect, a small change in a single variable can have an unpredictable outcome.
* '' *'' Finite subdivision rules'' – use a recursive topological algorithm for refining tilingsJ. W. Cannon, W. J. Floyd, W. R. Parry. ''Finite subdivision rules''. Conformal Geometry and Dynamics, vol. 5 (2001), pp. 153–196. and they are similar to the process of cell division.J. W. Cannon, W. Floyd and W. Parry
*'' Finite subdivision rules'' – use a recursive topological algorithm for refining tilingsJ. W. Cannon, W. J. Floyd, W. R. Parry. ''Finite subdivision rules''. Conformal Geometry and Dynamics, vol. 5 (2001), pp. 153–196. and they are similar to the process of cell division.J. W. Cannon, W. Floyd and W. Parry
''Crystal growth, biological cell growth and geometry''.
Pattern Formation in Biology, Vision and Dynamics, pp. 65–82. World Scientific, 2000. , . The iterative processes used in creating the
File:Frost patterns 2.jpg, Frost crystals occurring naturally on cold glass form fractal patterns
File:Optical Billiard Spheres dsweet.jpeg, Fractal basin boundary in a geometrical optical system
File:Glue1 800x600.jpg, A fractal is formed when pulling apart two glue-covered
File:Animated fractal mountain.gif, A fractal that models the surface of a mountain (animation)
File:FRACTAL-3d-FLOWER.jpg, 3D recursive image
File:Fractal-BUTTERFLY.jpg, Recursive fractal butterfly image
File:Apophysis-100303-104.jpg, A
''Exploring Fractals on the Macintosh''
Addison Wesley, 1995. * Lesmoir-Gordon, Nigel; ''The Colours of Infinity: The Beauty, The Power and the Sense of Fractals''. 2004. (The book comes with a related DVD of the
Hunting the Hidden Dimension
PBS ''
Benoit Mandelbrot: Fractals and the Art of Roughness
,
Technical Library on Fractals for controlling fluid
Equations of self-similar fractal measure based on the fractional-order calculus
��2007) {{Authority control Mathematical structures Topology Computational fields of study

 In
In mathematics
Mathematics is an area of knowledge that includes the topics of numbers, formulas and related structures, shapes and the spaces in which they are contained, and quantities and their changes. These topics are represented in modern mathematics ...
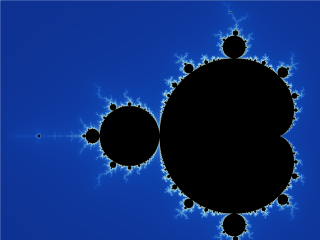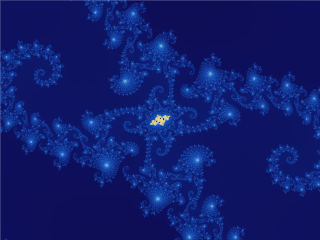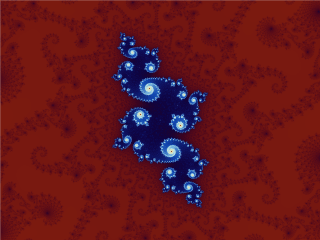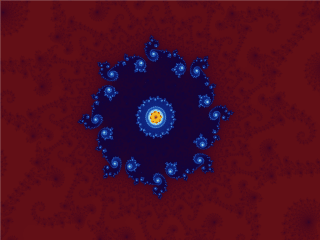
, a fractal is a geometric shape containing detailed structure at arbitrarily small scales, usually having a fractal dimension strictly exceeding the topological dimension. Many fractals appear similar at various scales, as illustrated in successive magnifications of the Mandelbrot set. This exhibition of similar patterns at increasingly smaller scales is called self-similarity, also known as expanding symmetry or unfolding symmetry; if this replication is exactly the same at every scale, as in the Menger sponge, the shape is called affine self-similar. Fractal geometry lies within the mathematical branch of measure theory
In mathematics, the concept of a measure is a generalization and formalization of geometrical measures ( length, area, volume) and other common notions, such as mass and probability of events. These seemingly distinct concepts have many simil ...
.
One way that fractals are different from finite geometric figures is how they scale
Scale or scales may refer to:
Mathematics
* Scale (descriptive set theory), an object defined on a set of points
* Scale (ratio), the ratio of a linear dimension of a model to the corresponding dimension of the original
* Scale factor, a number ...
. Doubling the edge lengths of a filled polygon multiplies its area by four, which is two (the ratio of the new to the old side length) raised to the power of two (the conventional dimension of the filled polygon). Likewise, if the radius of a filled sphere is doubled, its volume scales by eight, which is two (the ratio of the new to the old radius) to the power of three (the conventional dimension of the filled sphere). However, if a fractal's one-dimensional lengths are all doubled, the spatial content of the fractal scales by a power that is not necessarily an integer and is in general greater than its conventional dimension. This power is called the fractal dimension of the geometric object, to distinguish it from the conventional dimension (which is formally called the topological dimension).
Analytically, many fractals are nowhere differentiable. An infinite fractal curve can be conceived of as winding through space differently from an ordinary line – although it is still topologically 1-dimensional, its fractal dimension indicates that it locally fills space more efficiently than an ordinary line.
continuous
Continuity or continuous may refer to:
Mathematics
* Continuity (mathematics), the opposing concept to discreteness; common examples include
** Continuous probability distribution or random variable in probability and statistics
** Continuous ...
but not differentiable functions in the 19th century by the seminal work of Bernard Bolzano, Bernhard Riemann
Georg Friedrich Bernhard Riemann (; 17 September 1826 – 20 July 1866) was a German mathematician who made contributions to analysis, number theory, and differential geometry. In the field of real analysis, he is mostly known for the first rig ...
, and Karl Weierstrass, and on to the coining of the word ''fractal
In mathematics, a fractal is a geometric shape containing detailed structure at arbitrarily small scales, usually having a fractal dimension strictly exceeding the topological dimension. Many fractals appear similar at various scales, as illu ...
'' in the 20th century with a subsequent burgeoning of interest in fractals and computer-based modelling in the 20th century.
There is some disagreement among mathematicians about how the concept of a fractal should be formally defined. Mandelbrot himself summarized it as "beautiful, damn hard, increasingly useful. That's fractals." More formally, in 1982 Mandelbrot defined ''fractal'' as follows: "A fractal is by definition a set for which the Hausdorff–Besicovitch dimension
In mathematics, Hausdorff dimension is a measure of ''roughness'', or more specifically, fractal dimension, that was first introduced in 1918 by mathematician Felix Hausdorff. For instance, the Hausdorff dimension of a single point is zero, of a ...
strictly exceeds the topological dimension." Later, seeing this as too restrictive, he simplified and expanded the definition to this: "A fractal is a rough or fragmented geometric shape
A shape or figure is a graphical representation of an object or its external boundary, outline, or external surface, as opposed to other properties such as color, texture, or material type.
A plane shape or plane figure is constrained to lie on ...
that can be split into parts, each of which is (at least approximately) a reduced-size copy of the whole." Still later, Mandelbrot proposed "to use ''fractal'' without a pedantic definition, to use '' fractal dimension'' as a generic term applicable to ''all'' the variants".
The consensus among mathematicians is that theoretical fractals are infinitely self-similar iterated
Iteration is the repetition of a process in order to generate a (possibly unbounded) sequence of outcomes. Each repetition of the process is a single iteration, and the outcome of each iteration is then the starting point of the next iteration. ...
and detailed mathematical constructs, of which many examples have been formulated and studied. Fractals are not limited to geometric patterns, but can also describe processes in time. Fractal patterns with various degrees of self-similarity have been rendered or studied in visual, physical, and aural media and found in nature, technology, art, architectureOstwald, Michael J., and Vaughan, Josephine (2016) ''The Fractal Dimension of Architecture
''The Fractal Dimension of Architecture'' is a book that applies the mathematical concept of fractal dimension to the analysis of the architecture of buildings. It was written by Michael J. Ostwald and Josephine Vaughan, both of whom are architec ...
''. Birhauser, Basel. . and law. Fractals are of particular relevance in the field of chaos theory
Chaos theory is an interdisciplinary area of scientific study and branch of mathematics focused on underlying patterns and deterministic laws of dynamical systems that are highly sensitive to initial conditions, and were once thought to have co ...
because they show up in the geometric depictions of most chaotic processes (typically either as attractors or as boundaries between basins of attraction).
Etymology
The term "fractal" was coined by the mathematician Benoît Mandelbrot in 1975. Mandelbrot based it on the Latin , meaning "broken" or "fractured", and used it to extend the concept of theoretical fractional dimensions to geometric patterns in nature.Introduction

 The word "fractal" often has different connotations for the lay public as opposed to mathematicians, where the public is more likely to be familiar with
The word "fractal" often has different connotations for the lay public as opposed to mathematicians, where the public is more likely to be familiar with fractal art
Fractal art is a form of algorithmic art created by calculating fractal objects and representing the calculation results as still digital images, animations, and media. Fractal art developed from the mid-1980s onwards. It is a genre of computer ...
than the mathematical concept. The mathematical concept is difficult to define formally, even for mathematicians, but key features can be understood with a little mathematical background.
The feature of "self-similarity", for instance, is easily understood by analogy to zooming in with a lens or other device that zooms in on digital images to uncover finer, previously invisible, new structure. If this is done on fractals, however, no new detail appears; nothing changes and the same pattern repeats over and over, or for some fractals, nearly the same pattern reappears over and over. Self-similarity itself is not necessarily counter-intuitive (e.g., people have pondered self-similarity informally such as in the infinite regress in parallel mirrors or the homunculus, the little man inside the head of the little man inside the head ...). The difference for fractals is that the pattern reproduced must be detailed.
This idea of being detailed relates to another feature that can be understood without much mathematical background: Having a fractal dimension greater than its topological dimension, for instance, refers to how a fractal scales compared to how geometric shapes are usually perceived. A straight line, for instance, is conventionally understood to be one-dimensional; if such a figure is rep-tiled into pieces each 1/3 the length of the original, then there are always three equal pieces. A solid square is understood to be two-dimensional; if such a figure is rep-tiled into pieces each scaled down by a factor of 1/3 in both dimensions, there are a total of 32 = 9 pieces.
We see that for ordinary self-similar objects, being n-dimensional means that when it is rep-tiled into pieces each scaled down by a scale-factor of 1/''r'', there are a total of ''r''''n'' pieces. Now, consider the Koch curve. It can be rep-tiled into four sub-copies, each scaled down by a scale-factor of 1/3. So, strictly by analogy, we can consider the "dimension" of the Koch curve as being the unique real number ''D'' that satisfies 3''D'' = 4. This number is what mathematicians call the ''fractal dimension'' of the Koch curve; it is certainly ''not'' what is conventionally perceived as the dimension of a curve (this number is not even an integer!). In general, a key property of fractals is that the fractal dimension differs from the ''conventionally understood'' dimension (formally called the topological dimension).
 This also leads to understanding a third feature, that fractals as mathematical equations are "nowhere differentiable". In a concrete sense, this means fractals cannot be measured in traditional ways. To elaborate, in trying to find the length of a wavy non-fractal curve, one could find straight segments of some measuring tool small enough to lay end to end over the waves, where the pieces could get small enough to be considered to conform to the curve in the normal manner of measuring with a tape measure. But in measuring an infinitely "wiggly" fractal curve such as the Koch snowflake, one would never find a small enough straight segment to conform to the curve, because the jagged pattern would always re-appear, at arbitrarily small scales, essentially pulling a little more of the tape measure into the total length measured each time one attempted to fit it tighter and tighter to the curve. The result is that one must need infinite tape to perfectly cover the entire curve, i.e. the snowflake has an infinite perimeter.
This also leads to understanding a third feature, that fractals as mathematical equations are "nowhere differentiable". In a concrete sense, this means fractals cannot be measured in traditional ways. To elaborate, in trying to find the length of a wavy non-fractal curve, one could find straight segments of some measuring tool small enough to lay end to end over the waves, where the pieces could get small enough to be considered to conform to the curve in the normal manner of measuring with a tape measure. But in measuring an infinitely "wiggly" fractal curve such as the Koch snowflake, one would never find a small enough straight segment to conform to the curve, because the jagged pattern would always re-appear, at arbitrarily small scales, essentially pulling a little more of the tape measure into the total length measured each time one attempted to fit it tighter and tighter to the curve. The result is that one must need infinite tape to perfectly cover the entire curve, i.e. the snowflake has an infinite perimeter.
History
 The history of fractals traces a path from chiefly theoretical studies to modern applications in computer graphics, with several notable people contributing canonical fractal forms along the way.
A common theme in traditional African architecture is the use of fractal scaling, whereby small parts of the structure tend to look similar to larger parts, such as a circular village made of circular houses.
According to Pickover, the mathematics behind fractals began to take shape in the 17th century when the mathematician and philosopher Gottfried Leibniz pondered recursive self-similarity (although he made the mistake of thinking that only the straight line was self-similar in this sense).
In his writings, Leibniz used the term "fractional exponents", but lamented that "Geometry" did not yet know of them. Indeed, according to various historical accounts, after that point few mathematicians tackled the issues and the work of those who did remained obscured largely because of resistance to such unfamiliar emerging concepts, which were sometimes referred to as mathematical "monsters". Thus, it was not until two centuries had passed that on July 18, 1872 Karl Weierstrass presented the first definition of a function with a graph that would today be considered a fractal, having the non-
The history of fractals traces a path from chiefly theoretical studies to modern applications in computer graphics, with several notable people contributing canonical fractal forms along the way.
A common theme in traditional African architecture is the use of fractal scaling, whereby small parts of the structure tend to look similar to larger parts, such as a circular village made of circular houses.
According to Pickover, the mathematics behind fractals began to take shape in the 17th century when the mathematician and philosopher Gottfried Leibniz pondered recursive self-similarity (although he made the mistake of thinking that only the straight line was self-similar in this sense).
In his writings, Leibniz used the term "fractional exponents", but lamented that "Geometry" did not yet know of them. Indeed, according to various historical accounts, after that point few mathematicians tackled the issues and the work of those who did remained obscured largely because of resistance to such unfamiliar emerging concepts, which were sometimes referred to as mathematical "monsters". Thus, it was not until two centuries had passed that on July 18, 1872 Karl Weierstrass presented the first definition of a function with a graph that would today be considered a fractal, having the non-intuitive
Intuition is the ability to acquire knowledge without recourse to conscious reasoning. Different fields use the word "intuition" in very different ways, including but not limited to: direct access to unconscious knowledge; unconscious cognition; ...
property of being everywhere continuous
Continuity or continuous may refer to:
Mathematics
* Continuity (mathematics), the opposing concept to discreteness; common examples include
** Continuous probability distribution or random variable in probability and statistics
** Continuous ...
but nowhere differentiable at the Royal Prussian Academy of Sciences.
In addition, the quotient difference becomes arbitrarily large as the summation index increases. Not long after that, in 1883, Georg Cantor, who attended lectures by Weierstrass, published examples of subset
In mathematics, Set (mathematics), set ''A'' is a subset of a set ''B'' if all Element (mathematics), elements of ''A'' are also elements of ''B''; ''B'' is then a superset of ''A''. It is possible for ''A'' and ''B'' to be equal; if they are ...
s of the real line known as Cantor set
In mathematics, the Cantor set is a set of points lying on a single line segment that has a number of unintuitive properties. It was discovered in 1874 by Henry John Stephen Smith and introduced by German mathematician Georg Cantor in 1883.
Thr ...
s, which had unusual properties and are now recognized as fractals. Also in the last part of that century, Felix Klein and Henri Poincaré
Jules Henri Poincaré ( S: stress final syllable ; 29 April 1854 – 17 July 1912) was a French mathematician, theoretical physicist, engineer, and philosopher of science. He is often described as a polymath, and in mathematics as "The ...
introduced a category of fractal that has come to be called "self-inverse" fractals.

 One of the next milestones came in 1904, when
One of the next milestones came in 1904, when Helge von Koch
Niels Fabian Helge von Koch (25 January 1870 – 11 March 1924) was a Swedish mathematician who gave his name to the famous fractal known as the Koch snowflake, one of the earliest fractal curves to be described.
He was born to Swedish nobility. ...
, extending ideas of Poincaré and dissatisfied with Weierstrass's abstract and analytic definition, gave a more geometric definition including hand-drawn images of a similar function, which is now called the Koch snowflake. Another milestone came a decade later in 1915, when Wacław Sierpiński
Wacław Franciszek Sierpiński (; 14 March 1882 – 21 October 1969) was a Polish mathematician. He was known for contributions to set theory (research on the axiom of choice and the continuum hypothesis), number theory, theory of functions, and to ...
constructed his famous triangle then, one year later, his carpet. By 1918, two French mathematicians, Pierre Fatou
Pierre Joseph Louis Fatou (28 February 1878 – 9 August 1929) was a French mathematician and astronomer. He is known for major contributions to several branches of analysis. The Fatou lemma and the Fatou set are named after him.
Biography
P ...
and Gaston Julia, though working independently, arrived essentially simultaneously at results describing what is now seen as fractal behaviour associated with mapping complex numbers and iterative functions and leading to further ideas about attractors and repellors (i.e., points that attract or repel other points), which have become very important in the study of fractals.
Very shortly after that work was submitted, by March 1918, Felix Hausdorff expanded the definition of "dimension", significantly for the evolution of the definition of fractals, to allow for sets to have non-integer dimensions. The idea of self-similar curves was taken further by Paul Lévy, who, in his 1938 paper ''Plane or Space Curves and Surfaces Consisting of Parts Similar to the Whole'', described a new fractal curve, the Lévy C curve.


 Different researchers have postulated that without the aid of modern computer graphics, early investigators were limited to what they could depict in manual drawings, so lacked the means to visualize the beauty and appreciate some of the implications of many of the patterns they had discovered (the Julia set, for instance, could only be visualized through a few iterations as very simple drawings). That changed, however, in the 1960s, when
Different researchers have postulated that without the aid of modern computer graphics, early investigators were limited to what they could depict in manual drawings, so lacked the means to visualize the beauty and appreciate some of the implications of many of the patterns they had discovered (the Julia set, for instance, could only be visualized through a few iterations as very simple drawings). That changed, however, in the 1960s, when Benoit Mandelbrot
Benoit B. Mandelbrot (20 November 1924 – 14 October 2010) was a Polish-born French-American mathematician and polymath with broad interests in the practical sciences, especially regarding what he labeled as "the art of roughness" of phy ...
started writing about self-similarity in papers such as '' How Long Is the Coast of Britain? Statistical Self-Similarity and Fractional Dimension'', which built on earlier work by Lewis Fry Richardson
Lewis Fry Richardson, FRS (11 October 1881 – 30 September 1953) was an English mathematician, physicist, meteorologist, psychologist, and pacifist who pioneered modern mathematical techniques of weather forecasting, and the application of si ...
.
In 1975 Mandelbrot solidified hundreds of years of thought and mathematical development in coining the word "fractal" and illustrated his mathematical definition with striking computer-constructed visualizations. These images, such as of his canonical Mandelbrot set, captured the popular imagination; many of them were based on recursion, leading to the popular meaning of the term "fractal".
In 1980, Loren Carpenter
Loren C. Carpenter (born February 7, 1947) is a computer graphics researcher and developer.
Biography
He was a co-founder and chief scientist of Pixar Animation Studios. He is the co-inventor of the Reyes rendering algorithm and is one of the ...
gave a presentation at the SIGGRAPH
SIGGRAPH (Special Interest Group on Computer Graphics and Interactive Techniques) is an annual conference on computer graphics (CG) organized by the ACM SIGGRAPH, starting in 1974. The main conference is held in North America; SIGGRAPH Asia ...
where he introduced his software for generating and rendering fractally generated landscapes.
Definition and characteristics
One often cited description that Mandelbrot published to describe geometric fractals is "a rough or fragmentedgeometric shape
A shape or figure is a graphical representation of an object or its external boundary, outline, or external surface, as opposed to other properties such as color, texture, or material type.
A plane shape or plane figure is constrained to lie on ...
that can be split into parts, each of which is (at least approximately) a reduced-size copy of the whole"; this is generally helpful but limited. Authors disagree on the exact definition of ''fractal'', but most usually elaborate on the basic ideas of self-similarity and the unusual relationship fractals have with the space they are embedded in.
One point agreed on is that fractal patterns are characterized by fractal dimensions, but whereas these numbers quantify complexity
Complexity characterises the behaviour of a system or model whose components interaction, interact in multiple ways and follow local rules, leading to nonlinearity, randomness, collective dynamics, hierarchy, and emergence.
The term is generall ...
(i.e., changing detail with changing scale), they neither uniquely describe nor specify details of how to construct particular fractal patterns. In 1975 when Mandelbrot coined the word "fractal", he did so to denote an object whose Hausdorff–Besicovitch dimension
In mathematics, Hausdorff dimension is a measure of ''roughness'', or more specifically, fractal dimension, that was first introduced in 1918 by mathematician Felix Hausdorff. For instance, the Hausdorff dimension of a single point is zero, of a ...
is greater than its topological dimension. However, this requirement is not met by space-filling curves such as the Hilbert curve.
Because of the trouble involved in finding one definition for fractals, some argue that fractals should not be strictly defined at all. According to Falconer, fractals should be only generally characterized by a gestalt of the following features;
* Self-similarity, which may include:
:* Exact self-similarity: identical at all scales, such as the Koch snowflake
:* Quasi self-similarity: approximates the same pattern at different scales; may contain small copies of the entire fractal in distorted and degenerate forms; e.g., the Mandelbrot set's satellites are approximations of the entire set, but not exact copies.
:* Statistical self-similarity: repeats a pattern stochastic
Stochastic (, ) refers to the property of being well described by a random probability distribution. Although stochasticity and randomness are distinct in that the former refers to a modeling approach and the latter refers to phenomena themselv ...
ally so numerical or statistical measures are preserved across scales; e.g., randomly generated fractals like the well-known example of the coastline of Britain for which one would not expect to find a segment scaled and repeated as neatly as the repeated unit that defines fractals like the Koch snowflake.
:* Qualitative self-similarity: as in a time series
:* Multifractal scaling: characterized by more than one fractal dimension or scaling rule
* Fine or detailed structure at arbitrarily small scales. A consequence of this structure is fractals may have emergent properties
In philosophy, systems theory, science, and art, emergence occurs when an entity is observed to have properties its parts do not have on their own, properties or behaviors that emerge only when the parts interact in a wider whole.
Emergence ...
(related to the next criterion in this list).
* Irregularity locally and globally that cannot easily be described in the language of traditional Euclidean geometry other than as the limit of a recursively defined sequence of stages. For images of fractal patterns, this has been expressed by phrases such as "smoothly piling up surfaces" and "swirls upon swirls";''see Common techniques for generating fractals''.
As a group, these criteria form guidelines for excluding certain cases, such as those that may be self-similar without having other typically fractal features. A straight line, for instance, is self-similar but not fractal because it lacks detail, and is easily described in Euclidean language without a need for recursion.
Common techniques for generating fractals
 Images of fractals can be created by fractal generating programs. Because of the butterfly effect, a small change in a single variable can have an unpredictable outcome.
* ''
Images of fractals can be created by fractal generating programs. Because of the butterfly effect, a small change in a single variable can have an unpredictable outcome.
* ''Iterated function systems
In mathematics, iterated function systems (IFSs) are a method of constructing fractals; the resulting fractals are often self-similar. IFS fractals are more related to set theory than fractal geometry. They were introduced in 1981.
IFS fractal ...
(IFS)'' – use fixed geometric replacement rules; may be stochastic or deterministic; e.g., Koch snowflake, Cantor set
In mathematics, the Cantor set is a set of points lying on a single line segment that has a number of unintuitive properties. It was discovered in 1874 by Henry John Stephen Smith and introduced by German mathematician Georg Cantor in 1883.
Thr ...
, Haferman carpet, Sierpinski carpet, Sierpinski gasket, Peano curve
In geometry, the Peano curve is the first example of a space-filling curve to be discovered, by Giuseppe Peano in 1890. Peano's curve is a surjective, continuous function from the unit interval onto the unit square, however it is not injective. ...
, Harter-Heighway dragon curve, T-square, Menger sponge
* ''Strange attractor
In the mathematical field of dynamical systems, an attractor is a set of states toward which a system tends to evolve, for a wide variety of starting conditions of the system. System values that get close enough to the attractor values remain ...
s'' – use iterations of a map or solutions of a system of initial-value differential or difference equations that exhibit chaos (e.g., see multifractal image, or the logistic map
The logistic map is a polynomial mapping (equivalently, recurrence relation) of degree 2, often referred to as an archetypal example of how complex, chaotic behaviour can arise from very simple non-linear dynamical equations. The map was popular ...
)
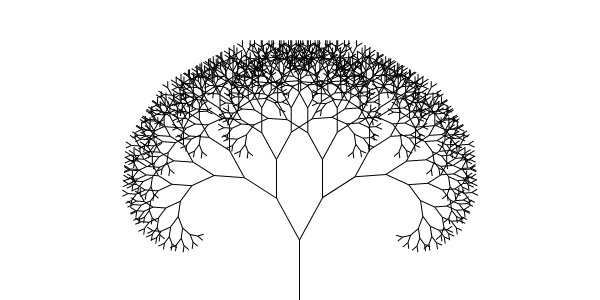
* '' L-systems'' – use string rewriting; may resemble branching patterns, such as in plants, biological cells (e.g., neurons and immune system cells), blood vessels, pulmonary structure, etc. or turtle graphics patterns such as space-filling curves
In mathematical analysis, a space-filling curve is a curve whose range contains the entire 2-dimensional unit square (or more generally an ''n''-dimensional unit hypercube). Because Giuseppe Peano (1858–1932) was the first to discover one, spac ...
and tilings
* ''Escape-time fractals'' – use a formula
In science, a formula is a concise way of expressing information symbolically, as in a mathematical formula or a ''chemical formula''. The informal use of the term ''formula'' in science refers to the general construct of a relationship betwee ...
or recurrence relation at each point in a space (such as the complex plane
In mathematics, the complex plane is the plane formed by the complex numbers, with a Cartesian coordinate system such that the -axis, called the real axis, is formed by the real numbers, and the -axis, called the imaginary axis, is formed by the ...
); usually quasi-self-similar; also known as "orbit" fractals; e.g., the Mandelbrot set, Julia set, Burning Ship fractal, Nova fractal
The Newton fractal is a boundary set in the complex plane which is characterized by Newton's method applied to a fixed polynomial or transcendental function. It is the Julia set of the meromorphic function which is given by Newton's method. ...
and Lyapunov fractal
In mathematics, Lyapunov fractals (also known as Markus–Lyapunov fractals) are bifurcational fractals derived from an extension of the logistic map in which the degree of the growth of the population, ''r'', periodically switches between ...
. The 2d vector fields that are generated by one or two iterations of escape-time formulae also give rise to a fractal form when points (or pixel data) are passed through this field repeatedly.
* ''Random fractals'' – use stochastic rules; e.g., Lévy flight
A Lévy flight is a random walk in which the step-lengths have a Lévy distribution, a probability distribution that is heavy-tailed. When defined as a walk in a space of dimension greater than one, the steps made are in isotropic random direct ...
, percolation clusters, self avoiding walks, fractal landscapes
A fractal landscape is a surface that is generated using a stochastic algorithm designed to produce fractal behavior that mimics the appearance of natural terrain. In other words, the result of the procedure is not a deterministic fractal surface, ...
, trajectories of Brownian motion and the Brownian tree
In probability theory, the Brownian tree, or Aldous tree, or Continuum Random Tree (CRT) is a special case from random real trees which may be defined from a Brownian excursion. The Brownian tree was defined and studied by David Aldous in three a ...
(i.e., dendritic fractals generated by modeling diffusion-limited aggregation
Diffusion-limited aggregation (DLA) is the process whereby particles undergoing a random walk due to Brownian motion cluster together to form aggregates of such particles. This theory, proposed by T.A. Witten Jr. and L.M. Sander in 1981, is app ...
or reaction-limited aggregation clusters).
 *'' Finite subdivision rules'' – use a recursive topological algorithm for refining tilingsJ. W. Cannon, W. J. Floyd, W. R. Parry. ''Finite subdivision rules''. Conformal Geometry and Dynamics, vol. 5 (2001), pp. 153–196. and they are similar to the process of cell division.J. W. Cannon, W. Floyd and W. Parry
*'' Finite subdivision rules'' – use a recursive topological algorithm for refining tilingsJ. W. Cannon, W. J. Floyd, W. R. Parry. ''Finite subdivision rules''. Conformal Geometry and Dynamics, vol. 5 (2001), pp. 153–196. and they are similar to the process of cell division.J. W. Cannon, W. Floyd and W. Parry''Crystal growth, biological cell growth and geometry''.
Pattern Formation in Biology, Vision and Dynamics, pp. 65–82. World Scientific, 2000. , . The iterative processes used in creating the
Cantor set
In mathematics, the Cantor set is a set of points lying on a single line segment that has a number of unintuitive properties. It was discovered in 1874 by Henry John Stephen Smith and introduced by German mathematician Georg Cantor in 1883.
Thr ...
and the Sierpinski carpet are examples of finite subdivision rules, as is barycentric subdivision.
Applications
Simulated fractals
Fractal patterns have been modeled extensively, albeit within a range of scales rather than infinitely, owing to the practical limits of physical time and space. Models may simulate theoretical fractals or natural phenomena with fractal features. The outputs of the modelling process may be highly artistic renderings, outputs for investigation, or benchmarks for fractal analysis. Some specific applications of fractals to technology are listed elsewhere. Images and other outputs of modelling are normally referred to as being "fractals" even if they do not have strictly fractal characteristics, such as when it is possible to zoom into a region of the fractal image that does not exhibit any fractal properties. Also, these may include calculation or display artifacts which are not characteristics of true fractals. Modeled fractals may be sounds, digital images, electrochemical patterns,circadian rhythm
A circadian rhythm (), or circadian cycle, is a natural, internal process that regulates the sleep–wake cycle and repeats roughly every 24 hours. It can refer to any process that originates within an organism (i.e., Endogeny (biology), endogeno ...
s, etc.
Fractal patterns have been reconstructed in physical 3-dimensional space and virtually, often called "in silico
In biology and other experimental sciences, an ''in silico'' experiment is one performed on computer or via computer simulation. The phrase is pseudo-Latin for 'in silicon' (correct la, in silicio), referring to silicon in computer chips. It ...
" modeling. Models of fractals are generally created using fractal-generating software that implements techniques such as those outlined above. As one illustration, trees, ferns, cells of the nervous system, blood and lung vasculature, and other branching patterns in nature can be modeled on a computer by using recursive algorithms and L-systems
An L-system or Lindenmayer system is a parallel rewriting system and a type of formal grammar. An L-system consists of an alphabet of symbols that can be used to make strings, a collection of production rules that expand each symbol into some ...
techniques.
The recursive nature of some patterns is obvious in certain examples—a branch from a tree or a frond from a fern is a miniature replica of the whole: not identical, but similar in nature. Similarly, random fractals have been used to describe/create many highly irregular real-world objects. A limitation of modeling fractals is that resemblance of a fractal model to a natural phenomenon does not prove that the phenomenon being modeled is formed by a process similar to the modeling algorithms.
Natural phenomena with fractal features
Approximate fractals found in nature display self-similarity over extended, but finite, scale ranges. The connection between fractals and leaves, for instance, is currently being used to determine how much carbon is contained in trees. Phenomena known to have fractal features include: * Actin cytoskeleton * Algae *Animal coloration
Animal coloration is the general appearance of an animal resulting from the reflection or emission of light from its surfaces. Some animals are brightly coloured, while others are hard to see. In some species, such as the peafowl, the male h ...
patterns
* Blood vessels and pulmonary vessels
The pulmonary circulation is a division of the circulatory system in all vertebrates. The circuit begins with deoxygenated blood returned from the body to the right atrium of the heart where it is pumped out from the right ventricle to the lungs. ...
* Brownian motion (generated by a one-dimensional Wiener process).
* Clouds and rainfall areas
* Coastlines
* Craters
* Crystals
* DNA
* Dust grains
* Earthquakes
An earthquake (also known as a quake, tremor or temblor) is the shaking of the surface of the Earth resulting from a sudden release of energy in the Earth's lithosphere that creates seismic waves. Earthquakes can range in intensity, from ...
* Fault lines
* Geometrical optics
* Heart rates
* Heart sounds
Heart sounds are the noises generated by the beating heart and the resultant flow of blood through it. Specifically, the sounds reflect the turbulence created when the heart valves snap shut. In cardiac auscultation, an examiner may use a stetho ...
* Lake shorelines and areas
* Lightning bolts
* Mountain goat horns
* Polymers
* Percolation
* Mountain ranges
* Ocean waves
In fluid dynamics, a wind wave, water wave, or wind-generated water wave, is a surface wave that occurs on the free surface of bodies of water as a result from the wind blowing over the water surface. The contact distance in the direction of t ...
* Pineapple
* Proteins
Proteins are large biomolecules and macromolecules that comprise one or more long chains of amino acid residues. Proteins perform a vast array of functions within organisms, including catalysing metabolic reactions, DNA replication, respo ...
* Psychedelic Experience
A psychedelic experience (known colloquially as a trip) is a temporary altered state of consciousness induced by the consumption of a psychedelic substance (most commonly LSD, mescaline, psilocybin mushrooms, or DMT). For example, an acid tr ...
* Purkinje cells
* Rings of Saturn
* River networks
* Romanesco broccoli
Romanesco broccoli (also known as Roman cauliflower, Broccolo Romanesco, Romanesque cauliflower, Romanesco or broccoflower) is an edible flower bud of the species ''Brassica oleracea''. It is chartreuse in color, and has a form naturally approx ...
* Snowflakes
* Soil pores
*Surfaces in turbulent flows
* Trees
acrylic
Acrylic may refer to:
Chemicals and materials
* Acrylic acid, the simplest acrylic compound
* Acrylate polymer, a group of polymers (plastics) noted for transparency and elasticity
* Acrylic resin, a group of related thermoplastic or thermosett ...
sheets
File:Square1.jpg, High-voltage breakdown within a block of acrylic glass creates a fractal Lichtenberg figure
A Lichtenberg figure (German ''Lichtenberg-Figuren''), or Lichtenberg dust figure, is a branching electric discharge that sometimes appears on the surface or in the interior of insulating materials. Lichtenberg figures are often associated wit ...
File:Romanesco broccoli (Brassica oleracea).jpg, Romanesco broccoli
Romanesco broccoli (also known as Roman cauliflower, Broccolo Romanesco, Romanesque cauliflower, Romanesco or broccoflower) is an edible flower bud of the species ''Brassica oleracea''. It is chartreuse in color, and has a form naturally approx ...
, showing self-similar
__NOTOC__
In mathematics, a self-similar object is exactly or approximately similar to a part of itself (i.e., the whole has the same shape as one or more of the parts). Many objects in the real world, such as coastlines, are statistically se ...
form approximating a natural fractal
File:Fractal defrosting patterns on Mars.jpg, Fractal defrosting patterns, polar Mars. The patterns are formed by sublimation of frozen CO2. Width of image is about a kilometer.
File:Brefeldia maxima plasmodium on wood.jpg, Slime mold ''Brefeldia maxima
''Brefeldia maxima'' is a species of non-parasitic plasmodial slime mold, and a member of the class Myxogastria, Myxomycetes. It is common name, commonly known as the tapioca slime mold because of its peculiar pure white, tapioca pudding-like app ...
'' growing fractally on wood
Fractals in cell biology
Fractals often appear in the realm of living organisms where they arise through branching processes and other complex pattern formation. Ian Wong and co-workers have shown that migrating cells can form fractals by clustering and branching. Nerve cells function through processes at the cell surface, with phenomena that are enhanced by largely increasing the surface to volume ratio. As a consequence nerve cells often are found to form into fractal patterns. These processes are crucial in cell physiology and different pathologies. Multiple subcellular structures also are found to assemble into fractals.Diego Krapf
Diego Krapf (born November 21, 1973) is an Argentine-Israeli-American physicist known for his work on anomalous diffusion and ergodicity breaking. He currently is a professor in the Department of Electrical and Computer Engineering at Colorado St ...
has shown that through branching processes the actin filaments in human cells assemble into fractal patterns. Similarly Matthias Weiss showed that the endoplasmic reticulum
The endoplasmic reticulum (ER) is, in essence, the transportation system of the eukaryotic cell, and has many other important functions such as protein folding. It is a type of organelle made up of two subunits – rough endoplasmic reticulum ( ...
displays fractal features. The current understanding is that fractals are ubiquitous in cell biology, from proteins, to organelle
In cell biology, an organelle is a specialized subunit, usually within a cell, that has a specific function. The name ''organelle'' comes from the idea that these structures are parts of cells, as organs are to the body, hence ''organelle,'' the ...
s, to whole cells.
In creative works
Since 1999 numerous scientific groups have performed fractal analysis on over 50 paintings created by Jackson Pollock by pouring paint directly onto horizontal canvasses. Recently, fractal analysis has been used to achieve a 93% success rate in distinguishing real from imitation Pollocks. Cognitive neuroscientists have shown that Pollock's fractals induce the same stress-reduction in observers as computer-generated fractals and Nature's fractals. Decalcomania, a technique used by artists such as Max Ernst, can produce fractal-like patterns. It involves pressing paint between two surfaces and pulling them apart. CyberneticistRon Eglash
Ron Eglash (born December 25, 1958 in Chestertown, Maryland) is an American who works in cybernetics, professor in the School of Information at the University of Michigan with a secondary appointment in the School of Design, and an author widely ...
has suggested that fractal geometry and mathematics are prevalent in African art, games, divination
Divination (from Latin ''divinare'', 'to foresee, to foretell, to predict, to prophesy') is the attempt to gain insight into a question or situation by way of an occultic, standardized process or ritual. Used in various forms throughout histor ...
, trade, and architecture. Circular houses appear in circles of circles, rectangular houses in rectangles of rectangles, and so on. Such scaling patterns can also be found in African textiles, sculpture, and even cornrow hairstyles. Hokky Situngkir
Hokky Situngkir (born February 7, 1978) is an Indonesian scientist who researches complexity theory at Surya University. He is the founder of the Bandung Fe Institute, a research institute for social complexity research. His academic activities ...
also suggested the similar properties in Indonesian traditional art, batik, and ornaments
An ornament is something used for decoration.
Ornament may also refer to:
Decoration
*Ornament (art), any purely decorative element in architecture and the decorative arts
*Biological ornament, a characteristic of animals that appear to serve on ...
found in traditional houses.
Ethnomathematician Ron Eglash has discussed the planned layout of Benin city
Benin City is the capital and largest city of Edo State, Edo State, Nigeria. It is the fourth-largest city in Nigeria according to the 2006 census, after Lagos, Kano (city), Kano, and Ibadan, with a population estimate of about 3,500,000 as of ...
using fractals as the basis, not only in the city itself and the villages but even in the rooms of houses. He commented that "When Europeans first came to Africa, they considered the architecture very disorganised and thus primitive. It never occurred to them that the Africans might have been using a form of mathematics that they hadn’t even discovered yet."
In a 1996 interview with Michael Silverblatt, David Foster Wallace admitted that the structure of the first draft of '' Infinite Jest'' he gave to his editor Michael Pietsch was inspired by fractals, specifically the Sierpinski triangle (a.k.a. Sierpinski gasket), but that the edited novel is "more like a lopsided Sierpinsky Gasket".
Some works by the Dutch artist M. C. Escher
Maurits Cornelis Escher (; 17 June 1898 – 27 March 1972) was a Dutch graphic artist who made mathematically inspired woodcuts, lithographs, and mezzotints.
Despite wide popular interest, Escher was for most of his life neglected in t ...
, such as Circle Limit III
''Circle Limit III'' is a woodcut made in 1959 by Dutch artist M. C. Escher, in which "strings of fish shoot up like rockets from infinitely far away" and then "fall back again whence they came".Escher, as quoted by .
It is one of a series of fo ...
, contain shapes repeated to infinity that become smaller and smaller as they get near to the edges, in a pattern that would always look the same if zoomed in.
fractal flame
Fractal flames are a member of the iterated function system class of fractals created by Scott Draves in 1992. Draves' open-source code was later ported into Adobe After Effects graphics softwareChris Gehman and Steve Reinke (2005). ''The Sharp ...
Physiological responses
Humans appear to be especially well-adapted to processing fractal patterns with D values between 1.3 and 1.5. When humans view fractal patterns with D values between 1.3 and 1.5, this tends to reduce physiological stress.Applications in technology
*Fractal antenna
A fractal antenna is an antenna that uses a fractal, self-similar design to maximize the effective length, or increase the perimeter (on inside sections or the outer structure), of material that can receive or transmit electromagnetic radiation ...
s
*Fractal transistor
* Fractal heat exchangers
* Digital imaging
* Architecture
* Urban growth
* Classification Classification is a process related to categorization, the process in which ideas and objects are recognized, differentiated and understood.
Classification is the grouping of related facts into classes.
It may also refer to:
Business, organizat ...
of histopathology
Histopathology (compound of three Greek words: ''histos'' "tissue", πάθος ''pathos'' "suffering", and -λογία '' -logia'' "study of") refers to the microscopic examination of tissue in order to study the manifestations of disease. Spe ...
slides
* Fractal landscape
A fractal landscape is a surface that is generated using a stochastic algorithm designed to produce fractal behavior that mimics the appearance of natural terrain. In other words, the result of the procedure is not a deterministic fractal surface, ...
or Coastline complexity
Complexity characterises the behaviour of a system or model whose components interaction, interact in multiple ways and follow local rules, leading to nonlinearity, randomness, collective dynamics, hierarchy, and emergence.
The term is generall ...
* Detecting 'life as we don't know it' by fractal analysis
* Enzymes ( Michaelis-Menten kinetics)
* Generation of new music
* Signal and image compression
Image compression is a type of data compression applied to digital images, to reduce their cost for storage or transmission. Algorithms may take advantage of visual perception and the statistical properties of image data to provide superior r ...
* Creation of digital photographic enlargements
* Fractal in soil mechanics
A fractal is an irregular geometric object with an infinite nesting of structure at all scales. It is mainly applicable in soil chromatography and soil micromorphology (Anderson, 1997). Internal structure, pore size distribution and pore geometry ...
* Computer and video game design
* Computer Graphics
* Organic
Organic may refer to:
* Organic, of or relating to an organism, a living entity
* Organic, of or relating to an anatomical organ
Chemistry
* Organic matter, matter that has come from a once-living organism, is capable of decay or is the product ...
environments
* Procedural generation
* Fractography
Fractography is the study of the fracture surfaces of materials. Fractographic methods are routinely used to determine the cause of failure in engineering structures, especially in product failure and the practice of forensic engineering or fa ...
and fracture mechanics
Fracture mechanics is the field of mechanics concerned with the study of the propagation of cracks in materials. It uses methods of analytical solid mechanics to calculate the driving force on a crack and those of experimental solid mechanics t ...
* Small angle scattering theory of fractally rough systems
* T-shirts and other fashion
* Generation of patterns for camouflage, such as MARPAT
* Digital sundial
A digital sundial is a clock that indicates the current time with numerals formed by the sunlight striking it. Like a classical sundial, the device contains no moving parts. It uses no electricity nor other manufactured sources of energy. The di ...
* Technical analysis of price series
* Fractals in networks
* Medicine
* Neuroscience
* Diagnostic Imaging
* Pathology
* Geology
* Geography
* Archaeology
* Soil mechanics
* Seismology
* Search and rescue
Search and rescue (SAR) is the search for and provision of aid to people who are in distress or imminent danger. The general field of search and rescue includes many specialty sub-fields, typically determined by the type of terrain the search ...
* Technical analysis
* Morton order space filling curves for GPU cache coherency in texture mapping, rasterisation and indexing of turbulence data.
See also
* Banach fixed point theorem * Bifurcation theory * Box counting *Cymatics
Cymatics (from grc, κῦμα, translit=kyma, translation=wave) is a subset of modal vibrational phenomena. The term was coined by Hans Jenny (1904-1972), a Swiss follower of the philosophical school known as anthroposophy. Typically the surf ...
*Determinism
Determinism is a philosophical view, where all events are determined completely by previously existing causes. Deterministic theories throughout the history of philosophy have developed from diverse and sometimes overlapping motives and consi ...
*Diamond-square algorithm
The diamond-square algorithm is a method for generating heightmaps for computer graphics. It is a slightly better algorithm than the three-dimensional implementation of the midpoint displacement algorithm, which produces two-dimensional landscap ...
*Droste effect
The Droste effect (), known in art as an example of ''mise en abyme'', is the effect of a picture recursively appearing within itself, in a place where a similar picture would realistically be expected to appear. This produces a loop which in ...
*Feigenbaum function In the study of dynamical systems the term Feigenbaum function has been used to describe two different functions introduced by the physicist Mitchell Feigenbaum:
* the solution to the Feigenbaum-Cvitanović functional equation; and
* the scaling fun ...
*Form constant
A form constant is one of several geometric patterns which are recurringly observed during hypnagogia, hallucinations and altered states of consciousness.
History
In 1926, Heinrich Klüver systematically studied the effects of mescaline (peyote ...
*Fractal cosmology
In physical cosmology, fractal cosmology is a set of minority cosmological theories which state that the distribution of matter in the Universe, or the structure of the universe itself, is a fractal across a wide range of scales (see also: multif ...
* Fractal derivative
* Fractalgrid
* Fractal string
*Fracton
A fracton is a collective quantized vibration on a substrate with a fractal structure..
Fractons are the fractal analog of phonons. Phonons are the result of applying translational symmetry to the potential in a Schrödinger equation. Fractal s ...
*Graftal
An L-system or Lindenmayer system is a parallel rewriting system and a type of formal grammar. An L-system consists of an alphabet of symbols that can be used to make strings, a collection of production rules that expand each symbol into some ...
* Greeble
* Infinite regress
* Lacunarity
* List of fractals by Hausdorff dimension
* Mandelbulb
* Mandelbox
* Macrocosm and microcosm
* Matryoshka doll
* Menger Sponge
* Multifractal system
*Newton fractal
The Newton fractal is a boundary set in the complex plane which is characterized by Newton's method applied to a fixed polynomial or transcendental function. It is the Julia set of the meromorphic function which is given by Newton's method. ...
* Percolation
*Power law
In statistics, a power law is a Function (mathematics), functional relationship between two quantities, where a Relative change and difference, relative change in one quantity results in a proportional relative change in the other quantity, inde ...
* Publications in fractal geometry
* Random walk
*Self-reference
Self-reference occurs in natural or formal languages when a sentence, idea or formula refers to itself. The reference may be expressed either directly—through some intermediate sentence or formula—or by means of some encoding. In philoso ...
* Self-similarity
* Systems theory
*Strange loop
A strange loop is a cyclic structure that goes through several levels in a hierarchical system. It arises when, by moving only upwards or downwards through the system, one finds oneself back where one started.
Strange loops may involve self-refe ...
* Turbulence
* Wiener process
Notes
References
Further reading
* Barnsley, Michael F.; and Rising, Hawley; ''Fractals Everywhere''. Boston: Academic Press Professional, 1993. * Duarte, German A.; ''Fractal Narrative. About the Relationship Between Geometries and Technology and Its Impact on Narrative Spaces''. Bielefeld: Transcript, 2014. * Falconer, Kenneth; ''Techniques in Fractal Geometry''. John Wiley and Sons, 1997. * Jürgens, Hartmut; Peitgen, Heinz-Otto; and Saupe, Dietmar; ''Chaos and Fractals: New Frontiers of Science''. New York: Springer-Verlag, 1992. * Mandelbrot, Benoit B.; ''The Fractal Geometry of Nature
''The Fractal Geometry of Nature'' is a 1982 book by the Franco-American mathematician Benoît Mandelbrot.
Overview
''The Fractal Geometry of Nature'' is a revised and enlarged version of his 1977 book entitled ''Fractals: Form, Chance and Dimen ...
''. New York: W. H. Freeman and Co., 1982.
* Peitgen, Heinz-Otto; and Saupe, Dietmar; eds.; ''The Science of Fractal Images''. New York: Springer-Verlag, 1988.
* Pickover, Clifford A.; ed.; ''Chaos and Fractals: A Computer Graphical Journey – A 10 Year Compilation of Advanced Research''. Elsevier, 1998.
* Jones, Jesse; ''Fractals for the Macintosh'', Waite Group Press, Corte Madera, CA, 1993. .
* Lauwerier, Hans; ''Fractals: Endlessly Repeated Geometrical Figures'', Translated by Sophia Gill-Hoffstadt, Princeton University Press, Princeton NJ, 1991. , cloth. paperback. "This book has been written for a wide audience..." Includes sample BASIC programs in an appendix.
*
* Wahl, Bernt; Van Roy, Peter; Larsen, Michael; and Kampman, Eric''Exploring Fractals on the Macintosh''
Addison Wesley, 1995. * Lesmoir-Gordon, Nigel; ''The Colours of Infinity: The Beauty, The Power and the Sense of Fractals''. 2004. (The book comes with a related DVD of the
Arthur C. Clarke
Sir Arthur Charles Clarke (16 December 191719 March 2008) was an English science-fiction writer, science writer, futurist, inventor, undersea explorer, and television series host.
He co-wrote the screenplay for the 1968 film '' 2001: A Spac ...
documentary introduction to the fractal concept and the Mandelbrot set.)
* Liu, Huajie; ''Fractal Art'', Changsha: Hunan Science and Technology Press, 1997, .
* Gouyet, Jean-François; ''Physics and Fractal Structures'' (Foreword by B. Mandelbrot); Masson, 1996. , and New York: Springer-Verlag, 1996. . Out-of-print. Available in PDF version at.
*
External links
*Hunting the Hidden Dimension
PBS ''
NOVA
A nova (plural novae or novas) is a transient astronomical event that causes the sudden appearance of a bright, apparently "new" star (hence the name "nova", which is Latin for "new") that slowly fades over weeks or months. Causes of the dramati ...
'', first aired August 24, 2011
Benoit Mandelbrot: Fractals and the Art of Roughness
,
TED
TED may refer to:
Economics and finance
* TED spread between U.S. Treasuries and Eurodollar
Education
* ''Türk Eğitim Derneği'', the Turkish Education Association
** TED Ankara College Foundation Schools, Turkey
** Transvaal Education Depa ...
, February 2010
Technical Library on Fractals for controlling fluid
Equations of self-similar fractal measure based on the fractional-order calculus
��2007) {{Authority control Mathematical structures Topology Computational fields of study