|
Circle Limit III
''Circle Limit III'' is a woodcut made in 1959 by Dutch artist M. C. Escher, in which "strings of fish shoot up like rockets from infinitely far away" and then "fall back again whence they came".Escher, as quoted by . It is one of a series of four woodcuts by Escher depicting ideas from hyperbolic geometry. Dutch physicist and mathematician Bruno Ernst called it "the best of the four".. Inspiration Escher became interested in tessellations of the plane after a 1936 visit to the Alhambra in Granada, Spain,.. and from the time of his 1937 artwork '' Metamorphosis I'' he had begun incorporating tessellated human and animal figures into his artworks. In a 1958 letter from Escher to H. S. M. Coxeter, Escher wrote that he was inspired to make his ''Circle Limit'' series by a figure in Coxeter's article "Crystal Symmetry and its Generalizations". Coxeter's figure depicts a tessellation of the hyperbolic plane In mathematics, hyperbolic geometry (also called Lobachevskian ge ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Escher Circle Limit III
Escher is a surname. Notable people with the surname include: * Alfred Escher (1819−1883), a Swiss politician and railway pioneer * Arnold Escher von der Linth (1807−1872), a Swiss geologist * Berend George Escher (1885−1967), a Dutch geologist, half-brother of M. C. Escher * George Arnold Escher (1843−1939), a Dutch civil engineer, foreign advisor to Japan, father of M. C. Escher * Gitta Escher (born 1957), a German gymnast * Hans Conrad Escher von der Linth (1767−1823), a Swiss scientist, civil engineer and politician * Heinrich Escher (1626−1710), a Swiss politician, mayor of Zürich * Josef Escher (1885−1954), a Swiss Federal Councilor * Luiz Jeferson Escher (born 1987), Brazilian footballer * Lydia Escher (1858−1891), a Swiss patron of the arts * M. C. Escher (Maurits Cornelis Escher; 1898−1972), a Dutch graphic artist * Rudolf George Escher (1912−1980), a Dutch composer and music theoretician * Sandra Escher (born 1945), a Dutch psychiatrist See also * ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Hypercycle (geometry)
In hyperbolic geometry, a hypercycle, hypercircle or equidistant curve is a curve whose points have the same orthogonal distance from a given straight line (its axis). Given a straight line and a point not on , one can construct a hypercycle by taking all points on the same side of as , with perpendicular distance to equal to that of . The line is called the ''axis'', ''center'', or ''base line'' of the hypercycle. The lines perpendicular to , which are also perpendicular to the hypercycle, are called the ''normals'' of the hypercycle. The segments of the normals between and the hypercycle are called the ''radii''. Their common length is called the ''distance'' or ''radius'' of the hypercycle. The hypercycles through a given point that share a tangent through that point converge towards a horocycle as their distances go towards infinity. Properties similar to those of Euclidean lines Hypercycles in hyperbolic geometry have some properties similar to those of lines in Eu ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Escher Museum
Escher in Het Paleis (''Escher in The Palace'') is a museum in The Hague, Netherlands, featuring the works of the Dutch graphical artist M. C. Escher. It is housed in the Lange Voorhout Palace since November 2002. In 2015 it was revealed that many of the prints on display at the museum were replicas, scanned from original prints and printed onto the same type of paper used by Escher, rather than original Escher prints as they had been labeled.. History The museum is housed in the Lange Voorhout Palace, a former royal residence dating back to the eighteenth century. Queen Emma bought the stately house in 1896. She used it as a winter palace from March 1901 until her death in March 1934. It was used by four subsequent Dutch queens for their business offices, until Queen Beatrix moved the office to Paleis Noordeinde. The first and second floors have exhibitions showing the royal period of the palace, highlighting Queen Emma's residence. The museum features a permanent display of a ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Taschen
Taschen is a luxury art book publisher founded in 1980 by Benedikt Taschen in Cologne, Germany. As of January 2017, Taschen is co-managed by Benedikt and his eldest daughter, Marlene Taschen. History The company began as Taschen Comics, publishing Benedikt's comic collection. Taschen focuses on making lesser-seen art available to mainstream bookstores, including fetishistic imagery, queer art, historical erotica, pornography, and adult magazines (including multiple books with ''Playboy'' magazine). The firm has brought potentially controversial art into broader public view, publishing it alongside its more mainstream books of comics reprints, art photography, painting, design, fashion, advertising history, film, and architecture.Degen Pener''Taschen Books Chief Reveals New Projects, Talks 'Fifty Shades' and $12M Books'' published in The Hollywood Reporter, 25 November 2014 Taschen publications are available in a various sizes, from oversized tomes to small pocket-sized ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Bilateral Symmetry
Symmetry in biology refers to the symmetry observed in organisms, including plants, animals, fungi, and bacteria. External symmetry can be easily seen by just looking at an organism. For example, take the face of a human being which has a plane of symmetry down its centre, or a pine cone with a clear symmetrical spiral pattern. Internal features can also show symmetry, for example the tubes in the human body (responsible for transporting gases, nutrients, and waste products) which are cylindrical and have several planes of symmetry. Biological symmetry can be thought of as a balanced distribution of duplicate body parts or shapes within the body of an organism. Importantly, unlike in mathematics, symmetry in biology is always approximate. For example, plant leaves – while considered symmetrical – rarely match up exactly when folded in half. Symmetry is one class of patterns in nature whereby there is near-repetition of the pattern element, either by reflection or rotation ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Orbifold Notation
In geometry, orbifold notation (or orbifold signature) is a system, invented by the mathematician William Thurston and promoted by John Conway, for representing types of symmetry groups in two-dimensional spaces of constant curvature. The advantage of the notation is that it describes these groups in a way which indicates many of the groups' properties: in particular, it follows William Thurston in describing the orbifold obtained by taking the quotient of Euclidean space by the group under consideration. Groups representable in this notation include the point groups on the sphere (S^2), the frieze groups and wallpaper groups of the Euclidean plane (E^2), and their analogues on the hyperbolic plane (H^2). Definition of the notation The following types of Euclidean transformation can occur in a group described by orbifold notation: * reflection through a line (or plane) * translation by a vector * rotation of finite order around a point * infinite rotation around a line in 3- ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
John Horton Conway
John Horton Conway (26 December 1937 – 11 April 2020) was an English mathematician active in the theory of finite groups, knot theory, number theory, combinatorial game theory and coding theory. He also made contributions to many branches of recreational mathematics, most notably the invention of the cellular automaton called the Game of Life. Born and raised in Liverpool, Conway spent the first half of his career at the University of Cambridge before moving to the United States, where he held the John von Neumann Professorship at Princeton University for the rest of his career. On 11 April 2020, at age 82, he died of complications from COVID-19. Early life and education Conway was born on 26 December 1937 in Liverpool, the son of Cyril Horton Conway and Agnes Boyce. He became interested in mathematics at a very early age. By the time he was 11, his ambition was to become a mathematician. After leaving sixth form, he studied mathematics at Gonville and Caius College, Camb ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Dihedral Symmetry
In mathematics, a dihedral group is the group of symmetries of a regular polygon, which includes rotations and reflections. Dihedral groups are among the simplest examples of finite groups, and they play an important role in group theory, geometry, and chemistry. The notation for the dihedral group differs in geometry and abstract algebra. In geometry, or refers to the symmetries of the -gon, a group of order . In abstract algebra, refers to this same dihedral group. This article uses the geometric convention, . Definition Elements A regular polygon with n sides has 2n different symmetries: n rotational symmetries and n reflection symmetries. Usually, we take n \ge 3 here. The associated rotations and reflections make up the dihedral group \mathrm_n. If n is odd, each axis of symmetry connects the midpoint of one side to the opposite vertex. If n is even, there are n/2 axes of symmetry connecting the midpoints of opposite sides and n/2 axes of symmetry connecting oppo ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Rotational Symmetry
Rotational symmetry, also known as radial symmetry in geometry, is the property a shape has when it looks the same after some rotation by a partial turn. An object's degree of rotational symmetry is the number of distinct orientations in which it looks exactly the same for each rotation. Certain geometric objects are partially symmetrical when rotated at certain angles such as squares rotated 90°, however the only geometric objects that are fully rotationally symmetric at any angle are spheres, circles and other spheroids. Formal treatment Formally the rotational symmetry is symmetry with respect to some or all rotations in ''m''-dimensional Euclidean space. Rotations are direct isometries, i.e., isometries preserving orientation. Therefore, a symmetry group of rotational symmetry is a subgroup of ''E''+(''m'') (see Euclidean group). Symmetry with respect to all rotations about all points implies translational symmetry with respect to all translations, so space is homo ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Polygon
In geometry, a polygon () is a plane figure that is described by a finite number of straight line segments connected to form a closed ''polygonal chain'' (or ''polygonal circuit''). The bounded plane region, the bounding circuit, or the two together, may be called a polygon. The segments of a polygonal circuit are called its '' edges'' or ''sides''. The points where two edges meet are the polygon's '' vertices'' (singular: vertex) or ''corners''. The interior of a solid polygon is sometimes called its ''body''. An ''n''-gon is a polygon with ''n'' sides; for example, a triangle is a 3-gon. A simple polygon is one which does not intersect itself. Mathematicians are often concerned only with the bounding polygonal chains of simple polygons and they often define a polygon accordingly. A polygonal boundary may be allowed to cross over itself, creating star polygons and other self-intersecting polygons. A polygon is a 2-dimensional example of the more general polytope in any number ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
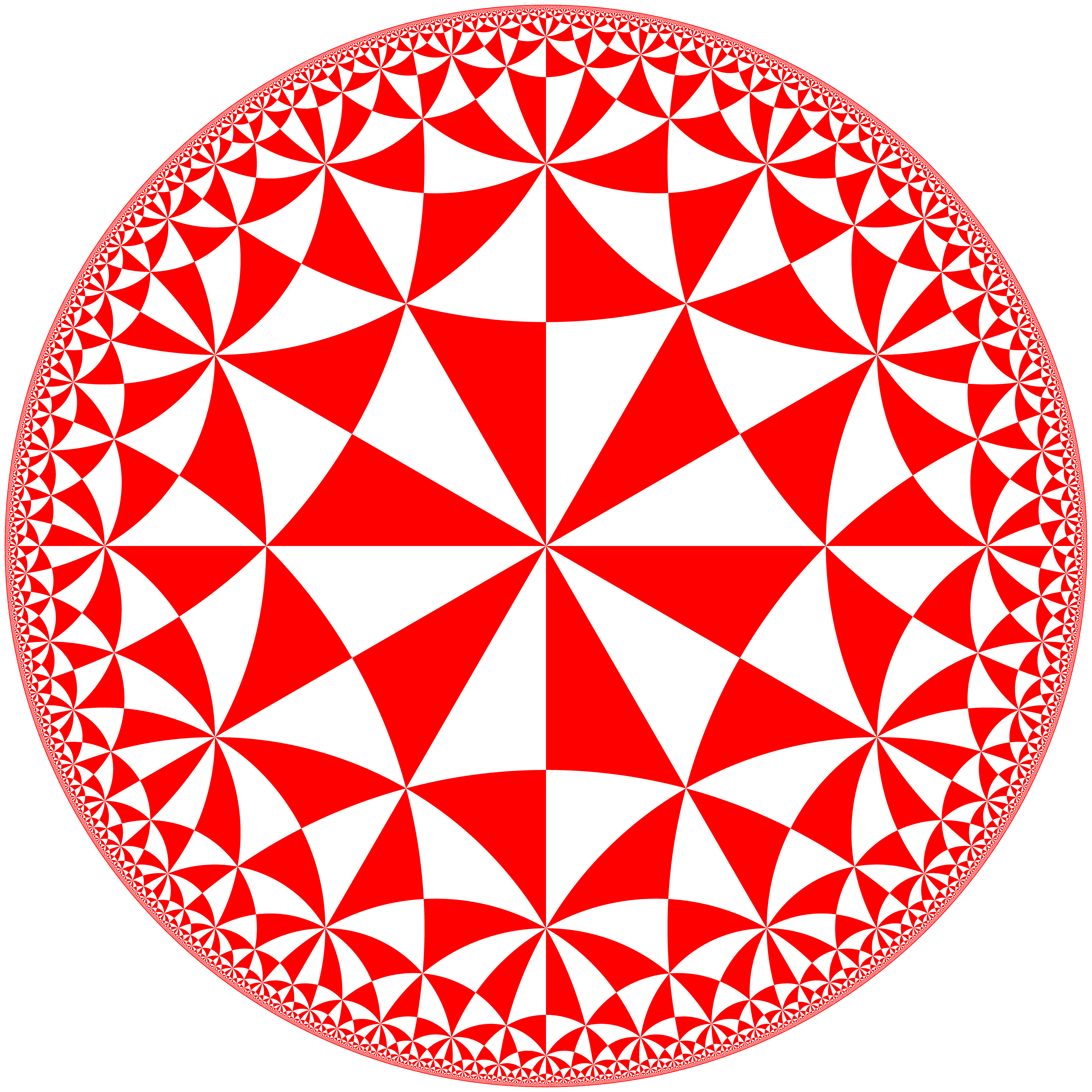
Octagonal Tiling
In geometry, the octagonal tiling is a regular tiling of the hyperbolic plane. It is represented by Schläfli symbol of ', having three regular octagons around each vertex. It also has a construction as a truncated order-8 square tiling, t. Uniform colorings Like the hexagonal tiling of the Euclidean plane, there are 3 uniform colorings of this hyperbolic tiling. The dual tiling V8.8.8 represents the fundamental domains of 4,4,4)symmetry. Related polyhedra and tilings This tiling is topologically part of sequence of regular polyhedra and tilings with Schläfli symbol . And also is topologically part of sequence of regular tilings with Schläfli symbol . From a Wythoff construction there are ten hyperbolic uniform tilings that can be based from the regular octagonal tiling. Drawing the tiles colored as red on the original faces, yellow at the original vertices, and blue along the original edges, there are 10 forms. See also * Tilings of regular polygons ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Dual Polyhedron
In geometry, every polyhedron is associated with a second dual structure, where the vertices of one correspond to the faces of the other, and the edges between pairs of vertices of one correspond to the edges between pairs of faces of the other. Such dual figures remain combinatorial or abstract polyhedra, but not all can also be constructed as geometric polyhedra. Starting with any given polyhedron, the dual of its dual is the original polyhedron. Duality preserves the symmetries of a polyhedron. Therefore, for many classes of polyhedra defined by their symmetries, the duals belong to a corresponding symmetry class. For example, the regular polyhedrathe (convex) Platonic solids and (star) Kepler–Poinsot polyhedraform dual pairs, where the regular tetrahedron is self-dual. The dual of an isogonal polyhedron (one in which any two vertices are equivalent under symmetries of the polyhedron) is an isohedral polyhedron (one in which any two faces are equivalent .., and vice vers ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
.svg "Click for more on -> Hypercycle (geometry)")
.svg "Click for more on -> Taschen"){kind=logo}
