Geometric Cremation Urn Athens Agora Museum on:
[Wikipedia]
[Google]
[Amazon]
Geometry (; ) is, with
 The earliest recorded beginnings of geometry can be traced to ancient
The earliest recorded beginnings of geometry can be traced to ancient 

 In Euclidean geometry, angles are used to study
In Euclidean geometry, angles are used to study
 A surface is a two-dimensional object, such as a sphere or paraboloid. In
A surface is a two-dimensional object, such as a sphere or paraboloid. In
 The concept of length or distance can be generalized, leading to the idea of
The concept of length or distance can be generalized, leading to the idea of
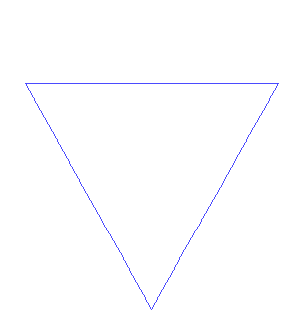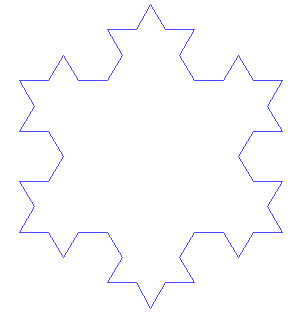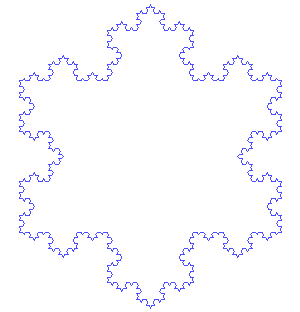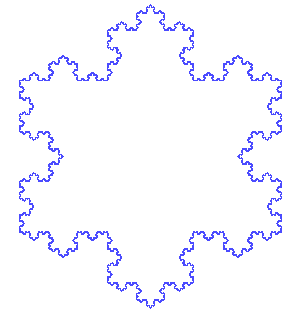 Where the traditional geometry allowed dimensions 1 (a
Where the traditional geometry allowed dimensions 1 (a
 The theme of
The theme of

 The field of
The field of
 Discrete geometry is a subject that has close connections with
Discrete geometry is a subject that has close connections with
 Mathematics and art are related in a variety of ways. For instance, the theory of perspective showed that there is more to geometry than just the metric properties of figures: perspective is the origin of projective geometry.
Artists have long used concepts of
Mathematics and art are related in a variety of ways. For instance, the theory of perspective showed that there is more to geometry than just the metric properties of figures: perspective is the origin of projective geometry.
Artists have long used concepts of

''Unusual Geometry Problems''
''The Math Forum'' – Geometry
*
*
*
Nature Precedings – ''Pegs and Ropes Geometry at Stonehenge''
* ttps://web.archive.org/web/20071004174210/http://www.gresham.ac.uk/event.asp?PageId=45&EventId=618 "4000 Years of Geometry" lecture by Robin Wilson given at Gresham College, 3 October 2007 (available for MP3 and MP4 download as well as a text file) *
Finitism in Geometry
at the Stanford Encyclopedia of Philosophy
Interactive geometry reference with hundreds of applets
Geometry classes
at
arithmetic
Arithmetic () is an elementary part of mathematics that consists of the study of the properties of the traditional operations on numbers— addition, subtraction, multiplication, division, exponentiation, and extraction of roots. In the 19th ...
, one of the oldest branches of mathematics
Mathematics is an area of knowledge that includes the topics of numbers, formulas and related structures, shapes and the spaces in which they are contained, and quantities and their changes. These topics are represented in modern mathematics ...
. It is concerned with properties of space such as the distance, shape, size, and relative position of figures. A mathematician who works in the field of geometry is called a '' geometer''.
Until the 19th century, geometry was almost exclusively devoted to Euclidean geometry, which includes the notions of point
Point or points may refer to:
Places
* Point, Lewis, a peninsula in the Outer Hebrides, Scotland
* Point, Texas, a city in Rains County, Texas, United States
* Point, the NE tip and a ferry terminal of Lismore, Inner Hebrides, Scotland
* Point ...
, line
Line most often refers to:
* Line (geometry), object with zero thickness and curvature that stretches to infinity
* Telephone line, a single-user circuit on a telephone communication system
Line, lines, The Line, or LINE may also refer to:
Arts ...
, plane
Plane(s) most often refers to:
* Aero- or airplane, a powered, fixed-wing aircraft
* Plane (geometry), a flat, 2-dimensional surface
Plane or planes may also refer to:
Biology
* Plane (tree) or ''Platanus'', wetland native plant
* Planes (gen ...
, distance
Distance is a numerical or occasionally qualitative measurement of how far apart objects or points are. In physics or everyday usage, distance may refer to a physical length or an estimation based on other criteria (e.g. "two counties over"). ...
, angle
In Euclidean geometry, an angle is the figure formed by two Ray (geometry), rays, called the ''Side (plane geometry), sides'' of the angle, sharing a common endpoint, called the ''vertex (geometry), vertex'' of the angle.
Angles formed by two ...
, surface, and curve
In mathematics, a curve (also called a curved line in older texts) is an object similar to a line (geometry), line, but that does not have to be Linearity, straight.
Intuitively, a curve may be thought of as the trace left by a moving point (ge ...
, as fundamental concepts.
During the 19th century several discoveries enlarged dramatically the scope of geometry. One of the oldest such discoveries is Carl Friedrich Gauss' ("remarkable theorem") that asserts roughly that the Gaussian curvature
In differential geometry, the Gaussian curvature or Gauss curvature of a surface at a point is the product of the principal curvatures, and , at the given point:
K = \kappa_1 \kappa_2.
The Gaussian radius of curvature is the reciprocal of .
F ...
of a surface is independent from any specific embedding in a Euclidean space
Euclidean space is the fundamental space of geometry, intended to represent physical space. Originally, that is, in Euclid's Elements, Euclid's ''Elements'', it was the three-dimensional space of Euclidean geometry, but in modern mathematics ther ...
. This implies that surfaces can be studied ''intrinsically'', that is, as stand-alone spaces, and has been expanded into the theory of manifold
In mathematics, a manifold is a topological space that locally resembles Euclidean space near each point. More precisely, an n-dimensional manifold, or ''n-manifold'' for short, is a topological space with the property that each point has a n ...
s and Riemannian geometry.
Later in the 19th century, it appeared that geometries without the parallel postulate (non-Euclidean geometries
In mathematics, non-Euclidean geometry consists of two geometries based on axioms closely related to those that specify Euclidean geometry. As Euclidean geometry lies at the intersection of metric geometry and affine geometry, non-Euclidean geo ...
) can be developed without introducing any contradiction. The geometry that underlies general relativity
General relativity, also known as the general theory of relativity and Einstein's theory of gravity, is the geometric theory of gravitation published by Albert Einstein in 1915 and is the current description of gravitation in modern physics ...
is a famous application of non-Euclidean geometry.
Since then, the scope of geometry has been greatly expanded, and the field has been split in many subfields that depend on the underlying methods—differential geometry
Differential geometry is a mathematical discipline that studies the geometry of smooth shapes and smooth spaces, otherwise known as smooth manifolds. It uses the techniques of differential calculus, integral calculus, linear algebra and multili ...
, algebraic geometry
Algebraic geometry is a branch of mathematics, classically studying zeros of multivariate polynomials. Modern algebraic geometry is based on the use of abstract algebraic techniques, mainly from commutative algebra, for solving geometrical ...
, computational geometry
Computational geometry is a branch of computer science devoted to the study of algorithms which can be stated in terms of geometry. Some purely geometrical problems arise out of the study of computational geometric algorithms, and such problems ar ...
, algebraic topology
Algebraic topology is a branch of mathematics that uses tools from abstract algebra to study topological spaces. The basic goal is to find algebraic invariant (mathematics), invariants that classification theorem, classify topological spaces up t ...
, discrete geometry (also known as ''combinatorial geometry''), etc.—or on the properties of Euclidean spaces that are disregarded— projective geometry that consider only alignment of points but not distance and parallelism, affine geometry that omits the concept of angle and distance, finite geometry that omits continuity, and others.
Originally developed to model the physical world, geometry has applications in almost all science
Science is a systematic endeavor that builds and organizes knowledge in the form of testable explanations and predictions about the universe.
Science may be as old as the human species, and some of the earliest archeological evidence for ...
s, and also in art
Art is a diverse range of human activity, and resulting product, that involves creative or imaginative talent expressive of technical proficiency, beauty, emotional power, or conceptual ideas.
There is no generally agreed definition of wha ...
, architecture
Architecture is the art and technique of designing and building, as distinguished from the skills associated with construction. It is both the process and the product of sketching, conceiving, planning, designing, and constructing building ...
, and other activities that are related to graphics
Graphics () are visual images or designs on some surface, such as a wall, canvas, screen, paper, or stone, to inform, illustrate, or entertain. In contemporary usage, it includes a pictorial representation of data, as in design and manufacture ...
. Geometry also has applications in areas of mathematics that are apparently unrelated. For example, methods of algebraic geometry are fundamental in Wiles's proof of Fermat's Last Theorem, a problem that was stated in terms of elementary arithmetic, and remained unsolved for several centuries.
History
 The earliest recorded beginnings of geometry can be traced to ancient
The earliest recorded beginnings of geometry can be traced to ancient Mesopotamia
Mesopotamia ''Mesopotamíā''; ar, بِلَاد ٱلرَّافِدَيْن or ; syc, ܐܪܡ ܢܗܪ̈ܝܢ, or , ) is a historical region of Western Asia situated within the Tigris–Euphrates river system, in the northern part of the F ...
and Egypt
Egypt ( ar, مصر , ), officially the Arab Republic of Egypt, is a transcontinental country spanning the northeast corner of Africa and southwest corner of Asia via a land bridge formed by the Sinai Peninsula. It is bordered by the Mediter ...
in the 2nd millennium BC. Early geometry was a collection of empirically discovered principles concerning lengths, angles, areas, and volumes, which were developed to meet some practical need in surveying
Surveying or land surveying is the technique, profession, art, and science of determining the terrestrial two-dimensional or three-dimensional positions of points and the distances and angles between them. A land surveying professional is ca ...
, construction
Construction is a general term meaning the art and science to form objects, systems, or organizations,"Construction" def. 1.a. 1.b. and 1.c. ''Oxford English Dictionary'' Second Edition on CD-ROM (v. 4.0) Oxford University Press 2009 and com ...
, astronomy
Astronomy () is a natural science that studies astronomical object, celestial objects and phenomena. It uses mathematics, physics, and chemistry in order to explain their origin and chronology of the Universe, evolution. Objects of interest ...
, and various crafts. The earliest known texts on geometry are the Egyptian
Egyptian describes something of, from, or related to Egypt.
Egyptian or Egyptians may refer to:
Nations and ethnic groups
* Egyptians, a national group in North Africa
** Egyptian culture, a complex and stable culture with thousands of years of ...
Rhind Papyrus (2000–1800 BC) and Moscow Papyrus
The Moscow Mathematical Papyrus, also named the Golenishchev Mathematical Papyrus after its first non-Egyptian owner, Egyptologist Vladimir Golenishchev, is an ancient Egyptian mathematical papyrus containing several problems in arithmetic, geom ...
(), and the Babylonian clay tablets, such as Plimpton 322 (1900 BC). For example, the Moscow Papyrus gives a formula for calculating the volume of a truncated pyramid, or frustum. Later clay tablets (350–50 BC) demonstrate that Babylonian astronomers implemented trapezoid
A quadrilateral with at least one pair of parallel sides is called a trapezoid () in American and Canadian English. In British and other forms of English, it is called a trapezium ().
A trapezoid is necessarily a Convex polygon, convex quadri ...
procedures for computing Jupiter's position and motion
In physics, motion is the phenomenon in which an object changes its position with respect to time. Motion is mathematically described in terms of displacement, distance, velocity, acceleration, speed and frame of reference to an observer and mea ...
within time-velocity space. These geometric procedures anticipated the Oxford Calculators
The Oxford Calculators were a group of 14th-century thinkers, almost all associated with Merton College, Oxford; for this reason they were dubbed "The Merton School". These men took a strikingly logical and mathematical approach to philosophical ...
, including the mean speed theorem, by 14 centuries. South of Egypt the ancient Nubians
Nubians () (Nobiin: ''Nobī,'' ) are an ethnic group indigenous to the region which is now northern Sudan and southern Egypt. They originate from the early inhabitants of the central Nile valley, believed to be one of the earliest cradles of c ...
established a system of geometry including early versions of sun clocks.
In the 7th century BC, the Greek mathematician Thales of Miletus
Thales of Miletus ( ; grc-gre, Θαλῆς; ) was a Greek mathematician, astronomer, statesman, and pre-Socratic philosopher from Miletus in Ionia, Asia Minor. He was one of the Seven Sages of Greece. Many, most notably Aristotle, regarded him ...
used geometry to solve problems such as calculating the height of pyramids and the distance of ships from the shore. He is credited with the first use of deductive reasoning applied to geometry, by deriving four corollaries to Thales's theorem. Pythagoras
Pythagoras of Samos ( grc, Πυθαγόρας ὁ Σάμιος, Pythagóras ho Sámios, Pythagoras the Samos, Samian, or simply ; in Ionian Greek; ) was an ancient Ionians, Ionian Ancient Greek philosophy, Greek philosopher and the eponymou ...
established the Pythagorean School
Pythagorean, meaning of or pertaining to the ancient Ionian mathematician, philosopher, and music theorist Pythagoras, may refer to:
Philosophy
* Pythagoreanism, the esoteric and metaphysical beliefs purported to have been held by Pythagoras
* Ne ...
, which is credited with the first proof of the Pythagorean theorem
In mathematics, the Pythagorean theorem or Pythagoras' theorem is a fundamental relation in Euclidean geometry between the three sides of a right triangle. It states that the area of the square whose side is the hypotenuse (the side opposite t ...
, though the statement of the theorem has a long history. Eudoxus (408–) developed the method of exhaustion
The method of exhaustion (; ) is a method of finding the area of a shape by inscribing inside it a sequence of polygons whose areas converge to the area of the containing shape. If the sequence is correctly constructed, the difference in area bet ...
, which allowed the calculation of areas and volumes of curvilinear figures, as well as a theory of ratios that avoided the problem of incommensurable magnitudes
In mathematics, the irrational numbers (from in- prefix assimilated to ir- (negative prefix, privative) + rational) are all the real numbers that are not rational numbers. That is, irrational numbers cannot be expressed as the ratio of two integ ...
, which enabled subsequent geometers to make significant advances. Around 300 BC, geometry was revolutionized by Euclid, whose ''Elements
Element or elements may refer to:
Science
* Chemical element, a pure substance of one type of atom
* Heating element, a device that generates heat by electrical resistance
* Orbital elements, parameters required to identify a specific orbit of ...
'', widely considered the most successful and influential textbook of all time, introduced mathematical rigor through the axiomatic method
In mathematics and logic, an axiomatic system is any set of axioms from which some or all axioms can be used in conjunction to logically derive theorems. A theory is a consistent, relatively-self-contained body of knowledge which usually contains ...
and is the earliest example of the format still used in mathematics today, that of definition, axiom, theorem, and proof. Although most of the contents of the ''Elements'' were already known, Euclid arranged them into a single, coherent logical framework. The ''Elements'' was known to all educated people in the West until the middle of the 20th century and its contents are still taught in geometry classes today. Archimedes
Archimedes of Syracuse (;; ) was a Greek mathematician, physicist, engineer, astronomer, and inventor from the ancient city of Syracuse in Sicily. Although few details of his life are known, he is regarded as one of the leading scientists ...
() of Syracuse, Italy used the method of exhaustion to calculate the area
Area is the quantity that expresses the extent of a region on the plane or on a curved surface. The area of a plane region or ''plane area'' refers to the area of a shape
A shape or figure is a graphics, graphical representation of an obje ...
under the arc of a parabola
In mathematics, a parabola is a plane curve which is mirror-symmetrical and is approximately U-shaped. It fits several superficially different mathematical descriptions, which can all be proved to define exactly the same curves.
One descript ...
with the summation of an infinite series, and gave remarkably accurate approximations of pi. He also studied the spiral
In mathematics, a spiral is a curve which emanates from a point, moving farther away as it revolves around the point.
Helices
Two major definitions of "spiral" in the American Heritage Dictionary are:volume
Volume is a measure of occupied three-dimensional space. It is often quantified numerically using SI derived units (such as the cubic metre and litre) or by various imperial or US customary units (such as the gallon, quart, cubic inch). The de ...
s of surfaces of revolution.

Indian
Indian or Indians may refer to:
Peoples South Asia
* Indian people, people of Indian nationality, or people who have an Indian ancestor
** Non-resident Indian, a citizen of India who has temporarily emigrated to another country
* South Asia ...
mathematicians also made many important contributions in geometry. The ''Shatapatha Brahmana
The Shatapatha Brahmana ( sa, शतपथब्राह्मणम् , Śatapatha Brāhmaṇam, meaning 'Brāhmaṇa of one hundred paths', abbreviated to 'SB') is a commentary on the Śukla (white) Yajurveda. It is attributed to the Vedic ...
'' (3rd century BC) contains rules for ritual geometric constructions that are similar to the '' Sulba Sutras''.
According to , the ''Śulba Sūtras'' contain "the earliest extant verbal expression of the Pythagorean Theorem in the world, although it had already been known to the Old Babylonians. They contain lists of Pythagorean triples, which are particular cases of Diophantine equations.: "The arithmetic content of the ''Śulva Sūtras'' consists of rules for finding Pythagorean triples such as (3, 4, 5), (5, 12, 13), (8, 15, 17), and (12, 35, 37). It is not certain what practical use these arithmetic rules had. The best conjecture is that they were part of religious ritual. A Hindu home was required to have three fires burning at three different altars. The three altars were to be of different shapes, but all three were to have the same area. These conditions led to certain "Diophantine" problems, a particular case of which is the generation of Pythagorean triples, so as to make one square integer equal to the sum of two others."
In the Bakhshali manuscript, there are a handful of geometric problems (including problems about volumes of irregular solids). The Bakhshali manuscript also "employs a decimal place value system with a dot for zero." Aryabhata's ''Aryabhatiya
''Aryabhatiya'' (IAST: ') or ''Aryabhatiyam'' ('), a Sanskrit astronomical treatise, is the ''magnum opus'' and only known surviving work of the 5th century Indian mathematician Aryabhata. Philosopher of astronomy Roger Billard estimates that th ...
'' (499) includes the computation of areas and volumes.
Brahmagupta
Brahmagupta ( – ) was an Indian mathematician and astronomer. He is the author of two early works on mathematics and astronomy: the ''Brāhmasphuṭasiddhānta'' (BSS, "correctly established doctrine of Brahma", dated 628), a theoretical trea ...
wrote his astronomical work '' '' in 628. Chapter 12, containing 66 Sanskrit
Sanskrit (; attributively , ; nominally , , ) is a classical language belonging to the Indo-Aryan branch of the Indo-European languages. It arose in South Asia after its predecessor languages had diffused there from the northwest in the late ...
verses, was divided into two sections: "basic operations" (including cube roots, fractions, ratio and proportion, and barter) and "practical mathematics" (including mixture, mathematical series, plane figures, stacking bricks, sawing of timber, and piling of grain). In the latter section, he stated his famous theorem on the diagonals of a cyclic quadrilateral. Chapter 12 also included a formula for the area of a cyclic quadrilateral (a generalization of Heron's formula), as well as a complete description of rational triangle
An integer triangle or integral triangle is a triangle all of whose sides have lengths that are integers. A rational triangle can be defined as one having all sides with rational length; any such rational triangle can be integrally rescaled (ca ...
s (''i.e.'' triangles with rational sides and rational areas).
In the Middle Ages
In the history of Europe, the Middle Ages or medieval period lasted approximately from the late 5th to the late 15th centuries, similar to the post-classical period of global history. It began with the fall of the Western Roman Empire a ...
, mathematics in medieval Islam
Mathematics during the Golden Age of Islam, especially during the 9th and 10th centuries, was built on Greek mathematics (Euclid, Archimedes, Apollonius of Perga, Apollonius) and Indian mathematics (Aryabhata, Brahmagupta). Important progress wa ...
contributed to the development of geometry, especially algebraic geometry
Algebraic geometry is a branch of mathematics, classically studying zeros of multivariate polynomials. Modern algebraic geometry is based on the use of abstract algebraic techniques, mainly from commutative algebra, for solving geometrical ...
. Al-Mahani
Abu-Abdullah Muhammad ibn Īsa Māhānī (, flourished c. 860 and died c. 880) was a Persian mathematician and astronomer born in Mahan, (in today Kermān, Iran) and active in Baghdad, Abbasid Caliphate. His known mathematical works included his ...
(b. 853) conceived the idea of reducing geometrical problems such as duplicating the cube to problems in algebra. Thābit ibn Qurra
Thābit ibn Qurra (full name: , ar, أبو الحسن ثابت بن قرة بن زهرون الحراني الصابئ, la, Thebit/Thebith/Tebit); 826 or 836 – February 19, 901, was a mathematician, physician, astronomer, and translator who ...
(known as Thebit in Latin
Latin (, or , ) is a classical language belonging to the Italic branch of the Indo-European languages. Latin was originally a dialect spoken in the lower Tiber area (then known as Latium) around present-day Rome, but through the power of the ...
) (836–901) dealt with arithmetic
Arithmetic () is an elementary part of mathematics that consists of the study of the properties of the traditional operations on numbers— addition, subtraction, multiplication, division, exponentiation, and extraction of roots. In the 19th ...
operations applied to ratio
In mathematics, a ratio shows how many times one number contains another. For example, if there are eight oranges and six lemons in a bowl of fruit, then the ratio of oranges to lemons is eight to six (that is, 8:6, which is equivalent to the ...
s of geometrical quantities, and contributed to the development of analytic geometry
In classical mathematics, analytic geometry, also known as coordinate geometry or Cartesian geometry, is the study of geometry using a coordinate system. This contrasts with synthetic geometry.
Analytic geometry is used in physics and engineerin ...
. Omar Khayyam (1048–1131) found geometric solutions to cubic equations. The theorems of Ibn al-Haytham
Ḥasan Ibn al-Haytham, Latinized as Alhazen (; full name ; ), was a medieval mathematician, astronomer, and physicist of the Islamic Golden Age from present-day Iraq.For the description of his main fields, see e.g. ("He is one of the prin ...
(Alhazen), Omar Khayyam and Nasir al-Din al-Tusi
Muhammad ibn Muhammad ibn al-Hasan al-Tūsī ( fa, محمد ابن محمد ابن حسن طوسی 18 February 1201 – 26 June 1274), better known as Nasir al-Din al-Tusi ( fa, نصیر الدین طوسی, links=no; or simply Tusi in the West ...
on quadrilaterals, including the Lambert quadrilateral and Saccheri quadrilateral
A Saccheri quadrilateral (also known as a Khayyam–Saccheri quadrilateral) is a quadrilateral with two equal sides perpendicular to the base. It is named after Giovanni Gerolamo Saccheri, who used it extensively in his book ''Euclides ab omni na ...
, were early results in hyperbolic geometry, and along with their alternative postulates, such as Playfair's axiom, these works had a considerable influence on the development of non-Euclidean geometry among later European geometers, including Vitello (), Gersonides (1288–1344), Alfonso, John Wallis
John Wallis (; la, Wallisius; ) was an English clergyman and mathematician who is given partial credit for the development of infinitesimal calculus. Between 1643 and 1689 he served as chief cryptographer for Parliament and, later, the royal ...
, and Giovanni Girolamo Saccheri.
In the early 17th century, there were two important developments in geometry. The first was the creation of analytic geometry, or geometry with coordinates
In geometry, a coordinate system is a system that uses one or more numbers, or coordinates, to uniquely determine the position of the points or other geometric elements on a manifold such as Euclidean space. The order of the coordinates is sig ...
and equation
In mathematics, an equation is a formula that expresses the equality of two expressions, by connecting them with the equals sign . The word ''equation'' and its cognates in other languages may have subtly different meanings; for example, in ...
s, by René Descartes
René Descartes ( or ; ; Latinized: Renatus Cartesius; 31 March 1596 – 11 February 1650) was a French philosopher, scientist, and mathematician, widely considered a seminal figure in the emergence of modern philosophy and science. Mathem ...
(1596–1650) and Pierre de Fermat
Pierre de Fermat (; between 31 October and 6 December 1607 – 12 January 1665) was a French mathematician who is given credit for early developments that led to infinitesimal calculus, including his technique of adequality. In particular, he ...
(1601–1665). This was a necessary precursor to the development of calculus
Calculus, originally called infinitesimal calculus or "the calculus of infinitesimals", is the mathematical study of continuous change, in the same way that geometry is the study of shape, and algebra is the study of generalizations of arithm ...
and a precise quantitative science of physics
Physics is the natural science that studies matter, its fundamental constituents, its motion and behavior through space and time, and the related entities of energy and force. "Physical science is that department of knowledge which r ...
. The second geometric development of this period was the systematic study of projective geometry by Girard Desargues (1591–1661). Projective geometry studies properties of shapes which are unchanged under projections and sections
Section, Sectioning or Sectioned may refer to:
Arts, entertainment and media
* Section (music), a complete, but not independent, musical idea
* Section (typography), a subdivision, especially of a chapter, in books and documents
** Section sig ...
, especially as they relate to artistic perspective.
Two developments in geometry in the 19th century changed the way it had been studied previously. These were the discovery of non-Euclidean geometries
In mathematics, non-Euclidean geometry consists of two geometries based on axioms closely related to those that specify Euclidean geometry. As Euclidean geometry lies at the intersection of metric geometry and affine geometry, non-Euclidean geo ...
by Nikolai Ivanovich Lobachevsky, János Bolyai and Carl Friedrich Gauss and of the formulation of symmetry
Symmetry (from grc, συμμετρία "agreement in dimensions, due proportion, arrangement") in everyday language refers to a sense of harmonious and beautiful proportion and balance. In mathematics, "symmetry" has a more precise definit ...
as the central consideration in the Erlangen programme
In mathematics, the Erlangen program is a method of characterizing geometries based on group theory and projective geometry. It was published by Felix Klein in 1872 as ''Vergleichende Betrachtungen über neuere geometrische Forschungen.'' It is nam ...
of Felix Klein (which generalized the Euclidean and non-Euclidean geometries). Two of the master geometers of the time were Bernhard Riemann
Georg Friedrich Bernhard Riemann (; 17 September 1826 – 20 July 1866) was a German mathematician who made contributions to analysis, number theory, and differential geometry. In the field of real analysis, he is mostly known for the first rig ...
(1826–1866), working primarily with tools from mathematical analysis
Analysis is the branch of mathematics dealing with continuous functions, limit (mathematics), limits, and related theories, such as Derivative, differentiation, Integral, integration, measure (mathematics), measure, infinite sequences, series (m ...
, and introducing the Riemann surface
In mathematics, particularly in complex analysis, a Riemann surface is a connected one-dimensional complex manifold. These surfaces were first studied by and are named after Bernhard Riemann. Riemann surfaces can be thought of as deformed vers ...
, and Henri Poincaré
Jules Henri Poincaré ( S: stress final syllable ; 29 April 1854 – 17 July 1912) was a French mathematician, theoretical physicist, engineer, and philosopher of science. He is often described as a polymath, and in mathematics as "The ...
, the founder of algebraic topology
Algebraic topology is a branch of mathematics that uses tools from abstract algebra to study topological spaces. The basic goal is to find algebraic invariant (mathematics), invariants that classification theorem, classify topological spaces up t ...
and the geometric theory of dynamical system
In mathematics, a dynamical system is a system in which a Function (mathematics), function describes the time dependence of a Point (geometry), point in an ambient space. Examples include the mathematical models that describe the swinging of a ...
s. As a consequence of these major changes in the conception of geometry, the concept of "space" became something rich and varied, and the natural background for theories as different as complex analysis
Complex analysis, traditionally known as the theory of functions of a complex variable, is the branch of mathematical analysis that investigates Function (mathematics), functions of complex numbers. It is helpful in many branches of mathemati ...
and classical mechanics
Classical mechanics is a physical theory describing the motion of macroscopic objects, from projectiles to parts of machinery, and astronomical objects, such as spacecraft, planets, stars, and galaxies. For objects governed by classical ...
.
Main concepts
The following are some of the most important concepts in geometry.Axioms
Euclid
Euclid (; grc-gre, Wikt:Εὐκλείδης, Εὐκλείδης; BC) was an ancient Greek mathematician active as a geometer and logician. Considered the "father of geometry", he is chiefly known for the ''Euclid's Elements, Elements'' trea ...
took an abstract approach to geometry in his Elements
Element or elements may refer to:
Science
* Chemical element, a pure substance of one type of atom
* Heating element, a device that generates heat by electrical resistance
* Orbital elements, parameters required to identify a specific orbit of ...
, one of the most influential books ever written. Euclid introduced certain axiom
An axiom, postulate, or assumption is a statement that is taken to be true, to serve as a premise or starting point for further reasoning and arguments. The word comes from the Ancient Greek word (), meaning 'that which is thought worthy or f ...
s, or postulates, expressing primary or self-evident properties of points, lines, and planes. He proceeded to rigorously deduce other properties by mathematical reasoning. The characteristic feature of Euclid's approach to geometry was its rigor, and it has come to be known as ''axiomatic'' or ''synthetic Synthetic things are composed of multiple parts, often with the implication that they are artificial. In particular, 'synthetic' may refer to:
Science
* Synthetic chemical or compound, produced by the process of chemical synthesis
* Synthetic o ...
'' geometry. At the start of the 19th century, the discovery of non-Euclidean geometries
In mathematics, non-Euclidean geometry consists of two geometries based on axioms closely related to those that specify Euclidean geometry. As Euclidean geometry lies at the intersection of metric geometry and affine geometry, non-Euclidean geo ...
by Nikolai Ivanovich Lobachevsky (1792–1856), János Bolyai
János Bolyai (; 15 December 1802 – 27 January 1860) or Johann Bolyai, was a Hungarian mathematician, who developed absolute geometry—a geometry that includes both Euclidean geometry and hyperbolic geometry. The discovery of a consisten ...
(1802–1860), Carl Friedrich Gauss (1777–1855) and others led to a revival of interest in this discipline, and in the 20th century, David Hilbert
David Hilbert (; ; 23 January 1862 – 14 February 1943) was a German mathematician, one of the most influential mathematicians of the 19th and early 20th centuries. Hilbert discovered and developed a broad range of fundamental ideas in many a ...
(1862–1943) employed axiomatic reasoning in an attempt to provide a modern foundation of geometry.
Objects
Points
Points are generally considered fundamental objects for building geometry. They may be defined by the properties that they must have, as in Euclid's definition as "that which has no part",''Euclid's Elements – All thirteen books in one volume'', Based on Heath's translation, Green Lion Press . or in synthetic geometry. In modern mathematics, they are generally defined aselements
Element or elements may refer to:
Science
* Chemical element, a pure substance of one type of atom
* Heating element, a device that generates heat by electrical resistance
* Orbital elements, parameters required to identify a specific orbit of ...
of a set
Set, The Set, SET or SETS may refer to:
Science, technology, and mathematics Mathematics
*Set (mathematics), a collection of elements
*Category of sets, the category whose objects and morphisms are sets and total functions, respectively
Electro ...
called space
Space is the boundless three-dimensional extent in which objects and events have relative position and direction. In classical physics, physical space is often conceived in three linear dimensions, although modern physicists usually consider ...
, which is itself axiomatically defined.
With these modern definitions, every geometric shape is defined as a set of points; this is not the case in synthetic geometry, where a line is another fundamental object that is not viewed as the set of the points through which it passes.
However, there are modern geometries in which points are not primitive objects, or even without points. One of the oldest such geometries is Whitehead's point-free geometry
In mathematics, point-free geometry is a geometry whose primitive ontological notion is ''region'' rather than point. Two axiomatic systems are set out below, one grounded in mereology, the other in mereotopology and known as ''connection theory' ...
, formulated by Alfred North Whitehead
Alfred North Whitehead (15 February 1861 – 30 December 1947) was an English mathematician and philosopher. He is best known as the defining figure of the philosophical school known as process philosophy, which today has found applicat ...
in 1919–1920.
Lines
Euclid
Euclid (; grc-gre, Wikt:Εὐκλείδης, Εὐκλείδης; BC) was an ancient Greek mathematician active as a geometer and logician. Considered the "father of geometry", he is chiefly known for the ''Euclid's Elements, Elements'' trea ...
described a line as "breadthless length" which "lies equally with respect to the points on itself". In modern mathematics, given the multitude of geometries, the concept of a line is closely tied to the way the geometry is described. For instance, in analytic geometry
In classical mathematics, analytic geometry, also known as coordinate geometry or Cartesian geometry, is the study of geometry using a coordinate system. This contrasts with synthetic geometry.
Analytic geometry is used in physics and engineerin ...
, a line in the plane is often defined as the set of points whose coordinates satisfy a given linear equation
In mathematics, a linear equation is an equation that may be put in the form
a_1x_1+\ldots+a_nx_n+b=0, where x_1,\ldots,x_n are the variables (or unknowns), and b,a_1,\ldots,a_n are the coefficients, which are often real numbers. The coefficien ...
, but in a more abstract setting, such as incidence geometry
In mathematics, incidence geometry is the study of incidence structures. A geometric structure such as the Euclidean plane is a complicated object that involves concepts such as length, angles, continuity, betweenness, and incidence. An ''incidenc ...
, a line may be an independent object, distinct from the set of points which lie on it. In differential geometry, a geodesic
In geometry, a geodesic () is a curve representing in some sense the shortest path ( arc) between two points in a surface, or more generally in a Riemannian manifold. The term also has meaning in any differentiable manifold with a connection. ...
is a generalization of the notion of a line to curved spaces.
Planes
In Euclidean geometry aplane
Plane(s) most often refers to:
* Aero- or airplane, a powered, fixed-wing aircraft
* Plane (geometry), a flat, 2-dimensional surface
Plane or planes may also refer to:
Biology
* Plane (tree) or ''Platanus'', wetland native plant
* Planes (gen ...
is a flat, two-dimensional surface that extends infinitely; the definitions for other types of geometries are generalizations of that. Planes are used in many areas of geometry. For instance, planes can be studied as a topological surface without reference to distances or angles; it can be studied as an affine space, where collinearity and ratios can be studied but not distances; it can be studied as the complex plane
In mathematics, the complex plane is the plane formed by the complex numbers, with a Cartesian coordinate system such that the -axis, called the real axis, is formed by the real numbers, and the -axis, called the imaginary axis, is formed by the ...
using techniques of complex analysis
Complex analysis, traditionally known as the theory of functions of a complex variable, is the branch of mathematical analysis that investigates Function (mathematics), functions of complex numbers. It is helpful in many branches of mathemati ...
; and so on.
Angles
Euclid
Euclid (; grc-gre, Wikt:Εὐκλείδης, Εὐκλείδης; BC) was an ancient Greek mathematician active as a geometer and logician. Considered the "father of geometry", he is chiefly known for the ''Euclid's Elements, Elements'' trea ...
defines a plane angle
In Euclidean geometry, an angle is the figure formed by two Ray (geometry), rays, called the ''Side (plane geometry), sides'' of the angle, sharing a common endpoint, called the ''vertex (geometry), vertex'' of the angle.
Angles formed by two ...
as the inclination to each other, in a plane, of two lines which meet each other, and do not lie straight with respect to each other. In modern terms, an angle is the figure formed by two rays, called the ''sides'' of the angle, sharing a common endpoint, called the ''vertex
Vertex, vertices or vertexes may refer to:
Science and technology Mathematics and computer science
*Vertex (geometry), a point where two or more curves, lines, or edges meet
*Vertex (computer graphics), a data structure that describes the position ...
'' of the angle.
polygon
In geometry, a polygon () is a plane figure that is described by a finite number of straight line segments connected to form a closed ''polygonal chain'' (or ''polygonal circuit''). The bounded plane region, the bounding circuit, or the two toge ...
s and triangle
A triangle is a polygon with three Edge (geometry), edges and three Vertex (geometry), vertices. It is one of the basic shapes in geometry. A triangle with vertices ''A'', ''B'', and ''C'' is denoted \triangle ABC.
In Euclidean geometry, an ...
s, as well as forming an object of study in their own right. The study of the angles of a triangle or of angles in a unit circle
In mathematics, a unit circle is a circle of unit radius—that is, a radius of 1. Frequently, especially in trigonometry, the unit circle is the circle of radius 1 centered at the origin (0, 0) in the Cartesian coordinate system in the Eucl ...
forms the basis of trigonometry
Trigonometry () is a branch of mathematics that studies relationships between side lengths and angles of triangles. The field emerged in the Hellenistic world during the 3rd century BC from applications of geometry to astronomical studies. T ...
.
In differential geometry
Differential geometry is a mathematical discipline that studies the geometry of smooth shapes and smooth spaces, otherwise known as smooth manifolds. It uses the techniques of differential calculus, integral calculus, linear algebra and multili ...
and calculus
Calculus, originally called infinitesimal calculus or "the calculus of infinitesimals", is the mathematical study of continuous change, in the same way that geometry is the study of shape, and algebra is the study of generalizations of arithm ...
, the angles between plane curves or space curve
In mathematics, a curve (also called a curved line in older texts) is an object similar to a line, but that does not have to be straight.
Intuitively, a curve may be thought of as the trace left by a moving point. This is the definition that a ...
s or surfaces can be calculated using the derivative
In mathematics, the derivative of a function of a real variable measures the sensitivity to change of the function value (output value) with respect to a change in its argument (input value). Derivatives are a fundamental tool of calculus. F ...
. Stewart, James (2012). ''Calculus: Early Transcendentals'', 7th ed., Brooks Cole Cengage Learning.
Curves
Acurve
In mathematics, a curve (also called a curved line in older texts) is an object similar to a line (geometry), line, but that does not have to be Linearity, straight.
Intuitively, a curve may be thought of as the trace left by a moving point (ge ...
is a 1-dimensional object that may be straight (like a line) or not; curves in 2-dimensional space are called plane curves and those in 3-dimensional space are called space curve
In mathematics, a curve (also called a curved line in older texts) is an object similar to a line, but that does not have to be straight.
Intuitively, a curve may be thought of as the trace left by a moving point. This is the definition that a ...
s.
In topology, a curve is defined by a function from an interval of the real numbers to another space. In differential geometry, the same definition is used, but the defining function is required to be differentiable Algebraic geometry studies algebraic curves, which are defined as algebraic varieties of dimension
In physics and mathematics, the dimension of a Space (mathematics), mathematical space (or object) is informally defined as the minimum number of coordinates needed to specify any Point (geometry), point within it. Thus, a Line (geometry), lin ...
one.
Surfaces
differential geometry
Differential geometry is a mathematical discipline that studies the geometry of smooth shapes and smooth spaces, otherwise known as smooth manifolds. It uses the techniques of differential calculus, integral calculus, linear algebra and multili ...
and topology
In mathematics, topology (from the Greek language, Greek words , and ) is concerned with the properties of a mathematical object, geometric object that are preserved under Continuous function, continuous Deformation theory, deformations, such ...
, surfaces are described by two-dimensional 'patches' (or neighborhoods
A neighbourhood (British English, Irish English, Australian English and Canadian English) or neighborhood (American English; American and British English spelling differences, see spelling differences) is a geographically localised community ...
) that are assembled by diffeomorphisms or homeomorphism
In the mathematical field of topology, a homeomorphism, topological isomorphism, or bicontinuous function is a bijective and continuous function between topological spaces that has a continuous inverse function. Homeomorphisms are the isomorphi ...
s, respectively. In algebraic geometry, surfaces are described by polynomial equations.
Manifolds
Amanifold
In mathematics, a manifold is a topological space that locally resembles Euclidean space near each point. More precisely, an n-dimensional manifold, or ''n-manifold'' for short, is a topological space with the property that each point has a n ...
is a generalization of the concepts of curve and surface. In topology
In mathematics, topology (from the Greek language, Greek words , and ) is concerned with the properties of a mathematical object, geometric object that are preserved under Continuous function, continuous Deformation theory, deformations, such ...
, a manifold is a topological space
In mathematics, a topological space is, roughly speaking, a geometrical space in which closeness is defined but cannot necessarily be measured by a numeric distance. More specifically, a topological space is a set whose elements are called points ...
where every point has a neighborhood
A neighbourhood (British English, Irish English, Australian English and Canadian English) or neighborhood (American English; see spelling differences) is a geographically localised community within a larger city, town, suburb or rural area, ...
that is homeomorphic
In the mathematical field of topology, a homeomorphism, topological isomorphism, or bicontinuous function is a bijective and continuous function between topological spaces that has a continuous inverse function. Homeomorphisms are the isomorphi ...
to Euclidean space. In differential geometry
Differential geometry is a mathematical discipline that studies the geometry of smooth shapes and smooth spaces, otherwise known as smooth manifolds. It uses the techniques of differential calculus, integral calculus, linear algebra and multili ...
, a differentiable manifold
In mathematics, a differentiable manifold (also differential manifold) is a type of manifold that is locally similar enough to a vector space to allow one to apply calculus. Any manifold can be described by a collection of charts (atlas). One ma ...
is a space where each neighborhood is diffeomorphic
In mathematics, a diffeomorphism is an isomorphism of smooth manifolds. It is an Inverse function, invertible Function (mathematics), function that maps one differentiable manifold to another such that both the function and its inverse function ...
to Euclidean space.
Manifolds are used extensively in physics, including in general relativity
General relativity, also known as the general theory of relativity and Einstein's theory of gravity, is the geometric theory of gravitation published by Albert Einstein in 1915 and is the current description of gravitation in modern physics ...
and string theory
In physics, string theory is a theoretical framework in which the point-like particles of particle physics are replaced by one-dimensional objects called strings. String theory describes how these strings propagate through space and interac ...
.
Length, area, and volume
Length
Length is a measure of distance. In the International System of Quantities, length is a quantity with dimension distance. In most systems of measurement a base unit for length is chosen, from which all other units are derived. In the Interna ...
, area
Area is the quantity that expresses the extent of a region on the plane or on a curved surface. The area of a plane region or ''plane area'' refers to the area of a shape
A shape or figure is a graphics, graphical representation of an obje ...
, and volume
Volume is a measure of occupied three-dimensional space. It is often quantified numerically using SI derived units (such as the cubic metre and litre) or by various imperial or US customary units (such as the gallon, quart, cubic inch). The de ...
describe the size or extent of an object in one dimension, two dimension, and three dimensions respectively.
In Euclidean geometry and analytic geometry
In classical mathematics, analytic geometry, also known as coordinate geometry or Cartesian geometry, is the study of geometry using a coordinate system. This contrasts with synthetic geometry.
Analytic geometry is used in physics and engineerin ...
, the length of a line segment can often be calculated by the Pythagorean theorem
In mathematics, the Pythagorean theorem or Pythagoras' theorem is a fundamental relation in Euclidean geometry between the three sides of a right triangle. It states that the area of the square whose side is the hypotenuse (the side opposite t ...
.
Area and volume can be defined as fundamental quantities separate from length, or they can be described and calculated in terms of lengths in a plane or 3-dimensional space. Mathematicians have found many explicit formulas for area and formulas for volume of various geometric objects. In calculus
Calculus, originally called infinitesimal calculus or "the calculus of infinitesimals", is the mathematical study of continuous change, in the same way that geometry is the study of shape, and algebra is the study of generalizations of arithm ...
, area and volume can be defined in terms of integral
In mathematics
Mathematics is an area of knowledge that includes the topics of numbers, formulas and related structures, shapes and the spaces in which they are contained, and quantities and their changes. These topics are represented i ...
s, such as the Riemann integral or the Lebesgue integral.
Metrics and measures
 The concept of length or distance can be generalized, leading to the idea of
The concept of length or distance can be generalized, leading to the idea of metrics
Metric or metrical may refer to:
* Metric system, an internationally adopted decimal system of measurement
* An adjective indicating relation to measurement in general, or a noun describing a specific type of measurement
Mathematics
In mathema ...
. For instance, the Euclidean metric measures the distance between points in the Euclidean plane
In mathematics, the Euclidean plane is a Euclidean space of dimension two. That is, a geometric setting in which two real quantities are required to determine the position of each point ( element of the plane), which includes affine notions of ...
, while the hyperbolic metric
In mathematics, a hyperbolic manifold is a space where every point looks locally like hyperbolic space of some dimension. They are especially studied in dimensions 2 and 3, where they are called Riemann surface#Hyperbolic Riemann surfaces, hyperbol ...
measures the distance in the hyperbolic plane
In mathematics, hyperbolic geometry (also called Lobachevskian geometry or Bolyai– Lobachevskian geometry) is a non-Euclidean geometry. The parallel postulate of Euclidean geometry is replaced with:
:For any given line ''R'' and point ''P'' ...
. Other important examples of metrics include the Lorentz metric
In differential geometry, a pseudo-Riemannian manifold, also called a semi-Riemannian manifold, is a differentiable manifold with a metric tensor that is everywhere nondegenerate. This is a generalization of a Riemannian manifold in which the r ...
of special relativity
In physics, the special theory of relativity, or special relativity for short, is a scientific theory regarding the relationship between space and time. In Albert Einstein's original treatment, the theory is based on two postulates:
# The laws o ...
and the semi- Riemannian metrics of general relativity
General relativity, also known as the general theory of relativity and Einstein's theory of gravity, is the geometric theory of gravitation published by Albert Einstein in 1915 and is the current description of gravitation in modern physics ...
.
In a different direction, the concepts of length, area and volume are extended by measure theory
In mathematics, the concept of a measure is a generalization and formalization of geometrical measures ( length, area, volume) and other common notions, such as mass and probability of events. These seemingly distinct concepts have many simil ...
, which studies methods of assigning a size or ''measure'' to sets, where the measures follow rules similar to those of classical area and volume.
Congruence and similarity
Congruence
Congruence may refer to:
Mathematics
* Congruence (geometry), being the same size and shape
* Congruence or congruence relation, in abstract algebra, an equivalence relation on an algebraic structure that is compatible with the structure
* In mod ...
and similarity are concepts that describe when two shapes have similar characteristics. In Euclidean geometry, similarity is used to describe objects that have the same shape, while congruence is used to describe objects that are the same in both size and shape. Hilbert, in his work on creating a more rigorous foundation for geometry, treated congruence as an undefined term whose properties are defined by axiom
An axiom, postulate, or assumption is a statement that is taken to be true, to serve as a premise or starting point for further reasoning and arguments. The word comes from the Ancient Greek word (), meaning 'that which is thought worthy or f ...
s.
Congruence and similarity are generalized in transformation geometry
In mathematics, transformation geometry (or transformational geometry) is the name of a mathematical and pedagogic take on the study of geometry by focusing on groups of geometric transformations, and properties that are invariant under them. ...
, which studies the properties of geometric objects that are preserved by different kinds of transformations.
Compass and straightedge constructions
Classical geometers paid special attention to constructing geometric objects that had been described in some other way. Classically, the only instruments used in most geometric constructions are thecompass
A compass is a device that shows the cardinal directions used for navigation and geographic orientation. It commonly consists of a magnetized needle or other element, such as a compass card or compass rose, which can pivot to align itself with ...
and straightedge
A straightedge or straight edge is a tool used for drawing straight lines, or checking their straightness. If it has equally spaced markings along its length, it is usually called a ruler.
Straightedges are used in the automotive service and ma ...
. Also, every construction had to be complete in a finite number of steps. However, some problems turned out to be difficult or impossible to solve by these means alone, and ingenious constructions using neusis
In geometry, the neusis (; ; plural: grc, νεύσεις, neuseis, label=none) is a geometric construction method that was used in antiquity by Greek mathematics, Greek mathematicians.
Geometric construction
The neusis construction consists ...
, parabolas and other curves, or mechanical devices, were found.
Dimension
 Where the traditional geometry allowed dimensions 1 (a
Where the traditional geometry allowed dimensions 1 (a line
Line most often refers to:
* Line (geometry), object with zero thickness and curvature that stretches to infinity
* Telephone line, a single-user circuit on a telephone communication system
Line, lines, The Line, or LINE may also refer to:
Arts ...
), 2 (a plane
Plane(s) most often refers to:
* Aero- or airplane, a powered, fixed-wing aircraft
* Plane (geometry), a flat, 2-dimensional surface
Plane or planes may also refer to:
Biology
* Plane (tree) or ''Platanus'', wetland native plant
* Planes (gen ...
) and 3 (our ambient world conceived of as three-dimensional space
Three-dimensional space (also: 3D space, 3-space or, rarely, tri-dimensional space) is a geometric setting in which three values (called ''parameters'') are required to determine the position (geometry), position of an element (i.e., Point (m ...
), mathematicians and physicists have used higher dimensions for nearly two centuries. One example of a mathematical use for higher dimensions is the configuration space of a physical system, which has a dimension equal to the system's degrees of freedom
Degrees of freedom (often abbreviated df or DOF) refers to the number of independent variables or parameters of a thermodynamic system. In various scientific fields, the word "freedom" is used to describe the limits to which physical movement or ...
. For instance, the configuration of a screw can be described by five coordinates.
In general topology, the concept of dimension has been extended from natural number
In mathematics, the natural numbers are those numbers used for counting (as in "there are ''six'' coins on the table") and ordering (as in "this is the ''third'' largest city in the country").
Numbers used for counting are called ''Cardinal n ...
s, to infinite dimension (Hilbert space
In mathematics, Hilbert spaces (named after David Hilbert) allow generalizing the methods of linear algebra and calculus from (finite-dimensional) Euclidean vector spaces to spaces that may be infinite-dimensional. Hilbert spaces arise natural ...
s, for example) and positive real number
In mathematics, a real number is a number that can be used to measure a ''continuous'' one-dimensional quantity such as a distance, duration or temperature. Here, ''continuous'' means that values can have arbitrarily small variations. Every real ...
s (in fractal geometry). In algebraic geometry
Algebraic geometry is a branch of mathematics, classically studying zeros of multivariate polynomials. Modern algebraic geometry is based on the use of abstract algebraic techniques, mainly from commutative algebra, for solving geometrical ...
, the dimension of an algebraic variety
In mathematics and specifically in algebraic geometry, the dimension of an algebraic variety may be defined in various equivalent ways.
Some of these definitions are of geometric nature, while some other are purely algebraic and rely on commutati ...
has received a number of apparently different definitions, which are all equivalent in the most common cases.
Symmetry
 The theme of
The theme of symmetry
Symmetry (from grc, συμμετρία "agreement in dimensions, due proportion, arrangement") in everyday language refers to a sense of harmonious and beautiful proportion and balance. In mathematics, "symmetry" has a more precise definit ...
in geometry is nearly as old as the science of geometry itself. Symmetric shapes such as the circle
A circle is a shape consisting of all points in a plane that are at a given distance from a given point, the centre. Equivalently, it is the curve traced out by a point that moves in a plane so that its distance from a given point is const ...
, regular polygons and platonic solid
In geometry, a Platonic solid is a convex, regular polyhedron in three-dimensional Euclidean space. Being a regular polyhedron means that the faces are congruent (identical in shape and size) regular polygons (all angles congruent and all edges c ...
s held deep significance for many ancient philosophers and were investigated in detail before the time of Euclid. Symmetric patterns occur in nature and were artistically rendered in a multitude of forms, including the graphics of Leonardo da Vinci
Leonardo di ser Piero da Vinci (15 April 14522 May 1519) was an Italian polymath of the High Renaissance who was active as a painter, Drawing, draughtsman, engineer, scientist, theorist, sculptor, and architect. While his fame initially res ...
, M. C. Escher
Maurits Cornelis Escher (; 17 June 1898 – 27 March 1972) was a Dutch graphic artist who made mathematically inspired woodcuts, lithographs, and mezzotints.
Despite wide popular interest, Escher was for most of his life neglected in t ...
, and others. In the second half of the 19th century, the relationship between symmetry and geometry came under intense scrutiny. Felix Klein's Erlangen program proclaimed that, in a very precise sense, symmetry, expressed via the notion of a transformation group, determines what geometry ''is''. Symmetry in classical Euclidean geometry is represented by congruences and rigid motions, whereas in projective geometry an analogous role is played by collineations, geometric transformations that take straight lines into straight lines. However it was in the new geometries of Bolyai and Lobachevsky, Riemann, Clifford Clifford may refer to:
People
*Clifford (name), an English given name and surname, includes a list of people with that name
*William Kingdon Clifford
*Baron Clifford
*Baron Clifford of Chudleigh
*Baron de Clifford
*Clifford baronets
*Clifford fami ...
and Klein, and Sophus Lie
Marius Sophus Lie ( ; ; 17 December 1842 – 18 February 1899) was a Norwegian mathematician. He largely created the theory of continuous symmetry and applied it to the study of geometry and differential equations.
Life and career
Marius Sophu ...
that Klein's idea to 'define a geometry via its symmetry group
In group theory, the symmetry group of a geometric object is the group of all transformations under which the object is invariant, endowed with the group operation of composition. Such a transformation is an invertible mapping of the ambient ...
' found its inspiration. Both discrete and continuous symmetries play prominent roles in geometry, the former in topology
In mathematics, topology (from the Greek language, Greek words , and ) is concerned with the properties of a mathematical object, geometric object that are preserved under Continuous function, continuous Deformation theory, deformations, such ...
and geometric group theory, the latter in Lie theory and Riemannian geometry.
A different type of symmetry is the principle of duality
Duality may refer to:
Mathematics
* Duality (mathematics), a mathematical concept
** Dual (category theory), a formalization of mathematical duality
** Duality (optimization)
** Duality (order theory), a concept regarding binary relations
** Dual ...
in projective geometry, among other fields. This meta-phenomenon can roughly be described as follows: in any theorem
In mathematics, a theorem is a statement that has been proved, or can be proved. The ''proof'' of a theorem is a logical argument that uses the inference rules of a deductive system to establish that the theorem is a logical consequence of th ...
, exchange ''point'' with ''plane'', ''join'' with ''meet'', ''lies in'' with ''contains'', and the result is an equally true theorem. A similar and closely related form of duality exists between a vector space
In mathematics and physics, a vector space (also called a linear space) is a set whose elements, often called ''vectors'', may be added together and multiplied ("scaled") by numbers called '' scalars''. Scalars are often real numbers, but can ...
and its dual space
In mathematics, any vector space ''V'' has a corresponding dual vector space (or just dual space for short) consisting of all linear forms on ''V'', together with the vector space structure of pointwise addition and scalar multiplication by const ...
.
Contemporary geometry
Euclidean geometry
Euclidean geometry is geometry in its classical sense. As it models the space of the physical world, it is used in many scientific areas, such asmechanics
Mechanics (from Ancient Greek: μηχανική, ''mēkhanikḗ'', "of machines") is the area of mathematics and physics concerned with the relationships between force, matter, and motion among physical objects. Forces applied to objects r ...
, astronomy
Astronomy () is a natural science that studies astronomical object, celestial objects and phenomena. It uses mathematics, physics, and chemistry in order to explain their origin and chronology of the Universe, evolution. Objects of interest ...
, crystallography
Crystallography is the experimental science of determining the arrangement of atoms in crystalline solids. Crystallography is a fundamental subject in the fields of materials science and solid-state physics (condensed matter physics). The wor ...
, and many technical fields, such as engineering
Engineering is the use of scientific method, scientific principles to design and build machines, structures, and other items, including bridges, tunnels, roads, vehicles, and buildings. The discipline of engineering encompasses a broad rang ...
, architecture
Architecture is the art and technique of designing and building, as distinguished from the skills associated with construction. It is both the process and the product of sketching, conceiving, planning, designing, and constructing building ...
, geodesy
Geodesy ( ) is the Earth science of accurately measuring and understanding Earth's figure (geometric shape and size), orientation in space, and gravity. The field also incorporates studies of how these properties change over time and equivale ...
, aerodynamics
Aerodynamics, from grc, ἀήρ ''aero'' (air) + grc, δυναμική (dynamics), is the study of the motion of air, particularly when affected by a solid object, such as an airplane wing. It involves topics covered in the field of fluid dyn ...
, and navigation
Navigation is a field of study that focuses on the process of monitoring and controlling the movement of a craft or vehicle from one place to another.Bowditch, 2003:799. The field of navigation includes four general categories: land navigation, ...
. The mandatory educational curriculum of the majority of nations includes the study of Euclidean concepts such as points
Point or points may refer to:
Places
* Point, Lewis, a peninsula in the Outer Hebrides, Scotland
* Point, Texas, a city in Rains County, Texas, United States
* Point, the NE tip and a ferry terminal of Lismore, Inner Hebrides, Scotland
* Point ...
, lines
Line most often refers to:
* Line (geometry), object with zero thickness and curvature that stretches to infinity
* Telephone line, a single-user circuit on a telephone communication system
Line, lines, The Line, or LINE may also refer to:
Arts ...
, planes
Plane(s) most often refers to:
* Aero- or airplane, a powered, fixed-wing aircraft
* Plane (geometry), a flat, 2-dimensional surface
Plane or planes may also refer to:
Biology
* Plane (tree) or ''Platanus'', wetland native plant
* ''Planes' ...
, angle
In Euclidean geometry, an angle is the figure formed by two Ray (geometry), rays, called the ''Side (plane geometry), sides'' of the angle, sharing a common endpoint, called the ''vertex (geometry), vertex'' of the angle.
Angles formed by two ...
s, triangle
A triangle is a polygon with three Edge (geometry), edges and three Vertex (geometry), vertices. It is one of the basic shapes in geometry. A triangle with vertices ''A'', ''B'', and ''C'' is denoted \triangle ABC.
In Euclidean geometry, an ...
s, congruence
Congruence may refer to:
Mathematics
* Congruence (geometry), being the same size and shape
* Congruence or congruence relation, in abstract algebra, an equivalence relation on an algebraic structure that is compatible with the structure
* In mod ...
, similarity, solid figures, circle
A circle is a shape consisting of all points in a plane that are at a given distance from a given point, the centre. Equivalently, it is the curve traced out by a point that moves in a plane so that its distance from a given point is const ...
s, and analytic geometry
In classical mathematics, analytic geometry, also known as coordinate geometry or Cartesian geometry, is the study of geometry using a coordinate system. This contrasts with synthetic geometry.
Analytic geometry is used in physics and engineerin ...
.
Differential geometry
Differential geometry
Differential geometry is a mathematical discipline that studies the geometry of smooth shapes and smooth spaces, otherwise known as smooth manifolds. It uses the techniques of differential calculus, integral calculus, linear algebra and multili ...
uses techniques of calculus
Calculus, originally called infinitesimal calculus or "the calculus of infinitesimals", is the mathematical study of continuous change, in the same way that geometry is the study of shape, and algebra is the study of generalizations of arithm ...
and linear algebra
Linear algebra is the branch of mathematics concerning linear equations such as:
:a_1x_1+\cdots +a_nx_n=b,
linear maps such as:
:(x_1, \ldots, x_n) \mapsto a_1x_1+\cdots +a_nx_n,
and their representations in vector spaces and through matrices.
...
to study problems in geometry. It has applications in physics
Physics is the natural science that studies matter, its fundamental constituents, its motion and behavior through space and time, and the related entities of energy and force. "Physical science is that department of knowledge which r ...
, econometrics
Econometrics is the application of Statistics, statistical methods to economic data in order to give Empirical evidence, empirical content to economic relationships.M. Hashem Pesaran (1987). "Econometrics," ''The New Palgrave: A Dictionary of ...
, and bioinformatics
Bioinformatics () is an interdisciplinary field that develops methods and software tools for understanding biological data, in particular when the data sets are large and complex. As an interdisciplinary field of science, bioinformatics combi ...
, among others.
In particular, differential geometry is of importance to mathematical physics
Mathematical physics refers to the development of mathematics, mathematical methods for application to problems in physics. The ''Journal of Mathematical Physics'' defines the field as "the application of mathematics to problems in physics and t ...
due to Albert Einstein
Albert Einstein ( ; ; 14 March 1879 – 18 April 1955) was a German-born theoretical physicist, widely acknowledged to be one of the greatest and most influential physicists of all time. Einstein is best known for developing the theory ...
's general relativity
General relativity, also known as the general theory of relativity and Einstein's theory of gravity, is the geometric theory of gravitation published by Albert Einstein in 1915 and is the current description of gravitation in modern physics ...
postulation that the universe
The universe is all of space and time and their contents, including planets, stars, galaxies, and all other forms of matter and energy. The Big Bang theory is the prevailing cosmological description of the development of the universe. Acc ...
is curved
In mathematics, a curve (also called a curved line in older texts) is an object similar to a line, but that does not have to be straight.
Intuitively, a curve may be thought of as the trace left by a moving point. This is the definition that ap ...
. Differential geometry can either be ''intrinsic'' (meaning that the spaces it considers are smooth manifolds whose geometric structure is governed by a Riemannian metric, which determines how distances are measured near each point) or ''extrinsic'' (where the object under study is a part of some ambient flat Euclidean space).
Non-Euclidean geometry
Euclidean geometry was not the only historical form of geometry studied.Spherical geometry
300px, A sphere with a spherical triangle on it.
Spherical geometry is the geometry of the two-dimensional surface of a sphere. In this context the word "sphere" refers only to the 2-dimensional surface and other terms like "ball" or "solid sp ...
has long been used by astronomers, astrologers, and navigators.
Immanuel Kant
Immanuel Kant (, , ; 22 April 1724 – 12 February 1804) was a German philosopher and one of the central Enlightenment thinkers. Born in Königsberg, Kant's comprehensive and systematic works in epistemology, metaphysics, ethics, and ...
argued that there is only one, ''absolute'', geometry, which is known to be true ''a priori'' by an inner faculty of mind: Euclidean geometry was synthetic a priori. This view was at first somewhat challenged by thinkers such as Saccheri, then finally overturned by the revolutionary discovery of non-Euclidean geometry in the works of Bolyai, Lobachevsky, and Gauss (who never published his theory). They demonstrated that ordinary Euclidean space
Euclidean space is the fundamental space of geometry, intended to represent physical space. Originally, that is, in Euclid's Elements, Euclid's ''Elements'', it was the three-dimensional space of Euclidean geometry, but in modern mathematics ther ...
is only one possibility for development of geometry. A broad vision of the subject of geometry was then expressed by Riemann in his 1867 inauguration lecture ''Über die Hypothesen, welche der Geometrie zu Grunde liegen'' (''On the hypotheses on which geometry is based''), published only after his death. Riemann's new idea of space proved crucial in Albert Einstein
Albert Einstein ( ; ; 14 March 1879 – 18 April 1955) was a German-born theoretical physicist, widely acknowledged to be one of the greatest and most influential physicists of all time. Einstein is best known for developing the theory ...
's general relativity theory
General relativity, also known as the general theory of relativity and Einstein's theory of gravity, is the geometric theory of gravitation published by Albert Einstein in 1915 and is the current description of gravitation in modern physics. G ...
. Riemannian geometry, which considers very general spaces in which the notion of length is defined, is a mainstay of modern geometry.
Topology

Topology
In mathematics, topology (from the Greek language, Greek words , and ) is concerned with the properties of a mathematical object, geometric object that are preserved under Continuous function, continuous Deformation theory, deformations, such ...
is the field concerned with the properties of continuous mappings, and can be considered a generalization of Euclidean geometry. In practice, topology often means dealing with large-scale properties of spaces, such as connectedness and compactness
In mathematics, specifically general topology, compactness is a property that seeks to generalize the notion of a closed and bounded subset of Euclidean space by making precise the idea of a space having no "punctures" or "missing endpoints", i ...
.
The field of topology, which saw massive development in the 20th century, is in a technical sense a type of transformation geometry
In mathematics, transformation geometry (or transformational geometry) is the name of a mathematical and pedagogic take on the study of geometry by focusing on groups of geometric transformations, and properties that are invariant under them. ...
, in which transformations are homeomorphism
In the mathematical field of topology, a homeomorphism, topological isomorphism, or bicontinuous function is a bijective and continuous function between topological spaces that has a continuous inverse function. Homeomorphisms are the isomorphi ...
s. This has often been expressed in the form of the saying 'topology is rubber-sheet geometry'. Subfields of topology include geometric topology
In mathematics, geometric topology is the study of manifolds and maps between them, particularly embeddings of one manifold into another.
History
Geometric topology as an area distinct from algebraic topology may be said to have originated i ...
, differential topology
In mathematics, differential topology is the field dealing with the topological properties and smooth properties of smooth manifolds. In this sense differential topology is distinct from the closely related field of differential geometry, which ...
, algebraic topology
Algebraic topology is a branch of mathematics that uses tools from abstract algebra to study topological spaces. The basic goal is to find algebraic invariant (mathematics), invariants that classification theorem, classify topological spaces up t ...
and general topology.
Algebraic geometry
 The field of
The field of algebraic geometry
Algebraic geometry is a branch of mathematics, classically studying zeros of multivariate polynomials. Modern algebraic geometry is based on the use of abstract algebraic techniques, mainly from commutative algebra, for solving geometrical ...
developed from the Cartesian geometry of co-ordinates
In geometry, a coordinate system is a system that uses one or more numbers, or coordinates, to uniquely determine the position of the points or other geometric elements on a manifold such as Euclidean space. The order of the coordinates is sign ...
. It underwent periodic periods of growth, accompanied by the creation and study of projective geometry, birational geometry, algebraic varieties, and commutative algebra
Commutative algebra, first known as ideal theory, is the branch of algebra that studies commutative rings, their ideals, and modules over such rings. Both algebraic geometry and algebraic number theory build on commutative algebra. Prominent ...
, among other topics. From the late 1950s through the mid-1970s it had undergone major foundational development, largely due to work of Jean-Pierre Serre and Alexander Grothendieck. This led to the introduction of schemes and greater emphasis on topological methods, including various cohomology theories
In mathematics, specifically in homology theory and algebraic topology, cohomology is a general term for a sequence of abelian groups, usually one associated with a topological space, often defined from a cochain complex. Cohomology can be viewed ...
. One of seven Millennium Prize problems
The Millennium Prize Problems are seven well-known complex mathematical problems selected by the Clay Mathematics Institute in 2000. The Clay Institute has pledged a US$1 million prize for the first correct solution to each problem. According t ...
, the Hodge conjecture, is a question in algebraic geometry. Wiles' proof of Fermat's Last Theorem uses advanced methods of algebraic geometry for solving a long-standing problem of number theory
Number theory (or arithmetic or higher arithmetic in older usage) is a branch of pure mathematics devoted primarily to the study of the integers and arithmetic function, integer-valued functions. German mathematician Carl Friedrich Gauss (1777� ...
.
In general, algebraic geometry studies geometry through the use of concepts in commutative algebra
Commutative algebra, first known as ideal theory, is the branch of algebra that studies commutative rings, their ideals, and modules over such rings. Both algebraic geometry and algebraic number theory build on commutative algebra. Prominent ...
such as multivariate polynomials. It has applications in many areas, including cryptography
Cryptography, or cryptology (from grc, , translit=kryptós "hidden, secret"; and ''graphein'', "to write", or ''-logia'', "study", respectively), is the practice and study of techniques for secure communication in the presence of adver ...
and string theory
In physics, string theory is a theoretical framework in which the point-like particles of particle physics are replaced by one-dimensional objects called strings. String theory describes how these strings propagate through space and interac ...
.
Complex geometry
Complex geometry studies the nature of geometric structures modelled on, or arising out of, thecomplex plane
In mathematics, the complex plane is the plane formed by the complex numbers, with a Cartesian coordinate system such that the -axis, called the real axis, is formed by the real numbers, and the -axis, called the imaginary axis, is formed by the ...
. Complex geometry lies at the intersection of differential geometry, algebraic geometry, and analysis of several complex variables
The theory of functions of several complex variables is the branch of mathematics dealing with complex-valued functions. The name of the field dealing with the properties of function of several complex variables is called several complex variable ...
, and has found applications to string theory
In physics, string theory is a theoretical framework in which the point-like particles of particle physics are replaced by one-dimensional objects called strings. String theory describes how these strings propagate through space and interac ...
and mirror symmetry.
Complex geometry first appeared as a distinct area of study in the work of Bernhard Riemann
Georg Friedrich Bernhard Riemann (; 17 September 1826 – 20 July 1866) was a German mathematician who made contributions to analysis, number theory, and differential geometry. In the field of real analysis, he is mostly known for the first rig ...
in his study of Riemann surface
In mathematics, particularly in complex analysis, a Riemann surface is a connected one-dimensional complex manifold. These surfaces were first studied by and are named after Bernhard Riemann. Riemann surfaces can be thought of as deformed vers ...
s. Work in the spirit of Riemann was carried out by the Italian school of algebraic geometry
In relation to the history of mathematics, the Italian school of algebraic geometry refers to mathematicians and their work in birational geometry, particularly on algebraic surfaces, centered around Rome roughly from 1885 to 1935. There were 30 ...
in the early 1900s. Contemporary treatment of complex geometry began with the work of Jean-Pierre Serre, who introduced the concept of sheaves to the subject, and illuminated the relations between complex geometry and algebraic geometry.
The primary objects of study in complex geometry are complex manifold
In differential geometry and complex geometry, a complex manifold is a manifold with an atlas of charts to the open unit disc in \mathbb^n, such that the transition maps are holomorphic.
The term complex manifold is variously used to mean a com ...
s, complex algebraic varieties
In algebraic geometry, a complex algebraic variety is an algebraic variety (in the scheme sense or otherwise) over the field of complex numbers. Parshin, Alexei N., and Igor Rostislavovich Shafarevich, eds. ''Algebraic Geometry III: Complex Algeb ...
, and complex analytic varieties
In mathematics, and in particular differential geometry and complex geometry, a complex analytic variety Complex analytic variety (or just variety) is sometimes required to be irreducible
and (or) reduced or complex analytic space is a generaliz ...
, and holomorphic vector bundles In mathematics, a holomorphic vector bundle is a complex vector bundle over a complex manifold such that the total space is a complex manifold and the projection map is holomorphic. Fundamental examples are the holomorphic tangent bundle of a com ...
and coherent sheaves over these spaces. Special examples of spaces studied in complex geometry include Riemann surfaces, and Calabi–Yau manifold
In algebraic geometry, a Calabi–Yau manifold, also known as a Calabi–Yau space, is a particular type of manifold which has properties, such as Ricci flatness, yielding applications in theoretical physics. Particularly in superstring ...
s, and these spaces find uses in string theory. In particular, worldsheet
In string theory, a worldsheet is a two-dimensional manifold which describes the embedding of a string in spacetime. The term was coined by Leonard Susskind as a direct generalization of the world line concept for a point particle in special and ...
s of strings are modelled by Riemann surfaces, and superstring theory predicts that the extra 6 dimensions of 10 dimensional spacetime
In physics, spacetime is a mathematical model that combines the three dimensions of space and one dimension of time into a single four-dimensional manifold. Spacetime diagrams can be used to visualize relativistic effects, such as why differen ...
may be modelled by Calabi–Yau manifolds.
Discrete geometry
convex geometry
In mathematics, convex geometry is the branch of geometry studying convex sets, mainly in Euclidean space. Convex sets occur naturally in many areas: computational geometry, convex analysis, discrete geometry, functional analysis, geometry of numbe ...
. It is concerned mainly with questions of relative position of simple geometric objects, such as points, lines and circles. Examples include the study of sphere packing
In geometry, a sphere packing is an arrangement of non-overlapping spheres within a containing space. The spheres considered are usually all of identical size, and the space is usually three-dimensional Euclidean space. However, sphere packing p ...
s, triangulations, the Kneser-Poulsen conjecture, etc. It shares many methods and principles with combinatorics
Combinatorics is an area of mathematics primarily concerned with counting, both as a means and an end in obtaining results, and certain properties of finite structures. It is closely related to many other areas of mathematics and has many appl ...
.
Computational geometry
Computational geometry
Computational geometry is a branch of computer science devoted to the study of algorithms which can be stated in terms of geometry. Some purely geometrical problems arise out of the study of computational geometric algorithms, and such problems ar ...
deals with algorithm
In mathematics and computer science, an algorithm () is a finite sequence of rigorous instructions, typically used to solve a class of specific Computational problem, problems or to perform a computation. Algorithms are used as specificat ...
s and their implementations for manipulating geometrical objects. Important problems historically have included the travelling salesman problem, minimum spanning tree
A minimum spanning tree (MST) or minimum weight spanning tree is a subset of the edges of a connected, edge-weighted undirected graph that connects all the vertices together, without any cycles and with the minimum possible total edge weight. T ...
s, hidden-line removal
In 3D computer graphics, solid objects are usually modeled by polyhedra. A face of a polyhedron is a planar polygon bounded by straight line segments, called edges. Curved surfaces are usually approximated by a polygon mesh. Computer programs ...
, and linear programming
Linear programming (LP), also called linear optimization, is a method to achieve the best outcome (such as maximum profit or lowest cost) in a mathematical model whose requirements are represented by linear function#As a polynomial function, li ...
.
Although being a young area of geometry, it has many applications in computer vision
Computer vision is an interdisciplinary scientific field that deals with how computers can gain high-level understanding from digital images or videos. From the perspective of engineering, it seeks to understand and automate tasks that the hum ...
, image processing
An image is a visual representation of something. It can be two-dimensional, three-dimensional, or somehow otherwise feed into the visual system to convey information. An image can be an artifact, such as a photograph or other two-dimensiona ...
, computer-aided design
Computer-aided design (CAD) is the use of computers (or ) to aid in the creation, modification, analysis, or optimization of a design. This software is used to increase the productivity of the designer, improve the quality of design, improve c ...
, medical imaging
Medical imaging is the technique and process of imaging the interior of a body for clinical analysis and medical intervention, as well as visual representation of the function of some organs or tissues (physiology). Medical imaging seeks to rev ...
, etc.
Geometric group theory
Geometric group theory uses large-scale geometric techniques to study finitely generated groups. It is closely connected to low-dimensional topology, such as in Grigori Perelman's proof of the Geometrization conjecture, which included the proof of thePoincaré conjecture
In the mathematics, mathematical field of geometric topology, the Poincaré conjecture (, , ) is a theorem about the Characterization (mathematics), characterization of the 3-sphere, which is the hypersphere that bounds the unit ball in four-dim ...
, a Millennium Prize Problem
The Millennium Prize Problems are seven well-known complex mathematical problems selected by the Clay Mathematics Institute in 2000. The Clay Institute has pledged a US$1 million prize for the first correct solution to each problem. According ...
.
Geometric group theory often revolves around the Cayley graph, which is a geometric representation of a group. Other important topics include quasi-isometries, Gromov-hyperbolic group
In group theory, more precisely in geometric group theory, a hyperbolic group, also known as a ''word hyperbolic group'' or ''Gromov hyperbolic group'', is a finitely generated group equipped with a word metric satisfying certain properties abstra ...
s, and right angled Artin groups.
Convex geometry
Convex geometry
In mathematics, convex geometry is the branch of geometry studying convex sets, mainly in Euclidean space. Convex sets occur naturally in many areas: computational geometry, convex analysis, discrete geometry, functional analysis, geometry of numbe ...
investigates convex shapes in the Euclidean space and its more abstract analogues, often using techniques of real analysis and discrete mathematics
Discrete mathematics is the study of mathematical structures that can be considered "discrete" (in a way analogous to discrete variables, having a bijection with the set of natural numbers) rather than "continuous" (analogously to continuous f ...
. It has close connections to convex analysis, optimization and functional analysis
Functional analysis is a branch of mathematical analysis, the core of which is formed by the study of vector spaces endowed with some kind of limit-related structure (e.g. Inner product space#Definition, inner product, Norm (mathematics)#Defini ...
and important applications in number theory
Number theory (or arithmetic or higher arithmetic in older usage) is a branch of pure mathematics devoted primarily to the study of the integers and arithmetic function, integer-valued functions. German mathematician Carl Friedrich Gauss (1777� ...
.
Convex geometry dates back to antiquity. Archimedes
Archimedes of Syracuse (;; ) was a Greek mathematician, physicist, engineer, astronomer, and inventor from the ancient city of Syracuse in Sicily. Although few details of his life are known, he is regarded as one of the leading scientists ...
gave the first known precise definition of convexity. The isoperimetric problem
In mathematics, the isoperimetric inequality is a geometric inequality involving the perimeter of a set and its volume. In n-dimensional space \R^n the inequality lower bounds the surface area or perimeter \operatorname(S) of a set S\subset\R^n by ...
, a recurring concept in convex geometry, was studied by the Greeks as well, including Zenodorus. Archimedes, Plato
Plato ( ; grc-gre, Πλάτων ; 428/427 or 424/423 – 348/347 BC) was a Greek philosopher born in Athens during the Classical period in Ancient Greece. He founded the Platonist school of thought and the Academy, the first institution ...
, Euclid
Euclid (; grc-gre, Wikt:Εὐκλείδης, Εὐκλείδης; BC) was an ancient Greek mathematician active as a geometer and logician. Considered the "father of geometry", he is chiefly known for the ''Euclid's Elements, Elements'' trea ...
, and later Kepler
Johannes Kepler (; ; 27 December 1571 – 15 November 1630) was a German astronomer, mathematician, astrologer, natural philosopher and writer on music. He is a key figure in the 17th-century Scientific Revolution, best known for his laws o ...
and Coxeter all studied convex polytope
A convex polytope is a special case of a polytope, having the additional property that it is also a convex set contained in the n-dimensional Euclidean space \mathbb^n. Most texts. use the term "polytope" for a bounded convex polytope, and the wo ...
s and their properties. From the 19th century on, mathematicians have studied other areas of convex mathematics, including higher-dimensional polytopes, volume and surface area of convex bodies, Gaussian curvature
In differential geometry, the Gaussian curvature or Gauss curvature of a surface at a point is the product of the principal curvatures, and , at the given point:
K = \kappa_1 \kappa_2.
The Gaussian radius of curvature is the reciprocal of .
F ...
, algorithms
In mathematics and computer science, an algorithm () is a finite sequence of rigorous instructions, typically used to solve a class of specific problems or to perform a computation. Algorithms are used as specifications for performing c ...
, tilings and lattices.
Applications
Geometry has found applications in many fields, some of which are described below.Art
 Mathematics and art are related in a variety of ways. For instance, the theory of perspective showed that there is more to geometry than just the metric properties of figures: perspective is the origin of projective geometry.
Artists have long used concepts of
Mathematics and art are related in a variety of ways. For instance, the theory of perspective showed that there is more to geometry than just the metric properties of figures: perspective is the origin of projective geometry.
Artists have long used concepts of proportion
Proportionality, proportion or proportional may refer to:
Mathematics
* Proportionality (mathematics), the property of two variables being in a multiplicative relation to a constant
* Ratio, of one quantity to another, especially of a part compare ...
in design. Vitruvius
Vitruvius (; c. 80–70 BC – after c. 15 BC) was a Roman architect and engineer during the 1st century BC, known for his multi-volume work entitled ''De architectura''. He originated the idea that all buildings should have three attribute ...
developed a complicated theory of ''ideal proportions'' for the human figure. These concepts have been used and adapted by artists from Michelangelo
Michelangelo di Lodovico Buonarroti Simoni (; 6 March 1475 – 18 February 1564), known as Michelangelo (), was an Italian sculptor, painter, architect, and poet of the High Renaissance. Born in the Republic of Florence, his work was insp ...
to modern comic book artists.
The golden ratio is a particular proportion that has had a controversial role in art. Often claimed to be the most aesthetically pleasing ratio of lengths, it is frequently stated to be incorporated into famous works of art, though the most reliable and unambiguous examples were made deliberately by artists aware of this legend.
Tilings, or tessellations, have been used in art throughout history. Islamic art makes frequent use of tessellations, as did the art of M. C. Escher
Maurits Cornelis Escher (; 17 June 1898 – 27 March 1972) was a Dutch graphic artist who made mathematically inspired woodcuts, lithographs, and mezzotints.
Despite wide popular interest, Escher was for most of his life neglected in t ...
. Escher's work also made use of hyperbolic geometry.
Cézanne advanced the theory that all images can be built up from the sphere
A sphere () is a Geometry, geometrical object that is a solid geometry, three-dimensional analogue to a two-dimensional circle. A sphere is the Locus (mathematics), set of points that are all at the same distance from a given point in three ...
, the cone, and the cylinder
A cylinder (from ) has traditionally been a three-dimensional solid, one of the most basic of curvilinear geometric shapes. In elementary geometry, it is considered a prism with a circle as its base.
A cylinder may also be defined as an infin ...
. This is still used in art theory today, although the exact list of shapes varies from author to author.
Architecture
Geometry has many applications in architecture. In fact, it has been said that geometry lies at the core of architectural design. Applications of geometry to architecture include the use of projective geometry to createforced perspective
Forced perspective is a technique that employs optical illusion to make an object appear farther away, closer, larger or smaller than it actually is. It manipulates human visual perception through the use of scaled objects and the correlation ...
, the use of conic section
In mathematics, a conic section, quadratic curve or conic is a curve obtained as the intersection of the surface of a cone with a plane. The three types of conic section are the hyperbola, the parabola, and the ellipse; the circle is a specia ...
s in constructing domes and similar objects, the use of tessellations
A tessellation or tiling is the covering of a surface, often a plane (mathematics), plane, using one or more geometric shapes, called ''tiles'', with no overlaps and no gaps. In mathematics, tessellation can be generalized to high-dimensional ...
, and the use of symmetry.
Physics
The field ofastronomy
Astronomy () is a natural science that studies astronomical object, celestial objects and phenomena. It uses mathematics, physics, and chemistry in order to explain their origin and chronology of the Universe, evolution. Objects of interest ...
, especially as it relates to mapping the positions of star
A star is an astronomical object comprising a luminous spheroid of plasma (physics), plasma held together by its gravity. The List of nearest stars and brown dwarfs, nearest star to Earth is the Sun. Many other stars are visible to the naked ...
s and planet
A planet is a large, rounded astronomical body that is neither a star nor its remnant. The best available theory of planet formation is the nebular hypothesis, which posits that an interstellar cloud collapses out of a nebula to create a you ...
s on the celestial sphere
In astronomy and navigation, the celestial sphere is an abstract sphere that has an arbitrarily large radius and is concentric to Earth. All objects in the sky can be conceived as being projected upon the inner surface of the celestial sphere, ...
and describing the relationship between movements of celestial bodies, have served as an important source of geometric problems throughout history.
Riemannian geometry and pseudo-Riemannian
In differential geometry, a pseudo-Riemannian manifold, also called a semi-Riemannian manifold, is a differentiable manifold with a metric tensor that is everywhere nondegenerate. This is a generalization of a Riemannian manifold in which the r ...
geometry are used in general relativity
General relativity, also known as the general theory of relativity and Einstein's theory of gravity, is the geometric theory of gravitation published by Albert Einstein in 1915 and is the current description of gravitation in modern physics ...
. String theory
In physics, string theory is a theoretical framework in which the point-like particles of particle physics are replaced by one-dimensional objects called strings. String theory describes how these strings propagate through space and interac ...
makes use of several variants of geometry, as does quantum information theory.
Other fields of mathematics
Calculus
Calculus, originally called infinitesimal calculus or "the calculus of infinitesimals", is the mathematical study of continuous change, in the same way that geometry is the study of shape, and algebra is the study of generalizations of arithm ...
was strongly influenced by geometry. For instance, the introduction of coordinates
In geometry, a coordinate system is a system that uses one or more numbers, or coordinates, to uniquely determine the position of the points or other geometric elements on a manifold such as Euclidean space. The order of the coordinates is sig ...
by René Descartes
René Descartes ( or ; ; Latinized: Renatus Cartesius; 31 March 1596 – 11 February 1650) was a French philosopher, scientist, and mathematician, widely considered a seminal figure in the emergence of modern philosophy and science. Mathem ...
and the concurrent developments of algebra
Algebra () is one of the broad areas of mathematics. Roughly speaking, algebra is the study of mathematical symbols and the rules for manipulating these symbols in formulas; it is a unifying thread of almost all of mathematics.
Elementary a ...
marked a new stage for geometry, since geometric figures such as plane curves could now be represented analytically in the form of functions and equations. This played a key role in the emergence of infinitesimal calculus
Calculus, originally called infinitesimal calculus or "the calculus of infinitesimals", is the mathematical study of continuous change, in the same way that geometry is the study of shape, and algebra is the study of generalizations of arithm ...
in the 17th century. Analytic geometry continues to be a mainstay of pre-calculus and calculus curriculum.
Another important area of application is number theory
Number theory (or arithmetic or higher arithmetic in older usage) is a branch of pure mathematics devoted primarily to the study of the integers and arithmetic function, integer-valued functions. German mathematician Carl Friedrich Gauss (1777� ...
. In ancient Greece
Ancient Greece ( el, Ἑλλάς, Hellás) was a northeastern Mediterranean civilization, existing from the Greek Dark Ages of the 12th–9th centuries BC to the end of classical antiquity ( AD 600), that comprised a loose collection of cult ...
the Pythagoreans considered the role of numbers in geometry. However, the discovery of incommensurable lengths contradicted their philosophical views. Since the 19th century, geometry has been used for solving problems in number theory, for example through the geometry of numbers Geometry of numbers is the part of number theory which uses geometry for the study of algebraic numbers. Typically, a ring of algebraic integers is viewed as a lattice in \mathbb R^n, and the study of these lattices provides fundamental information ...
or, more recently, scheme theory
In mathematics, a scheme is a mathematical structure that enlarges the notion of algebraic variety in several ways, such as taking account of multiplicities (the equations ''x'' = 0 and ''x''2 = 0 define the same algebraic variety but different sc ...
, which is used in Wiles's proof of Fermat's Last Theorem.
See also
Lists
* List of geometers ** :Algebraic geometers ** :Differential geometers ** :Geometers ** :Topologists *List of formulas in elementary geometry
This is a short list of some common mathematical shapes and figures and the formulas that describe them.
Two-dimensional shapes
:Sources:
Three-dimensional shapes
:Sources:
Sphere
The basic quantities describing a sphere (meanin ...
* List of geometry topics
* List of important publications in geometry
* Lists of mathematics topics
Lists of mathematics topics cover a variety of topics related to mathematics. Some of these lists link to hundreds of articles; some link only to a few. The template to the right includes links to alphabetical lists of all mathematical articles. T ...
Related topics
*Descriptive geometry
Descriptive geometry is the branch of geometry which allows the representation of three-dimensional objects in two dimensions by using a specific set of procedures. The resulting techniques are important for engineering, architecture, design and ...
* Finite geometry
* '' Flatland'', a book written by Edwin Abbott Abbott about two- and three-dimensional space
Three-dimensional space (also: 3D space, 3-space or, rarely, tri-dimensional space) is a geometric setting in which three values (called ''parameters'') are required to determine the position (geometry), position of an element (i.e., Point (m ...
, to understand the concept of four dimensions
* List of interactive geometry software
Other fields
* Molecular geometryNotes
Sources
* * * * *Further reading
* *External links
* Ageometry
Geometry (; ) is, with arithmetic, one of the oldest branches of mathematics. It is concerned with properties of space such as the distance, shape, size, and relative position of figures. A mathematician who works in the field of geometry is c ...
course from Wikiversity
Wikiversity is a Wikimedia Foundation project that supports learning communities, their learning materials, and resulting activities. It differs from Wikipedia in that it offers tutorials and other materials for the fostering of learning, rather ...
''Unusual Geometry Problems''
''The Math Forum'' – Geometry
*
*
*
Nature Precedings – ''Pegs and Ropes Geometry at Stonehenge''
* ttps://web.archive.org/web/20071004174210/http://www.gresham.ac.uk/event.asp?PageId=45&EventId=618 "4000 Years of Geometry" lecture by Robin Wilson given at Gresham College, 3 October 2007 (available for MP3 and MP4 download as well as a text file) *
Finitism in Geometry
at the Stanford Encyclopedia of Philosophy
Interactive geometry reference with hundreds of applets
Geometry classes
at
Khan Academy
Khan Academy is an American non-profit educational organization created in 2008 by Sal Khan. Its goal is creating a set of online tools that help educate students. The organization produces short lessons in the form of videos. Its website also in ...
{{Authority control