|
Archimedes
Archimedes of Syracuse (;; ) was a Greek mathematician, physicist, engineer, astronomer, and inventor from the ancient city of Syracuse in Sicily. Although few details of his life are known, he is regarded as one of the leading scientists in classical antiquity. Considered the greatest mathematician of ancient history, and one of the greatest of all time,* * * * * * * * * * Archimedes anticipated modern calculus and analysis by applying the concept of the infinitely small and the method of exhaustion to derive and rigorously prove a range of geometrical theorems. These include the area of a circle, the surface area and volume of a sphere, the area of an ellipse, the area under a parabola, the volume of a segment of a paraboloid of revolution, the volume of a segment of a hyperboloid of revolution, and the area of a spiral. Heath, Thomas L. 1897. ''Works of Archimedes''. Archimedes' other mathematical achievements include deriving an approximation of pi, de ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Archimedes' Screw
The Archimedes screw, also known as the Archimedean screw, hydrodynamic screw, water screw or Egyptian screw, is one of the earliest hydraulic machines. Using Archimedes screws as water pumps (Archimedes screw pump (ASP) or screw pump) dates back many centuries. As a machine used for transferring water from a low-lying body of water into irrigation ditches, water is pumped by turning a screw-shaped surface inside a pipe. In the modern world, Archimedes screw pumps are widely used in wastewater treatment plants and for dewatering low-lying regions. Archimedes Screws Turbines (ASTs) are a new form of small hydroelectric powerplant that can be applied even in low head sites. Archimedes screw generators operate in a wide range of flows (0.01 m^3/s to 14.5 m^3/s) and heads (0.1 m to 10 m), including low heads and moderate flow rates that is not ideal for traditional turbines and not occupied by high performance technologies. The Archimedes screw is a reversible hydraulic machine, a ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
List Of Things Named After Archimedes
Archimedes (c. 287 BC – c. 212 BC) is the eponym of all of the things (and topics) listed below. Mathematical concepts * Archimedean absolute value * Archimedean circle * Archimedean copula * Archimedean group * Archimedean ordered field * Archimedean point *Archimedean property *Archimedean solid *Archimedean spiral * Archimedean tiling * Archimedes' axiom *Archimedes' cattle problem * Archimedes' hat-box theorem * Archimedes constant *Archimedes number * Archimedes' quadruplets * Archimedes Square * Archimedes' twin circles * Heron–Archimedes formula *Non-Archimedean geometry *Non-Archimedean ordered field * Archimedes' ostomachion Physical concepts * Archimedes paradox *Archimedes' principle Technology Things invented by Archimedes * Archimedes' pulley *Archimedes' screw ** Archimedean turbine * Archimedes heat ray * Claw of Archimedes * Trammel of Archimedes Other * Archimedes bridge * Archimede combined cycle power plant * SS Archimedes * Archimedes Group ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Archimedes' Use Of Infinitesimals
''The Method of Mechanical Theorems'' ( el, Περὶ μηχανικῶν θεωρημάτων πρὸς Ἐρατοσθένη ἔφοδος), also referred to as ''The Method'', is one of the major surviving works of the ancient Greek polymath Archimedes. ''The Method'' takes the form of a letter from Archimedes to Eratosthenes, the chief librarian at the Library of Alexandria, and contains the first attested explicit use of indivisibles (sometimes erroneously referred to as infinitesimals). The work was originally thought to be lost, but in 1906 was rediscovered in the celebrated Archimedes Palimpsest. The palimpsest includes Archimedes' account of the "mechanical method", so called because it relies on the center of weights of figures (centroid) and the law of the lever, which were demonstrated by Archimedes in '' On the Equilibrium of Planes''. Archimedes did not admit the method of indivisibles as part of rigorous mathematics, and therefore did not publish his method in t ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Archimedes' Principle
Archimedes' principle (also spelled Archimedes's principle) states that the upward buoyant force that is exerted on a body immersed in a fluid, whether fully or partially, is equal to the weight of the fluid that the body displaces. Archimedes' principle is a law of physics fundamental to fluid mechanics. It was formulated by Archimedes of Syracuse. Explanation In '' On Floating Bodies'', Archimedes suggested that (c. 246 BC): Archimedes' principle allows the buoyancy of any floating object partially or fully immersed in a fluid to be calculated. The downward force on the object is simply its weight. The upward, or buoyant, force on the object is that stated by Archimedes' principle above. Thus, the net force on the object is the difference between the magnitudes of the buoyant force and its weight. If this net force is positive, the object rises; if negative, the object sinks; and if zero, the object is neutrally buoyant—that is, it remains in place without either rising or ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Greek Mathematics
Greek mathematics refers to mathematics texts and ideas stemming from the Archaic through the Hellenistic and Roman periods, mostly extant from the 7th century BC to the 4th century AD, around the shores of the Eastern Mediterranean. Greek mathematicians lived in cities spread over the entire Eastern Mediterranean from Italy to North Africa but were united by Greek culture and the Greek language. The word "mathematics" itself derives from the grc, , máthēma , meaning "subject of instruction". The study of mathematics for its own sake and the use of generalized mathematical theories and proofs is an important difference between Greek mathematics and those of preceding civilizations. Origins of Greek mathematics The origin of Greek mathematics is not well documented. The earliest advanced civilizations in Greece and in Europe were the Minoan and later Mycenaean civilizations, both of which flourished during the 2nd millennium BCE. While these civilizations possessed writin ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Apollonius Of Perga
Apollonius of Perga ( grc-gre, Ἀπολλώνιος ὁ Περγαῖος, Apollṓnios ho Pergaîos; la, Apollonius Pergaeus; ) was an Ancient Greek geometer and astronomer known for his work on conic sections. Beginning from the contributions of Euclid and Archimedes on the topic, he brought them to the state prior to the invention of analytic geometry. His definitions of the terms ellipse, parabola, and hyperbola are the ones in use today. Gottfried Wilhelm Leibniz stated “He who understands Archimedes and Apollonius will admire less the achievements of the foremost men of later times.” Apollonius worked on numerous other topics, including astronomy. Most of this work has not survived, where exceptions are typically fragments referenced by other authors like Pappus of Alexandria. His hypothesis of eccentric orbits to explain the apparently aberrant motion of the planets, commonly believed until the Middle Ages, was superseded during the Renaissance. The Apol ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Eutocius Of Ascalon
Eutocius of Ascalon (; el, Εὐτόκιος ὁ Ἀσκαλωνίτης; 480s – 520s) was a Palestinian-Greek mathematician who wrote commentaries on several Archimedean treatises and on the Apollonian ''Conics''. Life and work Little is known about the life of Eutocius. He was born in Ascalon, then in Palestina Prima. He lived during the reign of Justinian. Eutocius became head the school of philosophy in Athens following Ammonius and he was succeeded in this position by Olympiodorus, possibly as early as 525. He traveled to the greatest scientific centers of his time, including Alexandria, to conduct research on Archimedes' manuscripts. He wrote commentaries on Apollonius and on Archimedes. The surviving works of Eutocius are: *A Commentary on the first four books of the ''Conics'' of Apollonius. *Commentarieson: **the ''Sphere and Cylinder'' of Archimedes. **the '' Quadrature of the Circle'' of Archimedes (''In Archimedis circuli dimensionem'' in Latin). **the ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Syracuse, Sicily
Syracuse ( ; it, Siracusa ; scn, Sarausa ), ; grc-att, Συράκουσαι, Syrákousai, ; grc-dor, Συράκοσαι, Syrā́kosai, ; grc-x-medieval, Συρακοῦσαι, Syrakoûsai, ; el, label=Modern Greek, Συρακούσες, Syrakoúses, . is a historic city on the Italian island of Sicily, the capital of the Italian province of Syracuse. The city is notable for its rich Greek and Roman history, culture, amphitheatres, architecture, and as the birthplace of the pre-eminent mathematician and engineer Archimedes. This 2,700-year-old city played a key role in ancient times, when it was one of the major powers of the Mediterranean world. Syracuse is located in the southeast corner of the island of Sicily, next to the Gulf of Syracuse beside the Ionian Sea. It is situated in a drastic rise of land with depths being close to the city offshore although the city itself is generally not so hilly in comparison. The city was founded by Ancient Greek Corinthians a ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Fluid Statics
Fluid statics or hydrostatics is the branch of fluid mechanics that studies the condition of the equilibrium of a floating body and submerged body "fluids at hydrostatic equilibrium and the pressure in a fluid, or exerted by a fluid, on an immersed body". It encompasses the study of the conditions under which fluids are at rest in stable equilibrium as opposed to fluid dynamics, the study of fluids in motion. Hydrostatics is a subcategory of fluid statics, which is the study of all fluids, both compressible or incompressible, at rest. Hydrostatics is fundamental to hydraulics, the engineering of equipment for storing, transporting and using fluids. It is also relevant to geophysics and astrophysics (for example, in understanding plate tectonics and the anomalies of the Earth's gravitational field), to meteorology, to medicine (in the context of blood pressure), and many other fields. Hydrostatics offers physical explanations for many phenomena of everyday life, such as why a ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
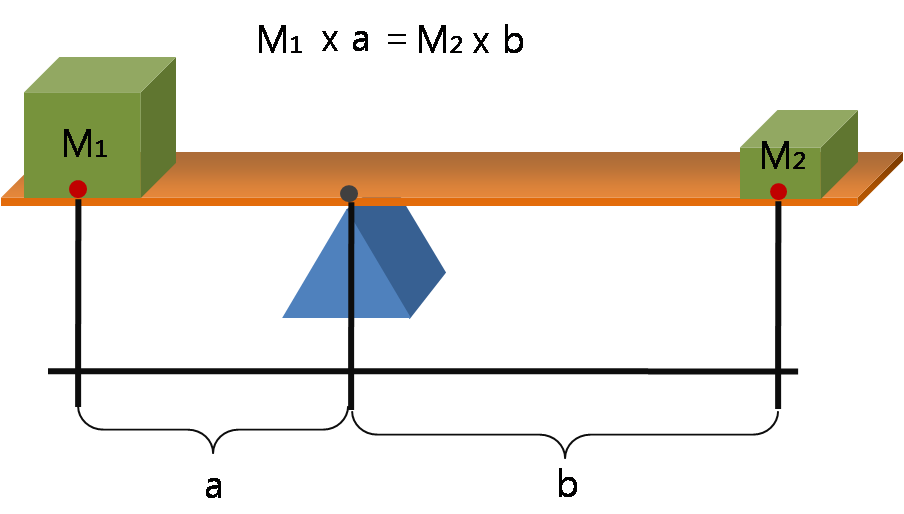
Lever
A lever is a simple machine consisting of a beam or rigid rod pivoted at a fixed hinge, or '' fulcrum''. A lever is a rigid body capable of rotating on a point on itself. On the basis of the locations of fulcrum, load and effort, the lever is divided into three types. Also, leverage is mechanical advantage gained in a system. It is one of the six simple machines identified by Renaissance scientists. A lever amplifies an input force to provide a greater output force, which is said to provide leverage. The ratio of the output force to the input force is the mechanical advantage of the lever. As such, the lever is a mechanical advantage device, trading off force against movement. Etymology The word "lever" entered English around 1300 from Old French, in which the word was ''levier''. This sprang from the stem of the verb ''lever'', meaning "to raise". The verb, in turn, goes back to the Latin ''levare'', itself from the adjective ''levis'', meaning "light" (as in "not heavy") ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Mathematics
Mathematics is an area of knowledge that includes the topics of numbers, formulas and related structures, shapes and the spaces in which they are contained, and quantities and their changes. These topics are represented in modern mathematics with the major subdisciplines of number theory, algebra, geometry, and analysis, respectively. There is no general consensus among mathematicians about a common definition for their academic discipline. Most mathematical activity involves the discovery of properties of abstract objects and the use of pure reason to prove them. These objects consist of either abstractions from nature orin modern mathematicsentities that are stipulated to have certain properties, called axioms. A ''proof'' consists of a succession of applications of deductive rules to already established results. These results include previously proved theorems, axioms, andin case of abstraction from naturesome basic properties that are considered true starting poin ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Physics
Physics is the natural science that studies matter, its fundamental constituents, its motion and behavior through space and time, and the related entities of energy and force. "Physical science is that department of knowledge which relates to the order of nature, or, in other words, to the regular succession of events." Physics is one of the most fundamental scientific disciplines, with its main goal being to understand how the universe behaves. "Physics is one of the most fundamental of the sciences. Scientists of all disciplines use the ideas of physics, including chemists who study the structure of molecules, paleontologists who try to reconstruct how dinosaurs walked, and climatologists who study how human activities affect the atmosphere and oceans. Physics is also the foundation of all engineering and technology. No engineer could design a flat-screen TV, an interplanetary spacecraft, or even a better mousetrap without first understanding the basic laws of physics ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
_by_Thomas_Degeorge.png)