Molecular energy state on:
[Wikipedia]
[Google]
[Amazon]
A
madsci.org, 17 March 1999, Dan Berger, Faculty Chemistry/Science, Bluffton College Since electrons are electrically attracted to the nucleus, an atom's electrons will generally occupy outer shells only if the more inner shells have already been completely filled by other electrons. However, this is not a strict requirement: atoms may have two or even three incomplete outer shells. (See Madelung rule for more details.) For an explanation of why electrons exist in these shells see
Corrosion Source. Retrieved on 1 December 2011. If the
 Quantized energy levels result from the wave behavior of particles, which gives a relationship between a particle's energy and its
Quantized energy levels result from the wave behavior of particles, which gives a relationship between a particle's energy and its
/ref> In polyatomic molecules, different vibrational and rotational energy levels are also involved. Roughly speaking, a molecular energy state, i.e. an

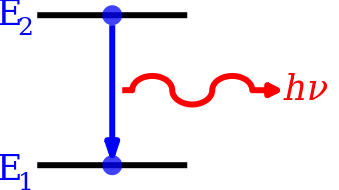 Electrons in atoms and molecules can change (make '' transitions'' in) energy levels by emitting or absorbing a
Electrons in atoms and molecules can change (make '' transitions'' in) energy levels by emitting or absorbing a
quantum mechanical
Quantum mechanics is a fundamental theory in physics that provides a description of the physical properties of nature at the scale of atoms and subatomic particles. It is the foundation of all quantum physics including quantum chemistry, qua ...
system or particle
In the physical sciences, a particle (or corpuscule in older texts) is a small localized object which can be described by several physical or chemical properties, such as volume, density, or mass.
They vary greatly in size or quantity, from ...
that is bound—that is, confined spatially—can only take on certain discrete values of energy, called energy levels. This contrasts with classical particles, which can have any amount of energy. The term is commonly used for the energy levels of the electron
The electron ( or ) is a subatomic particle with a negative one elementary electric charge. Electrons belong to the first generation of the lepton particle family,
and are generally thought to be elementary particles because they have n ...
s in atom
Every atom is composed of a nucleus and one or more electrons bound to the nucleus. The nucleus is made of one or more protons and a number of neutrons. Only the most common variety of hydrogen has no neutrons.
Every solid, liquid, gas, a ...
s, ions, or molecule
A molecule is a group of two or more atoms held together by attractive forces known as chemical bonds; depending on context, the term may or may not include ions which satisfy this criterion. In quantum physics, organic chemistry, and b ...
s, which are bound by the electric field of the nucleus
Nucleus ( : nuclei) is a Latin word for the seed inside a fruit. It most often refers to:
* Atomic nucleus, the very dense central region of an atom
*Cell nucleus, a central organelle of a eukaryotic cell, containing most of the cell's DNA
Nucl ...
, but can also refer to energy levels of nuclei or vibrational or rotational energy levels in molecules. The energy spectrum of a system with such discrete energy levels is said to be quantized.
In chemistry
Chemistry is the scientific study of the properties and behavior of matter. It is a natural science that covers the elements that make up matter to the compounds made of atoms, molecules and ions: their composition, structure, proper ...
and atomic physics
Atomic physics is the field of physics that studies atoms as an isolated system of electrons and an atomic nucleus. Atomic physics typically refers to the study of atomic structure and the interaction between atoms. It is primarily concerned wit ...
, an electron shell, or principal energy level, may be thought of as the orbit
In celestial mechanics, an orbit is the curved trajectory of an object such as the trajectory of a planet around a star, or of a natural satellite around a planet, or of an artificial satellite around an object or position in space such as ...
of one or more electrons
The electron ( or ) is a subatomic particle with a negative one elementary electric charge. Electrons belong to the first generation of the lepton particle family,
and are generally thought to be elementary particles because they have n ...
around an atom
Every atom is composed of a nucleus and one or more electrons bound to the nucleus. The nucleus is made of one or more protons and a number of neutrons. Only the most common variety of hydrogen has no neutrons.
Every solid, liquid, gas, a ...
's nucleus
Nucleus ( : nuclei) is a Latin word for the seed inside a fruit. It most often refers to:
* Atomic nucleus, the very dense central region of an atom
*Cell nucleus, a central organelle of a eukaryotic cell, containing most of the cell's DNA
Nucl ...
. The closest shell to the nucleus is called the " shell" (also called "K shell"), followed by the " shell" (or "L shell"), then the " shell" (or "M shell"), and so on farther and farther from the nucleus. The shells correspond with the principal quantum number
In quantum mechanics, the principal quantum number (symbolized ''n'') is one of four quantum numbers assigned to each electron in an atom to describe that electron's state. Its values are natural numbers (from 1) making it a discrete variable.
A ...
s (''n'' = 1, 2, 3, 4 ...) or are labeled alphabetically with letters used in the X-ray notation X-ray notation is a method of labeling atomic orbitals that grew out of X-ray science. Also known as IUPAC notation, it was adopted by the International Union of Pure and Applied Chemistry in 1991 as a simplification of the older Siegbahn notation. ...
(K, L, M, N...).
Each shell can contain only a fixed number of electrons: The first shell can hold up to two electrons, the second shell can hold up to eight (2 + 6) electrons, the third shell can hold up to 18 (2 + 6 + 10) and so on. The general formula is that the ''n''th shell can in principle hold up to 2 ''n''2 electrons.Re: Why do electron shells have set limits ?madsci.org, 17 March 1999, Dan Berger, Faculty Chemistry/Science, Bluffton College Since electrons are electrically attracted to the nucleus, an atom's electrons will generally occupy outer shells only if the more inner shells have already been completely filled by other electrons. However, this is not a strict requirement: atoms may have two or even three incomplete outer shells. (See Madelung rule for more details.) For an explanation of why electrons exist in these shells see
electron configuration
In atomic physics and quantum chemistry, the electron configuration is the distribution of electrons of an atom or molecule (or other physical structure) in atomic or molecular orbitals. For example, the electron configuration of the neon ato ...
.Electron SubshellsCorrosion Source. Retrieved on 1 December 2011. If the
potential energy
In physics, potential energy is the energy held by an object because of its position relative to other objects, stresses within itself, its electric charge, or other factors.
Common types of potential energy include the gravitational potenti ...
is set to zero at infinite
Infinite may refer to:
Mathematics
* Infinite set, a set that is not a finite set
*Infinity, an abstract concept describing something without any limit
Music
*Infinite (group), a South Korean boy band
*''Infinite'' (EP), debut EP of American m ...
distance from the atomic nucleus or molecule, the usual convention, then bound electron states have negative potential energy.
If an atom, ion, or molecule is at the lowest possible energy level, it and its electrons are said to be in the ''ground state
The ground state of a quantum-mechanical system is its stationary state of lowest energy; the energy of the ground state is known as the zero-point energy of the system. An excited state is any state with energy greater than the ground state. ...
''. If it is at a higher energy level, it is said to be '' excited'', or any electrons that have higher energy than the ground state are ''excited''. An energy level is regarded as degenerate if there is more than one measurable quantum mechanical state
State may refer to:
Arts, entertainment, and media Literature
* ''State Magazine'', a monthly magazine published by the U.S. Department of State
* ''The State'' (newspaper), a daily newspaper in Columbia, South Carolina, United States
* ''Our S ...
associated with it.
Explanation
 Quantized energy levels result from the wave behavior of particles, which gives a relationship between a particle's energy and its
Quantized energy levels result from the wave behavior of particles, which gives a relationship between a particle's energy and its wavelength
In physics, the wavelength is the spatial period of a periodic wave—the distance over which the wave's shape repeats.
It is the distance between consecutive corresponding points of the same phase on the wave, such as two adjacent crests, tro ...
. For a confined particle such as an electron
The electron ( or ) is a subatomic particle with a negative one elementary electric charge. Electrons belong to the first generation of the lepton particle family,
and are generally thought to be elementary particles because they have n ...
in an atom
Every atom is composed of a nucleus and one or more electrons bound to the nucleus. The nucleus is made of one or more protons and a number of neutrons. Only the most common variety of hydrogen has no neutrons.
Every solid, liquid, gas, a ...
, the wave function
A wave function in quantum physics is a mathematical description of the quantum state of an isolated quantum system. The wave function is a complex-valued probability amplitude, and the probabilities for the possible results of measurements mad ...
s that have well defined energies have the form of a standing wave
In physics, a standing wave, also known as a stationary wave, is a wave that oscillates in time but whose peak amplitude profile does not move in space. The peak amplitude of the wave oscillations at any point in space is constant with respect ...
. States having well-defined energies are called stationary state
A stationary state is a quantum state with all observables independent of time. It is an eigenvector of the energy operator (instead of a quantum superposition of different energies). It is also called energy eigenvector, energy eigenstate, ener ...
s because they are the states that do not change in time. Informally, these states correspond to a whole number of wavelengths of the wavefunction
A wave function in quantum physics is a mathematical description of the quantum state of an isolated quantum system. The wave function is a complex-valued probability amplitude, and the probabilities for the possible results of measurements mad ...
along a closed path (a path that ends where it started), such as a circular orbit around an atom, where the number of wavelengths gives the type of atomic orbital
In atomic theory and quantum mechanics, an atomic orbital is a function describing the location and wave-like behavior of an electron in an atom. This function can be used to calculate the probability of finding any electron of an atom in any spe ...
(0 for s-orbitals, 1 for p-orbitals and so on). Elementary examples that show mathematically how energy levels come about are the particle in a box
In quantum mechanics, the particle in a box model (also known as the infinite potential well or the infinite square well) describes a particle free to move in a small space surrounded by impenetrable barriers. The model is mainly used as a hypo ...
and the quantum harmonic oscillator
量子調和振動子 は、 古典調和振動子 の 量子力学 類似物です。任意の滑らかな ポテンシャル は通常、安定した 平衡点 の近くで 調和ポテンシャル として近似できるため、最� ...
.
Any superposition ( linear combination) of energy states is also a quantum state, but such states change with time and do not have well-defined energies. A measurement of the energy results in the collapse
Collapse or its variants may refer to:
Concepts
* Collapse (structural)
* Collapse (topology), a mathematical concept
* Collapsing manifold
* Collapse, the action of collapsing or telescoping objects
* Collapsing user interface elements
** ...
of the wavefunction, which results in a new state that consists of just a single energy state. Measurement of the possible energy levels of an object is called spectroscopy
Spectroscopy is the field of study that measures and interprets the electromagnetic spectra that result from the interaction between electromagnetic radiation and matter as a function of the wavelength or frequency of the radiation. Matter wa ...
.
History
The first evidence of quantization in atoms was the observation ofspectral lines
A spectral line is a dark or bright line in an otherwise uniform and continuous spectrum, resulting from emission or absorption of light in a narrow frequency range, compared with the nearby frequencies. Spectral lines are often used to ident ...
in light from the sun in the early 1800s by Joseph von Fraunhofer
Joseph Ritter von Fraunhofer (; ; 6 March 1787 – 7 June 1826) was a German physicist and optical lens manufacturer. He made optical glass, an achromatic telescope, and objective lenses. He also invented the spectroscope and developed diffract ...
and William Hyde Wollaston
William Hyde Wollaston (; 6 August 1766 – 22 December 1828) was an English chemist and physicist who is famous for discovering the chemical elements palladium and rhodium. He also developed a way to process platinum ore into malleable ingo ...
. The notion of energy levels was proposed in 1913 by Danish physicist Niels Bohr
Niels Henrik David Bohr (; 7 October 1885 – 18 November 1962) was a Danish physicist who made foundational contributions to understanding atomic structure and quantum theory, for which he received the Nobel Prize in Physics in 1922. B ...
in the Bohr theory
In atomic physics, the Bohr model or Rutherford–Bohr model, presented by Niels Bohr and Ernest Rutherford in 1913, is a system consisting of a small, dense nucleus surrounded by orbiting electrons—similar to the structure of the Solar Syste ...
of the atom. The modern quantum mechanical theory giving an explanation of these energy levels in terms of the Schrödinger equation
The Schrödinger equation is a linear partial differential equation that governs the wave function of a quantum-mechanical system. It is a key result in quantum mechanics, and its discovery was a significant landmark in the development of the ...
was advanced by Erwin Schrödinger
Erwin Rudolf Josef Alexander Schrödinger (, ; ; 12 August 1887 – 4 January 1961), sometimes written as or , was a Nobel Prize-winning Austrian physicist with Irish citizenship who developed a number of fundamental results in quantum theory ...
and Werner Heisenberg
Werner Karl Heisenberg () (5 December 1901 – 1 February 1976) was a German theoretical physicist and one of the main pioneers of the theory of quantum mechanics. He published his work in 1925 in a breakthrough paper. In the subsequent series ...
in 1926.
Atoms
Intrinsic energy levels
In the formulas for energy of electrons at various levels given below in an atom, the zero point for energy is set when the electron in question has completely left the atom, i.e. when the electron'sprincipal quantum number
In quantum mechanics, the principal quantum number (symbolized ''n'') is one of four quantum numbers assigned to each electron in an atom to describe that electron's state. Its values are natural numbers (from 1) making it a discrete variable.
A ...
. When the electron is bound to the atom in any closer value of , the electron's energy is lower and is considered negative.
Orbital state energy level: atom/ion with nucleus + one electron
Assume there is one electron in a givenatomic orbital
In atomic theory and quantum mechanics, an atomic orbital is a function describing the location and wave-like behavior of an electron in an atom. This function can be used to calculate the probability of finding any electron of an atom in any spe ...
in a hydrogen-like atom (ion). The energy of its state is mainly determined by the electrostatic interaction of the (negative) electron with the (positive) nucleus. The energy levels of an electron around a nucleus are given by :
:
(typically between 1 eV and 103 eV),
where is the Rydberg constant
In spectroscopy, the Rydberg constant, symbol R_\infty for
heavy atoms or R_\text for hydrogen, named after the Swedish physicist Johannes Rydberg, is a physical constant relating to the electromagnetic spectra of an atom. The constant first aro ...
, is the atomic number
The atomic number or nuclear charge number (symbol ''Z'') of a chemical element is the charge number of an atomic nucleus. For ordinary nuclei, this is equal to the proton number (''n''p) or the number of protons found in the nucleus of every ...
, is the principal quantum number
In quantum mechanics, the principal quantum number (symbolized ''n'') is one of four quantum numbers assigned to each electron in an atom to describe that electron's state. Its values are natural numbers (from 1) making it a discrete variable.
A ...
, is Planck's constant, and is the speed of light
The speed of light in vacuum, commonly denoted , is a universal physical constant that is important in many areas of physics. The speed of light is exactly equal to ). According to the special theory of relativity, is the upper limit ...
. For hydrogen-like atoms (ions) only, the Rydberg levels depend only on the principal quantum number .
This equation is obtained from combining the Rydberg formula for any hydrogen-like element (shown below) with assuming that the principal quantum number
In quantum mechanics, the principal quantum number (symbolized ''n'') is one of four quantum numbers assigned to each electron in an atom to describe that electron's state. Its values are natural numbers (from 1) making it a discrete variable.
A ...
above = in the Rydberg formula and (principal quantum number of the energy level the electron descends from, when emitting a photon
A photon () is an elementary particle that is a quantum of the electromagnetic field, including electromagnetic radiation such as light and radio waves, and the force carrier for the electromagnetic force. Photons are massless, so they always ...
). The Rydberg formula
In atomic physics, the Rydberg formula calculates the wavelengths of a spectral line in many chemical elements. The formula was primarily presented as a generalization of the Balmer series for all atomic electron transitions of hydrogen. It wa ...
was derived from empirical spectroscopic emission data.
:
An equivalent formula can be derived quantum mechanically from the time-independent Schrödinger equation
The Schrödinger equation is a linear partial differential equation that governs the wave function of a quantum-mechanical system. It is a key result in quantum mechanics, and its discovery was a significant landmark in the development of the ...
with a kinetic energy Hamiltonian operator
Hamiltonian may refer to:
* Hamiltonian mechanics, a function that represents the total energy of a system
* Hamiltonian (quantum mechanics), an operator corresponding to the total energy of that system
** Dyall Hamiltonian, a modified Hamiltonian ...
using a wave function
A wave function in quantum physics is a mathematical description of the quantum state of an isolated quantum system. The wave function is a complex-valued probability amplitude, and the probabilities for the possible results of measurements mad ...
as an eigenfunction
In mathematics, an eigenfunction of a linear operator ''D'' defined on some function space is any non-zero function f in that space that, when acted upon by ''D'', is only multiplied by some scaling factor called an eigenvalue. As an equation, th ...
to obtain the energy levels as eigenvalues
In linear algebra, an eigenvector () or characteristic vector of a linear transformation is a nonzero vector that changes at most by a scalar factor when that linear transformation is applied to it. The corresponding eigenvalue, often denoted b ...
, but the Rydberg constant would be replaced by other fundamental physics constants.
Electron-electron interactions in atoms
If there is more than one electron around the atom, electron-electron-interactions raise the energy level. These interactions are often neglected if the spatial overlap of the electron wavefunctions is low. For multi-electron atoms, interactions between electrons cause the preceding equation to be no longer accurate as stated simply with as theatomic number
The atomic number or nuclear charge number (symbol ''Z'') of a chemical element is the charge number of an atomic nucleus. For ordinary nuclei, this is equal to the proton number (''n''p) or the number of protons found in the nucleus of every ...
. A simple (though not complete) way to understand this is as a shielding effect
In chemistry, the shielding effect sometimes referred to as atomic shielding or electron shielding describes the attraction between an electron and the nucleus in any atom with more than one electron. The shielding effect can be defined as a re ...
, where the outer electrons see an effective nucleus of reduced charge, since the inner electrons are bound tightly to the nucleus and partially cancel its charge. This leads to an approximate correction where is substituted with an effective nuclear charge In atomic physics, the effective nuclear charge is the actual amount of positive (nuclear) charge experienced by an electron in a multi-electron atom. The term "effective" is used because the shielding effect of negatively charged electrons prevent ...
symbolized as that depends strongly on the principal quantum number.
In such cases, the orbital types (determined by the azimuthal quantum number
The azimuthal quantum number is a quantum number for an atomic orbital that determines its orbital angular momentum and describes the shape of the orbital. The azimuthal quantum number is the second of a set of quantum numbers that describe t ...
) as well as their levels within the molecule affect and therefore also affect the various atomic electron energy levels. The Aufbau principle
The aufbau principle , from the German ''Aufbauprinzip'' (building-up principle), also called the aufbau rule, states that in the ground state of an atom or ion, electrons fill subshells of the lowest available energy, then they fill subshells o ...
of filling an atom with electrons for an electron configuration
In atomic physics and quantum chemistry, the electron configuration is the distribution of electrons of an atom or molecule (or other physical structure) in atomic or molecular orbitals. For example, the electron configuration of the neon ato ...
takes these differing energy levels into account. For filling an atom with electrons in the ground state
The ground state of a quantum-mechanical system is its stationary state of lowest energy; the energy of the ground state is known as the zero-point energy of the system. An excited state is any state with energy greater than the ground state. ...
, the lowest energy levels are filled first and consistent with the Pauli exclusion principle
In quantum mechanics, the Pauli exclusion principle states that two or more identical particles with half-integer spins (i.e. fermions) cannot occupy the same quantum state within a quantum system simultaneously. This principle was formulated ...
, the Aufbau principle
The aufbau principle , from the German ''Aufbauprinzip'' (building-up principle), also called the aufbau rule, states that in the ground state of an atom or ion, electrons fill subshells of the lowest available energy, then they fill subshells o ...
, and Hund's rule
Hund's rule of maximum multiplicity is a rule based on observation of atomic spectra, which is used to predict the ground state of an atom or molecule with one or more open electronic shells. The rule states that for a given electron configuration ...
.
Fine structure splitting
Fine structure
In atomic physics, the fine structure describes the splitting of the spectral lines of atoms due to electron spin and relativistic corrections to the non-relativistic Schrödinger equation. It was first measured precisely for the hydrogen atom ...
arises from relativistic kinetic energy corrections, spin–orbit coupling (an electrodynamic interaction between the electron's spin
Spin or spinning most often refers to:
* Spinning (textiles), the creation of yarn or thread by twisting fibers together, traditionally by hand spinning
* Spin, the rotation of an object around a central axis
* Spin (propaganda), an intentionally b ...
and motion and the nucleus's electric field) and the Darwin term (contact term interaction of shell electrons inside the nucleus). These affect the levels by a typical order of magnitude of 10−3 eV.
Hyperfine structure
This even finer structure is due to electron–nucleus spin–spin interaction, resulting in a typical change in the energy levels by a typical order of magnitude of 10−4 eV.Energy levels due to external fields
Zeeman effect
There is an interaction energy associated with the magnetic dipole moment, , arising from the electronic orbital angular momentum, , given by : with :. Additionally taking into account the magnetic momentum arising from the electron spin. Due to relativistic effects (Dirac equation
In particle physics, the Dirac equation is a relativistic wave equation derived by British physicist Paul Dirac in 1928. In its free form, or including electromagnetic interactions, it describes all spin- massive particles, called "Dirac part ...
), there is a magnetic momentum, , arising from the electron spin
:,
with the electron-spin g-factor (about 2), resulting in a total magnetic moment, ,
:.
The interaction energy therefore becomes
:.
Stark effect
Molecules
Chemical bond
A chemical bond is a lasting attraction between atoms or ions that enables the formation of molecules and crystals. The bond may result from the electrostatic force between oppositely charged ions as in ionic bonds, or through the sharing of ...
s between atoms in a molecule form because they make the situation more stable for the involved atoms, which generally means the sum energy level for the involved atoms in the molecule is lower than if the atoms were not so bonded. As separate atoms approach each other to covalently bond, their orbitals affect each other's energy levels to form bonding and antibonding molecular orbital
In chemistry, a molecular orbital is a mathematical function describing the location and wave-like behavior of an electron in a molecule. This function can be used to calculate chemical and physical properties such as the probability of finding ...
s. The energy level of the bonding orbitals is lower, and the energy level of the antibonding orbitals is higher. For the bond in the molecule to be stable, the covalent bonding electrons occupy the lower energy bonding orbital, which may be signified by such symbols as σ or π depending on the situation. Corresponding anti-bonding orbitals can be signified by adding an asterisk to get σ* or π* orbitals. A non-bonding orbital
A non-bonding orbital, also known as ''non-bonding molecular orbital'' (NBMO), is a molecular orbital whose occupation by electrons neither increases nor decreases the bond order between the involved atoms. Non-bonding orbitals are often designat ...
in a molecule is an orbital with electrons in outer shell
Shell may refer to:
Architecture and design
* Shell (structure), a thin structure
** Concrete shell, a thin shell of concrete, usually with no interior columns or exterior buttresses
** Thin-shell structure
Science Biology
* Seashell, a hard o ...
s which do not participate in bonding and its energy level is the same as that of the constituent atom. Such orbitals can be designated as n orbitals. The electrons in an n orbital are typically lone pair
In chemistry, a lone pair refers to a pair of valence electrons that are not shared with another atom in a covalent bondIUPAC ''Gold Book'' definition''lone (electron) pair''/ref> and is sometimes called an unshared pair or non-bonding pair. Lone ...
s.
UV-Visible Absorption Spectra/ref> In polyatomic molecules, different vibrational and rotational energy levels are also involved. Roughly speaking, a molecular energy state, i.e. an
eigenstate
In quantum physics, a quantum state is a mathematical entity that provides a probability distribution for the outcomes of each possible measurement on a system. Knowledge of the quantum state together with the rules for the system's evolution in t ...
of the molecular Hamiltonian
In atomic, molecular, and optical physics and quantum chemistry, the molecular Hamiltonian is the Hamiltonian operator representing the energy of the electrons and nuclei in a molecule. This operator and the associated Schrödinger equation p ...
, is the sum of the electronic, vibrational, rotational, nuclear, and translational components, such that:
where is an eigenvalue
In linear algebra, an eigenvector () or characteristic vector of a linear transformation is a nonzero vector that changes at most by a scalar factor when that linear transformation is applied to it. The corresponding eigenvalue, often denoted b ...
of the electronic molecular Hamiltonian
In atomic, molecular, and optical physics and quantum chemistry, the molecular Hamiltonian is the Hamiltonian operator representing the energy of the electrons and nuclei in a molecule. This operator and the associated Schrödinger equation p ...
(the value of the potential energy surface
A potential energy surface (PES) describes the energy of a system, especially a collection of atoms, in terms of certain parameters, normally the positions of the atoms. The surface might define the energy as a function of one or more coordinates; ...
) at the equilibrium geometry of the molecule.
The molecular energy levels are labelled by the molecular term symbol In molecular physics, the molecular term symbol is a shorthand expression of the group representation and angular momenta that characterize the state of a molecule, i.e. its electronic quantum state which is an eigenstate of the electronic molecu ...
s. The specific energies of these components vary with the specific energy state and the substance.
Energy level diagrams
There are various types of energy level diagrams for bonds between atoms in a molecule. ;Examples :''Molecular orbital diagram
A molecular orbital diagram, or MO diagram, is a qualitative descriptive tool explaining chemical bonding in molecules in terms of molecular orbital theory in general and the linear combination of atomic orbitals (LCAO) method in particular. A f ...
s'', ''Jablonski diagram Jabłoński (Polish pronunciation: ; feminine: Jabłońska; plural: Jabłońscy) is a Polish surname derived from the noun ''jabłoń'' (''apple tree''). It appears in various forms when transliterated from Cyrillic alphabets.
People
* Aleksan ...
s'', and '' Franck–Condon'' diagrams.
Energy level transitions

 Electrons in atoms and molecules can change (make '' transitions'' in) energy levels by emitting or absorbing a
Electrons in atoms and molecules can change (make '' transitions'' in) energy levels by emitting or absorbing a photon
A photon () is an elementary particle that is a quantum of the electromagnetic field, including electromagnetic radiation such as light and radio waves, and the force carrier for the electromagnetic force. Photons are massless, so they always ...
(of electromagnetic radiation
In physics, electromagnetic radiation (EMR) consists of waves of the electromagnetic field, electromagnetic (EM) field, which propagate through space and carry momentum and electromagnetic radiant energy. It includes radio waves, microwaves, inf ...
), whose energy must be exactly equal to the energy difference between the two levels.
Electrons can also be completely removed from a chemical species such as an atom, molecule, or ion. Complete removal of an electron from an atom can be a form of ionization
Ionization, or Ionisation is the process by which an atom or a molecule acquires a negative or positive charge by gaining or losing electrons, often in conjunction with other chemical changes. The resulting electrically charged atom or molecule i ...
, which is effectively moving the electron out to an orbital with an infinite principal quantum number
In quantum mechanics, the principal quantum number (symbolized ''n'') is one of four quantum numbers assigned to each electron in an atom to describe that electron's state. Its values are natural numbers (from 1) making it a discrete variable.
A ...
, in effect so far away so as to have practically no more effect on the remaining atom (ion). For various types of atoms, there are 1st, 2nd, 3rd, etc. ionization energies
Ionization, or Ionisation is the process by which an atom or a molecule acquires a negative or positive charge by gaining or losing electrons, often in conjunction with other chemical changes. The resulting electrically charged atom or molecule i ...
for removing the 1st, then the 2nd, then the 3rd, etc. of the highest energy electrons, respectively, from the atom originally in the ground state
The ground state of a quantum-mechanical system is its stationary state of lowest energy; the energy of the ground state is known as the zero-point energy of the system. An excited state is any state with energy greater than the ground state. ...
. Energy in corresponding opposite quantities can also be released, sometimes in the form of photon energy
Photon energy is the energy carried by a single photon. The amount of energy is directly proportional to the photon's electromagnetic frequency and thus, equivalently, is inversely proportional to the wavelength. The higher the photon's frequency, ...
, when electrons are added to positively charged ions or sometimes atoms. Molecules can also undergo transitions in their vibrational or rotational energy levels. Energy level transitions can also be nonradiative, meaning emission or absorption of a photon is not involved.
If an atom, ion, or molecule is at the lowest possible energy level, it and its electrons are said to be in the ''ground state
The ground state of a quantum-mechanical system is its stationary state of lowest energy; the energy of the ground state is known as the zero-point energy of the system. An excited state is any state with energy greater than the ground state. ...
''. If it is at a higher energy level, it is said to be '' excited'', or any electrons that have higher energy than the ground state are ''excited''. Such a species can be excited to a higher energy level by absorbing a photon whose energy is equal to the energy difference between the levels. Conversely, an excited species can go to a lower energy level by spontaneously emitting a photon equal to the energy difference. A photon's energy is equal to Planck's constant () times its frequency
Frequency is the number of occurrences of a repeating event per unit of time. It is also occasionally referred to as ''temporal frequency'' for clarity, and is distinct from ''angular frequency''. Frequency is measured in hertz (Hz) which is eq ...
() and thus is proportional to its frequency, or inversely to its wavelength
In physics, the wavelength is the spatial period of a periodic wave—the distance over which the wave's shape repeats.
It is the distance between consecutive corresponding points of the same phase on the wave, such as two adjacent crests, tro ...
().
:,
since , the speed of light, equals to
Correspondingly, many kinds of spectroscopy
Spectroscopy is the field of study that measures and interprets the electromagnetic spectra that result from the interaction between electromagnetic radiation and matter as a function of the wavelength or frequency of the radiation. Matter wa ...
are based on detecting the frequency or wavelength of the emitted or absorbed photons to provide information on the material analyzed, including information on the energy levels and electronic structure of materials obtained by analyzing the spectrum
A spectrum (plural ''spectra'' or ''spectrums'') is a condition that is not limited to a specific set of values but can vary, without gaps, across a continuum. The word was first used scientifically in optics to describe the rainbow of colors i ...
.
An asterisk is commonly used to designate an excited state. An electron transition in a molecule's bond from a ground state to an excited state may have a designation such as σ → σ*, π → π*, or n → π* meaning excitation of an electron from a σ bonding to a σ antibonding
In chemical bonding theory, an antibonding orbital is a type of molecular orbital that weakens the chemical bond between two atoms and helps to raise the energy of the molecule relative to the separated atoms. Such an orbital has one or more no ...
orbital, from a π bonding to a π antibonding orbital, or from an n non-bonding to a π antibonding orbital. Reverse electron transitions for all these types of excited molecules are also possible to return to their ground states, which can be designated as σ* → σ, π* → π, or π* → n.
A transition in an energy level of an electron in a molecule may be combined with a vibrational transition
A molecular vibration is a periodic motion of the atoms of a molecule relative to each other, such that the center of mass of the molecule remains unchanged. The typical vibrational frequencies range from less than 1013 Hz to approximately 1014 H ...
and called a vibronic transition
Vibronic spectroscopy is a branch of molecular spectroscopy concerned with vibronic transitions: the simultaneous changes in electronic and vibrational energy levels of a molecule due to the absorption or emission of a photon of the appropriate ...
. A vibrational and rotational transition
In quantum mechanics, a rotational transition is an abrupt change in angular momentum. Like all other properties of a quantum particle, angular momentum is quantized, meaning it can only equal certain discrete values, which correspond to differe ...
may be combined by rovibrational coupling. In rovibronic coupling
Rovibronic coupling denotes the simultaneous interactions between rotational, vibrational, and electronic degrees of freedom in a molecule. When a rovibronic transition occurs, the rotational, vibrational, and electronic states change simultaneou ...
, electron transitions are simultaneously combined with both vibrational and rotational transitions. Photons involved in transitions may have energy of various ranges in the electromagnetic spectrum, such as X-ray
An X-ray, or, much less commonly, X-radiation, is a penetrating form of high-energy electromagnetic radiation. Most X-rays have a wavelength ranging from 10 picometers to 10 nanometers, corresponding to frequencies in the range 30&nb ...
, ultraviolet
Ultraviolet (UV) is a form of electromagnetic radiation with wavelength from 10 nanometer, nm (with a corresponding frequency around 30 Hertz, PHz) to 400 nm (750 Hertz, THz), shorter than that of visible light, but longer than ...
, visible light
Light or visible light is electromagnetic radiation that can be perceived by the human eye. Visible light is usually defined as having wavelengths in the range of 400–700 nanometres (nm), corresponding to frequencies of 750–420 te ...
, infrared
Infrared (IR), sometimes called infrared light, is electromagnetic radiation (EMR) with wavelengths longer than those of visible light. It is therefore invisible to the human eye. IR is generally understood to encompass wavelengths from around ...
, or microwave
Microwave is a form of electromagnetic radiation with wavelengths ranging from about one meter to one millimeter corresponding to frequencies between 300 MHz and 300 GHz respectively. Different sources define different frequency ran ...
radiation, depending on the type of transition. In a very general way, energy level differences between electronic states are larger, differences between vibrational levels are intermediate, and differences between rotational levels are smaller, although there can be overlap. Translational energy levels are practically continuous and can be calculated as kinetic energy using classical mechanics
Classical mechanics is a physical theory describing the motion of macroscopic objects, from projectiles to parts of machinery, and astronomical objects, such as spacecraft, planets, stars, and galaxies. For objects governed by classical ...
.
Higher temperature
Temperature is a physical quantity that expresses quantitatively the perceptions of hotness and coldness. Temperature is measured with a thermometer.
Thermometers are calibrated in various temperature scales that historically have relied o ...
causes fluid atoms and molecules to move faster increasing their translational energy, and thermally excites molecules to higher average amplitudes of vibrational and rotational modes (excites the molecules to higher internal energy levels). This means that as temperature rises, translational, vibrational, and rotational contributions to molecular heat capacity
Heat capacity or thermal capacity is a physical property of matter, defined as the amount of heat to be supplied to an object to produce a unit change in its temperature. The SI unit of heat capacity is joule per kelvin (J/K).
Heat capacity i ...
let molecules absorb heat and hold more internal energy
The internal energy of a thermodynamic system is the total energy contained within it. It is the energy necessary to create or prepare the system in its given internal state, and includes the contributions of potential energy and internal kinet ...
. Conduction of heat typically occurs as molecules or atoms collide transferring the heat between each other. At even higher temperatures, electrons can be thermally excited to higher energy orbitals in atoms or molecules. A subsequent drop of an electron to a lower energy level can release a photon, causing a possibly colored glow.
An electron farther from the nucleus has higher potential energy than an electron closer to the nucleus, thus it becomes less bound to the nucleus, since its potential energy is negative and inversely dependent on its distance from the nucleus.
Crystalline materials
Crystalline solid
A crystal or crystalline solid is a solid material whose constituents (such as atoms, molecules, or ions) are arranged in a highly ordered microscopic structure, forming a crystal lattice that extends in all directions. In addition, macros ...
s are found to have energy band
In solid-state physics, the electronic band structure (or simply band structure) of a solid describes the range of energy levels that electrons may have within it, as well as the ranges of energy that they may not have (called ''band gaps'' or ...
s, instead of or in addition to energy levels. Electrons can take on any energy within an unfilled band. At first this appears to be an exception to the requirement for energy levels. However, as shown in band theory
In solid-state physics, the electronic band structure (or simply band structure) of a solid describes the range of energy levels that electrons may have within it, as well as the ranges of energy that they may not have (called ''band gaps'' or '' ...
, energy bands are actually made up of many discrete energy levels which are too close together to resolve. Within a band the number of levels is of the order of the number of atoms in the crystal, so although electrons are actually restricted to these energies, they appear to be able to take on a continuum of values. The important energy levels in a crystal are the top of the valence band
In solid-state physics, the valence band and conduction band are the bands closest to the Fermi level, and thus determine the electrical conductivity of the solid. In nonmetals, the valence band is the highest range of electron energies in w ...
, the bottom of the conduction band
In solid-state physics, the valence band and conduction band are the bands closest to the Fermi level, and thus determine the electrical conductivity of the solid. In nonmetals, the valence band is the highest range of electron energies in w ...
, the Fermi level
The Fermi level of a solid-state body is the thermodynamic work required to add one electron to the body. It is a thermodynamic quantity usually denoted by ''µ'' or ''E''F
for brevity. The Fermi level does not include the work required to remove ...
, the vacuum level
In physics, the vacuum level refers to the energy of a free stationary electron that is outside of any material (it is in a perfect vacuum).
It may be taken as infinitely far away from a solid, or, defined to be near a surface. Its definition and m ...
, and the energy levels of any defect states in the crystal.
See also
*Perturbation theory (quantum mechanics)
In quantum mechanics, perturbation theory is a set of approximation schemes directly related to mathematical perturbation for describing a complicated quantum system in terms of a simpler one. The idea is to start with a simple system for whic ...
*Computational chemistry
Computational chemistry is a branch of chemistry that uses computer simulation to assist in solving chemical problems. It uses methods of theoretical chemistry, incorporated into computer programs, to calculate the structures and properties of m ...
References
{{DEFAULTSORT:Energy Level Chemical properties Atomic physics Molecular physics Quantum chemistry Theoretical chemistry Computational chemistry Spectroscopy pl:Powłoka elektronowa