Uniform Polyteron on:
[Wikipedia]
[Google]
[Amazon]
In
a,b,b,a, like ,3,3,3 doubling the symmetry order. Uniform polytopes in these group with symmetric rings contain this extended symmetry.
If all mirrors of a given color are unringed (inactive) in a given uniform polytope, it will have a lower symmetry construction by removing all of the inactive mirrors. If all the nodes of a given color are ringed (active), an alternation operation can generate a new 5-polytope with chiral symmetry, shown as "empty" circled nodes", but the geometry is not generally adjustable to create uniform solutions. ;Fundamental families
;Uniform prisms
There are 5 finite categorical
;Fundamental families
;Uniform prisms
There are 5 finite categorical
figure !colspan=6 , Facet counts by location: ,3,3,3, - BGCOLOR="#e0e0f0" !4, , 3, , 2, , 1, , 0 !
,3,3BR>(10) !
,3,2BR>(40) !
,3,3BR>(80) !
,3,3BR>(32) ! Alt , - BGCOLOR="#f0e0e0" !20 , , (0,0,0,0,1)√2, ,
triacontaditeron (tac)
, , 32, , 80, , 80, , 40, , 10 , ,
{3,3,4}, , - , , - , , - , , - , ,
{3,3,3}, , , - BGCOLOR="#f0e0e0" !21 , , (0,0,0,1,1)√2, ,
rectified triacontaditeron (rat)
, , 42, , 240, , 400, , 240, , 40 , ,
{ }×{3,4}, ,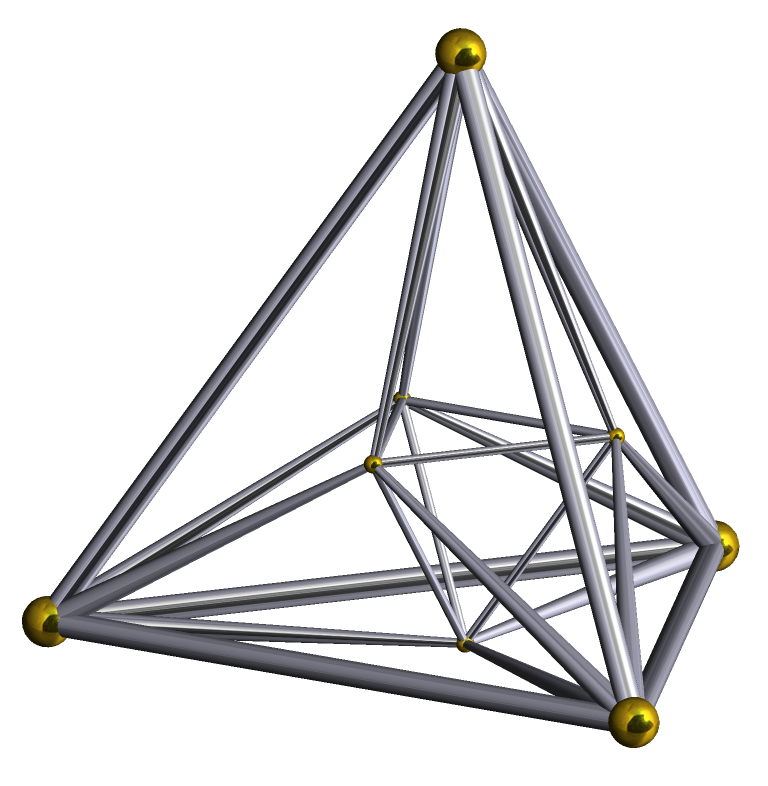
{3,3,4} , , - , , - , , - , ,
r{3,3,3} , , , - BGCOLOR="#f0e0e0" !22 , , (0,0,0,1,2)√2, ,
truncated triacontaditeron (tot)
, , 42, , 240, , 400, , 280, , 80 , ,
(Octah.pyr), ,
{3,3,4} , , - , , - , , - , ,
t{3,3,3}, , , - BGCOLOR="#e0f0e0" !23 , , (0,0,1,1,1)√2, ,
penteractitriacontaditeron (nit)
(Birectified 5-orthoplex)
, , 42, , 280, , 640, , 480, , 80 , ,
{4}×{3}, ,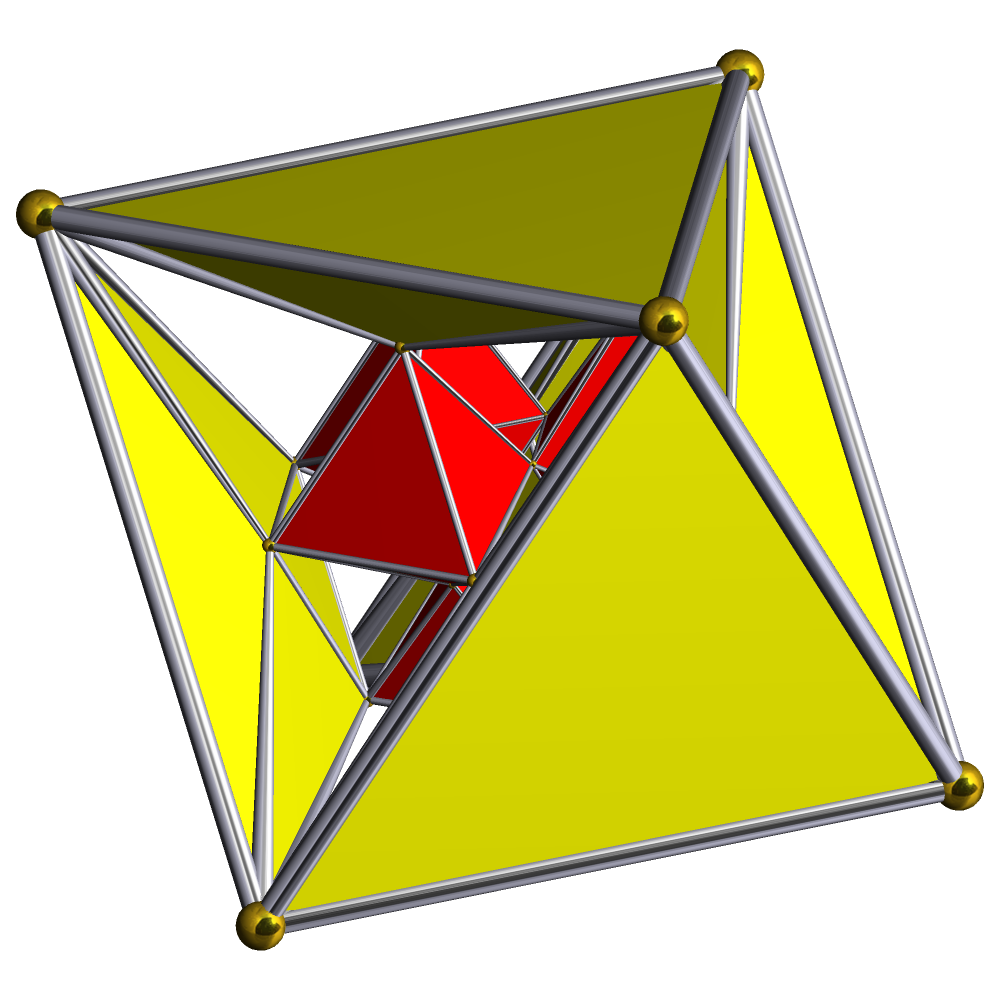
r{3,3,4} , , - , , - , , - , ,
r{3,3,3} , , , -BGCOLOR="#f0e0e0" !24 , , (0,0,1,1,2)√2, ,
small rhombated triacontaditeron (sart)
, , 82, , 640, , 1520, , 1200, , 240 , ,
Prism-wedge, ,
r{3,3,4}, ,
{ }×{3,4} , , - , , - , ,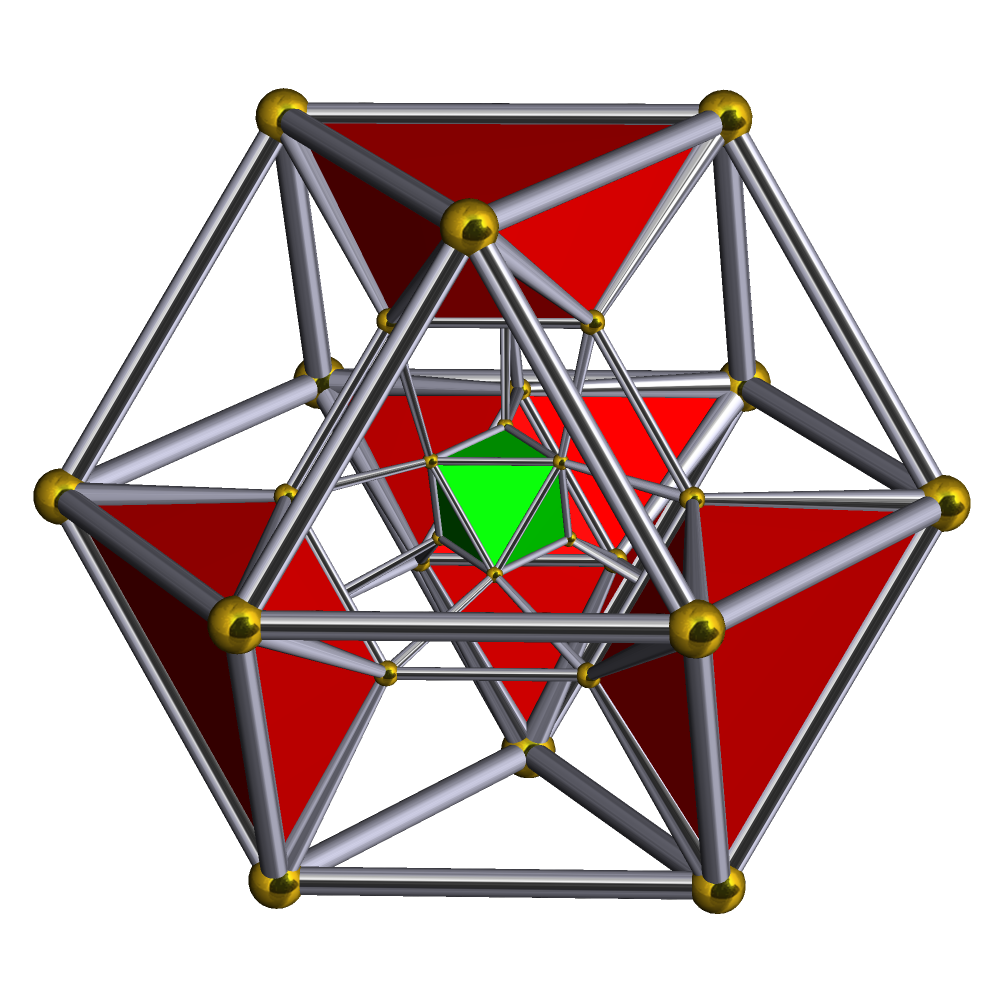
rr{3,3,3} , , , - BGCOLOR="#f0e0e0" !25 , , (0,0,1,2,2)√2, ,
bitruncated triacontaditeron (bittit)
, , 42, , 280, , 720, , 720, , 240 , , , ,
, , 
t{3,3,4} , , - , , - , , - , ,
2t{3,3,3} , , , - BGCOLOR="#f0e0e0" !26 , , (0,0,1,2,3)√2, , Cantitruncated 5-orthoplex
great rhombated triacontaditeron (gart)
, , 82, , 640, , 1520, , 1440, , 480 , , , ,
, , 
t{3,3,4}, ,
{ }×{3,4} , , -, , - , ,
t0,1,3{3,3,3} , , , - BGCOLOR="#e0e0f0" !27 , , (0,1,1,1,1)√2, , Rectified 5-cube
rectified penteract (rin)
, , 42, , 200, , 400, , 320, , 80 , ,
{3,3}×{ }, ,
r{4,3,3}, , - , , - , , - , ,
{3,3,3} , , , -BGCOLOR="#f0e0e0" !28 , , (0,1,1,1,2)√2, ,
small prismated triacontaditeron (spat)
, , 162, , 1200, , 2160, , 1440, , 320 , , , ,
, , 
r{4,3,3} , ,
{ }×r{3,4} , ,
{3}×{4}, , , ,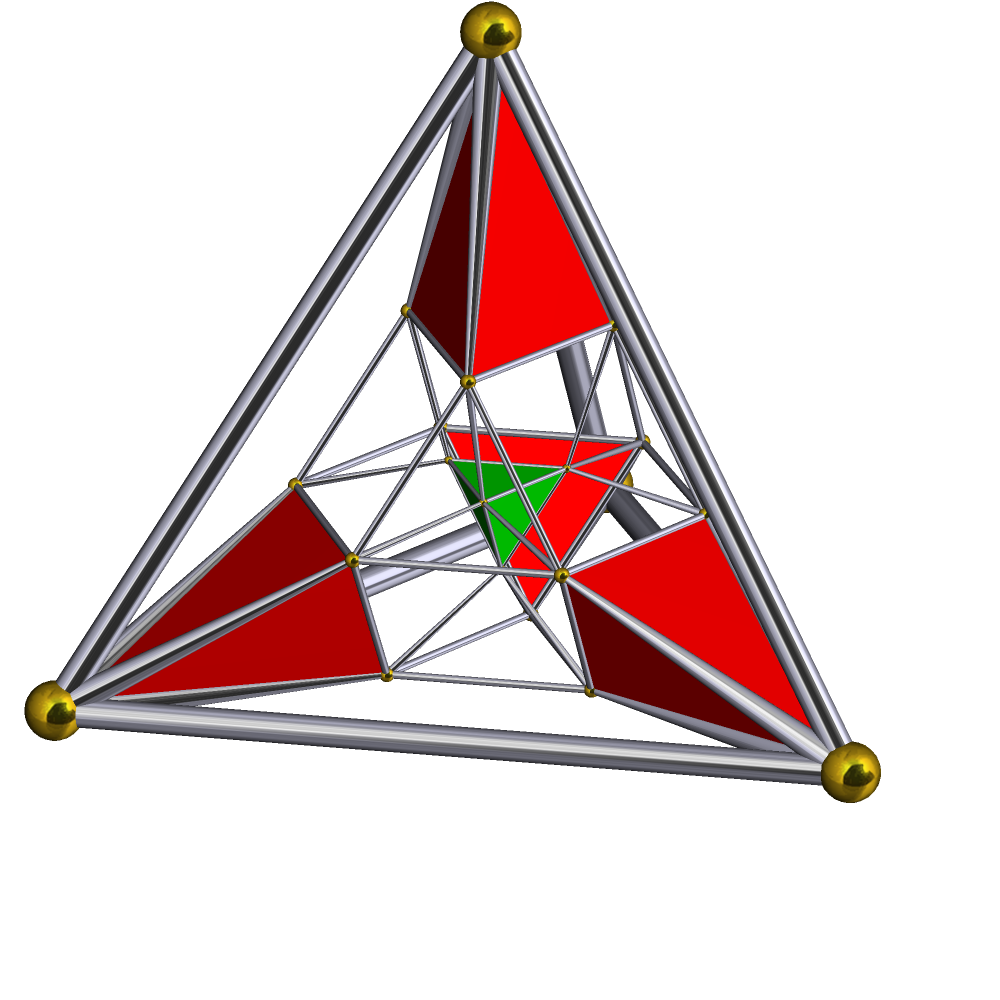
t0,3{3,3,3} , , , - BGCOLOR="#e0f0e0" !29 , , (0,1,1,2,2)√2, , Bicantellated 5-cube
small birhombated penteractitriacontaditeron (sibrant)
(Bicantellated 5-orthoplex)
, , 122, , 840, , 2160, , 1920, , 480 , , , ,
, , 
rr{3,3,4}, , - , ,
{4}×{3}, , - , ,
rr{3,3,3} , , , - BGCOLOR="#f0e0e0" !30 , , (0,1,1,2,3)√2, ,
prismatotruncated triacontaditeron (pattit)
, , 162, , 1440, , 3680, , 3360, , 960 , , , ,
, , 
rr{3,3,4} , ,
{ }×r{3,4} , ,
{6}×{4}, , - , ,
t0,1,3{3,3,3} , , , - BGCOLOR="#e0e0f0" !31 , , (0,1,2,2,2)√2, , Bitruncated 5-cube
bitruncated penteract (bittin)
, , 42, , 280, , 720, , 800, , 320 , , , ,
, , 
2t{4,3,3}, , - , , - , , - , ,
t{3,3,3} , , , - BGCOLOR="#f0e0e0" !32 , , (0,1,2,2,3)√2, , Runcicantellated 5-orthoplex
prismatorhombated triacontaditeron (pirt)
, , 162, , 1200, , 2960, , 2880, , 960 , , , ,
, , 
2t{4,3,3}, ,
{ }×t{3,4}, ,
{3}×{4} , , - , ,
t0,1,3{3,3,3} , , , - BGCOLOR="#e0f0e0" !33 , , (0,1,2,3,3)√2, , Bicantitruncated 5-cube
great birhombated triacontaditeron (gibrant)
(Bicantitruncated 5-orthoplex)
, , 122, , 840, , 2160, , 2400, , 960 , , , ,
, , 
tr{3,3,4}, , - , ,
{4}×{3}, , - , ,
rr{3,3,3} , , , - BGCOLOR="#f0e0e0" !34 , , (0,1,2,3,4)√2, , Runcicantitruncated 5-orthoplex
great prismated triacontaditeron (gippit)
, , 162, , 1440, , 4160, , 4800, , 1920 , , , ,
, , 
tr{3,3,4} , ,
{ }×t{3,4} , ,
{6}×{4}, , - , ,
t0,1,2,3{3,3,3} , , , - BGCOLOR="#e0e0f0" !35 , , (1,1,1,1,1), ,
penteract (pent)
, , 10, , 40, , 80, , 80, , 32 , ,
{3,3,3}, ,
{4,3,3}, , - , , - , , - , , - , , , - BGCOLOR="#e0f0e0" !36 , , (1,1,1,1,1)
+ (0,0,0,0,1)√2, ,
small cellated penteractitriacontaditeron (scant)
(Stericated 5-orthoplex)
, , 242, , 800, , 1040, , 640, , 160 , ,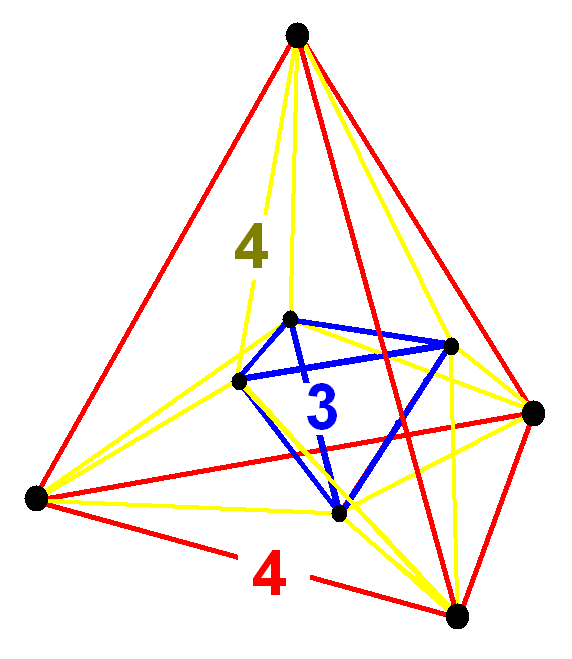
Tetr.antiprm, ,
{4,3,3}, ,
{4,3}×{ }, ,
{4}×{3}, ,
{ }×{3,3}, ,
{3,3,3} , , , - BGCOLOR="#e0e0f0" !37 , , (1,1,1,1,1)
+ (0,0,0,1,1)√2, ,
small prismated penteract (span)
, , 202, , 1240, , 2160, , 1440, , 320 , , , ,
, , 
t0,3{4,3,3}, , - , ,
{4}×{3}, ,
{ }×r{3,3}, ,
r{3,3,3} , , , - BGCOLOR="#f0e0e0" !38 , , (1,1,1,1,1)
+ (0,0,0,1,2)√2, , Steritruncated 5-orthoplex
celliprismated triacontaditeron (cappin)
, , 242, , 1520, , 2880, , 2240, , 640 , , , ,
, , 
t0,3{4,3,3} , ,
{4,3}×{ } , ,
{6}×{4} , ,
{ }×t{3,3} , ,
t{3,3,3} , , , - BGCOLOR="#e0e0f0" !39 , , (1,1,1,1,1)
+ (0,0,1,1,1)√2, ,
small rhombated penteract (sirn)
, , 122, , 680, , 1520, , 1280, , 320 , ,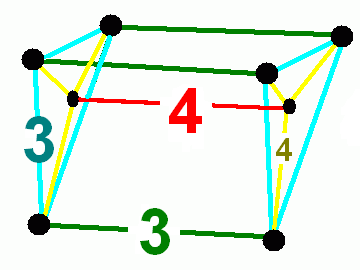
Prism-wedge, ,
rr{4,3,3}, , - , , - , ,
{ }×{3,3}, ,
r{3,3,3} , , , - BGCOLOR="#e0f0e0" !40 , , (1,1,1,1,1)
+ (0,0,1,1,2)√2, , Stericantellated 5-cube
cellirhombated penteractitriacontaditeron (carnit)
(Stericantellated 5-orthoplex)
, , 242, , 2080, , 4720, , 3840, , 960 , , , ,
, , 
rr{4,3,3}, ,
rr{4,3}×{ }, ,
{4}×{3}, ,
{ }×rr{3,3}, ,
rr{3,3,3} , , , - BGCOLOR="#e0e0f0" !41 , , (1,1,1,1,1)
+ (0,0,1,2,2)√2, , Runcicantellated 5-cube
prismatorhombated penteract (prin)
, , 202, , 1240, , 2960, , 2880, , 960 , ,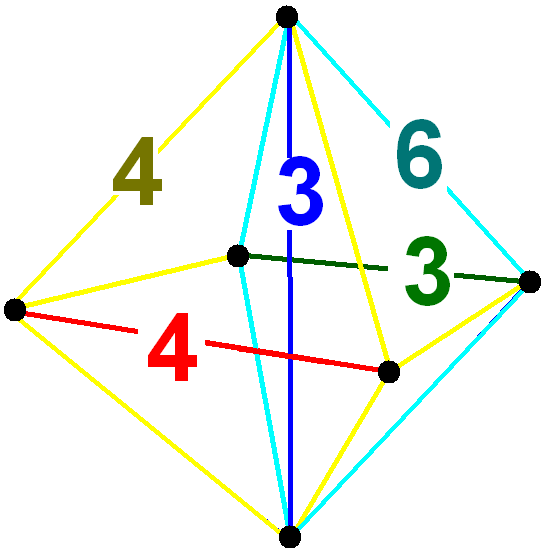 , ,
, , 
t0,2,3{4,3,3}, , - , ,
{4}×{3}, ,
{ }×t{3,3}, ,
2t{3,3,3} , , , - BGCOLOR="#f0e0e0" !42 , , (1,1,1,1,1)
+ (0,0,1,2,3)√2, , Stericantitruncated 5-orthoplex
celligreatorhombated triacontaditeron (cogart)
, , 242, , 2320, , 5920, , 5760, , 1920 , ,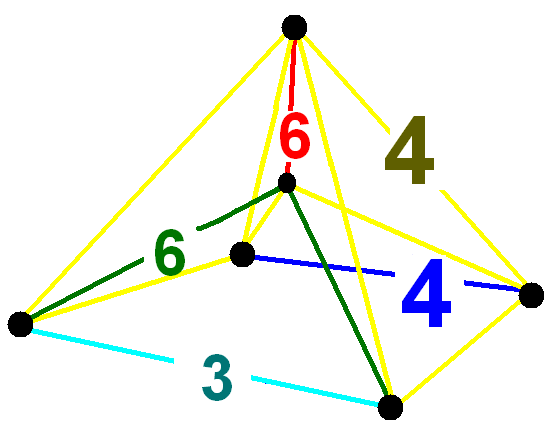 , ,
, , 
t0,2,3{4,3,3}, ,
rr{4,3}×{ }, ,
{6}×{4}, ,
{ }×tr{3,3}, ,
tr{3,3,3} , , , - BGCOLOR="#e0e0f0" !43 , , (1,1,1,1,1)
+ (0,1,1,1,1)√2, , Truncated 5-cube
truncated penteract (tan)
, , 42, , 200, , 400, , 400, , 160 , ,
Tetrah.pyr, ,
t{4,3,3}, , - , , - , , - , ,
{3,3,3} , , , - BGCOLOR="#e0e0f0" !44 , , (1,1,1,1,1)
+ (0,1,1,1,2)√2, , Steritruncated 5-cube
celliprismated triacontaditeron (capt)
, , 242, , 1600, , 2960, , 2240, , 640 , , , ,
, , 
t{4,3,3}, ,
t{4,3}×{ }, ,
{8}×{3}, ,
{ }×{3,3}, ,
t0,3{3,3,3} , , , - BGCOLOR="#e0e0f0" !45 , , (1,1,1,1,1)
+ (0,1,1,2,2)√2, , Runcitruncated 5-cube
prismatotruncated penteract (pattin)
, , 202, , 1560, , 3760, , 3360, , 960 , ,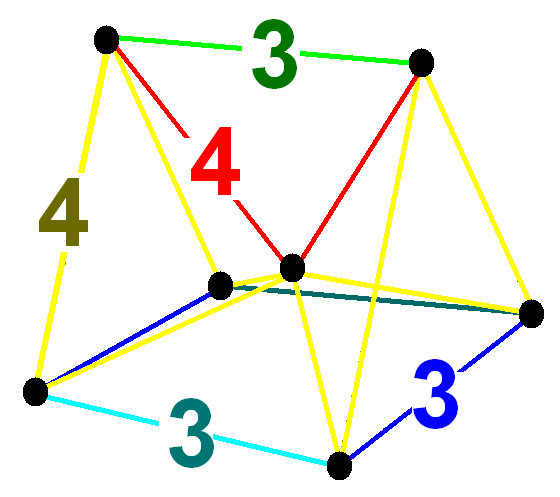 , ,
, , 
t0,1,3{4,3,3} , , - , ,
{8}×{3}, ,
{ }×r{3,3} , ,
rr{3,3,3} , , , - BGCOLOR="#e0f0e0" !46 , , (1,1,1,1,1)
+ (0,1,1,2,3)√2, , Steriruncitruncated 5-cube
celliprismatotruncated penteractitriacontaditeron (captint)
(Steriruncitruncated 5-orthoplex)
, , 242, , 2160, , 5760, , 5760, , 1920 , ,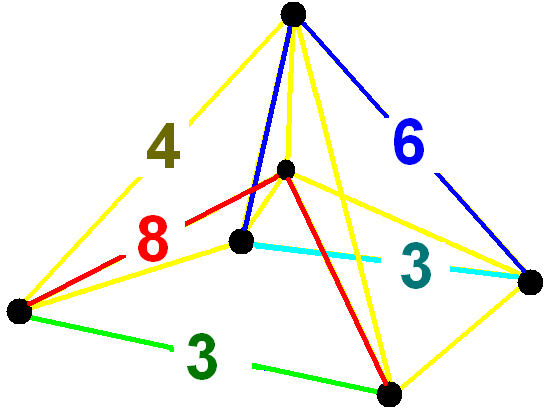 , ,
, , 
t0,1,3{4,3,3}, ,
t{4,3}×{ }, ,
{8}×{6}, ,
{ }×t{3,3}, ,
t0,1,3{3,3,3} , , , - BGCOLOR="#e0e0f0" !47 , , (1,1,1,1,1)
+ (0,1,2,2,2)√2, , Cantitruncated 5-cube
great rhombated penteract (girn)
, , 122, , 680, , 1520, , 1600, , 640 , , , ,
, , 
tr{4,3,3}, , - , , - , ,
{ }×{3,3}, ,
t{3,3,3} , , , - BGCOLOR="#e0e0f0" !48 , , (1,1,1,1,1)
+ (0,1,2,2,3)√2, , Stericantitruncated 5-cube
celligreatorhombated penteract (cogrin)
, , 242, , 2400, , 6000, , 5760, , 1920 , ,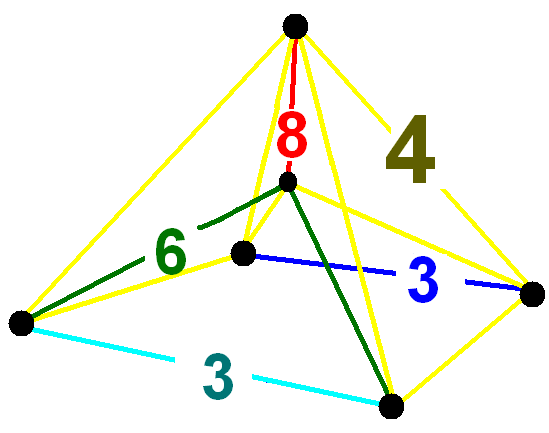 , ,
, , 
tr{4,3,3}, ,
tr{4,3}×{ }, ,
{8}×{3}, ,
{ }×rr{3,3}, ,
t0,1,3{3,3,3} , , , - BGCOLOR="#e0e0f0" !49 , , (1,1,1,1,1)
+ (0,1,2,3,3)√2, , Runcicantitruncated 5-cube
great prismated penteract (gippin)
, , 202, , 1560, , 4240, , 4800, , 1920 , , , ,
, , 
t0,1,2,3{4,3,3}, , - , ,
{8}×{3}, ,
{ }×t{3,3}, ,
tr{3,3,3} , , , - BGCOLOR="#e0f0e0" !50 , , (1,1,1,1,1)
+ (0,1,2,3,4)√2, , Omnitruncated 5-cube
great cellated penteractitriacontaditeron (gacnet)
(omnitruncated 5-orthoplex)
, , 242, , 2640, , 8160, , 9600, , 3840 , ,
Irr. {3,3,3}, ,
tr{4,3}×{ }, ,
tr{4,3}×{ }, ,
{8}×{6}, ,
{ }×tr{3,3}, ,
t0,1,2,3{3,3,3} , , , - BGCOLOR="#d0f0f0" !51 , ,
hemipenteract (hin)
= , 26 , 120 , 160 , 80 , 16 ,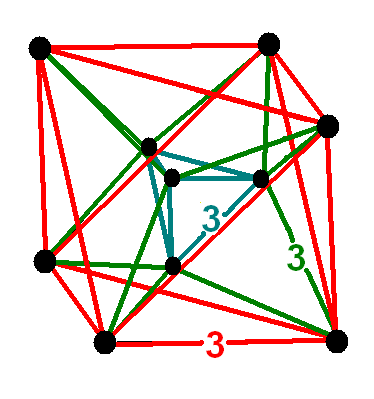
r{3,3,3} ,
h{4,3,3} , - , - , - , - , (16)

{3,3,3} , - BGCOLOR="#d0f0f0" !52 , ,
Truncated hemipenteract (thin)
= , 42 , 280 , 640 , 560 , 160 ,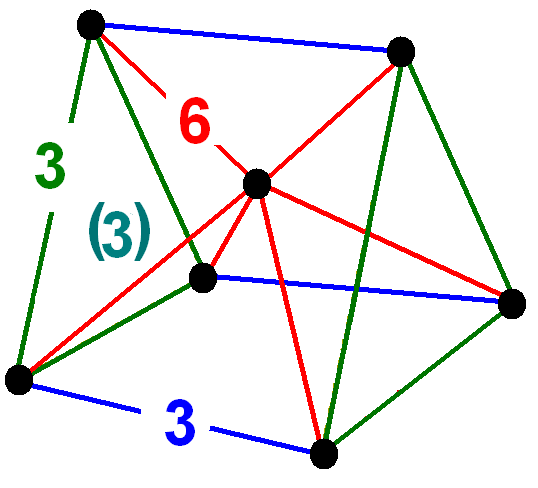 ,
, 
h2{4,3,3} , - , - , - , (16)

r{3,3,3} , (16)

t{3,3,3} , - BGCOLOR="#d0f0f0" !53 , , Runcic 5-cube
Small rhombated hemipenteract (sirhin)
= , 42 , 360 , 880 , 720 , 160 , ,
h3{4,3,3} , - , - , - , (16)

r{3,3,3} , (16)

rr{3,3,3} , - BGCOLOR="#d0f0f0" !54 , ,
Small prismated hemipenteract (siphin)
= , 82 , 480 , 720 , 400 , 80 , ,
h{4,3,3} ,
h{4,3}×{} , - , - , (16)

{3,3,3} , (16)

t0,3{3,3,3} , - BGCOLOR="#d0f0f0" !55 , , Runcicantic 5-cube
Great rhombated hemipenteract (girhin)
= , 42 , 360 , 1040 , 1200 , 480 , ,
h2,3{4,3,3} , - , - , - , (16)

2t{3,3,3} , (16)

tr{3,3,3} , - BGCOLOR="#d0f0f0" !56 , ,
Prismatotruncated hemipenteract (pithin)
= , 82 , 720 , 1840 , 1680 , 480 , ,
h2{4,3,3} ,
h2{4,3}×{} , - , - , (16)

rr{3,3,3} , (16)

t0,1,3{3,3,3} , - BGCOLOR="#d0f0f0" !57 , , Steriruncic 5-cube
Prismatorhombated hemipenteract (pirhin)
= , 82 , 560 , 1280 , 1120 , 320 , ,
h3{4,3,3} ,
h{4,3}×{} , - , - , (16)

t{3,3,3} , (16)

t0,1,3{3,3,3} , - BGCOLOR="#d0f0f0" !58 , , Steriruncicantic 5-cube
Great prismated hemipenteract (giphin)
= , 82 , 720 , 2080 , 2400 , 960 , ,
h2,3{4,3,3} ,
h2{4,3}×{} , - , - , (16)

tr{3,3,3} , (16)

t0,1,2,3{3,3,3} , - BGCOLOR="#d0f0f0" !Nonuniform , , Alternated runcicantitruncated 5-orthoplex
Snub prismatotriacontaditeron (snippit)
Snub hemipenteract (snahin)
= , 1122 , 6240 , 10880 , 6720 , 960 , ,
sr{3,3,4} , sr{2,3,4} , sr{3,2,4} , - , ht0,1,2,3{3,3,3} , (960)

Irr. {3,3,3} , - BGCOLOR="#d0f0f0" !Nonuniform , , Edge-snub 5-orthoplex
Pyritosnub penteract (pysnan)
, 1202 , 7920 , 15360 , 10560 , 1920 , , sr3{3,3,4} , sr3{2,3,4} , sr3{3,2,4} ,
s{3,3}×{ } , ht0,1,2,3{3,3,3} , (960)

Irr. {3,3}×{ } , - BGCOLOR="#d0f0f0" !Nonuniform , , Snub 5-cube
Snub penteract (snan)
, 2162 , 12240 , 21600 , 13440 , 960 , , ht0,1,2,3{3,3,4} , ht0,1,2,3{2,3,4} , ht0,1,2,3{3,2,4} , ht0,1,2,3{3,3,2} , ht0,1,2,3{3,3,3} , (1920)

Irr. {3,3,3}
Johnson and Bowers names !colspan=5, Element counts !rowspan=2, Vertex
figure !colspan=6 , Facets by location: 1,2,1 , - !4 !3 !2 !1 !0 !
,3,3BR>(16) !
1,1,1BR>(10) !
,3�
,3,3BR>(16) ! Alt , - ! 1, =
h{4,3,3,3},
Hemipenteract (hin) , 26 , 120 , 160 , 80 , 16 ,
r{3,3,3} ,
{3,3,3} ,
h{4,3,3} , - , - , - , , - ! 2, =
h2{4,3,3,3},
Truncated hemipenteract (thin) , 42 , 280 , 640 , 560 , 160 , ,
t{3,3,3} ,
h2{4,3,3} , - , - ,
r{3,3,3} , , - ! 3, =
h3{4,3,3,3}, runcic 5-cube
Small rhombated hemipenteract (sirhin) , 42 , 360 , 880 , 720 , 160 , ,
rr{3,3,3} ,
h3{4,3,3} , - , - ,
r{3,3,3} , , - ! 4, =
h4{4,3,3,3},
Small prismated hemipenteract (siphin) , 82 , 480 , 720 , 400 , 80 , ,
t0,3{3,3,3} ,
h{4,3,3} ,
h{4,3}×{} , - ,
{3,3,3} , , - ! 5, =
h2,3{4,3,3,3}, runcicantic 5-cube
Great rhombated hemipenteract (girhin) , 42 , 360 , 1040 , 1200 , 480 , ,
2t{3,3,3} ,
h2,3{4,3,3} , - , - ,
tr{3,3,3} , , - ! 6, =
h2,4{4,3,3,3},
Prismatotruncated hemipenteract (pithin) , 82 , 720 , 1840 , 1680 , 480 , ,
t0,1,3{3,3,3} ,
h2{4,3,3} ,
h2{4,3}×{} , - ,
rr{3,3,3} , , - ! 7, =
h3,4{4,3,3,3}, steriruncic 5-cube
Prismatorhombated hemipenteract (pirhin) , 82 , 560 , 1280 , 1120 , 320 , ,
t0,1,3{3,3,3} ,
h3{4,3,3} ,
h{4,3}×{} , - ,
t{3,3,3} , , - ! 8, =
h2,3,4{4,3,3,3}, steriruncicantic 5-cube
Great prismated hemipenteract (giphin) , 82 , 720 , 2080 , 2400 , 960 , ,
t0,1,2,3{3,3,3} ,
h2,3{4,3,3} ,
h2{4,3}×{} , - ,
tr{3,3,3} , , - bgcolor="#D0F0F0" ! Nonuniform , =
ht0,1,2,3{3,3,3,4}, alternated runcicantitruncated 5-orthoplex
Snub hemipenteract (snahin) , 1122 , 6240 , 10880 , 6720 , 960 , , ht0,1,2,3{3,3,3} ,
sr{3,3,4} , sr{2,3,4} , sr{3,2,4} , ht0,1,2,3{3,3,3} , (960)
Irr. {3,3,3}
and Schläfli
symbols
Name !colspan=5 rowspan=1, Element counts , - ! Facets, , Cells, , Faces, , Edges, , Vertices , - , 59 , = {3,3,3}×{ }
Rectified 5-cell prism (rappip) , 12, , 50, , 90, , 70, , 20 , - , 61 , = t{3,3,3}×{ }
Truncated 5-cell prism (tippip) , 12, , 50, , 100, , 100, , 40 , - , 62 , = rr{3,3,3}×{ }
Cantellated 5-cell prism (srippip) , 22, , 120, , 250, , 210, , 60 , - BGCOLOR="#e0f0e0" , 63 , = t0,3{3,3,3}×{ }
Runcinated 5-cell prism (spiddip) , 32, , 130, , 200, , 140, , 40 , - BGCOLOR="#e0f0e0" , 64 , = 2t{3,3,3}×{ }
Bitruncated 5-cell prism (decap) , 12, , 60, , 140, , 150, , 60 , - , 65 , = tr{3,3,3}×{ }
Cantitruncated 5-cell prism (grippip) , 22, , 120, , 280, , 300, , 120 , - , 66 , = t0,1,3{3,3,3}×{ }
Runcitruncated 5-cell prism (prippip) , 32, , 180, , 390, , 360, , 120 , - BGCOLOR="#e0f0e0" , 67 , = t0,1,2,3{3,3,3}×{ }
Omnitruncated 5-cell prism (gippiddip) , 32, , 210, , 540, , 600, , 240
and Schläfli
symbols
Name !colspan=5 rowspan=1, Element counts , - ! Facets, , Cells, , Faces, , Edges, , Vertices , - BGCOLOR="#f0e0e0" , 6'', , = {4,3,3}×{ }
Tesseractic prism (pent)
(Same as
Rectified tesseractic prism (rittip) , 26, , 136, , 272, , 224, , 64 , - BGCOLOR="#f0e0e0" , 69, , = t{4,3,3}×{ }
Truncated tesseractic prism (tattip) , 26, , 136, , 304, , 320, , 128 , - BGCOLOR="#f0e0e0" , 70, , = rr{4,3,3}×{ }
Cantellated tesseractic prism (srittip) , 58, , 360, , 784, , 672, , 192 , - BGCOLOR="#e0f0e0" , 71, , = t0,3{4,3,3}×{ }
Bitruncated tesseractic prism (tahp) , 26, , 168, , 432, , 480, , 192 , - BGCOLOR="#f0e0e0" , 73, , = tr{4,3,3}×{ }
Cantitruncated tesseractic prism (grittip) , 58, , 360, , 880, , 960, , 384 , - BGCOLOR="#f0e0e0" , 74, , = t0,1,3{4,3,3}×{ }
Runcitruncated tesseractic prism (prohp) , 82, , 528, , 1216, , 1152, , 384 , - BGCOLOR="#e0f0e0" , 75, , = t0,1,2,3{4,3,3}×{ }
Omnitruncated tesseractic prism (gidpithip) , 82, , 624, , 1696, , 1920, , 768 , - BGCOLOR="#e0e0f0" , 76, , = {3,3,4}×{ }
16-cell prism (hexip) , 18, , 64, , 88, , 56, , 16 , - BGCOLOR="#e0e0f0" , 77, , = r{3,3,4}×{ }
Rectified 16-cell prism (icope)
(Same as 24-cell prism) , 26, , 144, , 288, , 216, , 48 , - BGCOLOR="#e0e0f0" , 78, , = t{3,3,4}×{ }
Truncated 16-cell prism (thexip) , 26, , 144, , 312, , 288, , 96 , - BGCOLOR="#e0e0f0" , 79, , = rr{3,3,4}×{ }
Cantellated 16-cell prism (ricope)
(Same as rectified 24-cell prism) , 50, , 336, , 768, , 672, , 192 , - BGCOLOR="#e0e0f0" , 80, , = tr{3,3,4}×{ }
Cantitruncated 16-cell prism (ticope)
(Same as truncated 24-cell prism) , 50, , 336, , 864, , 960, , 384 , - BGCOLOR="#e0e0f0" , 81, , = t0,1,3{3,3,4}×{ }
Runcitruncated 16-cell prism (prittip) , 82, , 528, , 1216, , 1152, , 384 , - BGCOLOR="#a0e0f0" , 82, , = sr{3,3,4}×{ }
snub 24-cell prism (sadip) , 146, , 768, , 1392, , 960, , 192 , - BGCOLOR="#a0e0f0" , Nonuniform, ,
rectified tesseractic alterprism (rita) , 50, , 288, , 464, , 288, , 64 , - BGCOLOR="#a0e0f0" , Nonuniform, ,
truncated 16-cell alterprism (thexa) , 26, , 168, , 384, , 336, , 96 , - BGCOLOR="#a0e0f0" , Nonuniform, ,
bitruncated tesseractic alterprism (taha) , 50, , 288, , 624, , 576, , 192
and Schläfli
symbols
Name !colspan=5 rowspan=1, Element counts , - ! Facets, , Cells, , Faces, , Edges, , Vertices , - , 7, = {3,4,3}×{ }
rectified 24-cell prism (ricope) , 50, , 336, , 768, , 672, , 192 , - , 0, = t{3,4,3}×{ }
cantellated 24-cell prism (sricope) , 146, , 1008, , 2304, , 2016, , 576 , - BGCOLOR="#b0f0b0" , 84, , = t0,3{3,4,3}×{ }
runcinated 24-cell prism (spiccup) , 242, , 1152, , 1920, , 1296, , 288 , - BGCOLOR="#b0f0b0" , 85, , = 2t{3,4,3}×{ }
bitruncated 24-cell prism (contip) , 50, , 432, , 1248, , 1440, , 576 , - , 86, , = tr{3,4,3}×{ }
cantitruncated 24-cell prism (gricope) , 146, , 1008, , 2592, , 2880, , 1152 , - , 87, , = t0,1,3{3,4,3}×{ }
runcitruncated 24-cell prism (pricope) , 242, , 1584, , 3648, , 3456, , 1152 , - BGCOLOR="#b0f0b0" , 88, , = t0,1,2,3{3,4,3}×{ }
omnitruncated 24-cell prism (gippiccup) , 242, , 1872, , 5088, , 5760, , 2304 , - BGCOLOR="#b0e0f0" , 2, = s{3,4,3}×{ }
snub 24-cell prism (sadip) , 146, , 768, , 1392, , 960, , 192
and Schläfli
symbols
Name !colspan=5 rowspan=1, Element counts , - ! Facets, , Cells, , Faces, , Edges, , Vertices , - BGCOLOR="#f0e0e0" , 89, , = {5,3,3}×{ }
120-cell prism (hipe) , 122, , 960, , 2640, , 3000, , 1200 , - BGCOLOR="#f0e0e0" , 90, , = r{5,3,3}×{ }
Rectified 120-cell prism (rahipe) , 722, , 4560, , 9840, , 8400, , 2400 , - BGCOLOR="#f0e0e0" , 91, , = t{5,3,3}×{ }
Truncated 120-cell prism (thipe) , 722, , 4560, , 11040, , 12000, , 4800 , - BGCOLOR="#f0e0e0" , 92, , = rr{5,3,3}×{ }
Cantellated 120-cell prism (srahip) , 1922, , 12960, , 29040, , 25200, , 7200 , - BGCOLOR="#e0f0e0" , 93, , = t0,3{5,3,3}×{ }
Runcinated 120-cell prism (sidpixhip) , 2642, , 12720, , 22080, , 16800, , 4800 , - BGCOLOR="#e0f0e0" , 94, , = 2t{5,3,3}×{ }
Bitruncated 120-cell prism (xhip) , 722, , 5760, , 15840, , 18000, , 7200 , - BGCOLOR="#f0e0e0" , 95, , = tr{5,3,3}×{ }
Cantitruncated 120-cell prism (grahip) , 1922, , 12960, , 32640, , 36000, , 14400 , - BGCOLOR="#f0e0e0" , 96, , = t0,1,3{5,3,3}×{ }
Runcitruncated 120-cell prism (prixip) , 2642, , 18720, , 44880, , 43200, , 14400 , - BGCOLOR="#e0f0e0" , 97, , = t0,1,2,3{5,3,3}×{ }
Omnitruncated 120-cell prism (gidpixhip) , 2642, , 22320, , 62880, , 72000, , 28800 , - BGCOLOR="#e0e0f0" , 98, , = {3,3,5}×{ }
600-cell prism (exip) , 602, , 2400, , 3120, , 1560, , 240 , - BGCOLOR="#e0e0f0" , 99, , = r{3,3,5}×{ }
Rectified 600-cell prism (roxip) , 722, , 5040, , 10800, , 7920, , 1440 , - BGCOLOR="#e0e0f0" , 100, , = t{3,3,5}×{ }
Truncated 600-cell prism (texip) , 722, , 5040, , 11520, , 10080, , 2880 , - BGCOLOR="#e0e0f0" , 101, , = rr{3,3,5}×{ }
Cantellated 600-cell prism (srixip) , 1442, , 11520, , 28080, , 25200, , 7200 , - BGCOLOR="#e0e0f0" , 102, , = tr{3,3,5}×{ }
Cantitruncated 600-cell prism (grixip) , 1442, , 11520, , 31680, , 36000, , 14400 , - BGCOLOR="#e0e0f0" , 103, , = t0,1,3{3,3,5}×{ }
Runcitruncated 600-cell prism (prahip) , 2642, , 18720, , 44880, , 43200, , 14400
, - align=center ! Cantellated , t0,2{p,q,r,s}, , rr{p,q,r,s} , , align=left, In addition to vertex truncation, each original edge is ''beveled'' with new rectangular faces appearing in their place.
, - align=center ! Runcinated , colspan=2, t0,3{p,q,r,s} , , align=left, Runcination reduces cells and creates new cells at the vertices and edges. , - align=center ! Stericated , t0,4{p,q,r,s}, , 2r2r{p,q,r,s} , , align=left, Sterication reduces facets and creates new facets (hypercells) at the vertices and edges in the gaps. (Same as
= ,3,3,5
= ,3,3,5 There are 5 regular compact convex hyperbolic honeycombs in H4 space:Coxeter, The Beauty of Geometry: Twelve Essays, Chapter 10: Regular honeycombs in hyperbolic space, Summary tables IV p213 {, class="wikitable" , + Compact regular convex hyperbolic honeycombs , - !Honeycomb name ! Schläfli
Symbol
{p,q,r,s} !
type
{p,q,r} !Cell
type
{p,q} !Face
type
{p} !Face
figure
{s} !Edge
figure
{r,s} ! Vertex
figure
{q,r,s} !
Symbol
{p,q,r,s} !
type
{p,q,r} !Cell
type
{p,q} !Face
type
{p} !Face
figure
{s} !Edge
figure
{r,s} ! Vertex
figure
{q,r,s} !
= [(3,3,4,3,4)]:
= [3 �[]]: , align= = ,/3\,3,4
= ,4,31,1
= ,32,1
= ,31,1,1 , align= = ,4,3,4
** (Paper 22) H.S.M. Coxeter, ''Regular and Semi Regular Polytopes I'', ath. Zeit. 46 (1940) 380-407, MR 2,10** (Paper 23) H.S.M. Coxeter, ''Regular and Semi-Regular Polytopes II'', ath. Zeit. 188 (1985) 559-591(p. 287 5D Euclidean groups, p. 298 Four-dimensionsal honeycombs) ** (Paper 24) H.S.M. Coxeter, ''Regular and Semi-Regular Polytopes III'', ath. Zeit. 200 (1988) 3-45* N.W. Johnson: ''The Theory of Uniform Polytopes and Honeycombs'', Ph.D. Dissertation, University of Toronto, 1966 * James E. Humphreys, ''Reflection Groups and Coxeter Groups'', Cambridge studies in advanced mathematics, 29 (1990) (Page 141, 6.9 List of hyperbolic Coxeter groups, figure 2
geometry
Geometry (; ) is, with arithmetic, one of the oldest branches of mathematics. It is concerned with properties of space such as the distance, shape, size, and relative position of figures. A mathematician who works in the field of geometry is c ...
, a uniform 5-polytope
In geometry, a five-dimensional polytope (or 5-polytope) is a polytope in five-dimensional space, bounded by (4-polytope) facets, pairs of which share a polyhedral cell.
Definition
A 5-polytope is a closed five-dimensional figure with vertices ...
is a five-dimensional uniform polytope
In geometry, a uniform polytope of dimension three or higher is a vertex-transitive polytope bounded by uniform facets. The uniform polytopes in two dimensions are the regular polygons (the definition is different in 2 dimensions to exclude vert ...
. By definition, a uniform 5-polytope is vertex-transitive
In geometry, a polytope (e.g. a polygon or polyhedron) or a tiling is isogonal or vertex-transitive if all its vertices are equivalent under the symmetries of the figure. This implies that each vertex is surrounded by the same kinds of face in ...
and constructed from uniform 4-polytope
In geometry, a uniform 4-polytope (or uniform polychoron) is a 4-dimensional polytope which is vertex-transitive and whose cells are uniform polyhedra, and faces are regular polygons.
There are 47 non-prismatic convex uniform 4-polytopes. There ...
facets
A facet is a flat surface of a geometric shape, e.g., of a cut gemstone.
Facet may also refer to:
Arts, entertainment, and media
* ''Facets'' (album), an album by Jim Croce
* ''Facets'', a 1980 album by jazz pianist Monty Alexander and his tri ...
.
The complete set of convex uniform 5-polytopes has not been determined, but many can be made as Wythoff construction
In geometry, a Wythoff construction, named after mathematician Willem Abraham Wythoff, is a method for constructing a uniform polyhedron or plane tiling. It is often referred to as Wythoff's kaleidoscopic construction.
Construction process
...
s from a small set of symmetry groups. These construction operations are represented by the permutations of rings of the Coxeter diagram
Harold Scott MacDonald "Donald" Coxeter, (9 February 1907 – 31 March 2003) was a British and later also Canadian geometer. He is regarded as one of the greatest geometers of the 20th century.
Biography
Coxeter was born in Kensington to ...
s.
History of discovery
*Regular polytope
In mathematics, a regular polytope is a polytope whose symmetry group acts transitively on its flags, thus giving it the highest degree of symmetry. All its elements or -faces (for all , where is the dimension of the polytope) — cells, f ...
s: (convex faces)
**1852: Ludwig Schläfli
Ludwig Schläfli (15 January 1814 – 20 March 1895) was a Swiss mathematician, specialising in geometry and complex analysis (at the time called function theory) who was one of the key figures in developing the notion of higher-dimensional space ...
proved in his manuscript ''Theorie der vielfachen Kontinuität'' that there are exactly 3 regular polytopes in 5 or more dimension
In physics and mathematics, the dimension of a Space (mathematics), mathematical space (or object) is informally defined as the minimum number of coordinates needed to specify any Point (geometry), point within it. Thus, a Line (geometry), lin ...
s.
*Convex semiregular polytope
In geometry, by Thorold Gosset's definition a semiregular polytope is usually taken to be a polytope that is vertex-transitive and has all its facets being regular polytopes. E.L. Elte compiled a longer list in 1912 as ''The Semiregular Polytop ...
s: (Various definitions before Coxeter's uniform category)
**1900: Thorold Gosset
John Herbert de Paz Thorold Gosset (16 October 1869 – December 1962) was an English lawyer and an amateur mathematician. In mathematics, he is noted for discovering and classifying the semiregular polytopes in dimensions four and higher, and ...
enumerated the list of nonprismatic semiregular convex polytopes with regular facets (convex regular 4-polytope
In mathematics, a regular 4-polytope is a regular four-dimensional polytope. They are the four-dimensional analogues of the regular polyhedra in three dimensions and the regular polygons in two dimensions.
There are six convex and ten star regu ...
s) in his publication ''On the Regular and Semi-Regular Figures in Space of n Dimensions''.
*Convex uniform polytopes:
**1940-1988: The search was expanded systematically by H.S.M. Coxeter
Harold Scott MacDonald "Donald" Coxeter, (9 February 1907 – 31 March 2003) was a British and later also Canadian geometer. He is regarded as one of the greatest geometers of the 20th century.
Biography
Coxeter was born in Kensington t ...
in his publication ''Regular and Semi-Regular Polytopes I, II, and III''.
**1966: Norman W. Johnson
Norman Woodason Johnson () was a mathematician at Wheaton College, Norton, Massachusetts.
Early life and education
Norman Johnson was born on in Chicago. His father had a bookstore and published a local newspaper.
Johnson earned his unde ...
completed his Ph.D. Dissertation under Coxeter, ''The Theory of Uniform Polytopes and Honeycombs'', University of Toronto
* Non-convex uniform polytopes:
**1966: Johnson describes two non-convex uniform antiprisms in 5-space in his dissertation.
**2000-2022: Jonathan Bowers and other researchers search for other non-convex uniform 5-polytopes, with a current count of 1294 known uniform 5-polytopes outside infinite families (convex and non-convex), excluding the prisms of the uniform 4-polytopes. The list is not proven complete.
Regular 5-polytopes
Regular 5-polytopes can be represented by theSchläfli symbol
In geometry, the Schläfli symbol is a notation of the form \ that defines regular polytopes and tessellations.
The Schläfli symbol is named after the 19th-century Swiss mathematician Ludwig Schläfli, who generalized Euclidean geometry to more ...
, with s 4-polytope facets
A facet is a flat surface of a geometric shape, e.g., of a cut gemstone.
Facet may also refer to:
Arts, entertainment, and media
* ''Facets'' (album), an album by Jim Croce
* ''Facets'', a 1980 album by jazz pianist Monty Alexander and his tri ...
around each face
The face is the front of an animal's head that features the eyes, nose and mouth, and through which animals express many of their emotions. The face is crucial for human identity, and damage such as scarring or developmental deformities may aff ...
. There are exactly three such regular polytopes, all convex:
* - 5-simplex
In five-dimensional geometry, a 5-simplex is a self-dual regular 5-polytope. It has six vertices, 15 edges, 20 triangle faces, 15 tetrahedral cells, and 6 5-cell facets. It has a dihedral angle of cos−1(), or approximately 78.46°.
The 5-s ...
* - 5-cube
In five-dimensional geometry, a 5-cube is a name for a five-dimensional hypercube with 32 vertices, 80 edges, 80 square faces, 40 cubic cells, and 10 tesseract 4-faces.
It is represented by Schläfli symbol or , constructed as 3 tesseracts, ...
* - 5-orthoplex
In five-dimensional geometry, a 5-orthoplex, or 5-cross polytope, is a five-dimensional polytope with 10 vertices, 40 edges, 80 triangle faces, 80 tetrahedron cells, 32 5-cell 4-faces.
It has two constructed forms, the first being regular with ...
There are no nonconvex regular polytopes in 5 dimensions or above.
Convex uniform 5-polytopes
There are 104 known convex uniform 5-polytopes, plus a number of infinite families ofduoprism
In geometry of 4 dimensions or higher, a double prism or duoprism is a polytope resulting from the Cartesian product of two polytopes, each of two dimensions or higher. The Cartesian product of an -polytope and an -polytope is an -polytope, wher ...
prisms, and polygon-polyhedron duoprisms. All except the ''grand antiprism prism'' are based on Wythoff construction
In geometry, a Wythoff construction, named after mathematician Willem Abraham Wythoff, is a method for constructing a uniform polyhedron or plane tiling. It is often referred to as Wythoff's kaleidoscopic construction.
Construction process
...
s, reflection symmetry generated with Coxeter group
In mathematics, a Coxeter group, named after H. S. M. Coxeter, is an abstract group that admits a formal description in terms of reflections (or kaleidoscopic mirrors). Indeed, the finite Coxeter groups are precisely the finite Euclidean refl ...
s.Symmetry of uniform 5-polytopes in four dimensions
The5-simplex
In five-dimensional geometry, a 5-simplex is a self-dual regular 5-polytope. It has six vertices, 15 edges, 20 triangle faces, 15 tetrahedral cells, and 6 5-cell facets. It has a dihedral angle of cos−1(), or approximately 78.46°.
The 5-s ...
is the regular form in the A5 family. The 5-cube
In five-dimensional geometry, a 5-cube is a name for a five-dimensional hypercube with 32 vertices, 80 edges, 80 square faces, 40 cubic cells, and 10 tesseract 4-faces.
It is represented by Schläfli symbol or , constructed as 3 tesseracts, ...
and 5-orthoplex
In five-dimensional geometry, a 5-orthoplex, or 5-cross polytope, is a five-dimensional polytope with 10 vertices, 40 edges, 80 triangle faces, 80 tetrahedron cells, 32 5-cell 4-faces.
It has two constructed forms, the first being regular with ...
are the regular forms in the B5 family. The bifurcating graph of the D5 family contains the 5-orthoplex
In five-dimensional geometry, a 5-orthoplex, or 5-cross polytope, is a five-dimensional polytope with 10 vertices, 40 edges, 80 triangle faces, 80 tetrahedron cells, 32 5-cell 4-faces.
It has two constructed forms, the first being regular with ...
, as well as a 5-demicube
In five-dimensional geometry, a demipenteract or 5-demicube is a semiregular 5-polytope, constructed from a ''5-hypercube'' (penteract) with alternated vertices removed.
It was discovered by Thorold Gosset. Since it was the only semiregular 5- ...
which is an alternated 5-cube
In five-dimensional geometry, a 5-cube is a name for a five-dimensional hypercube with 32 vertices, 80 edges, 80 square faces, 40 cubic cells, and 10 tesseract 4-faces.
It is represented by Schläfli symbol or , constructed as 3 tesseracts, ...
.
Each reflective uniform 5-polytope can be constructed in one or more reflective point group in 5 dimensions by a Wythoff construction
In geometry, a Wythoff construction, named after mathematician Willem Abraham Wythoff, is a method for constructing a uniform polyhedron or plane tiling. It is often referred to as Wythoff's kaleidoscopic construction.
Construction process
...
, represented by rings around permutations of nodes in a Coxeter diagram
Harold Scott MacDonald "Donald" Coxeter, (9 February 1907 – 31 March 2003) was a British and later also Canadian geometer. He is regarded as one of the greatest geometers of the 20th century.
Biography
Coxeter was born in Kensington to ...
. Mirror hyperplane
In geometry, a hyperplane is a subspace whose dimension is one less than that of its ''ambient space''. For example, if a space is 3-dimensional then its hyperplanes are the 2-dimensional planes, while if the space is 2-dimensional, its hyper ...
s can be grouped, as seen by colored nodes, separated by even-branches. Symmetry groups of the form ,b,b,a have an extended symmetry,  ;Fundamental families
;Uniform prisms
There are 5 finite categorical
;Fundamental families
;Uniform prisms
There are 5 finite categorical uniform
A uniform is a variety of clothing worn by members of an organization while participating in that organization's activity. Modern uniforms are most often worn by armed forces and paramilitary organizations such as police, emergency services, se ...
prism
Prism usually refers to:
* Prism (optics), a transparent optical component with flat surfaces that refract light
* Prism (geometry), a kind of polyhedron
Prism may also refer to:
Science and mathematics
* Prism (geology), a type of sedimentary ...
atic families of polytopes based on the nonprismatic uniform 4-polytope
In geometry, a uniform 4-polytope (or uniform polychoron) is a 4-dimensional polytope which is vertex-transitive and whose cells are uniform polyhedra, and faces are regular polygons.
There are 47 non-prismatic convex uniform 4-polytopes. There ...
s. There is one infinite family of 5-polytopes based on prisms of the uniform duoprism
In geometry of 4 dimensions or higher, a double prism or duoprism is a polytope resulting from the Cartesian product of two polytopes, each of two dimensions or higher. The Cartesian product of an -polytope and an -polytope is an -polytope, wher ...
s ××.
;Uniform duoprisms
There are 3 categorical uniform
A uniform is a variety of clothing worn by members of an organization while participating in that organization's activity. Modern uniforms are most often worn by armed forces and paramilitary organizations such as police, emergency services, se ...
duoprism
In geometry of 4 dimensions or higher, a double prism or duoprism is a polytope resulting from the Cartesian product of two polytopes, each of two dimensions or higher. The Cartesian product of an -polytope and an -polytope is an -polytope, wher ...
atic families of polytopes based on Cartesian product
In mathematics, specifically set theory, the Cartesian product of two sets ''A'' and ''B'', denoted ''A''×''B'', is the set of all ordered pairs where ''a'' is in ''A'' and ''b'' is in ''B''. In terms of set-builder notation, that is
: A\ti ...
s of the uniform polyhedra
In geometry, a uniform polyhedron has regular polygons as faces and is vertex-transitive (i.e., there is an isometry mapping any vertex onto any other). It follows that all vertices are congruent.
Uniform polyhedra may be regular (if also fa ...
and regular polygon
In Euclidean geometry, a regular polygon is a polygon that is Equiangular polygon, direct equiangular (all angles are equal in measure) and Equilateral polygon, equilateral (all sides have the same length). Regular polygons may be either convex p ...
s: ×.
Enumerating the convex uniform 5-polytopes
*Simplex
In geometry, a simplex (plural: simplexes or simplices) is a generalization of the notion of a triangle or tetrahedron to arbitrary dimensions. The simplex is so-named because it represents the simplest possible polytope in any given dimension. ...
family: A5 4** 19 uniform 5-polytopes
* Hypercube
In geometry, a hypercube is an ''n''-dimensional analogue of a square () and a cube (). It is a closed, compact, convex figure whose 1- skeleton consists of groups of opposite parallel line segments aligned in each of the space's dimensions, ...
/Orthoplex
In geometry, a cross-polytope, hyperoctahedron, orthoplex, or cocube is a regular, convex polytope that exists in ''n''- dimensional Euclidean space. A 2-dimensional cross-polytope is a square, a 3-dimensional cross-polytope is a regular octahed ...
family: B5 ,33** 31 uniform 5-polytopes
* Demihypercube
In geometry, demihypercubes (also called ''n-demicubes'', ''n-hemicubes'', and ''half measure polytopes'') are a class of ''n''-polytopes constructed from alternation of an ''n''-hypercube, labeled as ''hγn'' for being ''half'' of the hype ...
D5/E5 family: 2,1,1** 23 uniform 5-polytopes (8 unique)
* Polychoral prisms:
** 56 uniform 5-polytope (45 unique) constructions based on prismatic families: ,3,3�nbsp;
In word processing and digital typesetting, a non-breaking space, , also called NBSP, required space, hard space, or fixed space (though it is not of fixed width), is a space character that prevents an automatic line break at its position. In ...
,3,3�nbsp;
In word processing and digital typesetting, a non-breaking space, , also called NBSP, required space, hard space, or fixed space (though it is not of fixed width), is a space character that prevents an automatic line break at its position. In ...
,3,3�nbsp;
In word processing and digital typesetting, a non-breaking space, , also called NBSP, required space, hard space, or fixed space (though it is not of fixed width), is a space character that prevents an automatic line break at its position. In ...
1,1,1�nbsp;
In word processing and digital typesetting, a non-breaking space, , also called NBSP, required space, hard space, or fixed space (though it is not of fixed width), is a space character that prevents an automatic line break at its position. In ...
** One non-Wythoffian
In geometry, a Wythoff construction, named after mathematician Willem Abraham Wythoff, is a method for constructing a uniform polyhedron or plane tiling. It is often referred to as Wythoff's kaleidoscopic construction.
Construction process
...
- The grand antiprism prism is the only known non-Wythoffian convex uniform 5-polytope, constructed from two grand antiprism
In geometry, the grand antiprism or pentagonal double antiprismoid is a uniform 4-polytope (4-dimensional uniform polytope) bounded by 320 cells: 20 pentagonal antiprisms, and 300 tetrahedra. It is an anomalous, non-Wythoffian uniform 4-polytope ...
s connected by polyhedral prisms.
That brings the tally to: 19+31+8+45+1=104
In addition there are:
* Infinitely many uniform 5-polytope constructions based on duoprism prismatic families: 'p''� 'q''�nbsp;
In word processing and digital typesetting, a non-breaking space, , also called NBSP, required space, hard space, or fixed space (though it is not of fixed width), is a space character that prevents an automatic line break at its position. In ...
* Infinitely many uniform 5-polytope constructions based on duoprismatic families: ,3� 'p'' ,3� 'p'' ,3� 'p''
The A5 family
There are 19 forms based on all permutations of theCoxeter diagram
Harold Scott MacDonald "Donald" Coxeter, (9 February 1907 – 31 March 2003) was a British and later also Canadian geometer. He is regarded as one of the greatest geometers of the 20th century.
Biography
Coxeter was born in Kensington to ...
s with one or more rings. (16+4-1 cases)
They are named by Norman Johnson from the Wythoff construction operations upon regular 5-simplex (hexateron).
The A5 family has symmetry of order 720 (6 factorial
In mathematics, the factorial of a non-negative denoted is the product of all positive integers less than or equal The factorial also equals the product of n with the next smaller factorial:
\begin
n! &= n \times (n-1) \times (n-2) \t ...
). 7 of the 19 figures, with symmetrically ringed Coxeter diagrams have doubled symmetry, order 1440.
The coordinates of uniform 5-polytopes with 5-simplex symmetry can be generated as permutations of simple integers in 6-space, all in hyperplanes with normal vector (1,1,1,1,1,1).
The B5 family
The B5 family has symmetry of order 3840 (5!×25). This family has 25−1=31 Wythoffian uniform polytopes generated by marking one or more nodes of theCoxeter diagram
Harold Scott MacDonald "Donald" Coxeter, (9 February 1907 – 31 March 2003) was a British and later also Canadian geometer. He is regarded as one of the greatest geometers of the 20th century.
Biography
Coxeter was born in Kensington to ...
. Also added are 8 uniform polytopes generated as alternations with half the symmetry, which form a complete duplicate of the D5 family as ... = ..... (There are more alternations that are not listed because they produce only repetitions, as ... = .... and ... = .... These would give a complete duplication of the uniform 5-polytopes numbered 20 through 34 with symmetry broken in half.)
For simplicity it is divided into two subgroups, each with 12 forms, and 7 "middle" forms which equally belong in both.
The 5-cube family of 5-polytopes are given by the convex hulls of the base points listed in the following table, with all permutations of coordinates and sign taken. Each base point generates a distinct uniform 5-polytope. All coordinates correspond with uniform 5-polytopes of edge length 2.
{, class="wikitable"
!rowspan=2, #
!rowspan=2, Base point
!rowspan=2, NameCoxeter diagram
Harold Scott MacDonald "Donald" Coxeter, (9 February 1907 – 31 March 2003) was a British and later also Canadian geometer. He is regarded as one of the greatest geometers of the 20th century.
Biography
Coxeter was born in Kensington to ...
!colspan=5, Element counts
!rowspan=2, Vertexfigure !colspan=6 , Facet counts by location: ,3,3,3, - BGCOLOR="#e0e0f0" !4, , 3, , 2, , 1, , 0 !
,3,3BR>(10) !
,3,2BR>(40) !
,2,3
The comma is a punctuation mark that appears in several variants in different languages. It has the same shape as an apostrophe or single closing quotation mark () in many typefaces, but it differs from them in being placed on the baseline ...
BR>(80)
! ,3,3BR>(80) !
,3,3BR>(32) ! Alt , - BGCOLOR="#f0e0e0" !20 , , (0,0,0,0,1)√2, ,
5-orthoplex
In five-dimensional geometry, a 5-orthoplex, or 5-cross polytope, is a five-dimensional polytope with 10 vertices, 40 edges, 80 triangle faces, 80 tetrahedron cells, 32 5-cell 4-faces.
It has two constructed forms, the first being regular with ...
triacontaditeron (tac)
, , 32, , 80, , 80, , 40, , 10 , ,

{3,3,4}, , - , , - , , - , , - , ,

{3,3,3}, , , - BGCOLOR="#f0e0e0" !21 , , (0,0,0,1,1)√2, ,
Rectified 5-orthoplex
In five-dimensional geometry, a rectified 5-orthoplex is a convex uniform 5-polytope, being a rectification of the regular 5-orthoplex.
There are 5 degrees of rectifications for any 5-polytope, the zeroth here being the 5-orthoplex itself, and th ...
rectified triacontaditeron (rat)
, , 42, , 240, , 400, , 240, , 40 , ,

{ }×{3,4}, ,

{3,3,4} , , - , , - , , - , ,

r{3,3,3} , , , - BGCOLOR="#f0e0e0" !22 , , (0,0,0,1,2)√2, ,
Truncated 5-orthoplex
In five-dimensional geometry, a truncated 5-orthoplex is a convex uniform 5-polytope, being a truncation of the regular 5-orthoplex.
There are 4 unique truncations of the 5-orthoplex. Vertices of the truncation 5-orthoplex are located as pairs on ...
truncated triacontaditeron (tot)
, , 42, , 240, , 400, , 280, , 80 , ,

(Octah.pyr), ,

{3,3,4} , , - , , - , , - , ,

t{3,3,3}, , , - BGCOLOR="#e0f0e0" !23 , , (0,0,1,1,1)√2, ,
Birectified 5-cube
In five-dimensional geometry, a rectified 5-cube is a convex uniform 5-polytope, being a rectification of the regular 5-cube.
There are 5 degrees of rectifications of a 5-polytope, the zeroth here being the 5-cube, and the 4th and last being the ...
penteractitriacontaditeron (nit)
(Birectified 5-orthoplex)
, , 42, , 280, , 640, , 480, , 80 , ,

{4}×{3}, ,

r{3,3,4} , , - , , - , , - , ,

r{3,3,3} , , , -BGCOLOR="#f0e0e0" !24 , , (0,0,1,1,2)√2, ,
Cantellated 5-orthoplex
In five-dimensional geometry, a cantellated 5-orthoplex is a convex uniform 5-polytope, being a cantellation of the regular 5-orthoplex.
There are 6 cantellation for the 5-orthoplex, including truncations. Some of them are more easily constructed ...
small rhombated triacontaditeron (sart)
, , 82, , 640, , 1520, , 1200, , 240 , ,

Prism-wedge, ,

r{3,3,4}, ,

{ }×{3,4} , , - , , - , ,

rr{3,3,3} , , , - BGCOLOR="#f0e0e0" !25 , , (0,0,1,2,2)√2, ,
Bitruncated 5-orthoplex
In five-dimensional geometry, a truncated 5-orthoplex is a convex uniform 5-polytope, being a truncation of the regular 5-orthoplex.
There are 4 unique truncations of the 5-orthoplex. Vertices of the truncation 5-orthoplex are located as pairs o ...
bitruncated triacontaditeron (bittit)
, , 42, , 280, , 720, , 720, , 240 , ,
 , ,
, , 
t{3,3,4} , , - , , - , , - , ,

2t{3,3,3} , , , - BGCOLOR="#f0e0e0" !26 , , (0,0,1,2,3)√2, , Cantitruncated 5-orthoplex
great rhombated triacontaditeron (gart)
, , 82, , 640, , 1520, , 1440, , 480 , ,
 , ,
, , 
t{3,3,4}, ,

{ }×{3,4} , , -, , - , ,

t0,1,3{3,3,3} , , , - BGCOLOR="#e0e0f0" !27 , , (0,1,1,1,1)√2, , Rectified 5-cube
rectified penteract (rin)
, , 42, , 200, , 400, , 320, , 80 , ,

{3,3}×{ }, ,

r{4,3,3}, , - , , - , , - , ,

{3,3,3} , , , -BGCOLOR="#f0e0e0" !28 , , (0,1,1,1,2)√2, ,
Runcinated 5-orthoplex
In five-dimensional geometry, a runcinated 5-orthoplex is a convex uniform 5-polytope with 3rd order truncation (runcination) of the regular 5-orthoplex.
There are 8 runcinations of the 5-orthoplex with permutations of truncations, and cantellati ...
small prismated triacontaditeron (spat)
, , 162, , 1200, , 2160, , 1440, , 320 , ,
 , ,
, , 
r{4,3,3} , ,

{ }×r{3,4} , ,

{3}×{4}, , , ,

t0,3{3,3,3} , , , - BGCOLOR="#e0f0e0" !29 , , (0,1,1,2,2)√2, , Bicantellated 5-cube
small birhombated penteractitriacontaditeron (sibrant)
(Bicantellated 5-orthoplex)
, , 122, , 840, , 2160, , 1920, , 480 , ,
 , ,
, , 
rr{3,3,4}, , - , ,

{4}×{3}, , - , ,

rr{3,3,3} , , , - BGCOLOR="#f0e0e0" !30 , , (0,1,1,2,3)√2, ,
Runcitruncated 5-orthoplex
In Five-dimensional space, five-dimensional geometry, a runcinated 5-orthoplex is a convex uniform 5-polytope with 3rd order Truncation (geometry), truncation (runcination) of the regular 5-orthoplex.
There are 8 runcinations of the 5-orthoplex wi ...
prismatotruncated triacontaditeron (pattit)
, , 162, , 1440, , 3680, , 3360, , 960 , ,
 , ,
, , 
rr{3,3,4} , ,

{ }×r{3,4} , ,

{6}×{4}, , - , ,

t0,1,3{3,3,3} , , , - BGCOLOR="#e0e0f0" !31 , , (0,1,2,2,2)√2, , Bitruncated 5-cube
bitruncated penteract (bittin)
, , 42, , 280, , 720, , 800, , 320 , ,
 , ,
, , 
2t{4,3,3}, , - , , - , , - , ,

t{3,3,3} , , , - BGCOLOR="#f0e0e0" !32 , , (0,1,2,2,3)√2, , Runcicantellated 5-orthoplex
prismatorhombated triacontaditeron (pirt)
, , 162, , 1200, , 2960, , 2880, , 960 , ,
 , ,
, , 
2t{4,3,3}, ,

{ }×t{3,4}, ,

{3}×{4} , , - , ,

t0,1,3{3,3,3} , , , - BGCOLOR="#e0f0e0" !33 , , (0,1,2,3,3)√2, , Bicantitruncated 5-cube
great birhombated triacontaditeron (gibrant)
(Bicantitruncated 5-orthoplex)
, , 122, , 840, , 2160, , 2400, , 960 , ,
 , ,
, , 
tr{3,3,4}, , - , ,

{4}×{3}, , - , ,

rr{3,3,3} , , , - BGCOLOR="#f0e0e0" !34 , , (0,1,2,3,4)√2, , Runcicantitruncated 5-orthoplex
great prismated triacontaditeron (gippit)
, , 162, , 1440, , 4160, , 4800, , 1920 , ,
 , ,
, , 
tr{3,3,4} , ,

{ }×t{3,4} , ,

{6}×{4}, , - , ,

t0,1,2,3{3,3,3} , , , - BGCOLOR="#e0e0f0" !35 , , (1,1,1,1,1), ,
5-cube
In five-dimensional geometry, a 5-cube is a name for a five-dimensional hypercube with 32 vertices, 80 edges, 80 square faces, 40 cubic cells, and 10 tesseract 4-faces.
It is represented by Schläfli symbol or , constructed as 3 tesseracts, ...
penteract (pent)
, , 10, , 40, , 80, , 80, , 32 , ,

{3,3,3}, ,

{4,3,3}, , - , , - , , - , , - , , , - BGCOLOR="#e0f0e0" !36 , , (1,1,1,1,1)
+ (0,0,0,0,1)√2, ,
Stericated 5-cube
In five-dimensional geometry, a stericated 5-cube is a convex uniform 5-polytope with fourth-order truncations (sterication) of the regular 5-cube.
There are eight degrees of sterication for the 5-cube, including permutations of runcination, ca ...
small cellated penteractitriacontaditeron (scant)
(Stericated 5-orthoplex)
, , 242, , 800, , 1040, , 640, , 160 , ,

Tetr.antiprm, ,

{4,3,3}, ,

{4,3}×{ }, ,

{4}×{3}, ,

{ }×{3,3}, ,

{3,3,3} , , , - BGCOLOR="#e0e0f0" !37 , , (1,1,1,1,1)
+ (0,0,0,1,1)√2, ,
Runcinated 5-cube
In five-dimensional geometry, a runcinated 5-cube is a convex uniform 5-polytope that is a runcination (a 3rd order truncation) of the regular 5-cube.
There are 8 unique degrees of runcinations of the 5-cube, along with permutations of truncation ...
small prismated penteract (span)
, , 202, , 1240, , 2160, , 1440, , 320 , ,
 , ,
, , 
t0,3{4,3,3}, , - , ,

{4}×{3}, ,

{ }×r{3,3}, ,

r{3,3,3} , , , - BGCOLOR="#f0e0e0" !38 , , (1,1,1,1,1)
+ (0,0,0,1,2)√2, , Steritruncated 5-orthoplex
celliprismated triacontaditeron (cappin)
, , 242, , 1520, , 2880, , 2240, , 640 , ,
 , ,
, , 
t0,3{4,3,3} , ,

{4,3}×{ } , ,

{6}×{4} , ,

{ }×t{3,3} , ,

t{3,3,3} , , , - BGCOLOR="#e0e0f0" !39 , , (1,1,1,1,1)
+ (0,0,1,1,1)√2, ,
Cantellated 5-cube
In six-dimensional geometry, a cantellated 5-cube is a convex uniform 5-polytope, being a cantellation of the regular 5-cube.
There are 6 unique cantellation for the 5-cube, including truncations. Half of them are more easily constructed from the ...
small rhombated penteract (sirn)
, , 122, , 680, , 1520, , 1280, , 320 , ,

Prism-wedge, ,

rr{4,3,3}, , - , , - , ,

{ }×{3,3}, ,

r{3,3,3} , , , - BGCOLOR="#e0f0e0" !40 , , (1,1,1,1,1)
+ (0,0,1,1,2)√2, , Stericantellated 5-cube
cellirhombated penteractitriacontaditeron (carnit)
(Stericantellated 5-orthoplex)
, , 242, , 2080, , 4720, , 3840, , 960 , ,
 , ,
, , 
rr{4,3,3}, ,

rr{4,3}×{ }, ,

{4}×{3}, ,

{ }×rr{3,3}, ,

rr{3,3,3} , , , - BGCOLOR="#e0e0f0" !41 , , (1,1,1,1,1)
+ (0,0,1,2,2)√2, , Runcicantellated 5-cube
prismatorhombated penteract (prin)
, , 202, , 1240, , 2960, , 2880, , 960 , ,
 , ,
, , 
t0,2,3{4,3,3}, , - , ,

{4}×{3}, ,

{ }×t{3,3}, ,

2t{3,3,3} , , , - BGCOLOR="#f0e0e0" !42 , , (1,1,1,1,1)
+ (0,0,1,2,3)√2, , Stericantitruncated 5-orthoplex
celligreatorhombated triacontaditeron (cogart)
, , 242, , 2320, , 5920, , 5760, , 1920 , ,
 , ,
, , 
t0,2,3{4,3,3}, ,

rr{4,3}×{ }, ,

{6}×{4}, ,

{ }×tr{3,3}, ,

tr{3,3,3} , , , - BGCOLOR="#e0e0f0" !43 , , (1,1,1,1,1)
+ (0,1,1,1,1)√2, , Truncated 5-cube
truncated penteract (tan)
, , 42, , 200, , 400, , 400, , 160 , ,

Tetrah.pyr, ,

t{4,3,3}, , - , , - , , - , ,

{3,3,3} , , , - BGCOLOR="#e0e0f0" !44 , , (1,1,1,1,1)
+ (0,1,1,1,2)√2, , Steritruncated 5-cube
celliprismated triacontaditeron (capt)
, , 242, , 1600, , 2960, , 2240, , 640 , ,
 , ,
, , 
t{4,3,3}, ,

t{4,3}×{ }, ,

{8}×{3}, ,

{ }×{3,3}, ,

t0,3{3,3,3} , , , - BGCOLOR="#e0e0f0" !45 , , (1,1,1,1,1)
+ (0,1,1,2,2)√2, , Runcitruncated 5-cube
prismatotruncated penteract (pattin)
, , 202, , 1560, , 3760, , 3360, , 960 , ,
 , ,
, , 
t0,1,3{4,3,3} , , - , ,

{8}×{3}, ,

{ }×r{3,3} , ,

rr{3,3,3} , , , - BGCOLOR="#e0f0e0" !46 , , (1,1,1,1,1)
+ (0,1,1,2,3)√2, , Steriruncitruncated 5-cube
celliprismatotruncated penteractitriacontaditeron (captint)
(Steriruncitruncated 5-orthoplex)
, , 242, , 2160, , 5760, , 5760, , 1920 , ,
 , ,
, , 
t0,1,3{4,3,3}, ,

t{4,3}×{ }, ,

{8}×{6}, ,

{ }×t{3,3}, ,

t0,1,3{3,3,3} , , , - BGCOLOR="#e0e0f0" !47 , , (1,1,1,1,1)
+ (0,1,2,2,2)√2, , Cantitruncated 5-cube
great rhombated penteract (girn)
, , 122, , 680, , 1520, , 1600, , 640 , ,
 , ,
, , 
tr{4,3,3}, , - , , - , ,

{ }×{3,3}, ,

t{3,3,3} , , , - BGCOLOR="#e0e0f0" !48 , , (1,1,1,1,1)
+ (0,1,2,2,3)√2, , Stericantitruncated 5-cube
celligreatorhombated penteract (cogrin)
, , 242, , 2400, , 6000, , 5760, , 1920 , ,
 , ,
, , 
tr{4,3,3}, ,

tr{4,3}×{ }, ,

{8}×{3}, ,

{ }×rr{3,3}, ,

t0,1,3{3,3,3} , , , - BGCOLOR="#e0e0f0" !49 , , (1,1,1,1,1)
+ (0,1,2,3,3)√2, , Runcicantitruncated 5-cube
great prismated penteract (gippin)
, , 202, , 1560, , 4240, , 4800, , 1920 , ,
 , ,
, , 
t0,1,2,3{4,3,3}, , - , ,

{8}×{3}, ,

{ }×t{3,3}, ,

tr{3,3,3} , , , - BGCOLOR="#e0f0e0" !50 , , (1,1,1,1,1)
+ (0,1,2,3,4)√2, , Omnitruncated 5-cube
great cellated penteractitriacontaditeron (gacnet)
(omnitruncated 5-orthoplex)
, , 242, , 2640, , 8160, , 9600, , 3840 , ,

Irr. {3,3,3}, ,

tr{4,3}×{ }, ,

tr{4,3}×{ }, ,

{8}×{6}, ,

{ }×tr{3,3}, ,

t0,1,2,3{3,3,3} , , , - BGCOLOR="#d0f0f0" !51 , ,
5-demicube
In five-dimensional geometry, a demipenteract or 5-demicube is a semiregular 5-polytope, constructed from a ''5-hypercube'' (penteract) with alternated vertices removed.
It was discovered by Thorold Gosset. Since it was the only semiregular 5- ...
hemipenteract (hin)
= , 26 , 120 , 160 , 80 , 16 ,

r{3,3,3} ,

h{4,3,3} , - , - , - , - , (16)

{3,3,3} , - BGCOLOR="#d0f0f0" !52 , ,
Cantic 5-cube
In geometry of five dimensions or higher, a cantic 5-cube, cantihalf 5-cube, truncated 5-demicube is a uniform 5-polytope, being a truncation of the 5-demicube. It has half the vertices of a cantellated 5-cube.
Cartesian coordinates
The Cartesi ...
Truncated hemipenteract (thin)
= , 42 , 280 , 640 , 560 , 160 ,
 ,
, 
h2{4,3,3} , - , - , - , (16)

r{3,3,3} , (16)

t{3,3,3} , - BGCOLOR="#d0f0f0" !53 , , Runcic 5-cube
Small rhombated hemipenteract (sirhin)
= , 42 , 360 , 880 , 720 , 160 , ,

h3{4,3,3} , - , - , - , (16)

r{3,3,3} , (16)

rr{3,3,3} , - BGCOLOR="#d0f0f0" !54 , ,
Steric 5-cube
In five-dimensional geometry, a steric 5-cube or (steric 5-demicube or sterihalf 5-cube) is a convex uniform 5-polytope. There are unique 4 steric forms of the 5-cube. Steric 5-cubes have half the vertices of stericated 5-cubes.
Steric 5-cube ...
Small prismated hemipenteract (siphin)
= , 82 , 480 , 720 , 400 , 80 , ,

h{4,3,3} ,

h{4,3}×{} , - , - , (16)

{3,3,3} , (16)

t0,3{3,3,3} , - BGCOLOR="#d0f0f0" !55 , , Runcicantic 5-cube
Great rhombated hemipenteract (girhin)
= , 42 , 360 , 1040 , 1200 , 480 , ,

h2,3{4,3,3} , - , - , - , (16)

2t{3,3,3} , (16)

tr{3,3,3} , - BGCOLOR="#d0f0f0" !56 , ,
Stericantic 5-cube
In five-dimensional geometry, a steric 5-cube or (steric 5-demicube or sterihalf 5-cube) is a convex uniform 5-polytope. There are unique 4 steric forms of the 5-cube. Steric 5-cubes have half the vertices of stericated 5-cubes.
Steric 5-cub ...
Prismatotruncated hemipenteract (pithin)
= , 82 , 720 , 1840 , 1680 , 480 , ,

h2{4,3,3} ,

h2{4,3}×{} , - , - , (16)

rr{3,3,3} , (16)

t0,1,3{3,3,3} , - BGCOLOR="#d0f0f0" !57 , , Steriruncic 5-cube
Prismatorhombated hemipenteract (pirhin)
= , 82 , 560 , 1280 , 1120 , 320 , ,

h3{4,3,3} ,

h{4,3}×{} , - , - , (16)

t{3,3,3} , (16)

t0,1,3{3,3,3} , - BGCOLOR="#d0f0f0" !58 , , Steriruncicantic 5-cube
Great prismated hemipenteract (giphin)
= , 82 , 720 , 2080 , 2400 , 960 , ,

h2,3{4,3,3} ,

h2{4,3}×{} , - , - , (16)

tr{3,3,3} , (16)

t0,1,2,3{3,3,3} , - BGCOLOR="#d0f0f0" !Nonuniform , , Alternated runcicantitruncated 5-orthoplex
Snub prismatotriacontaditeron (snippit)
Snub hemipenteract (snahin)
= , 1122 , 6240 , 10880 , 6720 , 960 , ,

sr{3,3,4} , sr{2,3,4} , sr{3,2,4} , - , ht0,1,2,3{3,3,3} , (960)

Irr. {3,3,3} , - BGCOLOR="#d0f0f0" !Nonuniform , , Edge-snub 5-orthoplex
Pyritosnub penteract (pysnan)
, 1202 , 7920 , 15360 , 10560 , 1920 , , sr3{3,3,4} , sr3{2,3,4} , sr3{3,2,4} ,

s{3,3}×{ } , ht0,1,2,3{3,3,3} , (960)

Irr. {3,3}×{ } , - BGCOLOR="#d0f0f0" !Nonuniform , , Snub 5-cube
Snub penteract (snan)
, 2162 , 12240 , 21600 , 13440 , 960 , , ht0,1,2,3{3,3,4} , ht0,1,2,3{2,3,4} , ht0,1,2,3{3,2,4} , ht0,1,2,3{3,3,2} , ht0,1,2,3{3,3,3} , (1920)

Irr. {3,3,3}
The D5 family
The D5 family has symmetry of order 1920 (5! x 24). This family has 23 Wythoffian uniform polytopes, from ''3×8-1'' permutations of the D5Coxeter diagram
Harold Scott MacDonald "Donald" Coxeter, (9 February 1907 – 31 March 2003) was a British and later also Canadian geometer. He is regarded as one of the greatest geometers of the 20th century.
Biography
Coxeter was born in Kensington to ...
with one or more rings. 15 (2×8-1) are repeated from the B5 family and 8 are unique to this family, though even those 8 duplicate the alternations from the B5 family.
In the 15 repeats, both of the nodes terminating the length-1 branches are ringed, so the two kinds of element are identical and the symmetry doubles: the relations are ... = .... and ... = ..., creating a complete duplication of the uniform 5-polytopes 20 through 34 above. The 8 new forms have one such node ringed and one not, with the relation ... = ... duplicating uniform 5-polytopes 51 through 58 above.
{, class="wikitable"
!rowspan=2, #
!rowspan=2, Coxeter diagram
Harold Scott MacDonald "Donald" Coxeter, (9 February 1907 – 31 March 2003) was a British and later also Canadian geometer. He is regarded as one of the greatest geometers of the 20th century.
Biography
Coxeter was born in Kensington to ...
Schläfli symbol
In geometry, the Schläfli symbol is a notation of the form \ that defines regular polytopes and tessellations.
The Schläfli symbol is named after the 19th-century Swiss mathematician Ludwig Schläfli, who generalized Euclidean geometry to more ...
symbolsJohnson and Bowers names !colspan=5, Element counts !rowspan=2, Vertex
figure !colspan=6 , Facets by location: 1,2,1 , - !4 !3 !2 !1 !0 !
,3,3BR>(16) !
1,1,1BR>(10) !
,3�
nbsp;
In word processing and digital typesetting, a non-breaking space, , also called NBSP, required space, hard space, or fixed space (though it is not of fixed width), is a space character that prevents an automatic line break at its position. In ...
BR>(40)
! nbsp;
In word processing and digital typesetting, a non-breaking space, , also called NBSP, required space, hard space, or fixed space (though it is not of fixed width), is a space character that prevents an automatic line break at its position. In ...
� �nbsp;
In word processing and digital typesetting, a non-breaking space, , also called NBSP, required space, hard space, or fixed space (though it is not of fixed width), is a space character that prevents an automatic line break at its position. In ...
BR>(80)
! ,3,3BR>(16) ! Alt , - ! 1, =
h{4,3,3,3},
5-demicube
In five-dimensional geometry, a demipenteract or 5-demicube is a semiregular 5-polytope, constructed from a ''5-hypercube'' (penteract) with alternated vertices removed.
It was discovered by Thorold Gosset. Since it was the only semiregular 5- ...
Hemipenteract (hin) , 26 , 120 , 160 , 80 , 16 ,
r{3,3,3} ,
{3,3,3} ,
h{4,3,3} , - , - , - , , - ! 2, =
h2{4,3,3,3},
cantic 5-cube
In geometry of five dimensions or higher, a cantic 5-cube, cantihalf 5-cube, truncated 5-demicube is a uniform 5-polytope, being a truncation of the 5-demicube. It has half the vertices of a cantellated 5-cube.
Cartesian coordinates
The Cartesi ...
Truncated hemipenteract (thin) , 42 , 280 , 640 , 560 , 160 , ,
t{3,3,3} ,
h2{4,3,3} , - , - ,
r{3,3,3} , , - ! 3, =
h3{4,3,3,3}, runcic 5-cube
Small rhombated hemipenteract (sirhin) , 42 , 360 , 880 , 720 , 160 , ,
rr{3,3,3} ,
h3{4,3,3} , - , - ,
r{3,3,3} , , - ! 4, =
h4{4,3,3,3},
steric 5-cube
In five-dimensional geometry, a steric 5-cube or (steric 5-demicube or sterihalf 5-cube) is a convex uniform 5-polytope. There are unique 4 steric forms of the 5-cube. Steric 5-cubes have half the vertices of stericated 5-cubes.
Steric 5-cube ...
Small prismated hemipenteract (siphin) , 82 , 480 , 720 , 400 , 80 , ,
t0,3{3,3,3} ,
h{4,3,3} ,
h{4,3}×{} , - ,
{3,3,3} , , - ! 5, =
h2,3{4,3,3,3}, runcicantic 5-cube
Great rhombated hemipenteract (girhin) , 42 , 360 , 1040 , 1200 , 480 , ,
2t{3,3,3} ,
h2,3{4,3,3} , - , - ,
tr{3,3,3} , , - ! 6, =
h2,4{4,3,3,3},
stericantic 5-cube
In five-dimensional geometry, a steric 5-cube or (steric 5-demicube or sterihalf 5-cube) is a convex uniform 5-polytope. There are unique 4 steric forms of the 5-cube. Steric 5-cubes have half the vertices of stericated 5-cubes.
Steric 5-cub ...
Prismatotruncated hemipenteract (pithin) , 82 , 720 , 1840 , 1680 , 480 , ,
t0,1,3{3,3,3} ,
h2{4,3,3} ,
h2{4,3}×{} , - ,
rr{3,3,3} , , - ! 7, =
h3,4{4,3,3,3}, steriruncic 5-cube
Prismatorhombated hemipenteract (pirhin) , 82 , 560 , 1280 , 1120 , 320 , ,
t0,1,3{3,3,3} ,
h3{4,3,3} ,
h{4,3}×{} , - ,
t{3,3,3} , , - ! 8, =
h2,3,4{4,3,3,3}, steriruncicantic 5-cube
Great prismated hemipenteract (giphin) , 82 , 720 , 2080 , 2400 , 960 , ,
t0,1,2,3{3,3,3} ,
h2,3{4,3,3} ,
h2{4,3}×{} , - ,
tr{3,3,3} , , - bgcolor="#D0F0F0" ! Nonuniform , =
ht0,1,2,3{3,3,3,4}, alternated runcicantitruncated 5-orthoplex
Snub hemipenteract (snahin) , 1122 , 6240 , 10880 , 6720 , 960 , , ht0,1,2,3{3,3,3} ,
sr{3,3,4} , sr{2,3,4} , sr{3,2,4} , ht0,1,2,3{3,3,3} , (960)
Irr. {3,3,3}
Uniform prismatic forms
There are 5 finite categoricaluniform
A uniform is a variety of clothing worn by members of an organization while participating in that organization's activity. Modern uniforms are most often worn by armed forces and paramilitary organizations such as police, emergency services, se ...
prism
Prism usually refers to:
* Prism (optics), a transparent optical component with flat surfaces that refract light
* Prism (geometry), a kind of polyhedron
Prism may also refer to:
Science and mathematics
* Prism (geology), a type of sedimentary ...
atic families of polytopes based on the nonprismatic uniform 4-polytope
In geometry, a 4-polytope (sometimes also called a polychoron, polycell, or polyhedroid) is a four-dimensional polytope. It is a connected and closed figure, composed of lower-dimensional polytopal elements: vertices, edges, faces (polygons), an ...
s. For simplicity, most alternations are not shown.
A4 × A1
This prismatic family has 9 forms: The A1 x A4 family has symmetry of order 240 (2*5!). {, class="wikitable" !rowspan=2, # !rowspan=2,Coxeter diagram
Harold Scott MacDonald "Donald" Coxeter, (9 February 1907 – 31 March 2003) was a British and later also Canadian geometer. He is regarded as one of the greatest geometers of the 20th century.
Biography
Coxeter was born in Kensington to ...
and Schläfli
symbols
Name !colspan=5 rowspan=1, Element counts , - ! Facets, , Cells, , Faces, , Edges, , Vertices , - , 59 , = {3,3,3}×{ }
5-cell prism
In geometry, a uniform 5-polytope is a five-dimensional uniform polytope. By definition, a uniform 5-polytope is vertex-transitive and constructed from uniform 4-polytope Facet (geometry), facets.
The complete set of convex uniform 5-polytopes ...
(penp)
, 7, , 20, , 30, , 25, , 10
, -
, 60
, = r{3,3,3}×{ }Rectified 5-cell prism (rappip) , 12, , 50, , 90, , 70, , 20 , - , 61 , = t{3,3,3}×{ }
Truncated 5-cell prism (tippip) , 12, , 50, , 100, , 100, , 40 , - , 62 , = rr{3,3,3}×{ }
Cantellated 5-cell prism (srippip) , 22, , 120, , 250, , 210, , 60 , - BGCOLOR="#e0f0e0" , 63 , = t0,3{3,3,3}×{ }
Runcinated 5-cell prism (spiddip) , 32, , 130, , 200, , 140, , 40 , - BGCOLOR="#e0f0e0" , 64 , = 2t{3,3,3}×{ }
Bitruncated 5-cell prism (decap) , 12, , 60, , 140, , 150, , 60 , - , 65 , = tr{3,3,3}×{ }
Cantitruncated 5-cell prism (grippip) , 22, , 120, , 280, , 300, , 120 , - , 66 , = t0,1,3{3,3,3}×{ }
Runcitruncated 5-cell prism (prippip) , 32, , 180, , 390, , 360, , 120 , - BGCOLOR="#e0f0e0" , 67 , = t0,1,2,3{3,3,3}×{ }
Omnitruncated 5-cell prism (gippiddip) , 32, , 210, , 540, , 600, , 240
B4 × A1
This prismatic family has 16 forms. (Three are shared with ,4,3�nbsp;
In word processing and digital typesetting, a non-breaking space, , also called NBSP, required space, hard space, or fixed space (though it is not of fixed width), is a space character that prevents an automatic line break at its position. In ...
family)
The A1×B4 family has symmetry of order 768 (254!).
The last three snubs can be realised with equal-length edges, but turn out nonuniform anyway because some of their 4-faces are not uniform 4-polytopes.
{, class="wikitable"
!rowspan=2, #
!rowspan=2, Coxeter diagram
Harold Scott MacDonald "Donald" Coxeter, (9 February 1907 – 31 March 2003) was a British and later also Canadian geometer. He is regarded as one of the greatest geometers of the 20th century.
Biography
Coxeter was born in Kensington to ...
and Schläfli
symbols
Name !colspan=5 rowspan=1, Element counts , - ! Facets, , Cells, , Faces, , Edges, , Vertices , - BGCOLOR="#f0e0e0" , 6'', , = {4,3,3}×{ }
Tesseractic prism (pent)
(Same as
5-cube
In five-dimensional geometry, a 5-cube is a name for a five-dimensional hypercube with 32 vertices, 80 edges, 80 square faces, 40 cubic cells, and 10 tesseract 4-faces.
It is represented by Schläfli symbol or , constructed as 3 tesseracts, ...
)
, 10, , 40, , 80, , 80, , 32
, - BGCOLOR="#f0e0e0"
, 68, , = r{4,3,3}×{ }Rectified tesseractic prism (rittip) , 26, , 136, , 272, , 224, , 64 , - BGCOLOR="#f0e0e0" , 69, , = t{4,3,3}×{ }
Truncated tesseractic prism (tattip) , 26, , 136, , 304, , 320, , 128 , - BGCOLOR="#f0e0e0" , 70, , = rr{4,3,3}×{ }
Cantellated tesseractic prism (srittip) , 58, , 360, , 784, , 672, , 192 , - BGCOLOR="#e0f0e0" , 71, , = t0,3{4,3,3}×{ }
Runcinated tesseractic prism
In geometry, runcination is an operation that cuts a regular polytope (or honeycomb) simultaneously along the faces, edges, and vertices, creating new facets in place of the original face, edge, and vertex centers.
It is a higher order truncatio ...
(sidpithip)
, 82, , 368, , 608, , 448, , 128
, - BGCOLOR="#e0f0e0"
, 72, , = 2t{4,3,3}×{ }Bitruncated tesseractic prism (tahp) , 26, , 168, , 432, , 480, , 192 , - BGCOLOR="#f0e0e0" , 73, , = tr{4,3,3}×{ }
Cantitruncated tesseractic prism (grittip) , 58, , 360, , 880, , 960, , 384 , - BGCOLOR="#f0e0e0" , 74, , = t0,1,3{4,3,3}×{ }
Runcitruncated tesseractic prism (prohp) , 82, , 528, , 1216, , 1152, , 384 , - BGCOLOR="#e0f0e0" , 75, , = t0,1,2,3{4,3,3}×{ }
Omnitruncated tesseractic prism (gidpithip) , 82, , 624, , 1696, , 1920, , 768 , - BGCOLOR="#e0e0f0" , 76, , = {3,3,4}×{ }
16-cell prism (hexip) , 18, , 64, , 88, , 56, , 16 , - BGCOLOR="#e0e0f0" , 77, , = r{3,3,4}×{ }
Rectified 16-cell prism (icope)
(Same as 24-cell prism) , 26, , 144, , 288, , 216, , 48 , - BGCOLOR="#e0e0f0" , 78, , = t{3,3,4}×{ }
Truncated 16-cell prism (thexip) , 26, , 144, , 312, , 288, , 96 , - BGCOLOR="#e0e0f0" , 79, , = rr{3,3,4}×{ }
Cantellated 16-cell prism (ricope)
(Same as rectified 24-cell prism) , 50, , 336, , 768, , 672, , 192 , - BGCOLOR="#e0e0f0" , 80, , = tr{3,3,4}×{ }
Cantitruncated 16-cell prism (ticope)
(Same as truncated 24-cell prism) , 50, , 336, , 864, , 960, , 384 , - BGCOLOR="#e0e0f0" , 81, , = t0,1,3{3,3,4}×{ }
Runcitruncated 16-cell prism (prittip) , 82, , 528, , 1216, , 1152, , 384 , - BGCOLOR="#a0e0f0" , 82, , = sr{3,3,4}×{ }
snub 24-cell prism (sadip) , 146, , 768, , 1392, , 960, , 192 , - BGCOLOR="#a0e0f0" , Nonuniform, ,
rectified tesseractic alterprism (rita) , 50, , 288, , 464, , 288, , 64 , - BGCOLOR="#a0e0f0" , Nonuniform, ,
truncated 16-cell alterprism (thexa) , 26, , 168, , 384, , 336, , 96 , - BGCOLOR="#a0e0f0" , Nonuniform, ,
bitruncated tesseractic alterprism (taha) , 50, , 288, , 624, , 576, , 192
F4 × A1
This prismatic family has 10 forms. The A1 x F4 family has symmetry of order 2304 (2*1152). Three polytopes 85, 86 and 89 (green background) have double symmetry 3,4,32], order 4608. The last one, snub 24-cell prism, (blue background) has +,4,3,2symmetry, order 1152. {, class="wikitable" !rowspan=2, # !rowspan=2,Coxeter diagram
Harold Scott MacDonald "Donald" Coxeter, (9 February 1907 – 31 March 2003) was a British and later also Canadian geometer. He is regarded as one of the greatest geometers of the 20th century.
Biography
Coxeter was born in Kensington to ...
and Schläfli
symbols
Name !colspan=5 rowspan=1, Element counts , - ! Facets, , Cells, , Faces, , Edges, , Vertices , - , 7, = {3,4,3}×{ }
24-cell prism
In geometry, a uniform 5-polytope is a five-dimensional uniform polytope. By definition, a uniform 5-polytope is vertex-transitive and constructed from uniform 4-polytope facets.
The complete set of convex uniform 5-polytopes has not been deter ...
(icope)
, 26, , 144, , 288, , 216, , 48
, -
, 9, = r{3,4,3}×{ }rectified 24-cell prism (ricope) , 50, , 336, , 768, , 672, , 192 , - , 0, = t{3,4,3}×{ }
truncated 24-cell prism
Truncation is the term used for limiting the number of digits right of the decimal point by discarding the least significant ones.
Truncation may also refer to:
Mathematics
* Truncation (statistics) refers to measurements which have been cut of ...
(ticope)
, 50, , 336, , 864, , 960, , 384
, -
, 83, , = rr{3,4,3}×{ }cantellated 24-cell prism (sricope) , 146, , 1008, , 2304, , 2016, , 576 , - BGCOLOR="#b0f0b0" , 84, , = t0,3{3,4,3}×{ }
runcinated 24-cell prism (spiccup) , 242, , 1152, , 1920, , 1296, , 288 , - BGCOLOR="#b0f0b0" , 85, , = 2t{3,4,3}×{ }
bitruncated 24-cell prism (contip) , 50, , 432, , 1248, , 1440, , 576 , - , 86, , = tr{3,4,3}×{ }
cantitruncated 24-cell prism (gricope) , 146, , 1008, , 2592, , 2880, , 1152 , - , 87, , = t0,1,3{3,4,3}×{ }
runcitruncated 24-cell prism (pricope) , 242, , 1584, , 3648, , 3456, , 1152 , - BGCOLOR="#b0f0b0" , 88, , = t0,1,2,3{3,4,3}×{ }
omnitruncated 24-cell prism (gippiccup) , 242, , 1872, , 5088, , 5760, , 2304 , - BGCOLOR="#b0e0f0" , 2, = s{3,4,3}×{ }
snub 24-cell prism (sadip) , 146, , 768, , 1392, , 960, , 192
H4 × A1
This prismatic family has 15 forms: The A1 x H4 family has symmetry of order 28800 (2*14400). {, class="wikitable" !rowspan=2, # !rowspan=2,Coxeter diagram
Harold Scott MacDonald "Donald" Coxeter, (9 February 1907 – 31 March 2003) was a British and later also Canadian geometer. He is regarded as one of the greatest geometers of the 20th century.
Biography
Coxeter was born in Kensington to ...
and Schläfli
symbols
Name !colspan=5 rowspan=1, Element counts , - ! Facets, , Cells, , Faces, , Edges, , Vertices , - BGCOLOR="#f0e0e0" , 89, , = {5,3,3}×{ }
120-cell prism (hipe) , 122, , 960, , 2640, , 3000, , 1200 , - BGCOLOR="#f0e0e0" , 90, , = r{5,3,3}×{ }
Rectified 120-cell prism (rahipe) , 722, , 4560, , 9840, , 8400, , 2400 , - BGCOLOR="#f0e0e0" , 91, , = t{5,3,3}×{ }
Truncated 120-cell prism (thipe) , 722, , 4560, , 11040, , 12000, , 4800 , - BGCOLOR="#f0e0e0" , 92, , = rr{5,3,3}×{ }
Cantellated 120-cell prism (srahip) , 1922, , 12960, , 29040, , 25200, , 7200 , - BGCOLOR="#e0f0e0" , 93, , = t0,3{5,3,3}×{ }
Runcinated 120-cell prism (sidpixhip) , 2642, , 12720, , 22080, , 16800, , 4800 , - BGCOLOR="#e0f0e0" , 94, , = 2t{5,3,3}×{ }
Bitruncated 120-cell prism (xhip) , 722, , 5760, , 15840, , 18000, , 7200 , - BGCOLOR="#f0e0e0" , 95, , = tr{5,3,3}×{ }
Cantitruncated 120-cell prism (grahip) , 1922, , 12960, , 32640, , 36000, , 14400 , - BGCOLOR="#f0e0e0" , 96, , = t0,1,3{5,3,3}×{ }
Runcitruncated 120-cell prism (prixip) , 2642, , 18720, , 44880, , 43200, , 14400 , - BGCOLOR="#e0f0e0" , 97, , = t0,1,2,3{5,3,3}×{ }
Omnitruncated 120-cell prism (gidpixhip) , 2642, , 22320, , 62880, , 72000, , 28800 , - BGCOLOR="#e0e0f0" , 98, , = {3,3,5}×{ }
600-cell prism (exip) , 602, , 2400, , 3120, , 1560, , 240 , - BGCOLOR="#e0e0f0" , 99, , = r{3,3,5}×{ }
Rectified 600-cell prism (roxip) , 722, , 5040, , 10800, , 7920, , 1440 , - BGCOLOR="#e0e0f0" , 100, , = t{3,3,5}×{ }
Truncated 600-cell prism (texip) , 722, , 5040, , 11520, , 10080, , 2880 , - BGCOLOR="#e0e0f0" , 101, , = rr{3,3,5}×{ }
Cantellated 600-cell prism (srixip) , 1442, , 11520, , 28080, , 25200, , 7200 , - BGCOLOR="#e0e0f0" , 102, , = tr{3,3,5}×{ }
Cantitruncated 600-cell prism (grixip) , 1442, , 11520, , 31680, , 36000, , 14400 , - BGCOLOR="#e0e0f0" , 103, , = t0,1,3{3,3,5}×{ }
Runcitruncated 600-cell prism (prahip) , 2642, , 18720, , 44880, , 43200, , 14400
Duoprism prisms
Uniform duoprism prisms, {''p''}×{''q''}×{ }, form an infinite class for all integers ''p'',''q''>2. {4}×{4}×{ } makes a lower symmetry form of the5-cube
In five-dimensional geometry, a 5-cube is a name for a five-dimensional hypercube with 32 vertices, 80 edges, 80 square faces, 40 cubic cells, and 10 tesseract 4-faces.
It is represented by Schläfli symbol or , constructed as 3 tesseracts, ...
.
{, class="wikitable"
, -
!rowspan=2, Coxeter diagram
Harold Scott MacDonald "Donald" Coxeter, (9 February 1907 – 31 March 2003) was a British and later also Canadian geometer. He is regarded as one of the greatest geometers of the 20th century.
Biography
Coxeter was born in Kensington to ...
!rowspan=2, Names
!colspan=6, Element counts
, -
! 4-faces
! Cells
! Faces
! Edges
! Vertices
, - align=center
, , , {''p''}×{''q''}×{ }, , ''p''+''q''+2, , 3''pq''+3''p''+3''q'', , 4''pq''+2''p''+2''q'', , 5''pq'', , 2''pq''
, - align=center
, , , {''p''}2×{ }, , 2(''p''+1), , 3''p''(''p''+1), , 4''p''(''p''+1), , 5''p''2, , 2''p''2
, - align=center
, , , {3}2×{ }, , 8, , 36, , 48, , 45, , 18
, - align=center
, , , {4}2×{ } = 5-cube
In five-dimensional geometry, a 5-cube is a name for a five-dimensional hypercube with 32 vertices, 80 edges, 80 square faces, 40 cubic cells, and 10 tesseract 4-faces.
It is represented by Schläfli symbol or , constructed as 3 tesseracts, ...
, , 10, , 40, , 80, , 80, , 32
Grand antiprism prism
The grand antiprism prism is the only known convex non-Wythoffian uniform 5-polytope. It has 200 vertices, 1100 edges, 1940 faces (40 pentagons, 500 squares, 1400 triangles), 1360 cells (600tetrahedra
In geometry, a tetrahedron (plural: tetrahedra or tetrahedrons), also known as a triangular pyramid, is a polyhedron composed of four triangular faces, six straight edges, and four vertex corners. The tetrahedron is the simplest of all the o ...
, 40 pentagonal antiprism
In geometry, the pentagonal antiprism is the third in an infinite set of antiprisms formed by an even-numbered sequence of triangle sides closed by two polygon caps. It consists of two pentagons joined to each other by a ring of 10 triangles for ...
s, 700 triangular prism
In geometry, a triangular prism is a three-sided prism; it is a polyhedron made of a triangular base, a translated copy, and 3 faces joining corresponding sides. A right triangular prism has rectangular sides, otherwise it is ''oblique''. A unif ...
s, 20 pentagonal prism
In geometry, the pentagonal prism is a prism with a pentagonal base. It is a type of heptahedron with seven faces, fifteen edges, and ten vertices. As a semiregular (or uniform) polyhedron
If faces are all regular, the pentagonal prism is ...
s), and 322 hypercells (2 grand antiprism
In geometry, the grand antiprism or pentagonal double antiprismoid is a uniform 4-polytope (4-dimensional uniform polytope) bounded by 320 cells: 20 pentagonal antiprisms, and 300 tetrahedra. It is an anomalous, non-Wythoffian uniform 4-polytope ...
s , 20 pentagonal antiprism
In geometry, the pentagonal antiprism is the third in an infinite set of antiprisms formed by an even-numbered sequence of triangle sides closed by two polygon caps. It consists of two pentagons joined to each other by a ring of 10 triangles for ...
prisms , and 300 tetrahedral prism
In geometry, a tetrahedral prism is a convex uniform 4-polytope. This 4-polytope has 6 polyhedral cells: 2 tetrahedra connected by 4 triangular prisms. It has 14 faces: 8 triangular and 6 square. It has 16 edges and 8 vertices.
It is one of 18 u ...
s ).
{, class="wikitable"
!rowspan=2, #
!rowspan=2, Name
!colspan=5, Element counts
, -
! Facets, , Cells, , Faces, , Edges, , Vertices
, -
, 104, , grand antiprism prism (gappip), , 322, , 1360, , 1940, , 1100, , 200
Notes on the Wythoff construction for the uniform 5-polytopes
Construction of the reflective 5-dimensionaluniform polytope
In geometry, a uniform polytope of dimension three or higher is a vertex-transitive polytope bounded by uniform facets. The uniform polytopes in two dimensions are the regular polygons (the definition is different in 2 dimensions to exclude vert ...
s are done through a Wythoff construction
In geometry, a Wythoff construction, named after mathematician Willem Abraham Wythoff, is a method for constructing a uniform polyhedron or plane tiling. It is often referred to as Wythoff's kaleidoscopic construction.
Construction process
...
process, and represented through a Coxeter diagram
Harold Scott MacDonald "Donald" Coxeter, (9 February 1907 – 31 March 2003) was a British and later also Canadian geometer. He is regarded as one of the greatest geometers of the 20th century.
Biography
Coxeter was born in Kensington to ...
, where each node represents a mirror. Nodes are ringed to imply which mirrors are active. The full set of uniform polytopes generated are based on the unique permutations of ringed nodes. Uniform 5-polytopes are named in relation to the regular polytope
In mathematics, a regular polytope is a polytope whose symmetry group acts transitively on its flags, thus giving it the highest degree of symmetry. All its elements or -faces (for all , where is the dimension of the polytope) — cells, f ...
s in each family. Some families have two regular constructors and thus may have two ways of naming them.
Here are the primary operators available for constructing and naming the uniform 5-polytopes.
The last operation, the snub, and more generally the alternation, are the operation that can create nonreflective forms. These are drawn with "hollow rings" at the nodes.
The prismatic forms and bifurcating graphs can use the same truncation indexing notation, but require an explicit numbering system on the nodes for clarity.
{, class="wikitable"
!Operation
!width=200 colspan=2, ExtendedSchläfli symbol
In geometry, the Schläfli symbol is a notation of the form \ that defines regular polytopes and tessellations.
The Schläfli symbol is named after the 19th-century Swiss mathematician Ludwig Schläfli, who generalized Euclidean geometry to more ...
!width=80, Coxeter diagram
Harold Scott MacDonald "Donald" Coxeter, (9 February 1907 – 31 March 2003) was a British and later also Canadian geometer. He is regarded as one of the greatest geometers of the 20th century.
Biography
Coxeter was born in Kensington to ...
!Description
, - align=center
! Parent
, t0{p,q,r,s}
, {p,q,r,s}
,
, Any regular 5-polytope
, - align=center
! Rectified
, t1{p,q,r,s}, , r{p,q,r,s}
,
, align=left, The edges are fully truncated into single points. The 5-polytope now has the combined faces of the parent and dual.
, - align=center
! Birectified
, t2{p,q,r,s}, , 2r{p,q,r,s}
,
, align=left, Birectification reduces faces to points, cells
Cell most often refers to:
* Cell (biology), the functional basic unit of life
Cell may also refer to:
Locations
* Monastic cell, a small room, hut, or cave in which a religious recluse lives, alternatively the small precursor of a monastery w ...
to their duals
''Duals'' is a compilation album by the Irish rock band U2. It was released in April 2011 to u2.com subscribers.
Track listing
:* "Where the Streets Have No Name" and "Amazing Grace" are studio mix of U2's performance at the Rose Bowl, Pas ...
.
, - align=center
! Trirectified
, t3{p,q,r,s}, , 3r{p,q,r,s}
,
, align=left, Trirectification reduces cells to points. (Dual rectification)
, - align=center
! Quadrirectified
, t4{p,q,r,s}, , 4r{p,q,r,s}
,
, align=left, Quadrirectification reduces 4-faces to points. (Dual)
, - align=center
! Truncated
, t0,1{p,q,r,s}, , t{p,q,r,s}
,
, align=left, Each original vertex is cut off, with a new face filling the gap. Truncation has a degree of freedom, which has one solution that creates a uniform truncated 5-polytope. The 5-polytope has its original faces doubled in sides, and contains the faces of the dual., - align=center ! Cantellated , t0,2{p,q,r,s}, , rr{p,q,r,s} , , align=left, In addition to vertex truncation, each original edge is ''beveled'' with new rectangular faces appearing in their place.
, - align=center ! Runcinated , colspan=2, t0,3{p,q,r,s} , , align=left, Runcination reduces cells and creates new cells at the vertices and edges. , - align=center ! Stericated , t0,4{p,q,r,s}, , 2r2r{p,q,r,s} , , align=left, Sterication reduces facets and creates new facets (hypercells) at the vertices and edges in the gaps. (Same as
expansion
Expansion may refer to:
Arts, entertainment and media
* ''L'Expansion'', a French monthly business magazine
* ''Expansion'' (album), by American jazz pianist Dave Burrell, released in 2004
* ''Expansions'' (McCoy Tyner album), 1970
* ''Expansio ...
operation for 5-polytopes.)
, - align=center
! Omnitruncated
, colspan=2, t0,1,2,3,4{p,q,r,s}
,
, align=left, All four operators, truncation, cantellation, runcination, and sterication are applied.
, - align=center
!Half
, colspan=2, h{2p,3,q,r}
,
, align=left, Alternation, same as
, - align=center
!Cantic
, colspan=2, h2{2p,3,q,r}
,
, align=left, Same as
, - align=center
!Runcic
, colspan=2, h3{2p,3,q,r}
,
, align=left, Same as
, - align=center
!Runcicantic
, colspan=2, h2,3{2p,3,q,r}
,
, align=left, Same as
, - align=center
!Steric
, colspan=2, h4{2p,3,q,r}
,
, align=left, Same as
, - align=center
!Steriruncic
, colspan=2, h3,4{2p,3,q,r}
,
, align=left, Same as
, - align=center
!Stericantic
, colspan=2, h2,4{2p,3,q,r}
,
, align=left, Same as
, - align=center
!Steriruncicantic
, colspan=2, h2,3,4{2p,3,q,r}
,
, align=left, Same as
, - align=center
!Snub
, colspan=2, s{p,2q,r,s}
,
, align=left, Alternated truncation
, - align=center
!Snub rectified
, colspan=2, sr{p,q,2r,s}
,
, align=left, Alternated truncated rectification
, - align=center
!
, colspan=2, ht0,1,2,3{p,q,r,s}
,
, align=left, Alternated runcicantitruncation
, - align=center
!Full snub
, colspan=2, ht0,1,2,3,4{p,q,r,s}
,
, align=left, Alternated omnitruncation
Regular and uniform honeycombs
There are five fundamental affineCoxeter groups
In mathematics, a Coxeter group, named after Harold Scott MacDonald Coxeter, H. S. M. Coxeter, is an group (mathematics), abstract group that admits a group presentation, formal description in terms of Reflection (mathematics), reflections (or Kal ...
, and 13 prismatic groups that generate regular and uniform tessellations in Euclidean 4-space.
{, class=wikitable
, + Fundamental groups
, -
!#
!colspan=3, Coxeter group
In mathematics, a Coxeter group, named after H. S. M. Coxeter, is an abstract group that admits a formal description in terms of reflections (or kaleidoscopic mirrors). Indeed, the finite Coxeter groups are precisely the finite Euclidean refl ...
!Coxeter diagram
Harold Scott MacDonald "Donald" Coxeter, (9 February 1907 – 31 March 2003) was a British and later also Canadian geometer. He is regarded as one of the greatest geometers of the 20th century.
Biography
Coxeter was born in Kensington to ...
!Forms
, - align=center
, 1, , , , [5/sup>.html"_;"title=".html"_;"title="[5">[5/sup>">.html"_;"title=" [5/sup>.html"_;"title="">[5/sup>.html"_;"title=".html"_;"title="[5">[5/sup>">.html"_;"title="[5">[5/sup>">[(3,3,3,3,3).html" ;"title=""> [5/sup>.html"_;"title=".html"_;"title="[5">[5/sup>">.html"_;"title="[5">[5/sup>">[(3,3,3,3,3)">.html" ;"title="/sup>.html" ;"title="">[5/sup>.html" ;"title=".html" ;"title=" [5/sup>">.html"_;"title="[5">[5/sup>">[(3,3,3,3,3)">">.html" ;"title="">[5/sup>">.html" ;"title="[5">[5/sup>">[(3,3,3,3,3)">">">7
, - align=center
, 2, , , , , , , , 19
, - align=center
, 3, , , , [4,3,31,1, [4,3,3,4,1+, = , , 23 (8 new)
, - align=center
, 4, , , , = , , 9 (0 new)
, - align=center
, 5, , , , , , , , 31 (21 new)
There are three regular honeycombs of Euclidean 4-space:
* tesseractic honeycomb, with symbols {4,3,3,4}, = . There are 19 uniform honeycombs in this family.
* 24-cell honeycomb, with symbols {3,4,3,3}, . There are 31 reflective uniform honeycombs in this family, and one alternated form.
** Truncated 24-cell honeycomb with symbols t{3,4,3,3},
** Snub 24-cell honeycomb, with symbols s{3,4,3,3}, and constructed by four snub 24-cell, one 16-cell, and five 5-cells at each vertex.
* 16-cell honeycomb
In Four-dimensional space, four-dimensional Euclidean geometry, the 16-cell honeycomb is one of the three regular space-filling tessellations (or honeycomb (geometry), honeycombs), represented by Schläfli symbol , and constructed by a 4-dimensiona ...
, with symbols {3,3,4,3},
Other families that generate uniform honeycombs:
* There are 23 uniquely ringed forms, 8 new ones in the 16-cell honeycomb
In Four-dimensional space, four-dimensional Euclidean geometry, the 16-cell honeycomb is one of the three regular space-filling tessellations (or honeycomb (geometry), honeycombs), represented by Schläfli symbol , and constructed by a 4-dimensiona ...
family. With symbols h{4,32,4} it is geometrically identical to the 16-cell honeycomb
In Four-dimensional space, four-dimensional Euclidean geometry, the 16-cell honeycomb is one of the three regular space-filling tessellations (or honeycomb (geometry), honeycombs), represented by Schläfli symbol , and constructed by a 4-dimensiona ...
, =
* There are 7 uniquely ringed forms from the , family, all new, including:
** 4-simplex honeycomb
** Truncated 4-simplex honeycomb
** Omnitruncated 4-simplex honeycomb
In four-dimensional Euclidean geometry, the 4-simplex honeycomb, 5-cell honeycomb or pentachoric-dispentachoric honeycomb is a space-filling tessellation honeycomb (geometry), honeycomb. It is composed of 5-cells and rectified 5-cells facets in a ...
* There are 9 uniquely ringed forms in the : 1,1,1,1 family, two new ones, including the quarter tesseractic honeycomb, = , and the bitruncated tesseractic honeycomb, = .
Non-Wythoffian
In geometry, a Wythoff construction, named after mathematician Willem Abraham Wythoff, is a method for constructing a uniform polyhedron or plane tiling. It is often referred to as Wythoff's kaleidoscopic construction.
Construction process
...
uniform tessellations in 4-space also exist by elongation (inserting layers), and gyration (rotating layers) from these reflective forms.
{, class=wikitable
, + Prismatic groups
, -
!#
!colspan=2, Coxeter group
In mathematics, a Coxeter group, named after H. S. M. Coxeter, is an abstract group that admits a formal description in terms of reflections (or kaleidoscopic mirrors). Indeed, the finite Coxeter groups are precisely the finite Euclidean refl ...
!Coxeter diagram
Harold Scott MacDonald "Donald" Coxeter, (9 February 1907 – 31 March 2003) was a British and later also Canadian geometer. He is regarded as one of the greatest geometers of the 20th century.
Biography
Coxeter was born in Kensington to ...
, -
, 1, , ×, , ,3,4,2,∞,
, -
, 2, , ×, , ,31,1,2,∞,
, -
, 3, , ×, , [4/sup>,2,∞.html"_;"title=".html"_;"title="[4">[4/sup>,2,∞">.html"_;"title="[4">[4/sup>,2,∞.html" ;"title="">[4/sup>,2,∞.html" ;"title=".html" ;"title="[4">[4/sup>,2,∞">.html" ;"title="[4">[4/sup>,2,∞">
, -
, 4, , ×x, , [4,4,2,∞,2,∞,
, -
, 5, , ×x, , [6,3,2,∞,2,∞], ,
, -
, 6, , ×x, , [3/sup>,2,∞,2,∞], ,
, -
, 7, , ×xx, , ��,2,∞,2,∞,2,∞,
, -
, 8, , x, , [3/sup>,2,3[3.html"_;"title=".html"_;"title="[3">[3/sup>,2,3[3">.html"_;"title=" [3/sup>,2,3[3/sup>.html"_;"title="">[3/sup>,2,3[3.html"_;"title=".html"_;"title="[3">[3/sup>,2,3[3">.html"_;"title="[3">[3/sup>,2,3[3/sup>">">[3/sup>,2,3[3.html"_;"title=".html"_;"title="[3">[3/sup>,2,3[3">.html"_;"title="[3">[3/sup>,2,3[3/sup>.html" ;"title="">[3/sup>,2,3[3/sup>.html" ;"title="">[3/sup>,2,3[3.html" ;"title=".html" ;"title="[3">[3/sup>,2,3[3">.html" ;"title="[3">[3/sup>,2,3[3/sup>">">[3/sup>,2,3[3.html" ;"title=".html" ;"title="[3">[3/sup>,2,3[3">.html" ;"title="[3">[3/sup>,2,3[3/sup>">
, -
, 9, , ×, , [3/sup>,2,4,4,
, -
, 10, , ×, , [3/sup>,2,6,3], ,
, -
, 11, , ×, , [4,4,2,4,4], ,
, -
, 12, , ×, , [4,4,2,6,3], ,
, -
, 13, , ×, , ,3,2,6,3,
Regular and uniform hyperbolic honeycombs
;Hyperbolic compact groups There are 5 compact hyperbolic Coxeter groups of rank 5, each generating uniform honeycombs in hyperbolic 4-space as permutations of rings of the Coxeter diagrams. {, class="wikitable" , valign=top align= = 3,3,3,3,4) , valign=top align= = ,3,31,1 , valign=top align= = ,3,3,5= ,3,3,5
= ,3,3,5 There are 5 regular compact convex hyperbolic honeycombs in H4 space:Coxeter, The Beauty of Geometry: Twelve Essays, Chapter 10: Regular honeycombs in hyperbolic space, Summary tables IV p213 {, class="wikitable" , + Compact regular convex hyperbolic honeycombs , - !Honeycomb name ! Schläfli
Symbol
{p,q,r,s} !
Coxeter diagram
Harold Scott MacDonald "Donald" Coxeter, (9 February 1907 – 31 March 2003) was a British and later also Canadian geometer. He is regarded as one of the greatest geometers of the 20th century.
Biography
Coxeter was born in Kensington to ...
!Facettype
{p,q,r} !Cell
type
{p,q} !Face
type
{p} !Face
figure
{s} !Edge
figure
{r,s} ! Vertex
figure
{q,r,s} !
Dual
Dual or Duals may refer to:
Paired/two things
* Dual (mathematics), a notion of paired concepts that mirror one another
** Dual (category theory), a formalization of mathematical duality
*** see more cases in :Duality theories
* Dual (grammatical ...
, - BGCOLOR="#ffe0e0" align=center
, Order-5 5-cell (pente), , {3,3,3,5}, , , , {3,3,3}, , {3,3}, , {3}, , {5}, , {3,5}, , {3,3,5}, , {5,3,3,3}
, - BGCOLOR="#e0e0ff" align=center
, Order-3 120-cell (hitte), , {5,3,3,3}, , , , {5,3,3}, , {5,3}, , {5}, , {3}, , {3,3}, , {3,3,3}, , {3,3,3,5}
, - BGCOLOR="#ffe0e0" align=center
, Order-5 tesseractic (pitest), , {4,3,3,5}, , , , {4,3,3}, , {4,3}, , {4}, , {5}, , {3,5}, , {3,3,5}, , {5,3,3,4}
, - BGCOLOR="#e0e0ff" align=center
, Order-4 120-cell (shitte), , {5,3,3,4}, , , , {5,3,3}, , {5,3}, , {5}, , {4}, , {3,4}, , {3,3,4}, , {4,3,3,5}
, - BGCOLOR="#e0ffe0" align=center
, Order-5 120-cell (phitte), , {5,3,3,5}, , , , {5,3,3}, , {5,3}, , {5}, , {5}, , {3,5}, , {3,3,5}, , Self-dual
There are also 4 regular compact hyperbolic star-honeycombs in H4 space:
{, class="wikitable"
, + Compact regular hyperbolic star-honeycombs
, -
!Honeycomb name
! SchläfliSymbol
{p,q,r,s} !
Coxeter diagram
Harold Scott MacDonald "Donald" Coxeter, (9 February 1907 – 31 March 2003) was a British and later also Canadian geometer. He is regarded as one of the greatest geometers of the 20th century.
Biography
Coxeter was born in Kensington to ...
!Facettype
{p,q,r} !Cell
type
{p,q} !Face
type
{p} !Face
figure
{s} !Edge
figure
{r,s} ! Vertex
figure
{q,r,s} !
Dual
Dual or Duals may refer to:
Paired/two things
* Dual (mathematics), a notion of paired concepts that mirror one another
** Dual (category theory), a formalization of mathematical duality
*** see more cases in :Duality theories
* Dual (grammatical ...
, - BGCOLOR="#ffe0e0" align=center
, Order-3 small stellated 120-cell, , {5/2,5,3,3}, , , , {5/2,5,3}, , {5/2,5}, , {5}, , {5}, , {3,3}, , {5,3,3}, , {3,3,5,5/2}
, - BGCOLOR="#e0e0ff" align=center
, Order-5/2 600-cell, , {3,3,5,5/2}, , , , {3,3,5}, , {3,3}, , {3}, , {5/2}, , {5,5/2}, , {3,5,5/2}, , {5/2,5,3,3}
, - BGCOLOR="#ffe0e0" align=center
, Order-5 icosahedral 120-cell, , {3,5,5/2,5}, , , , {3,5,5/2}, , {3,5}, , {3}, , {5}, , {5/2,5}, , {5,5/2,5}, , {5,5/2,5,3}
, - BGCOLOR="#e0e0ff" align=center
, Order-3 great 120-cell, , {5,5/2,5,3}, , , , {5,5/2,5}, , {5,5/2}, , {5}, , {3}, , {5,3}, , {5/2,5,3}, , {3,5,5/2,5}
;Hyperbolic paracompact groups
There are 9 paracompact hyperbolic Coxeter groups of rank 5, each generating uniform honeycombs in 4-space as permutations of rings of the Coxeter diagrams. Paracompact groups generate honeycombs with infinite facets
A facet is a flat surface of a geometric shape, e.g., of a cut gemstone.
Facet may also refer to:
Arts, entertainment, and media
* ''Facets'' (album), an album by Jim Croce
* ''Facets'', a 1980 album by jazz pianist Monty Alexander and his tri ...
or vertex figure
In geometry, a vertex figure, broadly speaking, is the figure exposed when a corner of a polyhedron or polytope is sliced off.
Definitions
Take some corner or Vertex (geometry), vertex of a polyhedron. Mark a point somewhere along each connect ...
s.
{, class=wikitable
, align=
= ,3[4/sup>.html"_;"title=".html"_;"title=",3[4">,3[4/sup>">.html"_;"title=",3[4">,3[4/sup>_
_=_[4,3[4.html" ;"title="">,3[4/sup>.html" ;"title=".html" ;"title=",3[4">,3[4/sup>">.html" ;"title=",3[4">,3[4/sup>
= [4,3[4">">,3[4/sup>.html" ;"title=".html" ;"title=",3[4">,3[4/sup>">.html" ;"title=",3[4">,3[4/sup>
= [4,3[4/sup>]: = [(3,3,4,3,4)]:
= [3 �[]]: , align= = ,/3\,3,4
= ,4,31,1
= ,32,1
= ,31,1,1 , align= = ,4,3,4
Notes
References
* T. Gosset: ''On the Regular and Semi-Regular Figures in Space of n Dimensions'',Messenger of Mathematics
The ''Messenger of Mathematics'' is a defunct British mathematics journal. The founding editor-in-chief was William Allen Whitworth with Charles Taylor and volumes 1–58 were published between 1872 and 1929. James Whitbread Lee Glaisher was the ...
, Macmillan, 1900 (3 regular and one semiregular 4-polytope)
* A. Boole Stott: ''Geometrical deduction of semiregular from regular polytopes and space fillings'', Verhandelingen of the Koninklijke academy van Wetenschappen width unit Amsterdam, Eerste Sectie 11,1, Amsterdam, 1910
* H.S.M. Coxeter
Harold Scott MacDonald "Donald" Coxeter, (9 February 1907 – 31 March 2003) was a British and later also Canadian geometer. He is regarded as one of the greatest geometers of the 20th century.
Biography
Coxeter was born in Kensington t ...
:
** H.S.M. Coxeter
Harold Scott MacDonald "Donald" Coxeter, (9 February 1907 – 31 March 2003) was a British and later also Canadian geometer. He is regarded as one of the greatest geometers of the 20th century.
Biography
Coxeter was born in Kensington t ...
, ''Regular Polytopes'', 3rd Edition, Dover New York, 1973 (p. 297 Fundamental regions for irreducible groups generated by reflections, Spherical and Euclidean)
** H.S.M. Coxeter
Harold Scott MacDonald "Donald" Coxeter, (9 February 1907 – 31 March 2003) was a British and later also Canadian geometer. He is regarded as one of the greatest geometers of the 20th century.
Biography
Coxeter was born in Kensington t ...
, ''The Beauty of Geometry: Twelve Essays'' (Chapter 10: Regular honeycombs in hyperbolic space, Summary tables IV p213)
* Kaleidoscopes: Selected Writings of H.S.M. Coxeter, edited by F. Arthur Sherk, Peter McMullen, Anthony C. Thompson, Asia Ivic Weiss, Wiley-Interscience Publication, 1995,** (Paper 22) H.S.M. Coxeter, ''Regular and Semi Regular Polytopes I'', ath. Zeit. 46 (1940) 380-407, MR 2,10** (Paper 23) H.S.M. Coxeter, ''Regular and Semi-Regular Polytopes II'', ath. Zeit. 188 (1985) 559-591(p. 287 5D Euclidean groups, p. 298 Four-dimensionsal honeycombs) ** (Paper 24) H.S.M. Coxeter, ''Regular and Semi-Regular Polytopes III'', ath. Zeit. 200 (1988) 3-45* N.W. Johnson: ''The Theory of Uniform Polytopes and Honeycombs'', Ph.D. Dissertation, University of Toronto, 1966 * James E. Humphreys, ''Reflection Groups and Coxeter Groups'', Cambridge studies in advanced mathematics, 29 (1990) (Page 141, 6.9 List of hyperbolic Coxeter groups, figure 2
External links
* – includes nonconvex forms as well as the duplicate constructions from the B5 and D5 families {{Honeycombs 5-polytopes eo:5-hiperpluredro