|
5-polytope
In geometry, a five-dimensional polytope (or 5-polytope) is a polytope in five-dimensional space, bounded by (4-polytope) facets, pairs of which share a polyhedral cell. Definition A 5-polytope is a closed five-dimensional figure with vertices, edges, faces, and cells, and 4-faces. A vertex is a point where five or more edges meet. An edge is a line segment where four or more faces meet, and a face is a polygon where three or more cells meet. A cell is a polyhedron, and a 4-face is a 4-polytope. Furthermore, the following requirements must be met: # Each cell must join exactly two 4-faces. # Adjacent 4-faces are not in the same four-dimensional hyperplane. # The figure is not a compound of other figures which meet the requirements. Characteristics The topology of any given 5-polytope is defined by its Betti numbers and torsion coefficients.Richeson, D.; ''Euler's Gem: The Polyhedron Formula and the Birth of Topoplogy'', Princeton, 2008. The value of the Euler characteristic ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Expanded 5-simplex
In five-dimensional geometry, a stericated 5-simplex is a convex uniform 5-polytope with fourth-order truncations (sterication) of the regular 5-simplex. There are six unique sterications of the 5-simplex, including permutations of truncations, cantellations, and runcinations. The simplest stericated 5-simplex is also called an expanded 5-simplex, with the first and last nodes ringed, for being constructible by an expansion operation applied to the regular 5-simplex. The highest form, the ''steriruncicantitruncated 5-simplex'' is more simply called an omnitruncated 5-simplex with all of the nodes ringed. Stericated 5-simplex A stericated 5-simplex can be constructed by an expansion operation applied to the regular 5-simplex, and thus is also sometimes called an expanded 5-simplex. It has 30 vertices, 120 edges, 210 faces (120 triangles and 90 squares), 180 cells (60 tetrahedra and 120 triangular prisms) and 62 4-faces (12 5-cells, 30 tetrahedral prisms and 20 3-3 duopri ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Rectified 5-orthoplex
In five-dimensional geometry, a rectified 5-orthoplex is a convex uniform 5-polytope, being a rectification of the regular 5-orthoplex. There are 5 degrees of rectifications for any 5-polytope, the zeroth here being the 5-orthoplex itself, and the 4th and last being the 5-cube. Vertices of the rectified 5-orthoplex are located at the edge-centers of the 5-orthoplex. Vertices of the birectified 5-orthoplex are located in the triangular face centers of the 5-orthoplex. Rectified 5-orthoplex Its 40 vertices represent the root vectors of the simple Lie group D5. The vertices can be seen in 3 hyperplanes, with the 10 vertices rectified 5-cells cells on opposite sides, and 20 vertices of a runcinated 5-cell passing through the center. When combined with the 10 vertices of the 5-orthoplex, these vertices represent the 50 root vectors of the B5 and C5 simple Lie groups. E. L. Elte identified it in 1912 as a semiregular polytope, identifying it as Cr51 as a first rectification of a 5-d ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
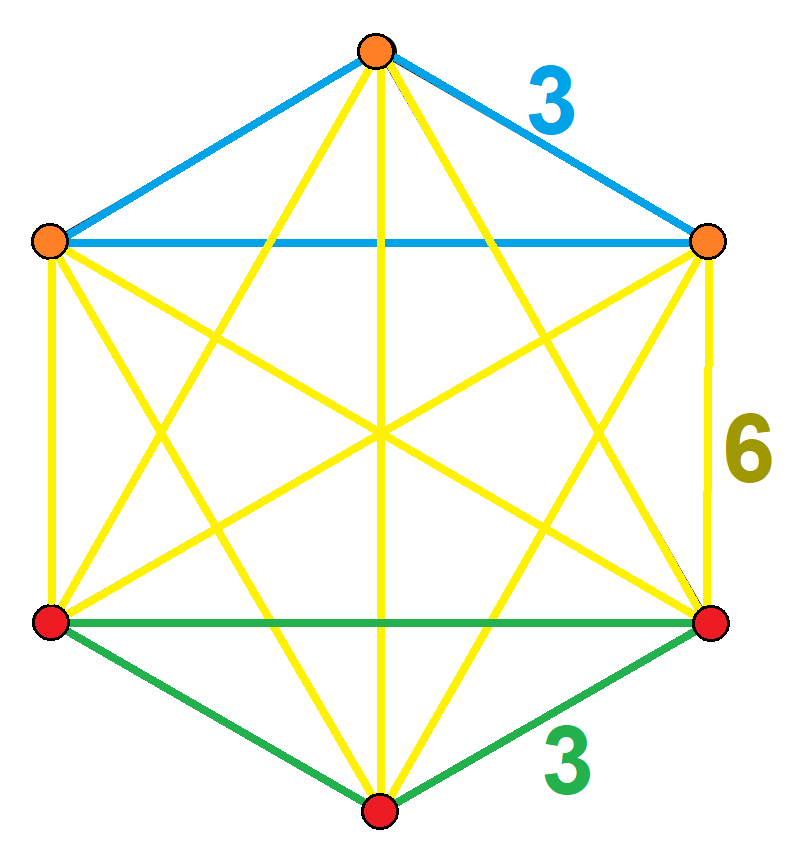
5-simplex T0
In five-dimensional geometry, a 5- simplex is a self-dual regular 5-polytope. It has six vertices, 15 edges, 20 triangle faces, 15 tetrahedral cells, and 6 5-cell facets. It has a dihedral angle of cos−1(), or approximately 78.46°. The 5-simplex is a solution to the problem: ''Make 20 equilateral triangles using 15 matchsticks, where each side of every triangle is exactly one matchstick.'' Alternate names It can also be called a hexateron, or hexa-5-tope, as a 6- facetted polytope in 5-dimensions. The name ''hexateron'' is derived from ''hexa-'' for having six facets and '' teron'' (with ''ter-'' being a corruption of ''tetra-'') for having four-dimensional facets. By Jonathan Bowers, a hexateron is given the acronym hix. As a configuration This configuration matrix represents the 5-simplex. The rows and columns correspond to vertices, edges, faces, cells and 4-faces. The diagonal numbers say how many of each element occur in the whole 5-simplex. The nondiagonal num ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
5-simplex
In five-dimensional geometry, a 5-simplex is a self-dual regular 5-polytope. It has six vertices, 15 edges, 20 triangle faces, 15 tetrahedral cells, and 6 5-cell facets. It has a dihedral angle of cos−1(), or approximately 78.46°. The 5-simplex is a solution to the problem: ''Make 20 equilateral triangles using 15 matchsticks, where each side of every triangle is exactly one matchstick.'' Alternate names It can also be called a hexateron, or hexa-5-tope, as a 6- facetted polytope in 5-dimensions. The name ''hexateron'' is derived from ''hexa-'' for having six facets and '' teron'' (with ''ter-'' being a corruption of ''tetra-'') for having four-dimensional facets. By Jonathan Bowers, a hexateron is given the acronym hix. As a configuration This configuration matrix represents the 5-simplex. The rows and columns correspond to vertices, edges, faces, cells and 4-faces. The diagonal numbers say how many of each element occur in the whole 5-simplex. The nondiagonal numb ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
5-simplex T04 A4
In five-dimensional geometry, a 5- simplex is a self-dual regular 5-polytope. It has six vertices, 15 edges, 20 triangle faces, 15 tetrahedral cells, and 6 5-cell facets. It has a dihedral angle of cos−1(), or approximately 78.46°. The 5-simplex is a solution to the problem: ''Make 20 equilateral triangles using 15 matchsticks, where each side of every triangle is exactly one matchstick.'' Alternate names It can also be called a hexateron, or hexa-5-tope, as a 6- facetted polytope in 5-dimensions. The name ''hexateron'' is derived from ''hexa-'' for having six facets and '' teron'' (with ''ter-'' being a corruption of ''tetra-'') for having four-dimensional facets. By Jonathan Bowers, a hexateron is given the acronym hix. As a configuration This configuration matrix represents the 5-simplex. The rows and columns correspond to vertices, edges, faces, cells and 4-faces. The diagonal numbers say how many of each element occur in the whole 5-simplex. The nondiagonal num ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
5-cube T4
In five-dimensional geometry, a 5-cube is a name for a five-dimensional hypercube with 32 vertices, 80 edges, 80 square faces, 40 cubic cells, and 10 tesseract 4-faces. It is represented by Schläfli symbol or , constructed as 3 tesseracts, , around each cubic ridge. It can be called a penteract, a portmanteau of the Greek word , for 'five' (dimensions), and the word ''tesseract'' (the 4-cube). It can also be called a regular deca-5-tope or decateron, being a 5-dimensional polytope constructed from 10 regular facets. Related polytopes It is a part of an infinite hypercube family. The dual of a 5-cube is the 5-orthoplex, of the infinite family of orthoplexes. Applying an '' alternation'' operation, deleting alternating vertices of the 5-cube, creates another uniform 5-polytope, called a 5-demicube, which is also part of an infinite family called the demihypercubes. The 5-cube can be seen as an ''order-3 tesseractic honeycomb'' on a 4-sphere. It is related to the Euclide ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
5-cube T0
In five-dimensional geometry, a 5-cube is a name for a five-dimensional hypercube with 32 vertices, 80 edges, 80 square faces, 40 cubic cells, and 10 tesseract 4-faces. It is represented by Schläfli symbol or , constructed as 3 tesseracts, , around each cubic ridge. It can be called a penteract, a portmanteau of the Greek word , for 'five' (dimensions), and the word ''tesseract'' (the 4-cube). It can also be called a regular deca-5-tope or decateron, being a 5-dimensional polytope constructed from 10 regular facets. Related polytopes It is a part of an infinite hypercube family. The dual of a 5-cube is the 5-orthoplex, of the infinite family of orthoplexes. Applying an '' alternation'' operation, deleting alternating vertices of the 5-cube, creates another uniform 5-polytope, called a 5-demicube, which is also part of an infinite family called the demihypercubes. The 5-cube can be seen as an ''order-3 tesseractic honeycomb'' on a 4-sphere. It is related to the Euclide ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
5-cube
In five-dimensional geometry, a 5-cube is a name for a five-dimensional hypercube with 32 vertices, 80 edges, 80 square faces, 40 cubic cells, and 10 tesseract 4-faces. It is represented by Schläfli symbol or , constructed as 3 tesseracts, , around each cubic ridge. It can be called a penteract, a portmanteau of the Greek word , for 'five' (dimensions), and the word ''tesseract'' (the 4-cube). It can also be called a regular deca-5-tope or decateron, being a 5-dimensional polytope constructed from 10 regular facets. Related polytopes It is a part of an infinite hypercube family. The dual of a 5-cube is the 5-orthoplex, of the infinite family of orthoplexes. Applying an '' alternation'' operation, deleting alternating vertices of the 5-cube, creates another uniform 5-polytope, called a 5-demicube, which is also part of an infinite family called the demihypercubes. The 5-cube can be seen as an ''order-3 tesseractic honeycomb'' on a 4-sphere. It is related to the Euclidea ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
5-cube T3
In five-dimensional geometry, a 5-cube is a name for a five-dimensional hypercube with 32 vertices, 80 edges, 80 square faces, 40 cubic cells, and 10 tesseract 4-faces. It is represented by Schläfli symbol or , constructed as 3 tesseracts, , around each cubic ridge. It can be called a penteract, a portmanteau of the Greek word , for 'five' (dimensions), and the word ''tesseract'' (the 4-cube). It can also be called a regular deca-5-tope or decateron, being a 5-dimensional polytope constructed from 10 regular facets. Related polytopes It is a part of an infinite hypercube family. The dual of a 5-cube is the 5-orthoplex, of the infinite family of orthoplexes. Applying an '' alternation'' operation, deleting alternating vertices of the 5-cube, creates another uniform 5-polytope, called a 5-demicube, which is also part of an infinite family called the demihypercubes. The 5-cube can be seen as an ''order-3 tesseractic honeycomb'' on a 4-sphere. It is related to the Eucl ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
5-demicube T0 D5
In five-dimensional geometry, a demipenteract or 5-demicube is a semiregular 5-polytope, constructed from a ''5-hypercube'' (penteract) with alternated vertices removed. It was discovered by Thorold Gosset. Since it was the only semiregular 5-polytope (made of more than one type of regular facets), he called it a 5-ic semi-regular. E. L. Elte identified it in 1912 as a semiregular polytope, labeling it as HM5 for a 5-dimensional ''half measure'' polytope. Coxeter named this polytope as 121 from its Coxeter diagram, which has branches of length 2, 1 and 1 with a ringed node on one of the short branches, and Schläfli symbol \left\ or . It exists in the k21 polytope family as 121 with the Gosset polytopes: 221, 321, and 421. The graph formed by the vertices and edges of the demipenteract is sometimes called the Clebsch graph, though that name sometimes refers to the folded cube graph of order five instead. Cartesian coordinates Cartesian coordinates for the vertices ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
5-demicube
In five-dimensional geometry, a demipenteract or 5-demicube is a semiregular 5-polytope, constructed from a ''5-hypercube'' (penteract) with alternated vertices removed. It was discovered by Thorold Gosset. Since it was the only semiregular 5-polytope (made of more than one type of regular facets), he called it a 5-ic semi-regular. E. L. Elte identified it in 1912 as a semiregular polytope, labeling it as HM5 for a 5-dimensional ''half measure'' polytope. Coxeter named this polytope as 121 from its Coxeter diagram, which has branches of length 2, 1 and 1 with a ringed node on one of the short branches, and Schläfli symbol \left\ or . It exists in the k21 polytope family as 121 with the Gosset polytopes: 221, 321, and 421. The graph formed by the vertices and edges of the demipenteract is sometimes called the Clebsch graph, though that name sometimes refers to the folded cube graph of order five instead. Cartesian coordinates Cartesian coordinates for the vertices of ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
List Of Regular Polytopes
This article lists the regular polytopes and regular polytope compounds in Euclidean geometry, Euclidean, spherical geometry, spherical and hyperbolic geometry, hyperbolic spaces. The Schläfli symbol describes every regular tessellation of an ''n''-sphere, Euclidean and hyperbolic spaces. A Schläfli symbol describing an ''n''-polytope equivalently describes a tessellation of an (''n'' − 1)-sphere. In addition, the symmetry of a regular polytope or tessellation is expressed as a Coxeter group, which Coxeter expressed identically to the Schläfli symbol, except delimiting by square brackets, a notation that is called Coxeter notation. Another related symbol is the Coxeter-Dynkin diagram which represents a symmetry group with no rings, and the represents regular polytope or tessellation with a ring on the first node. For example, the cube has Schläfli symbol , and with its octahedral symmetry, [4,3] or , it is represented by Coxeter diagram . The regular polytopes are ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |