Stokes wave on:
[Wikipedia]
[Google]
[Amazon]

 In
In

 According to Stokes's third-order theory, the
According to Stokes's third-order theory, the
 The surface elevation ''η'' and the velocity potential Φ are, according to Stokes's second-order theory of surface gravity waves on a fluid layer of
The surface elevation ''η'' and the velocity potential Φ are, according to Stokes's second-order theory of surface gravity waves on a fluid layer of
 For Stokes waves under the action of gravity, the third-order
For Stokes waves under the action of gravity, the third-order
 A fundamental problem in finding solutions for surface gravity waves is that
A fundamental problem in finding solutions for surface gravity waves is that
 ''Stokes's wave theory'', when using a low order of the perturbation expansion (e.g. up to second, third or fifth order), is valid for nonlinear waves on intermediate and deep water, that is for
''Stokes's wave theory'', when using a low order of the perturbation expansion (e.g. up to second, third or fifth order), is valid for nonlinear waves on intermediate and deep water, that is for
 By use of computer models, the Stokes expansion for surface gravity waves has been continued, up to high (117th) order by . Schwartz has found that the amplitude ''a'' (or ''a''1) of the first-order fundamental reaches a maximum ''before'' the maximum
By use of computer models, the Stokes expansion for surface gravity waves has been continued, up to high (117th) order by . Schwartz has found that the amplitude ''a'' (or ''a''1) of the first-order fundamental reaches a maximum ''before'' the maximum
 The maximum wave steepness, for periodic and propagating deep-water waves, is , so the wave height is about one-seventh () of the wavelength λ. And surface gravity waves of this maximum height have a sharp wave crest – with an angle of 120° (in the fluid domain) – also for finite depth, as shown by Stokes in 1880.
An accurate estimate of the highest wave steepness in deep water () was already made in 1893, by John Henry Michell, using a numerical method. A more detailed study of the behaviour of the highest wave near the sharp-cornered crest has been published by Malcolm A. Grant, in 1973. The existence of the highest wave on deep water with a sharp-angled crest of 120° was proved by John Toland in 1978. The convexity of η(x) between the successive maxima with a sharp-angled crest of 120° was independently proven by C.J. Amick et al. and Pavel I. Plotnikov in 1982
.
The highest Stokes wave – under the action of gravity – can be approximated with the following simple and accurate representation of the
The maximum wave steepness, for periodic and propagating deep-water waves, is , so the wave height is about one-seventh () of the wavelength λ. And surface gravity waves of this maximum height have a sharp wave crest – with an angle of 120° (in the fluid domain) – also for finite depth, as shown by Stokes in 1880.
An accurate estimate of the highest wave steepness in deep water () was already made in 1893, by John Henry Michell, using a numerical method. A more detailed study of the behaviour of the highest wave near the sharp-cornered crest has been published by Malcolm A. Grant, in 1973. The existence of the highest wave on deep water with a sharp-angled crest of 120° was proved by John Toland in 1978. The convexity of η(x) between the successive maxima with a sharp-angled crest of 120° was independently proven by C.J. Amick et al. and Pavel I. Plotnikov in 1982
.
The highest Stokes wave – under the action of gravity – can be approximated with the following simple and accurate representation of the
This was shown by T. Brooke Benjamin and Jim E. Feir in 1967. The
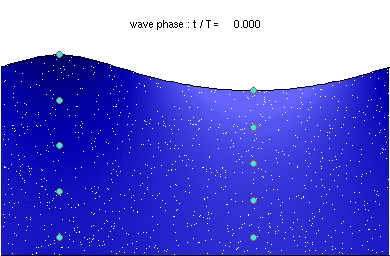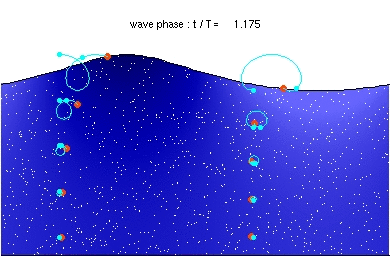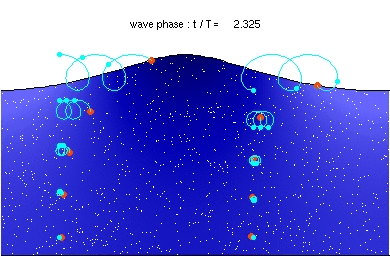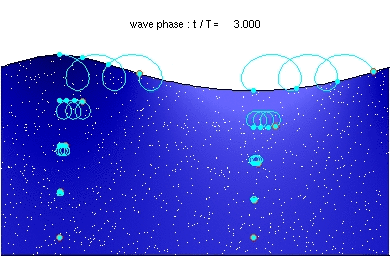 The waves of permanent form propagate with a constant
The waves of permanent form propagate with a constant
:Reprinted in: *
:Reprinted in: *
: And in (including corrections): : * * * * * * * and
:
 In
In fluid dynamics
In physics, physical chemistry and engineering, fluid dynamics is a subdiscipline of fluid mechanics that describes the flow of fluids – liquids and gases. It has several subdisciplines, including (the study of air and other gases in motion ...
, a Stokes wave is a nonlinear
In mathematics and science, a nonlinear system (or a non-linear system) is a system in which the change of the output is not proportional to the change of the input. Nonlinear problems are of interest to engineers, biologists, physicists, mathe ...
and periodic surface wave
In physics, a surface wave is a mechanical wave that propagates along the Interface (chemistry), interface between differing media. A common example is gravity waves along the surface of liquids, such as ocean waves. Gravity waves can also occu ...
on an inviscid fluid layer of constant mean depth.
This type of modelling has its origins in the mid 19th century when Sir George Stokes – using a perturbation series approach, now known as the Stokes expansion – obtained approximate solutions for nonlinear wave motion.
Stokes's wave theory is of direct practical use for waves on intermediate and deep water. It is used in the design of coastal
A coast (coastline, shoreline, seashore) is the land next to the sea or the line that forms the boundary between the land and the ocean or a lake. Coasts are influenced by the topography of the surrounding landscape and by aquatic erosion, su ...
and offshore structures, in order to determine the wave kinematics
In physics, kinematics studies the geometrical aspects of motion of physical objects independent of forces that set them in motion. Constrained motion such as linked machine parts are also described as kinematics.
Kinematics is concerned with s ...
(free surface
In physics, a free surface is the surface of a fluid that is subject to zero parallel shear stress,
such as the interface between two homogeneous fluids.
An example of two such homogeneous fluids would be a body of water (liquid) and the air in ...
elevation and flow velocities). The wave kinematics are subsequently needed in the design process
A design is the concept or proposal for an object, process, or system. The word ''design'' refers to something that is or has been intentionally created by a thinking agent, and is sometimes used to refer to the inherent nature of something ...
to determine the wave load
In physics, mathematics, engineering, and related fields, a wave is a propagating dynamic disturbance (change from equilibrium) of one or more quantities. '' Periodic waves'' oscillate repeatedly about an equilibrium (resting) value at some f ...
s on a structure. For long waves (as compared to depth) – and using only a few terms in the Stokes expansion – its applicability is limited to waves of small amplitude
The amplitude of a periodic variable is a measure of its change in a single period (such as time or spatial period). The amplitude of a non-periodic signal is its magnitude compared with a reference value. There are various definitions of am ...
. In such shallow water, a cnoidal wave
In fluid dynamics, a cnoidal wave is a nonlinear and exact periodic wave solution of the Korteweg–de Vries equation. These solutions are in terms of the Jacobi elliptic function ''cn'', which is why they are coined ''cn''oidal waves. They ar ...
theory often provides better periodic-wave approximations.
While, in the strict sense, ''Stokes wave'' refers to a progressive periodic wave of permanent form, the term is also used in connection with standing wave
In physics, a standing wave, also known as a stationary wave, is a wave that oscillates in time but whose peak amplitude profile does not move in space. The peak amplitude of the wave oscillations at any point in space is constant with respect t ...
s and even random waves.
Examples
The examples below describe Stokes waves under the action of gravity (withoutsurface tension
Surface tension is the tendency of liquid surfaces at rest to shrink into the minimum surface area possible. Surface tension (physics), tension is what allows objects with a higher density than water such as razor blades and insects (e.g. Ge ...
effects) in case of pure wave motion, so without an ambient mean current.
Third-order Stokes wave on deep water
free surface
In physics, a free surface is the surface of a fluid that is subject to zero parallel shear stress,
such as the interface between two homogeneous fluids.
An example of two such homogeneous fluids would be a body of water (liquid) and the air in ...
elevation ''η'', the velocity potential
A velocity potential is a scalar potential used in potential flow theory. It was introduced by Joseph-Louis Lagrange in 1788.
It is used in continuum mechanics, when a continuum occupies a simply-connected region and is irrotational. In such a ca ...
Φ, the phase speed
The phase velocity of a wave is the rate at which the wave propagates in any medium. This is the velocity at which the phase of any one frequency component of the wave travels. For such a component, any given phase of the wave (for example, ...
(or celerity) ''c'' and the wave phase
Phase or phases may refer to:
Science
*State of matter, or phase, one of the distinct forms in which matter can exist
*Phase (matter), a region of space throughout which all physical properties are essentially uniform
*Phase space, a mathematica ...
''θ'' are, for a progressive surface gravity wave on deep water – i.e. the fluid layer has infinite depth:
where
* ''x'' is the horizontal coordinate;
* ''z'' is the vertical coordinate, with the positive ''z''-direction upward – opposing to the direction of the Earth's gravity
The gravity of Earth, denoted by , is the net acceleration that is imparted to objects due to the combined effect of gravitation (from mass distribution within Earth) and the centrifugal force (from the Earth's rotation).
It is a vector qu ...
– and ''z'' = 0 corresponding with the mean
A mean is a quantity representing the "center" of a collection of numbers and is intermediate to the extreme values of the set of numbers. There are several kinds of means (or "measures of central tendency") in mathematics, especially in statist ...
surface elevation;
* ''t'' is time;
* ''a'' is the first-order wave amplitude
The amplitude of a periodic variable is a measure of its change in a single period (such as time or spatial period). The amplitude of a non-periodic signal is its magnitude compared with a reference value. There are various definitions of am ...
;
* ''k'' is the angular wavenumber
In the physical sciences, the wavenumber (or wave number), also known as repetency, is the spatial frequency of a wave. Ordinary wavenumber is defined as the number of wave cycles divided by length; it is a physical quantity with dimension of r ...
, with ''λ'' being the wavelength
In physics and mathematics, wavelength or spatial period of a wave or periodic function is the distance over which the wave's shape repeats.
In other words, it is the distance between consecutive corresponding points of the same ''phase (waves ...
;
* ''ω'' is the angular frequency
In physics, angular frequency (symbol ''ω''), also called angular speed and angular rate, is a scalar measure of the angle rate (the angle per unit time) or the temporal rate of change of the phase argument of a sinusoidal waveform or sine ...
, where ''τ'' is the period, and
* ''g'' is the strength
Strength may refer to:
Personal trait
*Physical strength, as in people or animals
*Character strengths like those listed in the Values in Action Inventory
*The exercise of willpower
Physics
* Mechanical strength, the ability to withstand ...
of the Earth's gravity, a constant in this approximation.
The expansion parameter ''ka'' is known as the wave steepness. The phase speed increases with increasing nonlinearity ''ka'' of the waves. The wave height
In fluid dynamics, the wave height of a surface wave is the difference between the elevations of a crest and a neighboring trough. ''Wave height'' is a term used by mariners, as well as in coastal, ocean and naval engineering.
At sea, the ...
''H'', being the difference between the surface elevation ''η'' at a crest and a trough
Trough may refer to:
In science
* Trough (geology), a long depression less steep than a trench
* Trough (meteorology), an elongated region of low atmospheric pressure
* Trough (physics), the lowest point on a wave
* Trough level (medicine), the l ...
, is:
Note that the second- and third-order terms in the velocity potential Φ are zero. Only at fourth order do contributions deviating from first-order theory – i.e. Airy wave theory – appear. Up to third order the orbital velocity field ''u'' = ∇Φ consists of a circular motion of the velocity vector at each position (''x'',''z''). As a result, the surface elevation of deep-water waves is to a good approximation trochoidal, as already noted by .
Stokes further observed, that although (in this Eulerian description) the third-order orbital velocity field consists of a circular motion at each point, the Lagrangian paths of fluid parcel
In fluid dynamics, a fluid parcel, also known as a fluid element or material element, is an infinitesimal volume of fluid, identifiable throughout its dynamic history while moving with the fluid flow. As it moves, the mass of a fluid parcel rema ...
s are not closed circles. This is due to the reduction of the velocity amplitude at increasing depth below the surface. This Lagrangian drift of the fluid parcels is known as the Stokes drift
For a pure wave motion in fluid dynamics, the Stokes drift velocity is the average velocity when following a specific fluid parcel as it travels with the fluid flow. For instance, a particle floating at the free surface of water waves, experienc ...
.
Second-order Stokes wave on arbitrary depth
mean
A mean is a quantity representing the "center" of a collection of numbers and is intermediate to the extreme values of the set of numbers. There are several kinds of means (or "measures of central tendency") in mathematics, especially in statist ...
depth ''h'':
Observe that for finite depth the velocity potential Φ contains a linear drift in time, independent of position (''x'' and ''z''). Both this temporal drift and the double-frequency term (containing sin 2θ) in Φ vanish for deep-water waves.
Stokes and Ursell parameters
The ratio ' of the free-surface amplitudes at second order and first order – according to Stokes's second-order theory – is: In deep water, for large ''kh'' the ratio ' has theasymptote
In analytic geometry, an asymptote () of a curve is a line such that the distance between the curve and the line approaches zero as one or both of the ''x'' or ''y'' coordinates tends to infinity. In projective geometry and related contexts, ...
For long waves, i.e. small ''kh'', the ratio ' behaves as
or, in terms of the wave height and wavelength :
with
Here ' is the Ursell parameter (or Stokes parameter). For long waves () of small height ''H'', i.e. , second-order Stokes theory is applicable. Otherwise, for fairly long waves () of appreciable height ''H'' a cnoidal wave
In fluid dynamics, a cnoidal wave is a nonlinear and exact periodic wave solution of the Korteweg–de Vries equation. These solutions are in terms of the Jacobi elliptic function ''cn'', which is why they are coined ''cn''oidal waves. They ar ...
description is more appropriate. According to Hedges, fifth-order Stokes theory is applicable for , and otherwise fifth-order cnoidal wave
In fluid dynamics, a cnoidal wave is a nonlinear and exact periodic wave solution of the Korteweg–de Vries equation. These solutions are in terms of the Jacobi elliptic function ''cn'', which is why they are coined ''cn''oidal waves. They ar ...
theory is preferable.
Third-order dispersion relation
dispersion relation
In the physical sciences and electrical engineering, dispersion relations describe the effect of dispersion on the properties of waves in a medium. A dispersion relation relates the wavelength or wavenumber of a wave to its frequency. Given the ...
is – according to Stokes's first definition of celerity:
This third-order dispersion relation is a direct consequence of avoiding secular terms, when inserting the second-order Stokes solution into the third-order equations (of the perturbation series for the periodic wave problem).
In deep water (short wavelength compared to the depth):
and in shallow water (long wavelengths compared to the depth):
As shown above, the long-wave Stokes expansion for the dispersion relation will only be valid for small enough values of the Ursell parameter: .
Overview
Stokes's approach to the nonlinear wave problem
 A fundamental problem in finding solutions for surface gravity waves is that
A fundamental problem in finding solutions for surface gravity waves is that boundary condition
In the study of differential equations, a boundary-value problem is a differential equation subjected to constraints called boundary conditions. A solution to a boundary value problem is a solution to the differential equation which also satis ...
s have to be applied at the position of the free surface
In physics, a free surface is the surface of a fluid that is subject to zero parallel shear stress,
such as the interface between two homogeneous fluids.
An example of two such homogeneous fluids would be a body of water (liquid) and the air in ...
, which is not known beforehand and is thus a part of the solution to be found.
Sir George Stokes solved this nonlinear wave problem in 1847 by expanding the relevant potential flow
In fluid dynamics, potential flow or irrotational flow refers to a description of a fluid flow with no vorticity in it. Such a description typically arises in the limit of vanishing viscosity, i.e., for an inviscid fluid and with no vorticity pre ...
quantities in a Taylor series
In mathematics, the Taylor series or Taylor expansion of a function is an infinite sum of terms that are expressed in terms of the function's derivatives at a single point. For most common functions, the function and the sum of its Taylor ser ...
around the mean (or still) surface elevation. As a result, the boundary conditions can be expressed in terms of quantities at the mean (or still) surface elevation (which is fixed and known).
Next, a solution for the nonlinear wave problem (including the Taylor series expansion around the mean or still surface elevation) is sought by means of a perturbation series – known as the ''Stokes expansion'' – in terms of a small parameter, most often the wave steepness. The unknown terms in the expansion can be solved sequentially. Often, only a small number of terms is needed to provide a solution of sufficient accuracy for engineering purposes. Typical applications are in the design of coastal
A coast (coastline, shoreline, seashore) is the land next to the sea or the line that forms the boundary between the land and the ocean or a lake. Coasts are influenced by the topography of the surrounding landscape and by aquatic erosion, su ...
and offshore structures, and of ships
A ship is a large vessel that travels the world's oceans and other navigable waterways, carrying cargo or passengers, or in support of specialized missions, such as defense, research and fishing. Ships are generally distinguished from boats, ...
.
Another property of nonlinear waves is that the phase speed
The phase velocity of a wave is the rate at which the wave propagates in any medium. This is the velocity at which the phase of any one frequency component of the wave travels. For such a component, any given phase of the wave (for example, ...
of nonlinear waves depends on the wave height
In fluid dynamics, the wave height of a surface wave is the difference between the elevations of a crest and a neighboring trough. ''Wave height'' is a term used by mariners, as well as in coastal, ocean and naval engineering.
At sea, the ...
. In a perturbation-series approach, this easily gives rise to a spurious secular variation
The secular variation of a time series is its long-term, non-periodic variation (see '' Decomposition of time series''). Whether a variation is perceived as secular or not depends on the available timescale: a variation that is secular over a times ...
of the solution, in contradiction with the periodic behaviour of the waves. Stokes solved this problem by also expanding the dispersion relationship into a perturbation series, by a method now known as the Lindstedt–Poincaré method.
Applicability
wavelength
In physics and mathematics, wavelength or spatial period of a wave or periodic function is the distance over which the wave's shape repeats.
In other words, it is the distance between consecutive corresponding points of the same ''phase (waves ...
s (''λ'') not large as compared with the mean depth (''h''). In shallow water, the low-order Stokes expansion breaks down (gives unrealistic results) for appreciable wave amplitude (as compared to the depth). Then, Boussinesq approximations are more appropriate. Further approximations on Boussinesq-type (multi-directional) wave equations lead – for one-way wave propagation – to the Korteweg–de Vries equation or the Benjamin–Bona–Mahony equation. Like (near) exact Stokes-wave solutions, these two equations have solitary wave (soliton
In mathematics and physics, a soliton is a nonlinear, self-reinforcing, localized wave packet that is , in that it preserves its shape while propagating freely, at constant velocity, and recovers it even after collisions with other such local ...
) solutions, besides periodic-wave solutions known as cnoidal wave
In fluid dynamics, a cnoidal wave is a nonlinear and exact periodic wave solution of the Korteweg–de Vries equation. These solutions are in terms of the Jacobi elliptic function ''cn'', which is why they are coined ''cn''oidal waves. They ar ...
s.
Modern extensions
Already in 1914, Wilton extended the Stokes expansion for deep-water surface gravity waves to tenth order, although introducing errors at the eight order. A fifth-order theory for finite depth was derived by De in 1955. For engineering use, the fifth-order formulations of Fenton are convenient, applicable to both Stokes first andsecond
The second (symbol: s) is a unit of time derived from the division of the day first into 24 hours, then to 60 minutes, and finally to 60 seconds each (24 × 60 × 60 = 86400). The current and formal definition in the International System of U ...
definition of phase speed (celerity). The demarcation between when fifth-order Stokes theory is preferable over fifth-order cnoidal wave
In fluid dynamics, a cnoidal wave is a nonlinear and exact periodic wave solution of the Korteweg–de Vries equation. These solutions are in terms of the Jacobi elliptic function ''cn'', which is why they are coined ''cn''oidal waves. They ar ...
theory is for Ursell parameters below about 40.
Different choices for the frame of reference and expansion parameters are possible in Stokes-like approaches to the nonlinear wave problem. In 1880, Stokes himself inverted the dependent and independent variables, by taking the velocity potential
A velocity potential is a scalar potential used in potential flow theory. It was introduced by Joseph-Louis Lagrange in 1788.
It is used in continuum mechanics, when a continuum occupies a simply-connected region and is irrotational. In such a ca ...
and stream function
In fluid dynamics, two types of stream function (or streamfunction) are defined:
* The two-dimensional (or Lagrange) stream function, introduced by Joseph Louis Lagrange in 1781, is defined for incompressible flow, incompressible (divergence-free ...
as the independent variables, and the coordinates (''x'',''z'') as the dependent variables, with ''x'' and ''z'' being the horizontal and vertical coordinates respectively. This has the advantage that the free surface, in a frame of reference in which the wave is steady (i.e. moving with the phase velocity), corresponds with a line on which the stream function is a constant. Then the free surface location is known beforehand, and not an unknown part of the solution. The disadvantage is that the radius of convergence
In mathematics, the radius of convergence of a power series is the radius of the largest Disk (mathematics), disk at the Power series, center of the series in which the series Convergent series, converges. It is either a non-negative real number o ...
of the rephrased series expansion reduces.
Another approach is by using the Lagrangian frame of reference, following the fluid parcel
In fluid dynamics, a fluid parcel, also known as a fluid element or material element, is an infinitesimal volume of fluid, identifiable throughout its dynamic history while moving with the fluid flow. As it moves, the mass of a fluid parcel rema ...
s. The Lagrangian formulations show enhanced convergence, as compared to the formulations in both the Eulerian frame, and in the frame with the potential and streamfunction as independent variables.
An exact solution for nonlinear pure capillary waves of permanent form, and for infinite fluid depth, was obtained by Crapper in 1957. Note that these capillary waves – being short waves forced by surface tension
Surface tension is the tendency of liquid surfaces at rest to shrink into the minimum surface area possible. Surface tension (physics), tension is what allows objects with a higher density than water such as razor blades and insects (e.g. Ge ...
, if gravity effects are negligible – have sharp troughs and flat crests. This contrasts with nonlinear surface gravity waves, which have sharp crests and flat troughs.
wave height
In fluid dynamics, the wave height of a surface wave is the difference between the elevations of a crest and a neighboring trough. ''Wave height'' is a term used by mariners, as well as in coastal, ocean and naval engineering.
At sea, the ...
''H'' is reached. Consequently, the wave steepness ''ka'' in terms of wave amplitude is not a monotone function up to the highest wave, and Schwartz utilizes instead ''kH'' as the expansion parameter. To estimate the highest wave in deep water, Schwartz has used Padé approximant
In mathematics, a Padé approximant is the "best" approximation of a function near a specific point by a rational function of given order. Under this technique, the approximant's power series agrees with the power series of the function it is ap ...
s and Domb–Sykes plots in order to improve the convergence of the Stokes expansion.
Extended tables of Stokes waves on various depths, computed by a different method (but in accordance with the results by others), are provided in .
Several exact relationships exist between integral properties – such as kinetic and potential energy
In physics, potential energy is the energy of an object or system due to the body's position relative to other objects, or the configuration of its particles. The energy is equal to the work done against any restoring forces, such as gravity ...
, horizontal wave momentum
In Newtonian mechanics, momentum (: momenta or momentums; more specifically linear momentum or translational momentum) is the product of the mass and velocity of an object. It is a vector quantity, possessing a magnitude and a direction. ...
and radiation stress – as found by . He shows, for deep-water waves, that many of these integral properties have a maximum before the maximum wave height is reached (in support of Schwartz's findings). , using a method similar to the one of Schwartz, computed and tabulated integral properties for a wide range of finite water depths (all reaching maxima below the highest wave height). Further, these integral properties play an important role in the conservation law
In physics, a conservation law states that a particular measurable property of an isolated physical system does not change as the system evolves over time. Exact conservation laws include conservation of mass-energy, conservation of linear momen ...
s for water waves, through Noether's theorem
Noether's theorem states that every continuous symmetry of the action of a physical system with conservative forces has a corresponding conservation law. This is the first of two theorems (see Noether's second theorem) published by the mat ...
.
In 2005, Hammack, Henderson and Segur have provided the first experimental evidence for the existence of three-dimensional progressive waves of permanent form in deep water – that is bi-periodic and two-dimensional progressive wave patterns of permanent form. The existence of these three-dimensional steady deep-water waves has been revealed in 2002, from a bifurcation study of two-dimensional Stokes waves by Craig and Nicholls, using numerical methods.
Convergence and instability
Convergence
Convergence of the Stokes expansion was first proved by for the case of small-amplitude waves – on the free surface of a fluid of infinite depth. This was extended shortly afterwards by for the case of finite depth and small-amplitude waves. Near the end of the 20th century, it was shown that for finite-amplitude waves the convergence of the Stokes expansion depends strongly on the formulation of the periodic wave problem. For instance, an inverse formulation of the periodic wave problem as used by Stokes – with the spatial coordinates as a function ofvelocity potential
A velocity potential is a scalar potential used in potential flow theory. It was introduced by Joseph-Louis Lagrange in 1788.
It is used in continuum mechanics, when a continuum occupies a simply-connected region and is irrotational. In such a ca ...
and stream function
In fluid dynamics, two types of stream function (or streamfunction) are defined:
* The two-dimensional (or Lagrange) stream function, introduced by Joseph Louis Lagrange in 1781, is defined for incompressible flow, incompressible (divergence-free ...
– does not converge for high-amplitude waves. While other formulations converge much more rapidly, e.g. in the Eulerian frame of reference (with the velocity potential or stream function as a function of the spatial coordinates).
Highest wave
free surface
In physics, a free surface is the surface of a fluid that is subject to zero parallel shear stress,
such as the interface between two homogeneous fluids.
An example of two such homogeneous fluids would be a body of water (liquid) and the air in ...
elevation ''η''(''x'',''t''):
with
for
and shifted horizontally over an integer
An integer is the number zero (0), a positive natural number (1, 2, 3, ...), or the negation of a positive natural number (−1, −2, −3, ...). The negations or additive inverses of the positive natural numbers are referred to as negative in ...
number of wavelengths to represent the other waves in the regular wave train. This approximation is accurate to within 0.7% everywhere, as compared with the "exact" solution for the highest wave.
Another accurate approximation – however less accurate than the previous one – of the fluid motion on the surface of the steepest wave is by analogy with the swing of a pendulum
A pendulum is a device made of a weight suspended from a pivot so that it can swing freely. When a pendulum is displaced sideways from its resting, equilibrium position, it is subject to a restoring force due to gravity that will accelerate i ...
in a grandfather clock
A grandfather clock (also a longcase clock, tall-case clock, grandfather's clock, hall clock or floor clock) is a tall, freestanding, weight-driven pendulum clock, with the pendulum held inside the tower or waist of the case. Clocks of this styl ...
.
Large library of Stokes waves computed with high precision for the case of infinite depth, represented with high accuracy (at least 27 digits after decimal point) as a Padé approximant
In mathematics, a Padé approximant is the "best" approximation of a function near a specific point by a rational function of given order. Under this technique, the approximant's power series agrees with the power series of the function it is ap ...
can be found at StokesWave.org
Instability
In deeper water, Stokes waves are unstable.For a review of the instability of Stokes waves see e.g.:This was shown by T. Brooke Benjamin and Jim E. Feir in 1967. The
Benjamin–Feir instability
In the fields of nonlinear optics and fluid dynamics, modulational instability or sideband instability is a phenomenon whereby deviations from a periodic waveform are reinforced by nonlinearity, leading to the generation of Frequency spectrum, spec ...
is a side-band or modulational instability, with the side-band modulations propagating in the same direction as the carrier wave
In telecommunications, a carrier wave, carrier signal, or just carrier, is a periodic waveform (usually sinusoidal) that conveys information through a process called ''modulation''. One or more of the wave's properties, such as amplitude or freq ...
; waves become unstable on deeper water for a relative depth (with ''k'' the wavenumber
In the physical sciences, the wavenumber (or wave number), also known as repetency, is the spatial frequency of a wave. Ordinary wavenumber is defined as the number of wave cycles divided by length; it is a physical quantity with dimension of ...
and ''h'' the mean water depth). The Benjamin–Feir instability can be described with the nonlinear Schrödinger equation
In theoretical physics, the (one-dimensional) nonlinear Schrödinger equation (NLSE) is a nonlinear variation of the Schrödinger equation. It is a classical field equation whose principal applications are to the propagation of light in nonli ...
, by inserting a Stokes wave with side bands. Subsequently, with a more refined analysis, it has been shown – theoretically and experimentally – that the Stokes wave and its side bands exhibit Fermi–Pasta–Ulam–Tsingou recurrence: a cyclic alternation between modulation and demodulation.
In 1978 Longuet-Higgins, by means of numerical modelling of fully non-linear waves and modulations (propagating in the carrier wave direction), presented a detailed analysis of the region of instability in deep water: both for superharmonics (for perturbations at the spatial scales smaller than the wavelength ) and subharmonics (for perturbations at the spatial scales larger than ).
With increase of Stokes wave's amplitude, new modes of superharmonic instability appear. Appearance of a new branch of instability happens when the energy of the wave passes extremum. Detailed analysis of the mechanism of appearance of the new branches of instability has shown that their behavior follows closely a simple law, which allows to find with a good accuracy instability growth rates for all known and predicted branches.
In Longuet-Higgins studies of two-dimensional wave motion, as well as the subsequent studies of three-dimensional modulations by McLean et al., new types of instabilities were found – these are associated with resonant
Resonance is a phenomenon that occurs when an object or system is subjected to an external force or vibration whose frequency matches a resonant frequency (or resonance frequency) of the system, defined as a frequency that generates a maximu ...
wave interactions between five (or more) wave components.
Stokes expansion
Governing equations for a potential flow
In many instances, the oscillatory flow in the fluid interior of surface waves can be described accurately usingpotential flow
In fluid dynamics, potential flow or irrotational flow refers to a description of a fluid flow with no vorticity in it. Such a description typically arises in the limit of vanishing viscosity, i.e., for an inviscid fluid and with no vorticity pre ...
theory, apart from boundary layer
In physics and fluid mechanics, a boundary layer is the thin layer of fluid in the immediate vicinity of a Boundary (thermodynamic), bounding surface formed by the fluid flowing along the surface. The fluid's interaction with the wall induces ...
s near the free surface and bottom (where vorticity
In continuum mechanics, vorticity is a pseudovector (or axial vector) field that describes the local spinning motion of a continuum near some point (the tendency of something to rotate), as would be seen by an observer located at that point an ...
is important, due to viscous effects, see Stokes boundary layer). Then, the flow velocity
In continuum mechanics the flow velocity in fluid dynamics, also macroscopic velocity in statistical mechanics, or drift velocity in electromagnetism, is a vector field used to mathematically describe the motion of a continuum. The length of the f ...
u can be described as the gradient
In vector calculus, the gradient of a scalar-valued differentiable function f of several variables is the vector field (or vector-valued function) \nabla f whose value at a point p gives the direction and the rate of fastest increase. The g ...
of a velocity potential
A velocity potential is a scalar potential used in potential flow theory. It was introduced by Joseph-Louis Lagrange in 1788.
It is used in continuum mechanics, when a continuum occupies a simply-connected region and is irrotational. In such a ca ...
:
Consequently, assuming incompressible flow
In fluid mechanics, or more generally continuum mechanics, incompressible flow is a flow in which the material density does not vary over time. Equivalently, the divergence of an incompressible flow velocity is zero. Under certain conditions, t ...
, the velocity field u is divergence-free and the velocity potential satisfies Laplace's equation
In mathematics and physics, Laplace's equation is a second-order partial differential equation named after Pierre-Simon Laplace, who first studied its properties in 1786. This is often written as
\nabla^2\! f = 0 or \Delta f = 0,
where \Delt ...
in the fluid interior.
The fluid region is described using three-dimensional Cartesian coordinate
In geometry, a Cartesian coordinate system (, ) in a plane is a coordinate system that specifies each point uniquely by a pair of real numbers called ''coordinates'', which are the signed distances to the point from two fixed perpendicular o ...
s (''x'',''y'',''z''), with ''x'' and ''y'' the horizontal coordinates, and ''z'' the vertical coordinate – with the positive ''z''-direction opposing the direction of the gravitational acceleration
In physics, gravitational acceleration is the acceleration of an object in free fall within a vacuum (and thus without experiencing drag (physics), drag). This is the steady gain in speed caused exclusively by gravitational attraction. All bodi ...
. Time is denoted with ''t''. The free surface is located at , and the bottom of the fluid region is at .
The free-surface boundary condition
In the study of differential equations, a boundary-value problem is a differential equation subjected to constraints called boundary conditions. A solution to a boundary value problem is a solution to the differential equation which also satis ...
s for surface gravity waves – using a potential flow
In fluid dynamics, potential flow or irrotational flow refers to a description of a fluid flow with no vorticity in it. Such a description typically arises in the limit of vanishing viscosity, i.e., for an inviscid fluid and with no vorticity pre ...
description – consist of a ''kinematic'' and a ''dynamic'' boundary condition.
The ''kinematic'' boundary condition ensures that the normal component
In mathematics, given a vector at a point on a curve, that vector can be decomposed uniquely as a sum of two vectors, one tangent to the curve, called the tangential component of the vector, and another one perpendicular to the curve, called the no ...
of the fluid's flow velocity
In continuum mechanics the flow velocity in fluid dynamics, also macroscopic velocity in statistical mechanics, or drift velocity in electromagnetism, is a vector field used to mathematically describe the motion of a continuum. The length of the f ...
, in matrix notation, at the free surface equals the normal velocity component of the free-surface motion :
The ''dynamic'' boundary condition states that, without surface tension
Surface tension is the tendency of liquid surfaces at rest to shrink into the minimum surface area possible. Surface tension (physics), tension is what allows objects with a higher density than water such as razor blades and insects (e.g. Ge ...
effects, the atmospheric pressure just above the free surface equals the fluid pressure
Pressure (symbol: ''p'' or ''P'') is the force applied perpendicular to the surface of an object per unit area over which that force is distributed. Gauge pressure (also spelled ''gage'' pressure)The preferred spelling varies by country and eve ...
just below the surface. For an unsteady potential flow this means that the Bernoulli equation
Bernoulli's principle is a key concept in fluid dynamics that relates pressure, speed and height. For example, for a fluid flowing horizontally Bernoulli's principle states that an increase in the speed occurs simultaneously with a decrease i ...
is to be applied at the free surface. In case of a constant atmospheric pressure, the dynamic boundary condition becomes:
where the constant atmospheric pressure has been taken equal to zero, without loss of generality
''Without loss of generality'' (often abbreviated to WOLOG, WLOG or w.l.o.g.; less commonly stated as ''without any loss of generality'' or ''with no loss of generality'') is a frequently used expression in mathematics. The term is used to indicat ...
.
Both boundary conditions contain the potential as well as the surface elevation ''η''. A (dynamic) boundary condition in terms of only the potential can be constructed by taking the material derivative
In continuum mechanics, the material derivative describes the time rate of change of some physical quantity (like heat or momentum) of a material element that is subjected to a space-and-time-dependent macroscopic velocity field. The material de ...
of the dynamic boundary condition, and using the kinematic boundary condition:
At the bottom of the fluid layer, impermeability requires the normal component
In mathematics, given a vector at a point on a curve, that vector can be decomposed uniquely as a sum of two vectors, one tangent to the curve, called the tangential component of the vector, and another one perpendicular to the curve, called the no ...
of the flow velocity to vanish:
where ''h''(''x'',''y'') is the depth of the bed below the datum
Data ( , ) are a collection of discrete or continuous value (semiotics), values that convey information, describing the quantity, qualitative property, quality, fact, statistics, other basic units of meaning, or simply sequences of symbols t ...
and ''n'' is the coordinate component in the direction normal to the bed.
For permanent waves above a horizontal bed, the mean depth ''h'' is a constant and the boundary condition at the bed becomes:
Taylor series in the free-surface boundary conditions
The free-surface boundary conditions and apply at the yet unknown free-surface elevation . They can be transformed into boundary conditions at a fixed elevation by use ofTaylor series
In mathematics, the Taylor series or Taylor expansion of a function is an infinite sum of terms that are expressed in terms of the function's derivatives at a single point. For most common functions, the function and the sum of its Taylor ser ...
expansions of the flow field around that elevation.
Without loss of generality the mean surface elevation – around which the Taylor series are developed – can be taken at . This assures the expansion is around an elevation in the proximity of the actual free-surface elevation. Convergence of the Taylor series for small-amplitude steady-wave motion was proved by .
The following notation is used: the Taylor series of some field around – and evaluated at – is:
with subscript zero meaning evaluation at , e.g.: .
Applying the Taylor expansion to free-surface boundary condition in terms of the potential Φ gives:
showing terms up to triple products of ''η'', ''Φ'' and ''u'', as required for the construction of the Stokes expansion up to third-order ((''ka'')3). Here, ''ka'' is the wave steepness, with ''k'' a characteristic wavenumber
In the physical sciences, the wavenumber (or wave number), also known as repetency, is the spatial frequency of a wave. Ordinary wavenumber is defined as the number of wave cycles divided by length; it is a physical quantity with dimension of ...
and ''a'' a characteristic wave amplitude
The amplitude of a periodic variable is a measure of its change in a single period (such as time or spatial period). The amplitude of a non-periodic signal is its magnitude compared with a reference value. There are various definitions of am ...
for the problem under study. The fields ''η'', ''Φ'' and ''u'' are assumed to be '(''ka'').
The dynamic free-surface boundary condition can be evaluated in terms of quantities at as:
The advantages of these Taylor-series expansions fully emerge in combination with a perturbation-series approach, for weakly non-linear waves .
Perturbation-series approach
The perturbation series are in terms of a small ordering parameter – which subsequently turns out to be proportional to (and of the order of) the wave slope ''ka'', see the series solution in this section. So, take : When applied in the flow equations, they should be valid independent of the particular value of ''ε''. By equating in powers of ''ε'', each term proportional to ''ε'' to a certain power has to equal to zero. As an example of how the perturbation-series approach works, consider the non-linear boundary condition ; it becomes: The resulting boundary conditions at for the first three orders are: ;First order: ;Second order: ;Third order: In a similar fashion – from the dynamic boundary condition – the conditions at at the orders 1, 2 and 3 become: ;First order: ;Second order: ;Third order: For the linear equations , and the perturbation technique results in a series of equations independent of the perturbation solutions at other orders: } The above perturbation equations can be solved sequentially, i.e. starting with first order, thereafter continuing with the second order, third order, etc.Application to progressive periodic waves of permanent form
 The waves of permanent form propagate with a constant
The waves of permanent form propagate with a constant phase velocity
The phase velocity of a wave is the rate at which the wave propagates in any medium. This is the velocity at which the phase of any one frequency component of the wave travels. For such a component, any given phase of the wave (for example, t ...
(or celerity), denoted as ''c''. If the steady wave motion is in the horizontal ''x''-direction, the flow quantities ''η'' and u are not separately dependent on ''x'' and time ''t'', but are functions of :
Further the waves are periodic – and because they are also of permanent form – both in horizontal space ''x'' and in time ''t'', with wavelength
In physics and mathematics, wavelength or spatial period of a wave or periodic function is the distance over which the wave's shape repeats.
In other words, it is the distance between consecutive corresponding points of the same ''phase (waves ...
''λ'' and period ''τ'' respectively. Note that ''Φ''(''x'',''z'',''t'') itself is not necessary periodic due to the possibility of a constant (linear) drift in ''x'' and/or ''t'':
with ''φ''(''x'',''z'',''t'') – as well as the derivatives ∂''Φ''/∂''t'' and ∂''Φ''/∂''x'' – being periodic. Here ''β'' is the mean flow velocity below trough
Trough may refer to:
In science
* Trough (geology), a long depression less steep than a trench
* Trough (meteorology), an elongated region of low atmospheric pressure
* Trough (physics), the lowest point on a wave
* Trough level (medicine), the l ...
level, and ''γ'' is related to the hydraulic head
Hydraulic head or piezometric head is a measurement related to liquid pressure (normalized by specific weight) and the liquid elevation above a vertical datum., 410 pages. See pp. 43–44., 650 pages. See p. 22, eq.3.2a.
It is usually meas ...
as observed in a frame of reference
In physics and astronomy, a frame of reference (or reference frame) is an abstract coordinate system, whose origin (mathematics), origin, orientation (geometry), orientation, and scale (geometry), scale have been specified in physical space. It ...
moving with the wave's phase velocity ''c'' (so the flow becomes steady in this reference frame).
In order to apply the Stokes expansion to progressive periodic waves, it is advantageous to describe them through Fourier series
A Fourier series () is an Series expansion, expansion of a periodic function into a sum of trigonometric functions. The Fourier series is an example of a trigonometric series. By expressing a function as a sum of sines and cosines, many problems ...
as a function of the wave phase
In physics and mathematics, the phase (symbol φ or ϕ) of a wave or other periodic function F of some real variable t (such as time) is an angle-like quantity representing the fraction of the cycle covered up to t. It is expressed in such a s ...
''θ''(''x'',''t''):
assuming waves propagating in the ''x''–direction. Here is the wavenumber
In the physical sciences, the wavenumber (or wave number), also known as repetency, is the spatial frequency of a wave. Ordinary wavenumber is defined as the number of wave cycles divided by length; it is a physical quantity with dimension of ...
, is the angular frequency
In physics, angular frequency (symbol ''ω''), also called angular speed and angular rate, is a scalar measure of the angle rate (the angle per unit time) or the temporal rate of change of the phase argument of a sinusoidal waveform or sine ...
and is the phase velocity
The phase velocity of a wave is the rate at which the wave propagates in any medium. This is the velocity at which the phase of any one frequency component of the wave travels. For such a component, any given phase of the wave (for example, t ...
.
Now, the free surface elevation ''η''(''x'',''t'') of a periodic wave can be described as the Fourier series
A Fourier series () is an Series expansion, expansion of a periodic function into a sum of trigonometric functions. The Fourier series is an example of a trigonometric series. By expressing a function as a sum of sines and cosines, many problems ...
:
Similarly, the corresponding expression for the velocity potential ''Φ''(''x'',''z'',''t'') is:
satisfying both the Laplace equation
In mathematics and physics, Laplace's equation is a second-order partial differential equation named after Pierre-Simon Laplace, who first studied its properties in 1786. This is often written as
\nabla^2\! f = 0 or \Delta f = 0,
where \Delt ...
in the fluid interior, as well as the boundary condition at the bed .
For a given value of the wavenumber ''k'', the parameters: ''A''n, ''B''n (with ), ''c'', ''β'' and ''γ'' have yet to be determined. They all can be expanded as perturbation series in ''ε''. provides these values for fifth-order Stokes's wave theory.
For progressive periodic waves, derivatives with respect to ''x'' and ''t'' of functions ''f''(''θ'',''z'') of ''θ''(''x'',''t'') can be expressed as derivatives with respect to ''θ'':
The important point for non-linear waves – in contrast to linear Airy wave theory – is that the phase velocity ''c'' also depends on the wave amplitude ''a'', besides its dependence on wavelength and mean depth ''h''. Negligence of the dependence of ''c'' on wave amplitude results in the appearance of secular terms, in the higher-order contributions to the perturbation-series solution. already applied the required non-linear correction to the phase speed ''c'' in order to prevent secular behaviour. A general approach to do so is now known as the Lindstedt–Poincaré method. Since the wavenumber ''k'' is given and thus fixed, the non-linear behaviour of the phase velocity is brought into account by also expanding the angular frequency ''ω'' into a perturbation series:
Here ''ω''0 will turn out to be related to the wavenumber ''k'' through the linear dispersion relation
In the physical sciences and electrical engineering, dispersion relations describe the effect of dispersion on the properties of waves in a medium. A dispersion relation relates the wavelength or wavenumber of a wave to its frequency. Given the ...
. However time derivatives, through , now also give contributions – containing ''ω''1, ''ω''2, etc. – to the governing equations at higher orders in the perturbation series. By tuning ''ω''1, ''ω''2, etc., secular behaviour can be prevented. For surface gravity waves, it is found that and the first non-zero contribution to the dispersion relation comes from ''ω''2 (see e.g. the sub-section " Third-order dispersion relation" above).
Stokes's two definitions of wave celerity
For non-linear surface waves there is, in general, ambiguity in splitting the total motion into a wave part and amean
A mean is a quantity representing the "center" of a collection of numbers and is intermediate to the extreme values of the set of numbers. There are several kinds of means (or "measures of central tendency") in mathematics, especially in statist ...
part. As a consequence, there is some freedom in choosing the phase speed (celerity) of the wave. identified two logical definitions of phase speed, known as Stokes's first and second definition of wave celerity:
#Stokes's first definition of wave celerity has, for a pure wave motion, the mean value
A mean is a quantity representing the "center" of a collection of numbers and is intermediate to the extreme values of the set of numbers. There are several kinds of means (or "measures of central tendency") in mathematics, especially in statist ...
of the horizontal Eulerian flow-velocity ''Ū''E at any location below trough
Trough may refer to:
In science
* Trough (geology), a long depression less steep than a trench
* Trough (meteorology), an elongated region of low atmospheric pressure
* Trough (physics), the lowest point on a wave
* Trough level (medicine), the l ...
level equal to zero. Due to the irrotationality of potential flow, together with the horizontal sea bed and periodicity the mean horizontal velocity, the mean horizontal velocity is a constant between bed and trough level. So in Stokes first definition the wave is considered from a frame of reference
In physics and astronomy, a frame of reference (or reference frame) is an abstract coordinate system, whose origin (mathematics), origin, orientation (geometry), orientation, and scale (geometry), scale have been specified in physical space. It ...
moving with the mean horizontal velocity ''Ū''E. This is an advantageous approach when the mean Eulerian flow velocity ''Ū''E is known, e.g. from measurements.
#Stokes's second definition of wave celerity is for a frame of reference where the mean horizontal mass transport of the wave motion equal to zero. This is different from the first definition due to the mass transport in the splash zone, i.e. between the trough and crest level, in the wave propagation direction. This wave-induced mass transport is caused by the positive correlation
In statistics, correlation or dependence is any statistical relationship, whether causal or not, between two random variables or bivariate data. Although in the broadest sense, "correlation" may indicate any type of association, in statistics ...
between surface elevation and horizontal velocity. In the reference frame for Stokes's second definition, the wave-induced mass transport is compensated by an opposing undertow (so ''Ū''E < 0 for waves propagating in the positive ''x''-direction). This is the logical definition for waves generated in a wave flume in the laboratory, or waves moving perpendicular towards a beach.
As pointed out by Michael E. McIntyre, the mean horizontal mass transport will be (near) zero for a wave group
In physics, a wave packet (also known as a wave train or wave group) is a short burst of localized wave action that travels as a unit, outlined by an envelope. A wave packet can be analyzed into, or can be synthesized from, a potentially-infini ...
approaching into still water, with also in deep water the mass transport caused by the waves balanced by an opposite mass transport in a return flow (undertow). This is due to the fact that otherwise a large mean force will be needed to accelerate the body of water into which the wave group is propagating.
Notes
References
By Sir George Gabriel Stokes
*:Reprinted in: *
Other historical references
* * * * *:Reprinted in: *
More recent (since 1960)
* * * *: And in (including corrections): : * * * * * * * and
:
External links
* {{physical oceanography Fluid dynamics Water waves Articles containing video clips