Regular Star 4-polytope on:
[Wikipedia]
[Google]
[Amazon]
 In
In
 The Schläfli–Hess 4-polytopes are the complete set of 10
The Schläfli–Hess 4-polytopes are the complete set of 10
Conway (abbrev.) ! Orthogonal
projection ! Schläfli
Coxeter ! C
{p, q} ! F
{p} ! E
{r} ! V
{q, r} ! Dens. ! χ , - align=center BGCOLOR="#e0e0ff" ,
polyicosahedron (pI) , , {3,5,5/2}
, {3,5,5/2}
, 120
{3,5}
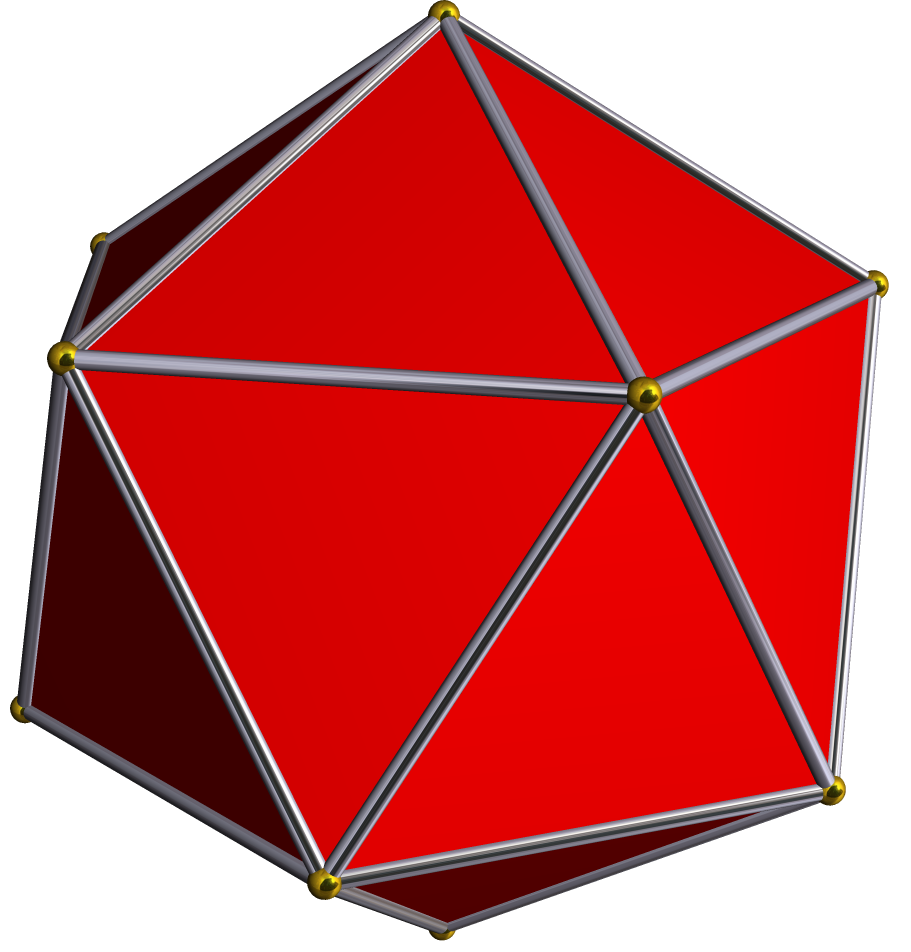 , 1200
, 1200
{3}
 , 720
, 720
{5/2}
 , 120
, 120
{5,5/2}
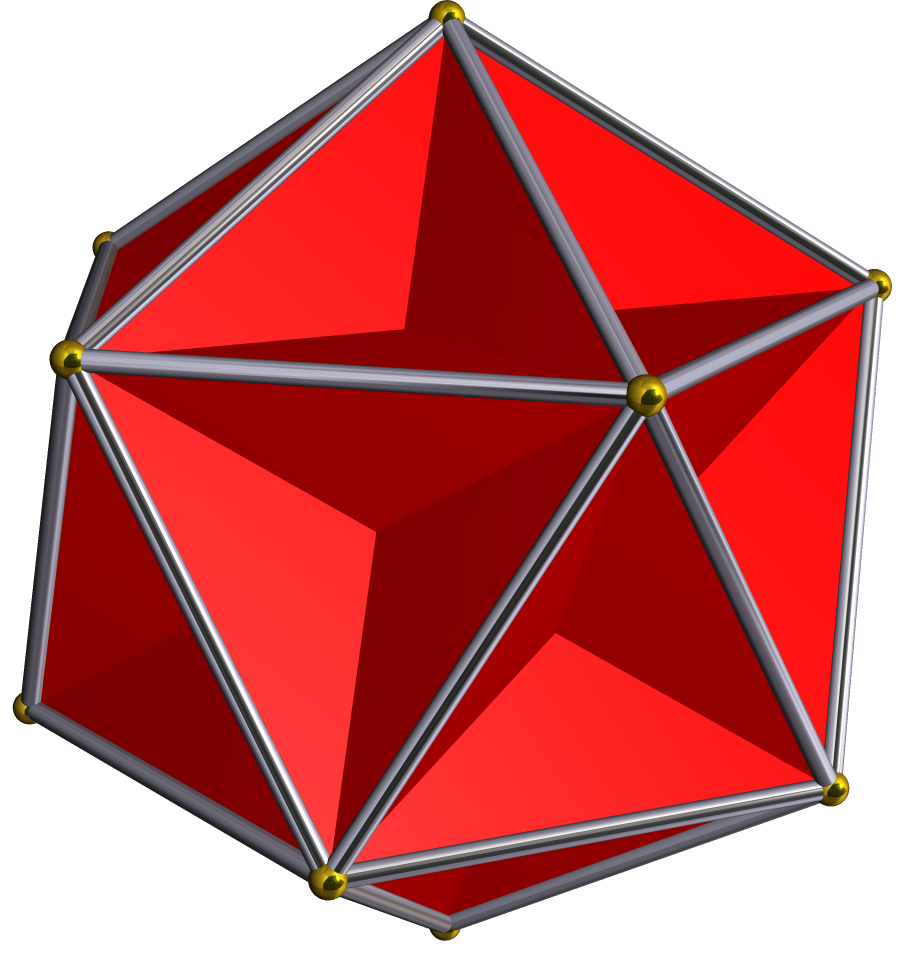 , 4
, 480
, - align=center BGCOLOR="#ffe0e0"
, Small stellated 120-cell
, 4
, 480
, - align=center BGCOLOR="#ffe0e0"
, Small stellated 120-cell
stellated polydodecahedron (spD) , , {5/2,5,3}
, {5/2,5,3}
, 120
{5/2,5}
 , 720
, 720
{5/2}
 , 1200
, 1200
{3}
 , 120
, 120
{5,3}
 , 4
, −480
, - align=center BGCOLOR="#e0ffe0"
,
, 4
, −480
, - align=center BGCOLOR="#e0ffe0"
,
great polydodecahedron (gpD) ,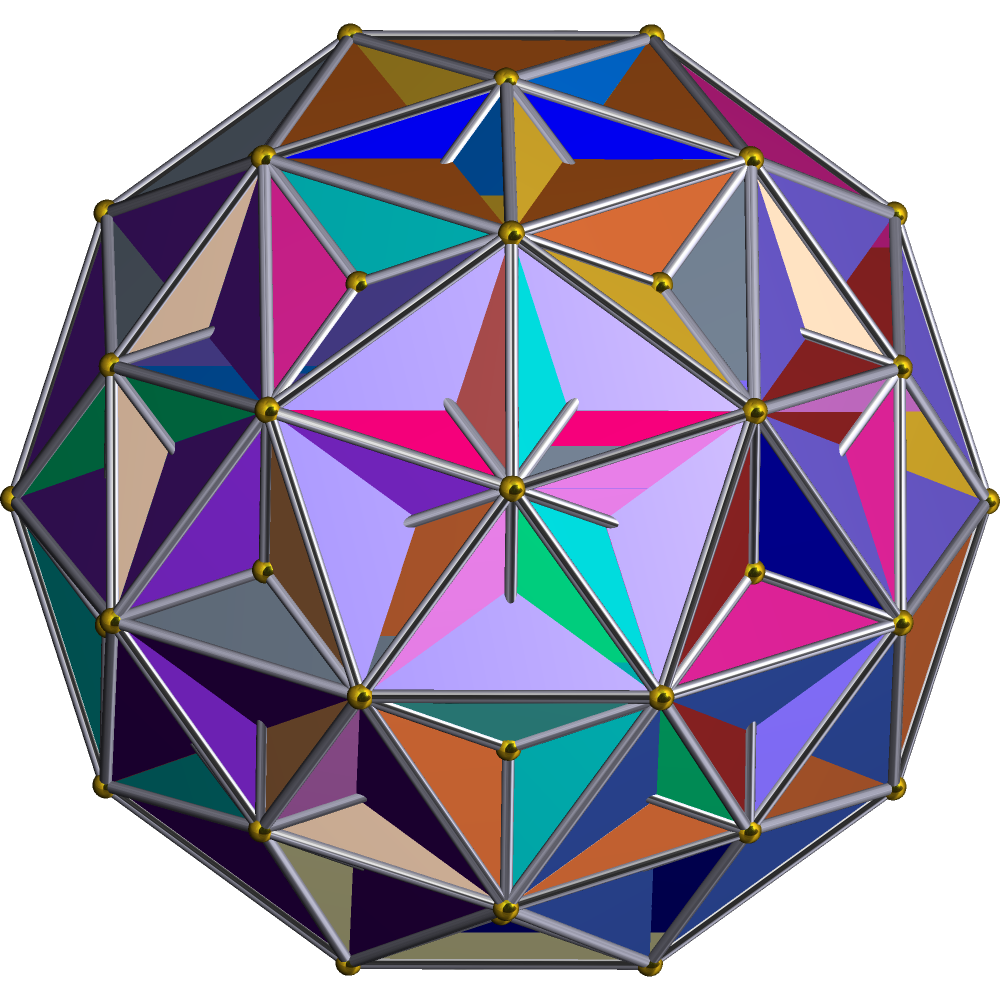 , {5,5/2,5}
, {5,5/2,5}
, 120
{5,5/2}
 , 720
, 720
{5}
 , 720
, 720
{5}
 , 120
, 120
{5/2,5}
 , 6
, 0
, - align=center BGCOLOR="#e0e0ff"
,
, 6
, 0
, - align=center BGCOLOR="#e0e0ff"
,
grand polydodecahedron (apD) , , {5,3,5/2}
, {5,3,5/2}
, 120
{5,3}
 , 720
, 720
{5}
 , 720
, 720
{5/2}
 , 120
, 120
{3,5/2}
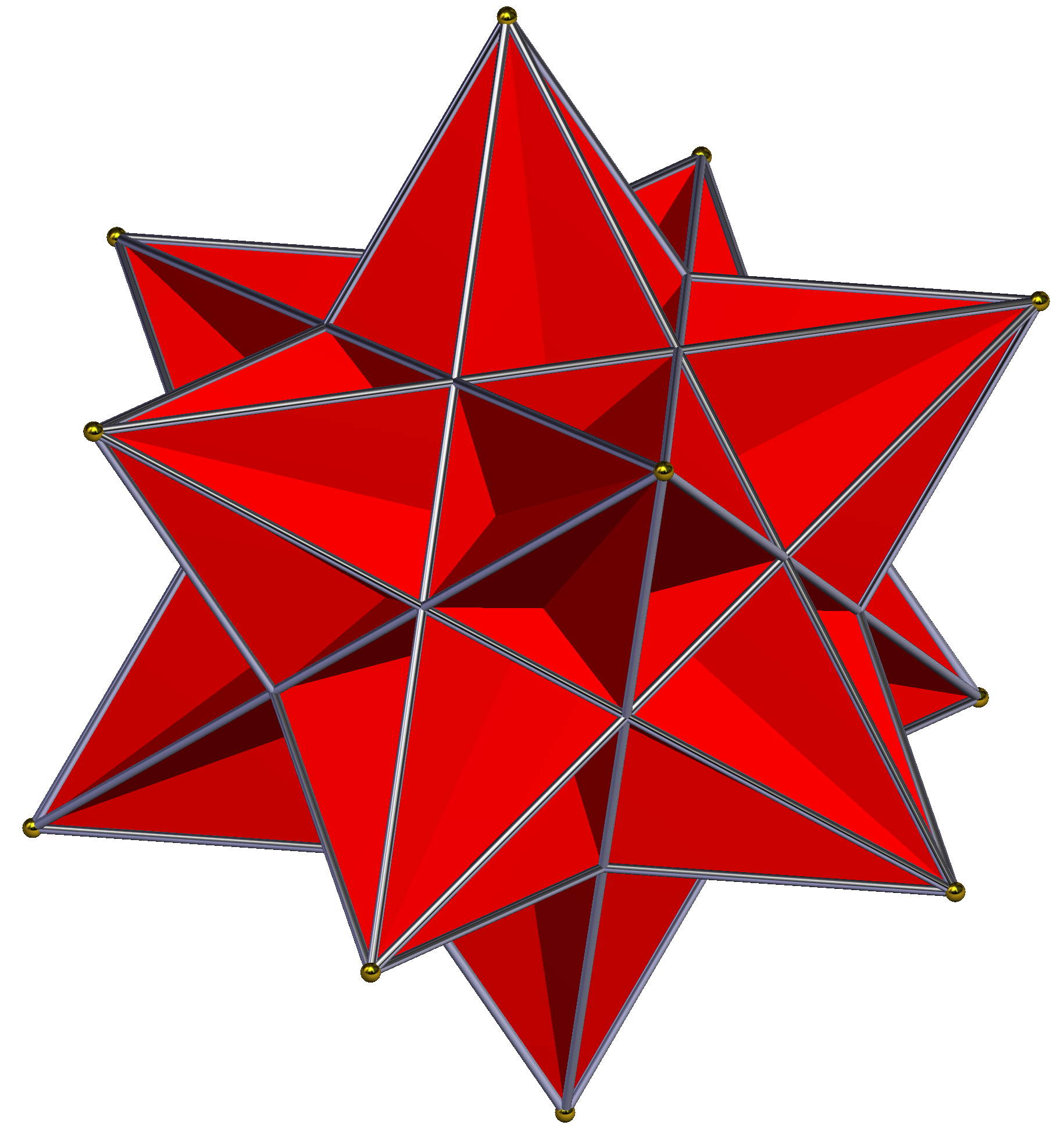 , 20
, 0
, - align=center BGCOLOR="#ffe0e0"
, Great stellated 120-cell
, 20
, 0
, - align=center BGCOLOR="#ffe0e0"
, Great stellated 120-cell
great stellated polydodecahedron (gspD) , , {5/2,3,5}
, {5/2,3,5}
, 120
{5/2,3}
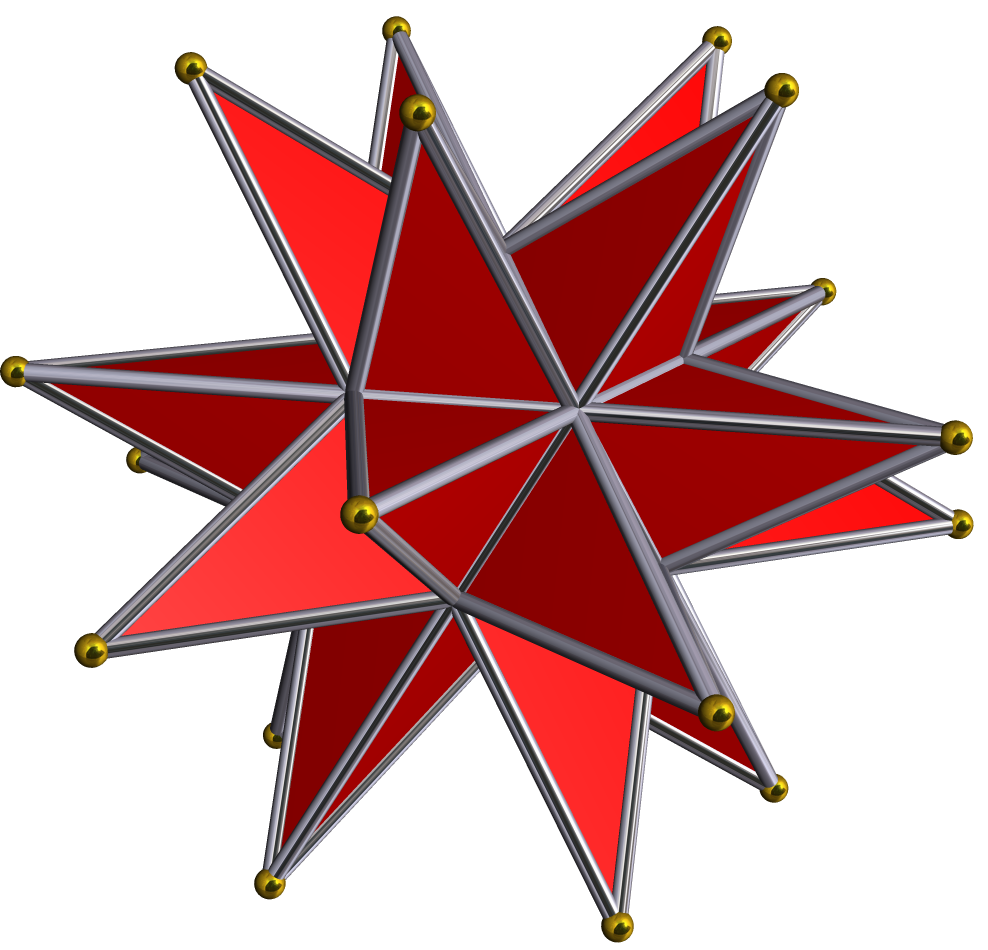 , 720
, 720
{5/2}
 , 720
, 720
{5}
 , 120
, 120
{3,5}
 , 20
, 0
, - align=center BGCOLOR="#e0ffe0"
, Grand stellated 120-cell
, 20
, 0
, - align=center BGCOLOR="#e0ffe0"
, Grand stellated 120-cell
grand stellated polydodecahedron (aspD) , , {5/2,5,5/2}
, {5/2,5,5/2}
, 120
{5/2,5}
 , 720
, 720
{5/2}
 , 720
, 720
{5/2}
 , 120
, 120
{5,5/2}
 , 66
, 0
, - align=center BGCOLOR="#e0e0ff"
,
, 66
, 0
, - align=center BGCOLOR="#e0e0ff"
,
great grand polydodecahedron (gapD) , , {5,5/2,3}
, {5,5/2,3}
, 120
{5,5/2}
 , 720
, 720
{5}
 , 1200
, 1200
{3}
 , 120
, 120
{5/2,3}
 , 76
, −480
, - align=center BGCOLOR="#ffe0e0"
, Great icosahedral 120-cell
, 76
, −480
, - align=center BGCOLOR="#ffe0e0"
, Great icosahedral 120-cell
great polyicosahedron (gpI) ,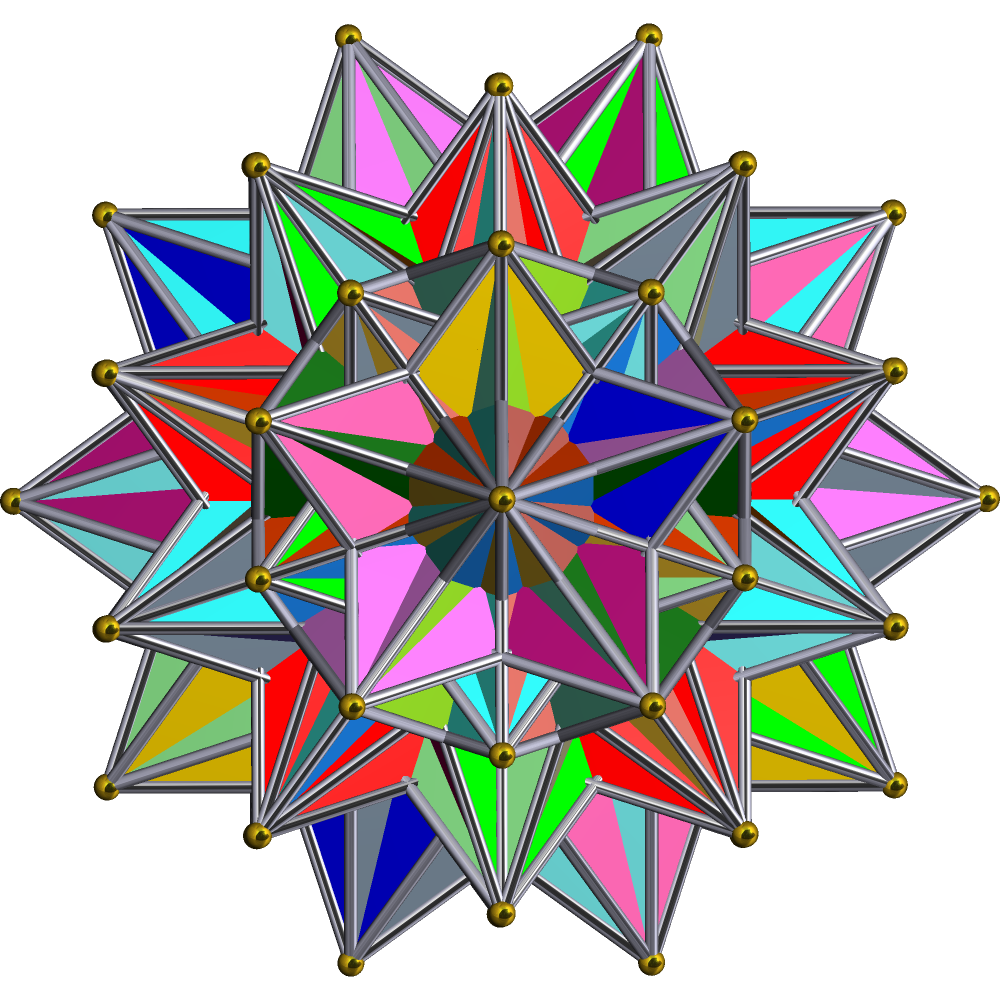 , {3,5/2,5}
, {3,5/2,5}
, 120
{3,5/2}
 , 1200
, 1200
{3}
 , 720
, 720
{5}
 , 120
, 120
{5/2,5}
 , 76
, 480
, - align=center BGCOLOR="#e0e0ff"
, Grand 600-cell
, 76
, 480
, - align=center BGCOLOR="#e0e0ff"
, Grand 600-cell
grand polytetrahedron (apT) , , {3,3,5/2}
, {3,3,5/2}
, 600
{3,3}
 , 1200
, 1200
{3}
 , 720
, 720
{5/2}
 , 120
, 120
{3,5/2}
 , 191
, 0
, - align=center BGCOLOR="#ffe0e0"
, Great grand stellated 120-cell
, 191
, 0
, - align=center BGCOLOR="#ffe0e0"
, Great grand stellated 120-cell
great grand stellated polydodecahedron (gaspD) ,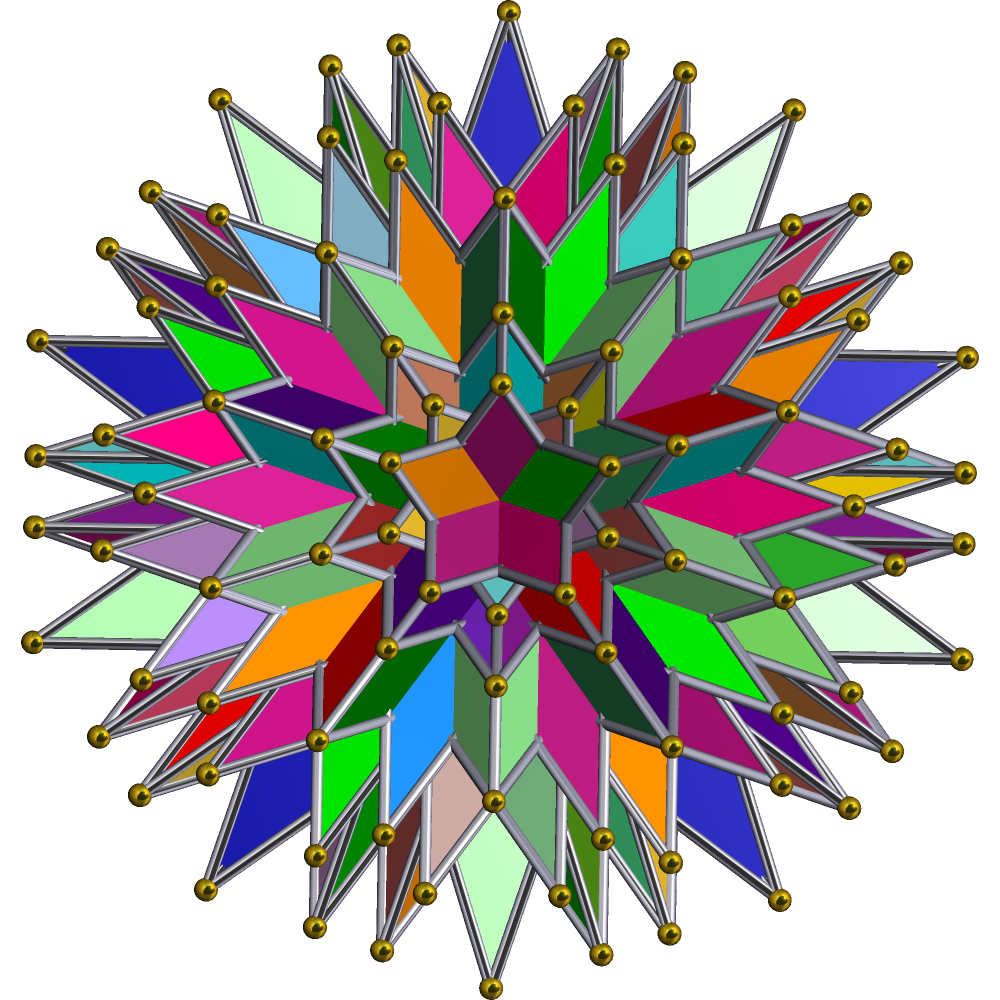 , {5/2,3,3}
, {5/2,3,3}
, 120
{5/2,3}
 , 720
, 720
{5/2}
 , 1200
, 1200
{3}
 , 600
, 600
{3,3}
 , 191
, 0
, 191
, 0
Jonathan Bowers, 16 regular 4-polytopesRegular 4D Polytope Foldouts
A collection of stereographic projections of 4-polytopes.
A Catalog of Uniform PolytopesDimensions
2 hour film about the fourth dimension (contains stereographic projections of all regular 4-polytopes)
Reguläre Polytope
{{DEFAULTSORT:Regular 4-polytope 4-polytopes
mathematics
Mathematics is an area of knowledge that includes the topics of numbers, formulas and related structures, shapes and the spaces in which they are contained, and quantities and their changes. These topics are represented in modern mathematics ...
, a regular 4-polytope is a regular
The term regular can mean normal or in accordance with rules. It may refer to:
People
* Moses Regular (born 1971), America football player
Arts, entertainment, and media Music
* "Regular" (Badfinger song)
* Regular tunings of stringed instrum ...
four-dimensional polytope. They are the four-dimensional analogues of the regular polyhedra in three dimensions and the regular polygons in two dimensions.
There are six convex and ten star
A star is an astronomical object comprising a luminous spheroid of plasma (physics), plasma held together by its gravity. The List of nearest stars and brown dwarfs, nearest star to Earth is the Sun. Many other stars are visible to the naked ...
regular 4-polytopes, giving a total of sixteen.
History
The convex regular 4-polytopes were first described by the Swiss mathematician Ludwig Schläfli in the mid-19th century. He discovered that there are precisely six such figures. Schläfli also found four of the regular star 4-polytopes: thegrand 120-cell
In geometry, the grand 120-cell or grand polydodecahedron is a regular star 4-polytope with Schläfli symbol . It is one of 10 regular Schläfli-Hess polytopes.
It is one of four ''regular star 4-polytopes'' discovered by Ludwig Schläfli. It is n ...
, great stellated 120-cell, grand 600-cell, and great grand stellated 120-cell. He skipped the remaining six because he would not allow forms that failed the Euler characteristic
In mathematics, and more specifically in algebraic topology and polyhedral combinatorics, the Euler characteristic (or Euler number, or Euler–Poincaré characteristic) is a topological invariant, a number that describes a topological space ...
on cells or vertex figures (for zero-hole tori: ''F'' − ''E'' + ''V'' 2). That excludes cells and vertex figures such as the great dodecahedron and small stellated dodecahedron .
Edmund Hess (1843–1903) published the complete list in his 1883 German book ''Einleitung in die Lehre von der Kugelteilung mit besonderer Berücksichtigung ihrer Anwendung auf die Theorie der Gleichflächigen und der gleicheckigen Polyeder''.
Construction
The existence of a regular 4-polytope is constrained by the existence of the regular polyhedra which form its cells and a dihedral angle constraint : to ensure that the cells meet to form a closed 3-surface. The six convex and ten star polytopes described are the only solutions to these constraints. There are four nonconvexSchläfli symbol
In geometry, the Schläfli symbol is a notation of the form \ that defines regular polytopes and tessellations.
The Schläfli symbol is named after the 19th-century Swiss mathematician Ludwig Schläfli, who generalized Euclidean geometry to more ...
s that have valid cells and vertex figures , and pass the dihedral test, but fail to produce finite figures: , , , .
Regular convex 4-polytopes
The regular convex 4-polytopes are the four-dimensional analogues of the Platonic solids in three dimensions and the convex regular polygons in two dimensions. Five of the six are clearly analogues of the five corresponding Platonic solids. The sixth, the24-cell
In geometry, the 24-cell is the convex regular 4-polytope (four-dimensional analogue of a Platonic solid) with Schläfli symbol . It is also called C24, or the icositetrachoron, octaplex (short for "octahedral complex"), icosatetrahedroid, oct ...
, has no regular analogue in three dimensions. However, there exists a pair of irregular solids, the cuboctahedron and its dual the rhombic dodecahedron, which are partial analogues to the 24-cell (in complementary ways). Together they can be seen as the three-dimensional analogue of the 24-cell.
Each convex regular 4-polytope is bounded by a set of 3-dimensional ''cells
Cell most often refers to:
* Cell (biology), the functional basic unit of life
Cell may also refer to:
Locations
* Monastic cell, a small room, hut, or cave in which a religious recluse lives, alternatively the small precursor of a monastery w ...
'' which are all Platonic solids of the same type and size. These are fitted together along their respective faces (face-to-face) in a regular fashion.
Properties
Like their 3-dimensional analogues, the convex regular 4-polytopes can be naturally ordered by size as a measure of 4-dimensional content (hypervolume) for the same radius. Each greater polytope in the sequence is ''rounder'' than its predecessor, enclosing more content within the same radius. The 4-simplex (5-cell) is the limit smallest case, and the 120-cell is the largest. Complexity (as measured by comparing configuration matrices or simply the number of vertices) follows the same ordering. The following table lists some properties of the six convex regular 4-polytopes. The symmetry groups of these 4-polytopes are all Coxeter groups and given in the notation described in that article. The number following the name of the group is theorder
Order, ORDER or Orders may refer to:
* Categorization, the process in which ideas and objects are recognized, differentiated, and understood
* Heterarchy, a system of organization wherein the elements have the potential to be ranked a number of d ...
of the group.
John Conway advocated the names simplex, orthoplex, tesseract, octaplex or polyoctahedron (pO), tetraplex or polytetrahedron (pT), and dodecaplex or polydodecahedron (pD).
Norman Johnson advocated the names n-cell, or pentachoron, hexadecachoron, tesseract or octachoron, icositetrachoron, hexacosichoron, and hecatonicosachoron (or dodecacontachoron), coining the term ''polychoron'' being a 4D analogy to the 3D polyhedron, and 2D polygon, expressed from the Greek roots ''poly'' ("many") and ''choros'' ("room" or "space").
The Euler characteristic
In mathematics, and more specifically in algebraic topology and polyhedral combinatorics, the Euler characteristic (or Euler number, or Euler–Poincaré characteristic) is a topological invariant, a number that describes a topological space ...
for all 4-polytopes is zero, we have the 4-dimensional analogue of Euler's polyhedral formula:
:
where ''N''''k'' denotes the number of ''k''-faces in the polytope (a vertex is a 0-face, an edge is a 1-face, etc.).
The topology of any given 4-polytope is defined by its Betti numbers and torsion coefficients.
As configurations
A regular 4-polytope can be completely described as a configuration matrix containing counts of its component elements. The rows and columns correspond to vertices, edges, faces, and cells. The diagonal numbers (upper left to lower right) say how many of each element occur in the whole 4-polytope. The non-diagonal numbers say how many of the column's element occur in or at the row's element. For example, there are 2 vertices ''in'' each edge (each edge ''has'' 2 vertices), and 2 cells meet ''at'' each face (each face ''belongs to'' 2 cells), in any regular 4-polytope. Notice that the configuration for the dual polytope can be obtained by rotating the matrix by 180 degrees.Visualization
The following table shows some 2-dimensional projections of these 4-polytopes. Various other visualizations can be found in the external links below. The Coxeter-Dynkin diagram graphs are also given below theSchläfli symbol
In geometry, the Schläfli symbol is a notation of the form \ that defines regular polytopes and tessellations.
The Schläfli symbol is named after the 19th-century Swiss mathematician Ludwig Schläfli, who generalized Euclidean geometry to more ...
.
Regular star (Schläfli–Hess) 4-polytopes
 The Schläfli–Hess 4-polytopes are the complete set of 10
The Schläfli–Hess 4-polytopes are the complete set of 10 regular
The term regular can mean normal or in accordance with rules. It may refer to:
People
* Moses Regular (born 1971), America football player
Arts, entertainment, and media Music
* "Regular" (Badfinger song)
* Regular tunings of stringed instrum ...
self-intersecting star polychora ( four-dimensional polytopes).Coxeter, ''Star polytopes and the Schläfli function f{α,β,γ)'' p. 122 2. ''The Schläfli-Hess polytopes'' They are named in honor of their discoverers: Ludwig Schläfli and Edmund Hess. Each is represented by a Schläfli symbol
In geometry, the Schläfli symbol is a notation of the form \ that defines regular polytopes and tessellations.
The Schläfli symbol is named after the 19th-century Swiss mathematician Ludwig Schläfli, who generalized Euclidean geometry to more ...
{''p'',''q'',''r''} in which one of the numbers is . They are thus analogous to the regular nonconvex Kepler–Poinsot polyhedra, which are in turn analogous to the pentagram.
Names
Their names given here were given by John Conway, extending Cayley's names for the Kepler–Poinsot polyhedra: along with ''stellated'' and ''great'', he adds a ''grand'' modifier. Conway offered these operational definitions: #stellation
In geometry, stellation is the process of extending a polygon in two dimensions, polyhedron in three dimensions, or, in general, a polytope in ''n'' dimensions to form a new figure. Starting with an original figure, the process extends specific el ...
– replaces edges by longer edges in same lines. (Example: a pentagon
In geometry, a pentagon (from the Greek πέντε ''pente'' meaning ''five'' and γωνία ''gonia'' meaning ''angle'') is any five-sided polygon or 5-gon. The sum of the internal angles in a simple pentagon is 540°.
A pentagon may be simpl ...
stellates into a pentagram)
#greatening – replaces the faces by large ones in same planes. (Example: an icosahedron
In geometry, an icosahedron ( or ) is a polyhedron with 20 faces. The name comes and . The plural can be either "icosahedra" () or "icosahedrons".
There are infinitely many non- similar shapes of icosahedra, some of them being more symmetrica ...
greatens into a great icosahedron)
#aggrandizement – replaces the cells by large ones in same 3-spaces. (Example: a 600-cell aggrandizes into a grand 600-cell)
John Conway names the 10 forms from 3 regular celled 4-polytopes: pT=polytetrahedron {3,3,5} (a tetrahedral 600-cell), pI=polyicoshedron {3,5,} (an icosahedral 120-cell
In geometry, the icosahedral 120-cell, polyicosahedron, faceting, faceted 600-cell or icosaplex is a regular star 4-polytope with Schläfli symbol . It is one of 10 regular Schläfli-Hess polytopes.
It is constructed by 5 icosahedron, icosahedra a ...
), and pD=polydodecahedron {5,3,3} (a dodecahedral 120-cell
In geometry, the 120-cell is the convex regular 4-polytope (four-dimensional analogue of a Platonic solid) with Schläfli symbol . It is also called a C120, dodecaplex (short for "dodecahedral complex"), hyperdodecahedron, polydodecahedron, heca ...
), with prefix modifiers: ''g'', ''a'', and ''s'' for great, (ag)grand, and stellated. The final stellation, the ''great grand stellated polydodecahedron'' contains them all as ''gaspD''.
Symmetry
All ten polychora have ,3,5( H4) hexacosichoric symmetry. They are generated from 6 related Goursat tetrahedra rational-order symmetry groups: ,5,5/2 ,5/2,5 ,3,5/2 /2,5,5/2 ,5/2,3 and ,3,5/2 Each group has 2 regular star-polychora, except for two groups which are self-dual, having only one. So there are 4 dual-pairs and 2 self-dual forms among the ten regular star polychora.Properties
Note: * There are 2 unique vertex arrangements, matching those of the120-cell
In geometry, the 120-cell is the convex regular 4-polytope (four-dimensional analogue of a Platonic solid) with Schläfli symbol . It is also called a C120, dodecaplex (short for "dodecahedral complex"), hyperdodecahedron, polydodecahedron, heca ...
and 600-cell.
* There are 4 unique edge arrangements, which are shown as ''wireframes'' orthographic projections
Orthographic projection (also orthogonal projection and analemma) is a means of representing three-dimensional objects in two dimensions. Orthographic projection is a form of parallel projection in which all the projection lines are orthogonal t ...
.
* There are 7 unique face arrangements, shown as ''solids'' (face-colored) orthographic projections.
The cells (polyhedra), their faces (polygons), the ''polygonal edge figures'' and ''polyhedral vertex figures'' are identified by their Schläfli symbol
In geometry, the Schläfli symbol is a notation of the form \ that defines regular polytopes and tessellations.
The Schläfli symbol is named after the 19th-century Swiss mathematician Ludwig Schläfli, who generalized Euclidean geometry to more ...
s.
{, class="wikitable sortable"
! NameConway (abbrev.) ! Orthogonal
projection ! Schläfli
Coxeter ! C
{p, q} ! F
{p} ! E
{r} ! V
{q, r} ! Dens. ! χ , - align=center BGCOLOR="#e0e0ff" ,
Icosahedral 120-cell
In geometry, the icosahedral 120-cell, polyicosahedron, faceting, faceted 600-cell or icosaplex is a regular star 4-polytope with Schläfli symbol . It is one of 10 regular Schläfli-Hess polytopes.
It is constructed by 5 icosahedron, icosahedra a ...
polyicosahedron (pI) ,
 , {3,5,5/2}
, {3,5,5/2}, 120
{3,5}
 , 1200
, 1200{3}
{5/2}
{5,5/2}
 , 4
, 480
, - align=center BGCOLOR="#ffe0e0"
, Small stellated 120-cell
, 4
, 480
, - align=center BGCOLOR="#ffe0e0"
, Small stellated 120-cellstellated polydodecahedron (spD) ,
 , {5/2,5,3}
, {5/2,5,3}, 120
{5/2,5}
 , 720
, 720{5/2}
{3}
{5,3}
 , 4
, −480
, - align=center BGCOLOR="#e0ffe0"
,
, 4
, −480
, - align=center BGCOLOR="#e0ffe0"
, Great 120-cell
In geometry, the great 120-cell or great polydodecahedron is a regular star 4-polytope with Schläfli symbol . It is one of 10 regular Schläfli-Hess polytopes. It is one of the two such polytopes that is self-dual.
Related polytopes
It has ...
great polydodecahedron (gpD) ,
 , {5,5/2,5}
, {5,5/2,5}, 120
{5,5/2}
 , 720
, 720{5}
{5}
{5/2,5}
 , 6
, 0
, - align=center BGCOLOR="#e0e0ff"
,
, 6
, 0
, - align=center BGCOLOR="#e0e0ff"
, Grand 120-cell
In geometry, the grand 120-cell or grand polydodecahedron is a regular star 4-polytope with Schläfli symbol . It is one of 10 regular Schläfli-Hess polytopes.
It is one of four ''regular star 4-polytopes'' discovered by Ludwig Schläfli. It is n ...
grand polydodecahedron (apD) ,
 , {5,3,5/2}
, {5,3,5/2}, 120
{5,3}
 , 720
, 720{5}
{5/2}
{3,5/2}
 , 20
, 0
, - align=center BGCOLOR="#ffe0e0"
, Great stellated 120-cell
, 20
, 0
, - align=center BGCOLOR="#ffe0e0"
, Great stellated 120-cellgreat stellated polydodecahedron (gspD) ,
 , {5/2,3,5}
, {5/2,3,5}, 120
{5/2,3}
 , 720
, 720{5/2}
{5}
{3,5}
 , 20
, 0
, - align=center BGCOLOR="#e0ffe0"
, Grand stellated 120-cell
, 20
, 0
, - align=center BGCOLOR="#e0ffe0"
, Grand stellated 120-cellgrand stellated polydodecahedron (aspD) ,
 , {5/2,5,5/2}
, {5/2,5,5/2}, 120
{5/2,5}
 , 720
, 720{5/2}
{5/2}
{5,5/2}
 , 66
, 0
, - align=center BGCOLOR="#e0e0ff"
,
, 66
, 0
, - align=center BGCOLOR="#e0e0ff"
, Great grand 120-cell
In geometry, the great grand 120-cell or great grand polydodecahedron is a regular star 4-polytope with Schläfli symbol . It is one of 10 regular Schläfli-Hess polytopes.
Related polytopes
It has the same edge arrangement as the small stella ...
great grand polydodecahedron (gapD) ,
 , {5,5/2,3}
, {5,5/2,3}, 120
{5,5/2}
 , 720
, 720{5}
{3}
{5/2,3}
 , 76
, −480
, - align=center BGCOLOR="#ffe0e0"
, Great icosahedral 120-cell
, 76
, −480
, - align=center BGCOLOR="#ffe0e0"
, Great icosahedral 120-cellgreat polyicosahedron (gpI) ,
 , {3,5/2,5}
, {3,5/2,5}, 120
{3,5/2}
 , 1200
, 1200{3}
{5}
{5/2,5}
 , 76
, 480
, - align=center BGCOLOR="#e0e0ff"
, Grand 600-cell
, 76
, 480
, - align=center BGCOLOR="#e0e0ff"
, Grand 600-cellgrand polytetrahedron (apT) ,
 , {3,3,5/2}
, {3,3,5/2}, 600
{3,3}
 , 1200
, 1200{3}
{5/2}
{3,5/2}
 , 191
, 0
, - align=center BGCOLOR="#ffe0e0"
, Great grand stellated 120-cell
, 191
, 0
, - align=center BGCOLOR="#ffe0e0"
, Great grand stellated 120-cellgreat grand stellated polydodecahedron (gaspD) ,
 , {5/2,3,3}
, {5/2,3,3}, 120
{5/2,3}
 , 720
, 720{5/2}
{3}
{3,3}
 , 191
, 0
, 191
, 0
See also
* Regular polytope *List of regular polytopes
This article lists the regular polytopes and regular polytope compounds in Euclidean geometry, Euclidean, spherical geometry, spherical and hyperbolic geometry, hyperbolic spaces.
The Schläfli symbol describes every regular tessellation of an ' ...
* Infinite regular 4-polytopes:
** One regular Euclidean honeycomb: {4,3,4}
** Four compact regular hyperbolic honeycombs: {3,5,3}, {4,3,5}, {5,3,4}, {5,3,5}
** Eleven paracompact regular hyperbolic honeycombs: {3,3,6}, {6,3,3}, {3,4,4}, {4,4,3}, {3,6,3}, {4,3,6}, {6,3,4}, {4,4,4}, {5,3,6}, {6,3,5}, and {6,3,6}.
*Abstract
Abstract may refer to:
* ''Abstract'' (album), 1962 album by Joe Harriott
* Abstract of title a summary of the documents affecting title to parcel of land
* Abstract (law), a summary of a legal document
* Abstract (summary), in academic publishi ...
regular 4-polytopes:
** 11-cell
In mathematics, the 11-cell (or hendecachoron) is a self-dual abstract regular 4-polytope ( four-dimensional polytope). Its 11 cells are hemi-icosahedral. It has 11 vertices, 55 edges and 55 faces. It has Schläfli symbol , with 3 hemi-icosahedr ...
{3,5,3}
** 57-cell
In mathematics, the 57-cell (pentacontakaiheptachoron) is a self-dual abstract regular 4-polytope ( four-dimensional polytope). Its 57 cells are hemi-dodecahedra. It also has 57 vertices, 171 edges and 171 two-dimensional faces.
The symmetry or ...
{5,3,5}
* Uniform 4-polytope uniform 4-polytope families constructed from these 6 regular forms.
* Platonic solid
* Kepler-Poinsot polyhedra — regular star polyhedron
* Star polygon
In geometry, a star polygon is a type of non-convex polygon. Regular star polygons have been studied in depth; while star polygons in general appear not to have been formally defined, certain notable ones can arise through truncation operations ...
— regular star polygons
* 4-polytope
In geometry, a 4-polytope (sometimes also called a polychoron, polycell, or polyhedroid) is a four-dimensional polytope. It is a connected and closed figure, composed of lower-dimensional polytopal elements: vertices, edges, faces (polygons), an ...
* 5-polytope
In geometry, a five-dimensional polytope (or 5-polytope) is a polytope in five-dimensional space, bounded by (4-polytope) facets, pairs of which share a polyhedral cell.
Definition
A 5-polytope is a closed five-dimensional figure with vertices ...
* 6-polytope
In six-dimensional geometry, a six-dimensional polytope or 6-polytope is a polytope, bounded by 5-polytope facets.
Definition
A 6-polytope is a closed six-dimensional figure with vertices, edges, faces, cells (3-faces), 4-faces, and 5-faces. A ...
References
Citations
Bibliography
* * * * * * * ** (Paper 10) * *External links
*Jonathan Bowers, 16 regular 4-polytopes
A collection of stereographic projections of 4-polytopes.
A Catalog of Uniform Polytopes
2 hour film about the fourth dimension (contains stereographic projections of all regular 4-polytopes)
Reguläre Polytope
{{DEFAULTSORT:Regular 4-polytope 4-polytopes