|
Complex Quadratic Polynomial
A complex quadratic polynomial is a quadratic polynomial whose coefficients and variable are complex numbers. Properties Quadratic polynomials have the following properties, regardless of the form: *It is a unicritical polynomial, i.e. it has one finite critical point in the complex plane, Dynamical plane consist of maximally 2 basins: basin of infinity and basin of finite critical point ( if finite critical point do not escapes) *It can be postcritically finite, i.e. the orbit of the critical point can be finite, because the critical point is periodic or preperiodic. * It is a unimodal function, * It is a rational function, * It is an entire function. Forms When the quadratic polynomial has only one variable (univariate), one can distinguish its four main forms: * The general form: f(x) = a_2 x^2 + a_1 x + a_0 where a_2 \ne 0 * The factored form used for the logistic map: f_r(x) = r x (1-x) * f_(x) = x^2 +\lambda x which has an indifferent fixed point with multiplier ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Complex Quadratic Polynomial
A complex quadratic polynomial is a quadratic polynomial whose coefficients and variable are complex numbers. Properties Quadratic polynomials have the following properties, regardless of the form: *It is a unicritical polynomial, i.e. it has one finite critical point in the complex plane, Dynamical plane consist of maximally 2 basins: basin of infinity and basin of finite critical point ( if finite critical point do not escapes) *It can be postcritically finite, i.e. the orbit of the critical point can be finite, because the critical point is periodic or preperiodic. * It is a unimodal function, * It is a rational function, * It is an entire function. Forms When the quadratic polynomial has only one variable (univariate), one can distinguish its four main forms: * The general form: f(x) = a_2 x^2 + a_1 x + a_0 where a_2 \ne 0 * The factored form used for the logistic map: f_r(x) = r x (1-x) * f_(x) = x^2 +\lambda x which has an indifferent fixed point with multiplier ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Periodic Points Of Complex Quadratic Mappings
This article describes periodic points of some complex quadratic maps. A map is a formula for computing a value of a variable based on its own previous value or values; a quadratic map is one that involves the previous value raised to the powers one and two; and a complex map is one in which the variable and the parameters are complex numbers. A periodic point of a map is a value of the variable that occurs repeatedly after intervals of a fixed length. These periodic points play a role in the theories of Fatou and Julia sets. Definitions Let :f_c(z) = z^2+c\, be the complex quadric mapping, where z and c are complex numbers. Notationally, f^ _c (z) is the k-fold composition of f_c with itself (not to be confused with the kth derivative of f_c)—that is, the value after the ''k''-th iteration of the function f _c. Thus :f^ _c (z) = f_c(f^ _c (z)). Periodic points of a complex quadratic mapping of period p are points z of the dynamical plane such that :f^ _c (z) = z, ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Quadratic Polynomial
In mathematics, a quadratic polynomial is a polynomial of degree two in one or more variables. A quadratic function is the polynomial function defined by a quadratic polynomial. Before 20th century, the distinction was unclear between a polynomial and its associated polynomial function; so "quadratic polynomial" and "quadratic function" were almost synonymous. This is still the case in many elementary courses, where both terms are often abbreviated as "quadratic". For example, a univariate (single-variable) quadratic function has the form :f(x)=ax^2+bx+c,\quad a \ne 0, where is its variable. The graph of a univariate quadratic function is a parabola, a curve that has an axis of symmetry parallel to the -axis. If a quadratic function is equated with zero, then the result is a quadratic equation. The solutions of a quadratic equation are the zeros of the corresponding quadratic function. The bivariate case in terms of variables and has the form : f(x,y) = a x^2 + ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Bodil Branner
Bodil Branner (born 5 February 1943, in Aarhus) is a retired Danish mathematician, one of the founders of European Women in Mathematics and a former chair of the Danish Mathematical Society. Her research concerned holomorphic dynamics and the history of mathematics. Education and career Branner studied mathematics and physics at Aarhus University, where mathematician Svend Bundgaard was one of her mentors, and in 1967 earned a master's degree (the highest degree then available) under the supervision of Leif Kristensen. She had intended to travel to the U.S. for a doctorate, but her husband, a chemist, took an industry job in Copenhagen. Branner could not get a job as a high school teacher because she did not have a teaching qualification, but Bundgaard found her a position as a faculty assistant for Bent Fabricius-Bjerre at the Technical University of Denmark. Despite this not beginning as an actual faculty position, she eventually earned tenure there in the 1970s. She was the fi ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
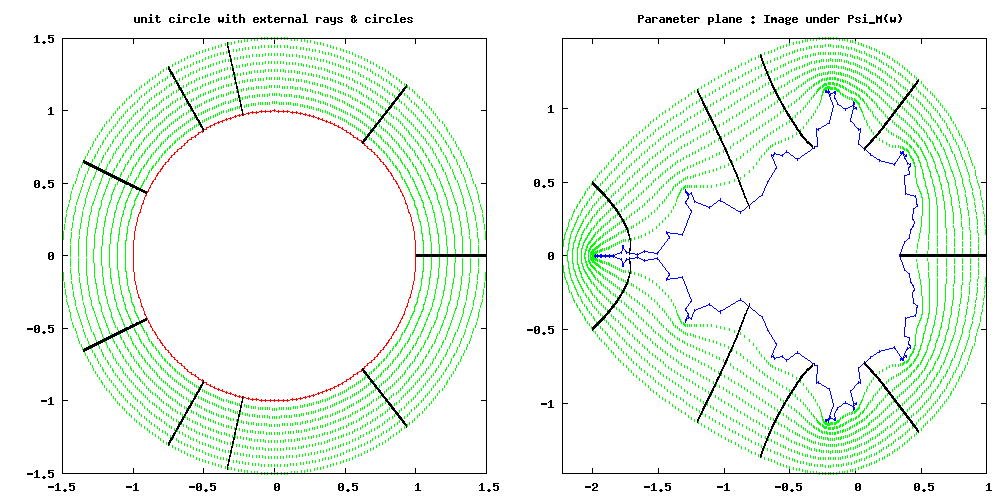
External Ray
An external ray is a curve that runs from infinity toward a Julia or Mandelbrot set. Although this curve is only rarely a half-line (ray) it is called a ray because it is an image of a ray. External rays are used in complex analysis, particularly in complex dynamics and geometric function theory. History External rays were introduced in Douady and Hubbard's study of the Mandelbrot set Types Criteria for classification : * plane : parameter or dynamic * map * bifurcation of dynamic rays * Stretching * landing plane External rays of (connected) Julia sets on dynamical plane are often called dynamic rays. External rays of the Mandelbrot set (and similar one-dimensional connectedness loci) on parameter plane are called parameter rays. bifurcation Dynamic ray can be: * bifurcated = branched = broken * smooth = unbranched = unbroken When the filled Julia set is connected, there are no branching external rays. When the Julia set is not connected then some exte ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
External Ray
An external ray is a curve that runs from infinity toward a Julia or Mandelbrot set. Although this curve is only rarely a half-line (ray) it is called a ray because it is an image of a ray. External rays are used in complex analysis, particularly in complex dynamics and geometric function theory. History External rays were introduced in Douady and Hubbard's study of the Mandelbrot set Types Criteria for classification : * plane : parameter or dynamic * map * bifurcation of dynamic rays * Stretching * landing plane External rays of (connected) Julia sets on dynamical plane are often called dynamic rays. External rays of the Mandelbrot set (and similar one-dimensional connectedness loci) on parameter plane are called parameter rays. bifurcation Dynamic ray can be: * bifurcated = branched = broken * smooth = unbranched = unbroken When the filled Julia set is connected, there are no branching external rays. When the Julia set is not connected then some exte ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Iterated Function
In mathematics, an iterated function is a function (that is, a function from some set to itself) which is obtained by composing another function with itself a certain number of times. The process of repeatedly applying the same function is called iteration. In this process, starting from some initial object, the result of applying a given function is fed again in the function as input, and this process is repeated. For example on the image on the right: :with the circle‑shaped symbol of function composition. Iterated functions are objects of study in computer science, fractals, dynamical systems, mathematics and renormalization group physics. Definition The formal definition of an iterated function on a set ''X'' follows. Let be a set and be a function. Defining as the ''n''-th iterate of (a notation introduced by Hans Heinrich Bürmann and John Frederick William Herschel), where ''n'' is a non-negative integer, by: f^0 ~ \stackrel ~ \operatorna ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Dyadic Transformation
The dyadic transformation (also known as the dyadic map, bit shift map, 2''x'' mod 1 map, Bernoulli map, doubling map or sawtooth map) is the mapping (i.e., recurrence relation) : T: , 1) \to [0, 1)^\infty : x \mapsto (x_0, x_1, x_2, \ldots) (where [0, 1)^\infty is the set of sequences from [0, 1)) produced by the rule : x_0 = x : \text n \ge 0,\ x_ = (2 x_n) \bmod 1. Equivalently, the dyadic transformation can also be defined as the iterated function map of the piecewise linear function : T(x)=\begin2x & 0 \le x < \frac \\2x-1 & \frac \le x < 1. \end The name ''bit shift map'' arises because, if the value of an iterate is written in notation, the next iterate is obtained by shifting the binary point one bit to the right, and if the bit to the left of the new binary point is a "one", replacing it with a zero. The dyadic transfo ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Fatou Set
In the context of complex dynamics, a branch of mathematics, the Julia set and the Fatou set are two complementary sets (Julia "laces" and Fatou "dusts") defined from a function. Informally, the Fatou set of the function consists of values with the property that all nearby values behave similarly under repeated iteration of the function, and the Julia set consists of values such that an arbitrarily small perturbation can cause drastic changes in the sequence of iterated function values. Thus the behavior of the function on the Fatou set is "regular", while on the Julia set its behavior is "chaotic". The Julia set of a function is commonly denoted \operatorname(f), and the Fatou set is denoted \operatorname(f). These sets are named after the French mathematicians Gaston Julia and Pierre Fatou whose work began the study of complex dynamics during the early 20th century. Formal definition Let f(z) be a non-constant holomorphic function from the Riemann sphere ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Julia Set
In the context of complex dynamics, a branch of mathematics, the Julia set and the Fatou set are two complementary sets (Julia "laces" and Fatou "dusts") defined from a function. Informally, the Fatou set of the function consists of values with the property that all nearby values behave similarly under repeated iteration of the function, and the Julia set consists of values such that an arbitrarily small perturbation can cause drastic changes in the sequence of iterated function values. Thus the behavior of the function on the Fatou set is "regular", while on the Julia set its behavior is "chaotic". The Julia set of a function is commonly denoted \operatorname(f), and the Fatou set is denoted \operatorname(f). These sets are named after the French mathematicians Gaston Julia and Pierre Fatou whose work began the study of complex dynamics during the early 20th century. Formal definition Let f(z) be a non-constant holomorphic function from the Riemann sphere ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Mandelbrot Set
The Mandelbrot set () is the set of complex numbers c for which the function f_c(z)=z^2+c does not diverge to infinity when iterated from z=0, i.e., for which the sequence f_c(0), f_c(f_c(0)), etc., remains bounded in absolute value. This set was first defined and drawn by Robert W. Brooks and Peter Matelski in 1978, as part of a study of Kleinian groups. Afterwards, in 1980, Benoit Mandelbrot obtained high-quality visualizations of the set while working at IBM's Thomas J. Watson Research Center in Yorktown Heights, New York. Images of the Mandelbrot set exhibit an elaborate and infinitely complicated boundary that reveals progressively ever-finer recursive detail at increasing magnifications; mathematically, one would say that the boundary of the Mandelbrot set is a ''fractal curve''. The "style" of this recursive detail depends on the region of the set boundary being examined. Mandelbrot set images may be created by sampling the complex numbers and testing, for ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Complex Dynamics
Complex dynamics is the study of dynamical systems defined by iteration of functions on complex number spaces. Complex analytic dynamics is the study of the dynamics of specifically analytic functions. Techniques *General **Montel's theorem **Poincaré metric **Schwarz lemma **Riemann mapping theorem **Carathéodory's theorem (conformal mapping) **Böttcher's equation *Combinatorial ** Hubbard trees ** Spider algorithm ** Tuning **Laminations ** Devil's Staircase algorithm (Cantor function) **Orbit portraits ** Yoccoz puzzles Parts * Holomorphic dynamics (dynamics of holomorphic functions) ** in one complex variable ** in several complex variables * Conformal dynamics unites holomorphic dynamics in one complex variable with differentiable dynamics in one real variable. See also *Arithmetic dynamics *Chaos theory *Complex analysis *Complex quadratic polynomial *Fatou set *Infinite compositions of analytic functions *Julia set *Mandelbrot set *Symbolic dynamics Notes Referen ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |