|
Binary Octahedral Group
In mathematics, the binary octahedral group, name as 2O or Coxeter&Moser: Generators and Relations for discrete groups: : Rl = Sm = Tn = RST is a certain nonabelian group of order 48. It is an extension of the chiral octahedral group ''O'' or (2,3,4) of order 24 by a cyclic group of order 2, and is the preimage of the octahedral group under the 2:1 covering homomorphism \operatorname(3) \to \operatorname(3) of the special orthogonal group by the spin group. It follows that the binary octahedral group is a discrete subgroup of Spin(3) of order 48. The binary octahedral group is most easily described concretely as a discrete subgroup of the unit quaternions, under the isomorphism \operatorname(3) \cong \operatorname(1) where Sp(1) is the multiplicative group of unit quaternions. (For a description of this homomorphism see the article on quaternions and spatial rotations.) Elements Explicitly, the binary octahedral group is given as the union of the 24 Hurwitz units :\ with all 24 ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Mathematics
Mathematics is an area of knowledge that includes the topics of numbers, formulas and related structures, shapes and the spaces in which they are contained, and quantities and their changes. These topics are represented in modern mathematics with the major subdisciplines of number theory, algebra, geometry, and analysis, respectively. There is no general consensus among mathematicians about a common definition for their academic discipline. Most mathematical activity involves the discovery of properties of abstract objects and the use of pure reason to prove them. These objects consist of either abstractions from nature orin modern mathematicsentities that are stipulated to have certain properties, called axioms. A ''proof'' consists of a succession of applications of deductive rules to already established results. These results include previously proved theorems, axioms, andin case of abstraction from naturesome basic properties that are considered true starting points of ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Short Exact Sequence
An exact sequence is a sequence of morphisms between objects (for example, groups, rings, modules, and, more generally, objects of an abelian category) such that the image of one morphism equals the kernel of the next. Definition In the context of group theory, a sequence :G_0\;\xrightarrow\; G_1 \;\xrightarrow\; G_2 \;\xrightarrow\; \cdots \;\xrightarrow\; G_n of groups and group homomorphisms is said to be exact at G_i if \operatorname(f_i)=\ker(f_). The sequence is called exact if it is exact at each G_i for all 1\leq i |
Quotient Group
A quotient group or factor group is a mathematical group obtained by aggregating similar elements of a larger group using an equivalence relation that preserves some of the group structure (the rest of the structure is "factored" out). For example, the cyclic group of addition modulo ''n'' can be obtained from the group of integers under addition by identifying elements that differ by a multiple of n and defining a group structure that operates on each such class (known as a congruence class) as a single entity. It is part of the mathematical field known as group theory. For a congruence relation on a group, the equivalence class of the identity element is always a normal subgroup of the original group, and the other equivalence classes are precisely the cosets of that normal subgroup. The resulting quotient is written G\,/\,N, where G is the original group and N is the normal subgroup. (This is pronounced G\bmod N, where \mbox is short for modulo.) Much of the importance o ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Index (group Theory)
In mathematics, specifically group theory, the index of a subgroup ''H'' in a group ''G'' is the number of left cosets of ''H'' in ''G'', or equivalently, the number of right cosets of ''H'' in ''G''. The index is denoted , G:H, or :H/math> or (G:H). Because ''G'' is the disjoint union of the left cosets and because each left coset has the same size as ''H'', the index is related to the orders of the two groups by the formula :, G, = , G:H, , H, (interpret the quantities as cardinal numbers if some of them are infinite). Thus the index , G:H, measures the "relative sizes" of ''G'' and ''H''. For example, let G = \Z be the group of integers under addition, and let H = 2\Z be the subgroup consisting of the even integers. Then 2\Z has two cosets in \Z, namely the set of even integers and the set of odd integers, so the index , \Z:2\Z, is 2. More generally, , \Z:n\Z, = n for any positive integer ''n''. When ''G'' is finite, the formula may be written as , G:H, = , G, /, ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Normal Subgroup
In abstract algebra, a normal subgroup (also known as an invariant subgroup or self-conjugate subgroup) is a subgroup that is invariant under conjugation by members of the group of which it is a part. In other words, a subgroup N of the group G is normal in G if and only if gng^ \in N for all g \in G and n \in N. The usual notation for this relation is N \triangleleft G. Normal subgroups are important because they (and only they) can be used to construct quotient groups of the given group. Furthermore, the normal subgroups of G are precisely the kernels of group homomorphisms with domain G, which means that they can be used to internally classify those homomorphisms. Évariste Galois was the first to realize the importance of the existence of normal subgroups. Definitions A subgroup N of a group G is called a normal subgroup of G if it is invariant under conjugation; that is, the conjugation of an element of N by an element of G is always in N. The usual notation for this re ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Lipschitz Unit
In mathematics, a Hurwitz quaternion (or Hurwitz integer) is a quaternion whose components are ''either'' all integers ''or'' all half-integers (halves of odd integers; a mixture of integers and half-integers is excluded). The set of all Hurwitz quaternions is :H = \left\. That is, either ''a'', ''b'', ''c'', ''d'' are all integers, or they are all half-integers. ''H'' is closed under quaternion multiplication and addition, which makes it a subring of the ring of all quaternions H. Hurwitz quaternions were introduced by . A Lipschitz quaternion (or Lipschitz integer) is a quaternion whose components are all integers. The set of all Lipschitz quaternions :L = \left\ forms a subring of the Hurwitz quaternions ''H''. Hurwitz integers have the advantage over Lipschitz integers that it is possible to perform Euclidean division on them, obtaining a small remainder. Both the Hurwitz and Lipschitz quaternions are examples of noncommutative domains which are not division rings. Struct ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Quaternion Group
In group theory, the quaternion group Q8 (sometimes just denoted by Q) is a non-abelian group of order eight, isomorphic to the eight-element subset \ of the quaternions under multiplication. It is given by the group presentation :\mathrm_8 = \langle \bar,i,j,k \mid \bar^2 = e, \;i^2 = j^2 = k^2 = ijk = \bar \rangle , where ''e'' is the identity element and commutes with the other elements of the group. Another presentation of Q8 is :\mathrm_8 = \langle a,b \mid a^4 = e, a^2 = b^2, ba = a^b\rangle. Compared to dihedral group The quaternion group Q8 has the same order as the dihedral group D4, but a different structure, as shown by their Cayley and cycle graphs: In the diagrams for D4, the group elements are marked with their action on a letter F in the defining representation R2. The same cannot be done for Q8, since it has no faithful representation in R2 or R3. D4 can be realized as a subset of the split-quaternions in the same way that Q8 can be viewed as a sub ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
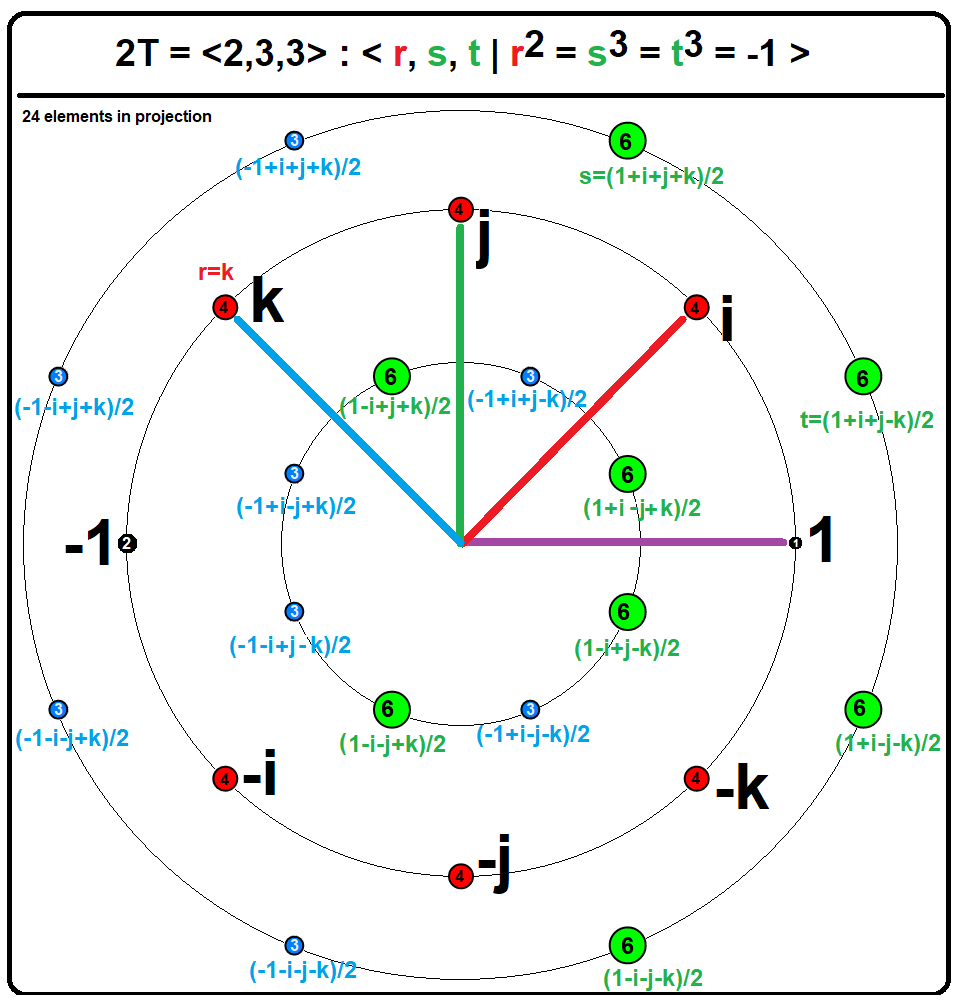
Binary Tetrahedral Group
In mathematics, the binary tetrahedral group, denoted 2T or , Coxeter&Moser: Generators and Relations for discrete groups: : Rl = Sm = Tn = RST is a certain nonabelian group of order 24. It is an extension of the tetrahedral group T or (2,3,3) of order 12 by a cyclic group of order 2, and is the preimage of the tetrahedral group under the 2:1 covering homomorphism Spin(3) → SO(3) of the special orthogonal group by the spin group. It follows that the binary tetrahedral group is a discrete subgroup of Spin(3) of order 24. The complex reflection group named 3(24)3 by G.C. Shephard or 3 and by Coxeter, is isomorphic to the binary tetrahedral group. The binary tetrahedral group is most easily described concretely as a discrete subgroup of the unit quaternions, under the isomorphism , where Sp(1) is the multiplicative group of unit quaternions. (For a description of this homomorphism see the article on quaternions and spatial rotations.) Elements Explicit ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Binary Octahedral Group Subgroups
Binary may refer to: Science and technology Mathematics * Binary number, a representation of numbers using only two digits (0 and 1) * Binary function, a function that takes two arguments * Binary operation, a mathematical operation that takes two arguments * Binary relation, a relation involving two elements * Binary-coded decimal, a method for encoding for decimal digits in binary sequences * Finger binary, a system for counting in binary numbers on the fingers of human hands Computing * Binary code, the digital representation of text and data * Bit, or binary digit, the basic unit of information in computers * Binary file, composed of something other than human-readable text ** Executable, a type of binary file that contains machine code for the computer to execute * Binary tree, a computer tree data structure in which each node has at most two children Astronomy * Binary star, a star system with two stars in it * Binary planet, two planetary bodies of comparable mas ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Group Presentation
In mathematics, a presentation is one method of specifying a group. A presentation of a group ''G'' comprises a set ''S'' of generators—so that every element of the group can be written as a product of powers of some of these generators—and a set ''R'' of relations among those generators. We then say ''G'' has presentation :\langle S \mid R\rangle. Informally, ''G'' has the above presentation if it is the "freest group" generated by ''S'' subject only to the relations ''R''. Formally, the group ''G'' is said to have the above presentation if it is isomorphic to the quotient of a free group on ''S'' by the normal subgroup generated by the relations ''R''. As a simple example, the cyclic group of order ''n'' has the presentation :\langle a \mid a^n = 1\rangle, where 1 is the group identity. This may be written equivalently as :\langle a \mid a^n\rangle, thanks to the convention that terms that do not include an equals sign are taken to be equal to the group identity. S ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Automorphism Group
In mathematics, the automorphism group of an object ''X'' is the group consisting of automorphisms of ''X'' under composition of morphisms. For example, if ''X'' is a finite-dimensional vector space, then the automorphism group of ''X'' is the group of invertible linear transformations from ''X'' to itself (the general linear group of ''X''). If instead ''X'' is a group, then its automorphism group \operatorname(X) is the group consisting of all group automorphisms of ''X''. Especially in geometric contexts, an automorphism group is also called a symmetry group. A subgroup of an automorphism group is sometimes called a transformation group. Automorphism groups are studied in a general way in the field of category theory. Examples If ''X'' is a set with no additional structure, then any bijection from ''X'' to itself is an automorphism, and hence the automorphism group of ''X'' in this case is precisely the symmetric group of ''X''. If the set ''X'' has additional struct ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Inner Automorphism Group
In abstract algebra an inner automorphism is an automorphism of a group, ring, or algebra given by the conjugation action of a fixed element, called the ''conjugating element''. They can be realized via simple operations from within the group itself, hence the adjective "inner". These inner automorphisms form a subgroup of the automorphism group, and the quotient of the automorphism group by this subgroup is defined as the outer automorphism group. Definition If is a group and is an element of (alternatively, if is a ring, and is a unit), then the function :\begin \varphi_g\colon G&\to G \\ \varphi_g(x)&:= g^xg \end is called (right) conjugation by (see also conjugacy class). This function is an endomorphism of : for all x_1,x_2\in G, :\varphi_g(x_1 x_2) = g^ x_1 x_2g = \left(g^ x_1 g\right)\left(g^ x_2 g\right) = \varphi_g(x_1)\varphi_g(x_2), where the second equality is given by the insertion of the identity between x_1 and x_2. Furthermore, it has a left and r ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |