|
Symmetric
Symmetry () in everyday life refers to a sense of harmonious and beautiful proportion and balance. In mathematics, the term has a more precise definition and is usually used to refer to an object that is invariant under some transformations, such as translation, reflection, rotation, or scaling. Although these two meanings of the word can sometimes be told apart, they are intricately related, and hence are discussed together in this article. Mathematical symmetry may be observed with respect to the passage of time; as a spatial relationship; through geometric transformations; through other kinds of functional transformations; and as an aspect of abstract objects, including theoretic models, language, and music. This article describes symmetry from three perspectives: in mathematics, including geometry, the most familiar type of symmetry for many people; in science and nature; and in the arts, covering architecture, art, and music. The opposite of symmetry is asymmetr ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Asymmetry
Asymmetry is the absence of, or a violation of, symmetry (the property of an object being invariant to a transformation, such as reflection). Symmetry is an important property of both physical and abstract systems and it may be displayed in precise terms or in more aesthetic terms. The absence of or violation of symmetry that are either expected or desired can have important consequences for a system. In organisms Due to how cell (biology), cells divide in organisms, asymmetry in organisms is fairly usual in at least one dimension, with Symmetry in biology, biological symmetry also being common in at least one dimension. Louis Pasteur proposed that biological molecules are asymmetric because the cosmic [i.e. physical] forces that preside over their formation are themselves asymmetric. While at his time, and even now, the symmetry of physical processes are highlighted, it is known that there are fundamental physical asymmetries, starting with time. Asymmetry in biology Asy ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Asymmetric (PSF)
Asymmetric may refer to: *Asymmetry Asymmetry is the absence of, or a violation of, symmetry (the property of an object being invariant to a transformation, such as reflection). Symmetry is an important property of both physical and abstract systems and it may be displayed in pre ... in geometry, chemistry, and physics Computing * Asymmetric cryptography, in public-key cryptography * Asymmetric digital subscriber line, Internet connectivity * Asymmetric multiprocessing, in computer architecture Other * Asymmetric relation, in set theory * Asymmetric synthesis, in organic synthesis * Asymmetric warfare, in modern war * Asymmetric Publications, a video game company * ''Asymmetry'' (Mallory Knox album), 2014 * ''Asymmetry'' (Karnivool album), 2013 * Asymmetry (population ethics) * Asymmetry (novel), a 2018 novel by Lisa Halliday See also * * {{disambiguation ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Molecule
A molecule is a group of two or more atoms that are held together by Force, attractive forces known as chemical bonds; depending on context, the term may or may not include ions that satisfy this criterion. In quantum physics, organic chemistry, and biochemistry, the distinction from ions is dropped and ''molecule'' is often used when referring to polyatomic ions. A molecule may be homonuclear, that is, it consists of atoms of one chemical element, e.g. two atoms in the oxygen molecule (O2); or it may be heteronuclear, a chemical compound composed of more than one element, e.g. water (molecule), water (two hydrogen atoms and one oxygen atom; H2O). In the kinetic theory of gases, the term ''molecule'' is often used for any gaseous particle regardless of its composition. This relaxes the requirement that a molecule contains two or more atoms, since the noble gases are individual atoms. Atoms and complexes connected by non-covalent interactions, such as hydrogen bonds or ionic ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Quilt
A quilt is a multi-layered textile, traditionally composed of two or more layers of fabric or fiber. Commonly three layers are used with a filler material. These layers traditionally include a woven cloth top, a layer of padding, batting or wadding, and a woven back combined using the techniques of quilting. This is the process of sewing on the face of the fabric, and not just the edges, to combine the three layers together to reinforce the material. Stitching patterns can be a decorative element. A single piece of fabric can be used for the top of a quilt (a "whole-cloth quilt"), but in many cases the top is created from smaller fabric pieces joined, or patchwork. The pattern and color of these pieces creates the design. Quilts may contain valuable historical information about their creators, "visualizing particular segments of history in tangible, textured ways". In the twenty-first century, quilts are frequently displayed as non-utilitarian works of art but historically qui ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Mathematics
Mathematics is a field of study that discovers and organizes methods, Mathematical theory, theories and theorems that are developed and Mathematical proof, proved for the needs of empirical sciences and mathematics itself. There are many areas of mathematics, which include number theory (the study of numbers), algebra (the study of formulas and related structures), geometry (the study of shapes and spaces that contain them), Mathematical analysis, analysis (the study of continuous changes), and set theory (presently used as a foundation for all mathematics). Mathematics involves the description and manipulation of mathematical object, abstract objects that consist of either abstraction (mathematics), abstractions from nature orin modern mathematicspurely abstract entities that are stipulated to have certain properties, called axioms. Mathematics uses pure reason to proof (mathematics), prove properties of objects, a ''proof'' consisting of a succession of applications of in ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Invariant (mathematics)
In mathematics, an invariant is a property of a mathematical object (or a class of mathematical objects) which remains unchanged after operations or transformations of a certain type are applied to the objects. The particular class of objects and type of transformations are usually indicated by the context in which the term is used. For example, the area of a triangle is an invariant with respect to isometries of the Euclidean plane. The phrases "invariant under" and "invariant to" a transformation are both used. More generally, an invariant with respect to an equivalence relation is a property that is constant on each equivalence class. Invariants are used in diverse areas of mathematics such as geometry, topology, algebra and discrete mathematics. Some important classes of transformations are defined by an invariant they leave unchanged. For example, conformal maps are defined as transformations of the plane that preserve angles. The discovery of invariants is an import ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Time
Time is the continuous progression of existence that occurs in an apparently irreversible process, irreversible succession from the past, through the present, and into the future. It is a component quantity of various measurements used to sequence events, to compare the duration of events (or the intervals between them), and to quantify rates of change of quantities in material reality or in the qualia, conscious experience. Time is often referred to as a fourth dimension, along with Three-dimensional space, three spatial dimensions. Time is one of the seven fundamental physical quantities in both the International System of Units (SI) and International System of Quantities. The SI base unit of time is the second, which is defined by measuring the electronic transition frequency of caesium atoms. General relativity is the primary framework for understanding how spacetime works. Through advances in both theoretical and experimental investigations of spacetime, it has been shown ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Scaling (geometry)
In affine geometry, uniform scaling (or isotropic scaling) is a linear transformation that enlarges (increases) or shrinks (diminishes) objects by a '' scale factor'' that is the same in all directions ( isotropically). The result of uniform scaling is similar (in the geometric sense) to the original. A scale factor of 1 is normally allowed, so that congruent shapes are also classed as similar. Uniform scaling happens, for example, when enlarging or reducing a photograph, or when creating a scale model of a building, car, airplane, etc. More general is scaling with a separate scale factor for each axis direction. Non-uniform scaling (anisotropic scaling) is obtained when at least one of the scaling factors is different from the others; a special case is directional scaling or stretching (in one direction). Non-uniform scaling changes the shape of the object; e.g. a square may change into a rectangle, or into a parallelogram if the sides of the square are not parallel to th ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Reflection (mathematics)
In mathematics, a reflection (also spelled reflexion) is a mapping from a Euclidean space to itself that is an isometry with a hyperplane as the set of fixed points; this set is called the axis (in dimension 2) or plane (in dimension 3) of reflection. The image of a figure by a reflection is its mirror image in the axis or plane of reflection. For example the mirror image of the small Latin letter p for a reflection with respect to a vertical axis (a ''vertical reflection'') would look like q. Its image by reflection in a horizontal axis (a ''horizontal reflection'') would look like b. A reflection is an involution: when applied twice in succession, every point returns to its original location, and every geometrical object is restored to its original state. The term ''reflection'' is sometimes used for a larger class of mappings from a Euclidean space to itself, namely the non-identity isometries that are involutions. The set of fixed points (the "mirror") of such an isome ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
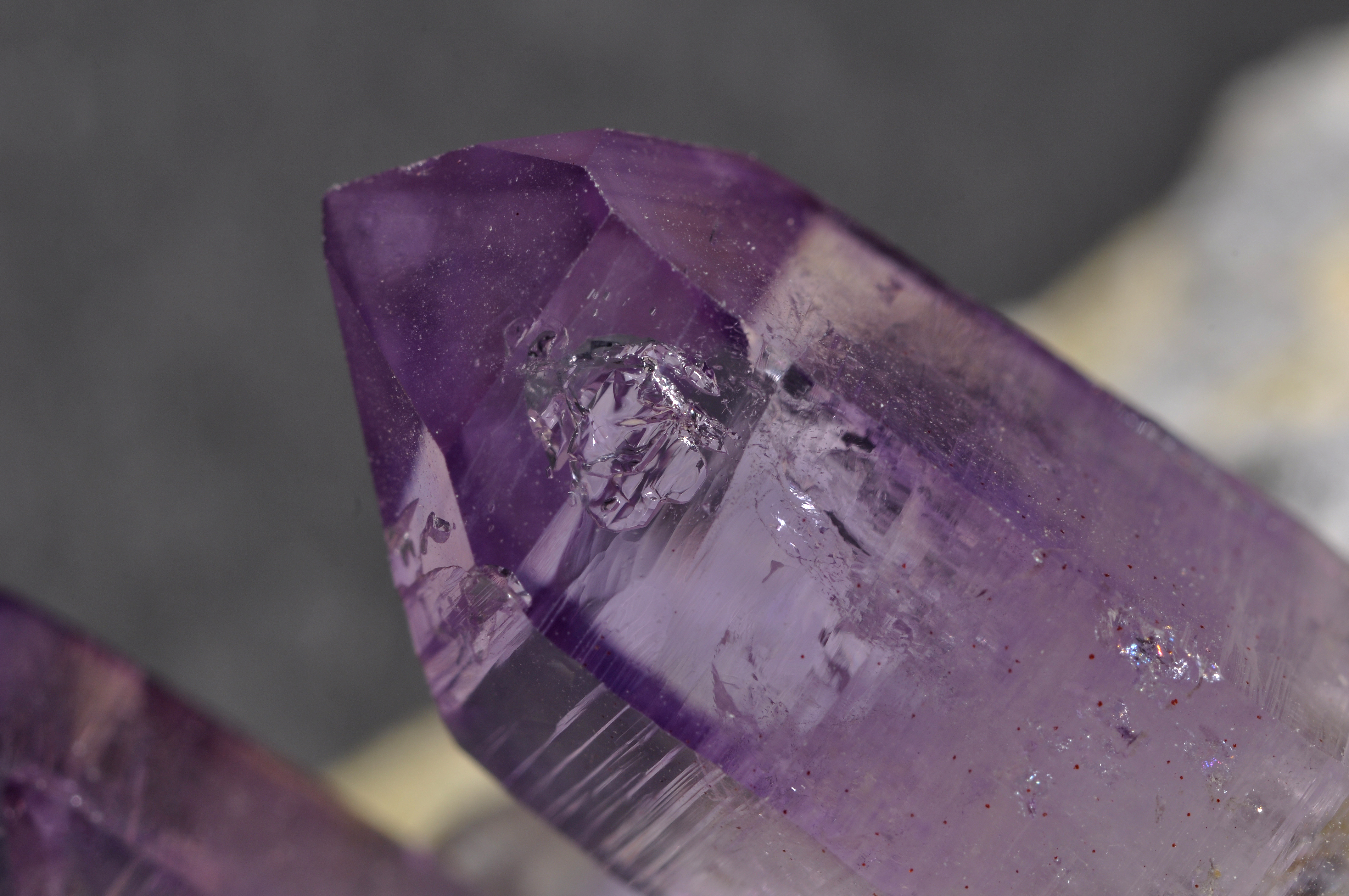
Crystal
A crystal or crystalline solid is a solid material whose constituents (such as atoms, molecules, or ions) are arranged in a highly ordered microscopic structure, forming a crystal lattice that extends in all directions. In addition, macroscopic single crystals are usually identifiable by their geometrical shape, consisting of flat faces with specific, characteristic orientations. The scientific study of crystals and crystal formation is known as crystallography. The process of crystal formation via mechanisms of crystal growth is called crystallization or solidification. The word ''crystal'' derives from the Ancient Greek word (), meaning both "ice" and " rock crystal", from (), "icy cold, frost". Examples of large crystals include snowflakes, diamonds, and table salt. Most inorganic solids are not crystals but polycrystals, i.e. many microscopic crystals fused together into a single solid. Polycrystals include most metals, rocks, ceramics, and ice. A third cat ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Pamment
Tiles are usually thin, square or rectangular coverings manufactured from hard-wearing material such as ceramic, Rock (geology), stone, metal, baked clay, or even glass. They are generally fixed in place in an array to cover roofs, floors, walls, edges, or other objects such as tabletops. Alternatively, tile can sometimes refer to similar units made from lightweight materials such as perlite, wood, and mineral wool, typically used for wall and ceiling applications. In another sense, a tile is a construction tile or similar object, such as rectangular counters used in playing games (see tile-based game). The word is derived from the French Language, French word ''tuile'', which is, in turn, from the Latin Language, Latin word ''tegula'', meaning a roof tile composed of fired clay. Tiles are often used to form wall and floor coverings, and can range from simple square tiles to complex or mosaics. Tiles are most often made of pottery, ceramic, typically Ceramic glaze, glazed for ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |