|
Static Pressure
In fluid mechanics the term static pressure refers to a term in Bernoulli's equation written words as ''static pressure + dynamic pressure = total pressure''. Since pressure measurements at any single point in a fluid always give the static pressure value, the 'static' is often dropped. In the design and operation of aircraft, ''static pressure'' is the air pressure in the aircraft's static pressure system. Static pressure in fluid dynamics The concept of pressure is central to the study of fluids. A pressure can be identified for every point in a body of fluid, regardless of whether the fluid is in motion. Pressure can be measured using an aneroid, Bourdon tube, mercury column, or various other methods. The concepts of ''total pressure'' and '' dynamic pressure'' arise from Bernoulli's equation and are significant in the study of all fluid flows. These two pressures are not pressures in the usual sense - they cannot be measured using a pressure sensor. To avoid potential ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Fluid Mechanics
Fluid mechanics is the branch of physics concerned with the mechanics of fluids (liquids, gases, and plasma (physics), plasmas) and the forces on them. Originally applied to water (hydromechanics), it found applications in a wide range of disciplines, including mechanical engineering, mechanical, aerospace engineering, aerospace, civil engineering, civil, chemical engineering, chemical, and biomedical engineering, as well as geophysics, oceanography, meteorology, astrophysics, and biology. It can be divided into ''fluid statics'', the study of various fluids at rest; and ''fluid dynamics'', the study of the effect of forces on fluid motion. It is a branch of ''continuum mechanics'', a subject which models matter without using the information that it is made out of atoms; that is, it models matter from a macroscopic viewpoint rather than from microscopic. Fluid mechanics, especially fluid dynamics, is an active field of research, typically mathematically complex. Many problems a ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Speed Of Sound
The speed of sound is the distance travelled per unit of time by a sound wave as it propagates through an elasticity (solid mechanics), elastic medium. More simply, the speed of sound is how fast vibrations travel. At , the speed of sound in air is about , or in or one mile in . It depends strongly on temperature as well as the medium through which a sound wave is propagating. At , the speed of sound in dry air (sea level 14.7 psi) is about . The speed of sound in an ideal gas depends only on its temperature and composition. The speed has a weak dependence on frequency and pressure in dry air, deviating slightly from ideal behavior. In colloquial speech, ''speed of sound'' refers to the speed of sound waves in Earth's atmosphere, air. However, the speed of sound varies from substance to substance: typically, sound travels most slowly in gases, faster in liquids, and fastest in solids. For example, while sound travels at in air, it travels at in water (almost 4.3 times a ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Standard Conditions For Temperature And Pressure
Standard temperature and pressure (STP) or standard conditions for temperature and pressure are various standard sets of conditions for experimental measurements used to allow comparisons to be made between different sets of data. The most used standards are those of the International Union of Pure and Applied Chemistry (IUPAC) and the National Institute of Standards and Technology (NIST), although these are not universally accepted. Other organizations have established a variety of other definitions. In industry and commerce, the standard conditions for temperature and pressure are often necessary for expressing the volumes of gases and liquids and related quantities such as the rate of volumetric flow (the volumes of gases vary significantly with temperature and pressure): standard cubic meters per second (Sm3/s), and normal cubic meters per second (Nm3/s). Many technical publications (books, journals, advertisements for equipment and machinery) simply state "standard cond ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Stagnation Pressure
In fluid dynamics, stagnation pressure, also referred to as total pressure, is what the pressure would be if all the kinetic energy of the fluid were to be converted into pressure in a reversable manner.; it is defined as the sum of the free-stream static pressure and the free-stream dynamic pressure. The Bernoulli equation applicable to incompressible flow shows that the stagnation pressure is equal to the dynamic pressure and static pressure combined.Clancy, L.J. (1975), ''Aerodynamics'', Pitman Publishing Limited, London. In compressible flows, stagnation pressure is also equal to total pressure as well, provided that the fluid entering the stagnation point is brought to rest Isentropic flow, isentropically. Stagnation pressure is sometimes referred to as pitot pressure because the two pressures are equal. Magnitude The magnitude of stagnation pressure can be derived from Bernoulli's principle, Bernoulli equation for incompressible flow and no height changes. For any two po ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Pascal's Law
Pascal's law (also Pascal's principle or the principle of transmission of fluid-pressure) is a principle in fluid mechanics that states that a pressure change at any point in a confined incompressible fluid is transmitted throughout the fluid such that the same change occurs everywhere. The law was established by French people, French mathematician Blaise Pascal in 1653 and published in 1663. Definition Pascal's principle is defined as: Fluid column with gravity For a fluid column in a uniform gravity (e.g. in a hydraulic press), this principle can be stated mathematically as: \Delta p = \rho g \cdot\Delta h\, where The intuitive explanation of this formula is that the change in pressure between two elevations is due to the weight of the fluid between the elevations. Note that the variation with height does not depend on any additional pressures. Therefore, Pascal's law can be interpreted as saying that ''any change in pressure applied'' at any given point of the fluid i ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Fluid Statics
In physics, a fluid is a liquid, gas, or other material that may continuously move and deform (''flow'') under an applied shear stress, or external force. They have zero shear modulus, or, in simpler terms, are substances which cannot resist any shear force applied to them. Although the term ''fluid'' generally includes both the liquid and gas phases, its definition varies among branches of science. Definitions of '' solid'' vary as well, and depending on field, some substances can have both fluid and solid properties. Non-Newtonian fluids like Silly Putty appear to behave similar to a solid when a sudden force is applied. Substances with a very high viscosity such as pitch appear to behave like a solid (see pitch drop experiment) as well. In particle physics, the concept is extended to include fluidic matters other than liquids or gases. A fluid in medicine or biology refers to any liquid constituent of the body ( body fluid), whereas "liquid" is not used in this ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Hydrostatics
Hydrostatics is the branch of fluid mechanics that studies fluids at hydrostatic equilibrium and "the pressure in a fluid or exerted by a fluid on an immersed body". The word "hydrostatics" is sometimes used to refer specifically to water and other liquids, but more often it includes both gases and liquids, whether compressible or incompressible. It encompasses the study of the conditions under which fluids are at rest in stable equilibrium. It is opposed to ''fluid dynamics'', the study of fluids in motion. Hydrostatics is fundamental to ''hydraulics'', the engineering of equipment for storing, transporting and using fluids. It is also relevant to geophysics and astrophysics (for example, in understanding plate tectonics and the anomalies of the Earth's gravitational field), to meteorology, to medicine (in the context of blood pressure), and many other fields. Hydrostatics offers physical explanations for many phenomena of everyday life, such as why atmospheric pressur ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Freestream
The freestream is the air far upstream of an aerodynamic Aerodynamics () is the study of the motion of atmosphere of Earth, air, particularly when affected by a solid object, such as an airplane wing. It involves topics covered in the field of fluid dynamics and its subfield of gas dynamics, and is an ... body, that is, before the body has a chance to deflect, slow down or compress the air. Freestream conditions are usually denoted with a \infty symbol, e.g. V_\infty, meaning the freestream velocity. References *Anderson, John D., 1989. ''Introduction to Flight'', 3rd Ed. McGraw-Hill Aerodynamics {{Fluiddynamics-stub ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Position Error
Position error is one of the errors affecting the systems in an aircraft for measuring airspeed and altitude. It is not practical or necessary for an aircraft to have an airspeed indicating system and an altitude indicating system that are exactly accurate. A small amount of error is tolerable. It is caused by the location of the static vent that supplies air pressure to the airspeed indicator and altimeter; there is no position on an aircraft where, at all angles of attack, the static pressure is always equal to atmospheric pressure. Static system All aircraft are equipped with a small hole in the surface of the aircraft called the static port. The air pressure in the vicinity of the static port is conveyed by a conduit to the altimeter and the airspeed indicator. This static port and the conduit constitute the aircraft's static system. The objective of the static system is to sense the pressure of the air at the altitude at which the aircraft is flying. In an ideal stati ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
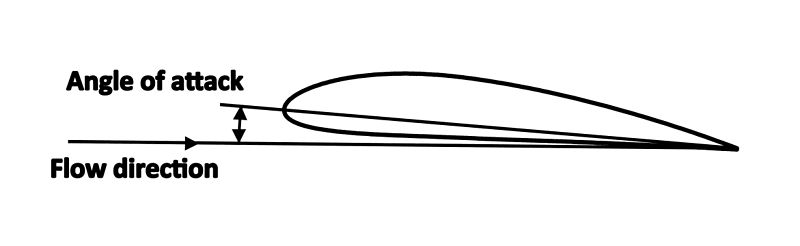
Angle Of Attack
In fluid dynamics, angle of attack (AOA, α, or \alpha) is the angle between a Airfoil#Airfoil terminology, reference line on a body (often the chord (aircraft), chord line of an airfoil) and the vector (geometry), vector representing the relative motion between the body and the fluid through which it is moving. Angle of attack is the angle between the body's reference line and the oncoming flow. This article focuses on the most common application, the angle of attack of a wing or airfoil moving through air. In aerodynamics, angle of attack specifies the angle between the chord line of the wing of a fixed-wing aircraft and the vector representing the relative motion between the aircraft and the atmosphere. Since a wing can have twist, a chord line of the whole wing may not be definable, so an alternate reference line is simply defined. Often, the chord line of the Wing root, root of the wing is chosen as the reference line. Another choice is to use a horizontal line on the fuse ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Altitude
Altitude is a distance measurement, usually in the vertical or "up" direction, between a reference datum (geodesy), datum and a point or object. The exact definition and reference datum varies according to the context (e.g., aviation, geometry, geographical survey, sport, or atmospheric pressure). Although the term ''altitude'' is commonly used to mean the height above sea level of a location, in geography the term elevation is often preferred for this usage. In aviation, altitude is typically measured relative to mean sea level or above ground level to ensure safe navigation and flight operations. In geometry and geographical surveys, altitude helps create accurate topographic maps and understand the terrain's elevation. For high-altitude trekking and sports, knowing and adapting to altitude is vital for performance and safety. Higher altitudes mean reduced oxygen levels, which can lead to altitude sickness if proper acclimatization measures are not taken. Vertical distance ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Airspeed Indicator
The airspeed indicator (ASI) or airspeed gauge is a flight instrument indicating the airspeed of an aircraft in kilometres per hour (km/h), knots (kn or kt), miles per hour (MPH) and/or metres per second (m/s). The recommendation by ICAO is to use km/h, however knots (kt) is currently the most used unit. The ASI measures the pressure differential between static pressure from the static port, and total pressure from the pitot tube. This difference in pressure is registered with the ASI pointer on the face of the instrument. Colour-coded speeds and ranges The ASI has standard colour-coded markings to indicate safe operation within the limitations of the aircraft. At a glance, the pilot can determine a recommended speed (V speeds) or if speed adjustments are needed. Single and multi-engine aircraft have common markings. For instance, the green arc indicates the normal operating range of the aircraft, from ''V''S1 to ''V''NO. The white arc indicates the flap operating range ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |