|
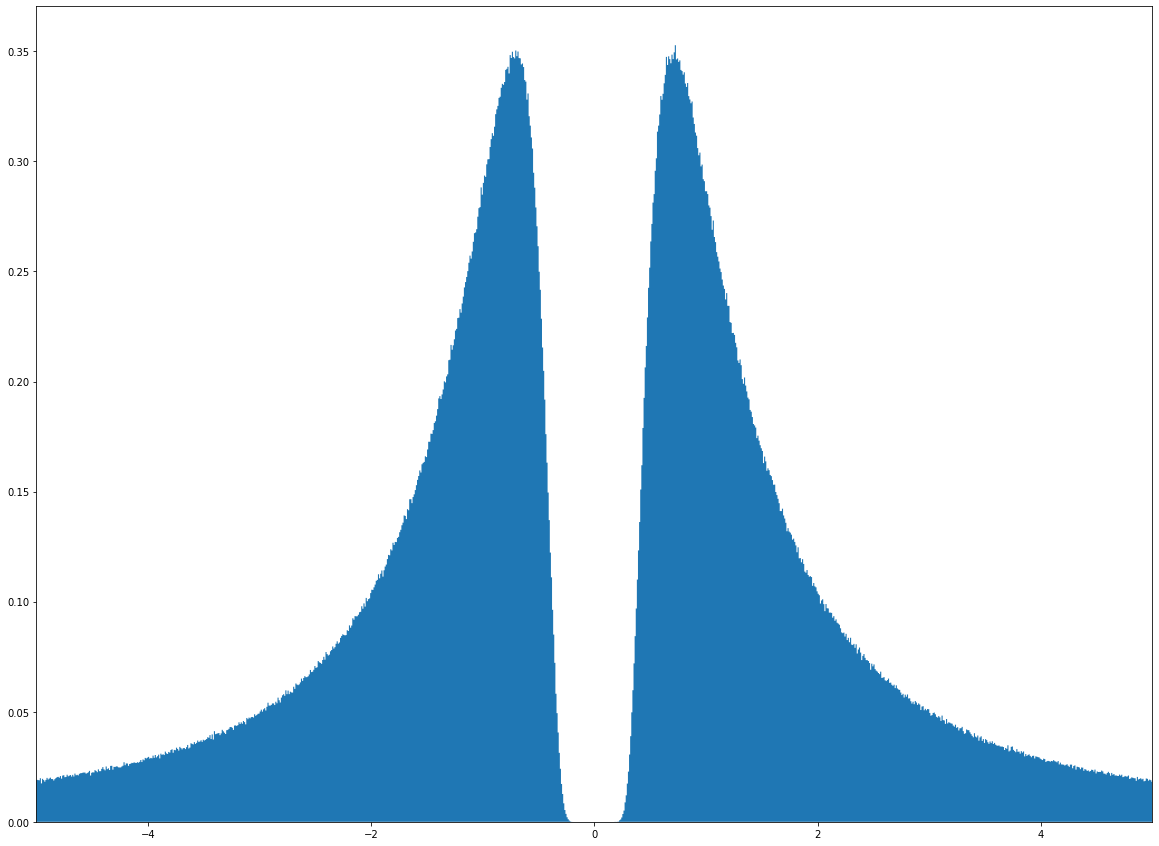
Reciprocal Normal Distribution
In probability theory and statistics, an inverse distribution is the distribution of the reciprocal of a random variable. Inverse distributions arise in particular in the Bayesian context of prior distributions and posterior distributions for scale parameters. In the algebra of random variables, inverse distributions are special cases of the class of ratio distributions, in which the numerator random variable has a degenerate distribution. Relation to original distribution In general, given the probability distribution of a random variable ''X'' with strictly positive support, it is possible to find the distribution of the reciprocal, ''Y'' = 1 / ''X''. If the distribution of ''X'' is continuous with density function ''f''(''x'') and cumulative distribution function ''F''(''x''), then the cumulative distribution function, ''G''(''y''), of the reciprocal is found by noting that : G(y) = \Pr(Y \leq y) = \Pr\left(X \geq \frac\right) = 1-\Pr\left(X<\frac\right) = 1 - F\left( ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Probability Theory
Probability theory is the branch of mathematics concerned with probability. Although there are several different probability interpretations, probability theory treats the concept in a rigorous mathematical manner by expressing it through a set of axioms of probability, axioms. Typically these axioms formalise probability in terms of a probability space, which assigns a measure (mathematics), measure taking values between 0 and 1, termed the probability measure, to a set of outcomes called the sample space. Any specified subset of the sample space is called an event (probability theory), event. Central subjects in probability theory include discrete and continuous random variables, probability distributions, and stochastic processes (which provide mathematical abstractions of determinism, non-deterministic or uncertain processes or measured Quantity, quantities that may either be single occurrences or evolve over time in a random fashion). Although it is not possible to perfectly p ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Uniform Distribution (continuous)
In probability theory and statistics, the continuous uniform distribution or rectangular distribution is a family of symmetric probability distributions. The distribution describes an experiment where there is an arbitrary outcome that lies between certain bounds. The bounds are defined by the parameters, ''a'' and ''b'', which are the minimum and maximum values. The interval can either be closed (e.g. , b or open (e.g. (a, b)). Therefore, the distribution is often abbreviated ''U'' (''a'', ''b''), where U stands for uniform distribution. The difference between the bounds defines the interval length; all intervals of the same length on the distribution's support are equally probable. It is the maximum entropy probability distribution for a random variable ''X'' under no constraint other than that it is contained in the distribution's support. Definitions Probability density function The probability density function of the continuous uniform distribution is: : f(x)=\be ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Dawson's Function
In mathematics, the Dawson function or Dawson integral (named after H. G. Dawson) is the one-sided Fourier–Laplace sine transform of the Gaussian function. Definition The Dawson function is defined as either: D_+(x) = e^ \int_0^x e^\,dt, also denoted as F(x) or D(x), or alternatively D_-(x) = e^ \int_0^x e^\,dt.\! The Dawson function is the one-sided Fourier–Laplace sine transform of the Gaussian function, >D_+(x) = \frac12 \int_0^\infty e^\,\sin(xt)\,dt. It is closely related to the error function erf, as : D_+(x) = e^ \operatorname (x) = - e^ \operatorname (ix) where erfi is the imaginary error function, Similarly, D_-(x) = \frac e^ \operatorname(x) in terms of the real error function, erf. In terms of either erfi or the Faddeeva function w(z), the Dawson function can be extended to the entire complex plane:Mofreh R. Zaghloul and Ahmed N. Ali,Algorithm 916: Computing the Faddeyeva and Voigt Functions" ''ACM Trans. Math. Soft.'' 38 (2), 15 (2011). Preprint available ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Principal Value
In mathematics, specifically complex analysis, the principal values of a multivalued function are the values along one chosen branch of that function, so that it is single-valued. The simplest case arises in taking the square root of a positive real number. For example, 4 has two square roots: 2 and −2; of these the positive root, 2, is considered the principal root and is denoted as \sqrt. Motivation Consider the complex logarithm function log ''z''. It is defined as the complex number ''w'' such that :e^w = z. Now, for example, say we wish to find log ''i''. This means we want to solve :e^w = i for ''w''. Clearly ''i''π/2 is a solution. But is it the only solution? Of course, there are other solutions, which is evidenced by considering the position of ''i'' in the complex plane and in particular its argument arg ''i''. We can rotate counterclockwise π/2 radians from 1 to reach ''i'' initially, but if we rotate further another 2π we reach ''i'' again. So, ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Management Science (journal)
''For the theoretical and practical problem-solving subfield of Management, see Management Science.'' ''Management Science'' is a peer-reviewed academic journal that covers research on all aspects of management related to strategy, entrepreneurship, innovation, information technology, and organizations as well as all functional areas of business, such as accounting, finance, marketing, and operations. It is published by the Institute for Operations Research and the Management Sciences and was established in 1954 by the institute's precursor, the Institute of Management Sciences. C. West Churchman was the founding editor-in-chief. According to the ''Journal Citation Reports'', the journal has a 2018 impact factor of 4.219. Editors-in-chief The following persons are, or have been, editors-in-chief: *2018–2020: David Simchi-Levi *2014–2018: Teck-Hua Ho *2009–2014: Gérard Cachon *2003–2008: Wallace Hopp *1997–2002: Hau L. Lee *1993–1997: Gabriel R. Bitran *1983– ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Monte Carlo Simulation
Monte Carlo methods, or Monte Carlo experiments, are a broad class of computational algorithms that rely on repeated random sampling to obtain numerical results. The underlying concept is to use randomness to solve problems that might be deterministic in principle. They are often used in physical and mathematical problems and are most useful when it is difficult or impossible to use other approaches. Monte Carlo methods are mainly used in three problem classes: optimization, numerical integration, and generating draws from a probability distribution. In physics-related problems, Monte Carlo methods are useful for simulating systems with many coupled degrees of freedom, such as fluids, disordered materials, strongly coupled solids, and cellular structures (see cellular Potts model, interacting particle systems, McKean–Vlasov processes, kinetic models of gases). Other examples include modeling phenomena with significant uncertainty in inputs such as the calculation of ris ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Bimodal Distribution
In statistics, a multimodal distribution is a probability distribution with more than one mode. These appear as distinct peaks (local maxima) in the probability density function, as shown in Figures 1 and 2. Categorical, continuous, and discrete data can all form multimodal distributions. Among univariate analyses, multimodal distributions are commonly bimodal. Terminology When the two modes are unequal the larger mode is known as the major mode and the other as the minor mode. The least frequent value between the modes is known as the antimode. The difference between the major and minor modes is known as the amplitude. In time series the major mode is called the acrophase and the antimode the batiphase. Galtung's classification Galtung introduced a classification system (AJUS) for distributions: *A: unimodal distribution – peak in the middle *J: unimodal – peak at either end *U: bimodal – peaks at both ends *S: bimodal or multimodal – multiple peaks This c ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Heavy-tailed Distribution
In probability theory, heavy-tailed distributions are probability distributions whose tails are not exponentially bounded: that is, they have heavier tails than the exponential distribution. In many applications it is the right tail of the distribution that is of interest, but a distribution may have a heavy left tail, or both tails may be heavy. There are three important subclasses of heavy-tailed distributions: the fat-tailed distributions, the long-tailed distributions and the subexponential distributions. In practice, all commonly used heavy-tailed distributions belong to the subexponential class. There is still some discrepancy over the use of the term heavy-tailed. There are two other definitions in use. Some authors use the term to refer to those distributions which do not have all their power moments finite; and some others to those distributions that do not have a finite variance. The definition given in this article is the most general in use, and includes all di ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Standard Normal Distribution
In statistics, a normal distribution or Gaussian distribution is a type of continuous probability distribution for a real-valued random variable. The general form of its probability density function is : f(x) = \frac e^ The parameter \mu is the mean or expectation of the distribution (and also its median and mode), while the parameter \sigma is its standard deviation. The variance of the distribution is \sigma^2. A random variable with a Gaussian distribution is said to be normally distributed, and is called a normal deviate. Normal distributions are important in statistics and are often used in the natural and social sciences to represent real-valued random variables whose distributions are not known. Their importance is partly due to the central limit theorem. It states that, under some conditions, the average of many samples (observations) of a random variable with finite mean and variance is itself a random variable—whose distribution converges to a normal dist ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Graph Of Inverse Of The Normal Distribution
Graph may refer to: Mathematics *Graph (discrete mathematics), a structure made of vertices and edges **Graph theory, the study of such graphs and their properties * Graph (topology), a topological space resembling a graph in the sense of discrete mathematics *Graph of a function * Graph of a relation *Graph paper * Chart, a means of representing data (also called a graph) Computing *Graph (abstract data type), an abstract data type representing relations or connections * graph (Unix), Unix command-line utility *Conceptual graph, a model for knowledge representation and reasoning Other uses * HMS ''Graph'', a submarine of the UK Royal Navy See also *Complex network *Graf *Graff (other) *Graph database *Grapheme, in linguistics *Graphemics *Graphic (other) *-graphy (suffix from the Greek for "describe," "write" or "draw") * List of information graphics software *Statistical graphics Statistical graphics, also known as statistical graphical techniques, are gr ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Normal Distribution
In statistics, a normal distribution or Gaussian distribution is a type of continuous probability distribution for a real-valued random variable. The general form of its probability density function is : f(x) = \frac e^ The parameter \mu is the mean or expectation of the distribution (and also its median and mode), while the parameter \sigma is its standard deviation. The variance of the distribution is \sigma^2. A random variable with a Gaussian distribution is said to be normally distributed, and is called a normal deviate. Normal distributions are important in statistics and are often used in the natural and social sciences to represent real-valued random variables whose distributions are not known. Their importance is partly due to the central limit theorem. It states that, under some conditions, the average of many samples (observations) of a random variable with finite mean and variance is itself a random variable—whose distribution converges to a normal dist ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Bimodal
In statistics, a multimodal distribution is a probability distribution with more than one mode. These appear as distinct peaks (local maxima) in the probability density function, as shown in Figures 1 and 2. Categorical, continuous, and discrete data can all form multimodal distributions. Among univariate analyses, multimodal distributions are commonly bimodal. Terminology When the two modes are unequal the larger mode is known as the major mode and the other as the minor mode. The least frequent value between the modes is known as the antimode. The difference between the major and minor modes is known as the amplitude. In time series the major mode is called the acrophase and the antimode the batiphase. Galtung's classification Galtung introduced a classification system (AJUS) for distributions: *A: unimodal distribution – peak in the middle *J: unimodal – peak at either end *U: bimodal – peaks at both ends *S: bimodal or multimodal – multiple peaks This c ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |