monohedral tiling on:
[Wikipedia]
[Google]
[Amazon]
A tessellation or tiling is the covering of a
 Tessellations were used by the
Tessellations were used by the  Some two hundred years later in 1891, the Russian crystallographer Yevgraf Fyodorov proved that every periodic tiling of the plane features one of seventeen different groups of isometries. Fyodorov's work marked the unofficial beginning of the mathematical study of tessellations. Other prominent contributors include
Some two hundred years later in 1891, the Russian crystallographer Yevgraf Fyodorov proved that every periodic tiling of the plane features one of seventeen different groups of isometries. Fyodorov's work marked the unofficial beginning of the mathematical study of tessellations. Other prominent contributors include
 Tessellation in two dimensions, also called planar tiling, is a topic in geometry that studies how shapes, known as ''tiles'', can be arranged to fill a plane without any gaps, according to a given set of rules. These rules can be varied. Common ones are that there must be no gaps between tiles, and that no corner of one tile can lie along the edge of another. The tessellations created by bonded brickwork do not obey this rule. Among those that do, a regular tessellation has both identical regular tiles and identical regular corners or vertices, having the same angle between adjacent edges for every tile. There are only three shapes that can form such regular tessellations: the equilateral
Tessellation in two dimensions, also called planar tiling, is a topic in geometry that studies how shapes, known as ''tiles'', can be arranged to fill a plane without any gaps, according to a given set of rules. These rules can be varied. Common ones are that there must be no gaps between tiles, and that no corner of one tile can lie along the edge of another. The tessellations created by bonded brickwork do not obey this rule. Among those that do, a regular tessellation has both identical regular tiles and identical regular corners or vertices, having the same angle between adjacent edges for every tile. There are only three shapes that can form such regular tessellations: the equilateral  More formally, a tessellation or tiling is a
More formally, a tessellation or tiling is a
 A is a tessellation in which all tiles are
A is a tessellation in which all tiles are  A semi-regular (or Archimedean) tessellation uses more than one type of regular polygon in an isogonal arrangement. There are eight semi-regular tilings (or nine if the mirror-image pair of tilings counts as two). These can be described by their
A semi-regular (or Archimedean) tessellation uses more than one type of regular polygon in an isogonal arrangement. There are eight semi-regular tilings (or nine if the mirror-image pair of tilings counts as two). These can be described by their
 Tilings with
Tilings with

 Truchet tiles are square tiles decorated with patterns so they do not have
Truchet tiles are square tiles decorated with patterns so they do not have
 Sometimes the colour of a tile is understood as part of the tiling; at other times arbitrary colours may be applied later. When discussing a tiling that is displayed in colours, to avoid ambiguity one needs to specify whether the colours are part of the tiling or just part of its illustration. This affects whether tiles with the same shape but different colours are considered identical, which in turn affects questions of symmetry. The
Sometimes the colour of a tile is understood as part of the tiling; at other times arbitrary colours may be applied later. When discussing a tiling that is displayed in colours, to avoid ambiguity one needs to specify whether the colours are part of the tiling or just part of its illustration. This affects whether tiles with the same shape but different colours are considered identical, which in turn affects questions of symmetry. The
 Next to the various tilings by regular polygons, tilings by other polygons have also been studied.
Any triangle or
Next to the various tilings by regular polygons, tilings by other polygons have also been studied.
Any triangle or
 Tessellation can be extended to three dimensions. Certain
Tessellation can be extended to three dimensions. Certain  Tessellations in three or more dimensions are called honeycombs. In three dimensions there is just one regular honeycomb, which has eight cubes at each polyhedron vertex. Similarly, in three dimensions there is just one quasiregular honeycomb, which has eight
Tessellations in three or more dimensions are called honeycombs. In three dimensions there is just one regular honeycomb, which has eight cubes at each polyhedron vertex. Similarly, in three dimensions there is just one quasiregular honeycomb, which has eight

 It is possible to tessellate in
It is possible to tessellate in
 In architecture, tessellations have been used to create decorative motifs since ancient times.
In architecture, tessellations have been used to create decorative motifs since ancient times.
 The
The  In botany, the term "tessellate" describes a checkered pattern, for example on a flower petal, tree bark, or fruit. Flowers including the
In botany, the term "tessellate" describes a checkered pattern, for example on a flower petal, tree bark, or fruit. Flowers including the
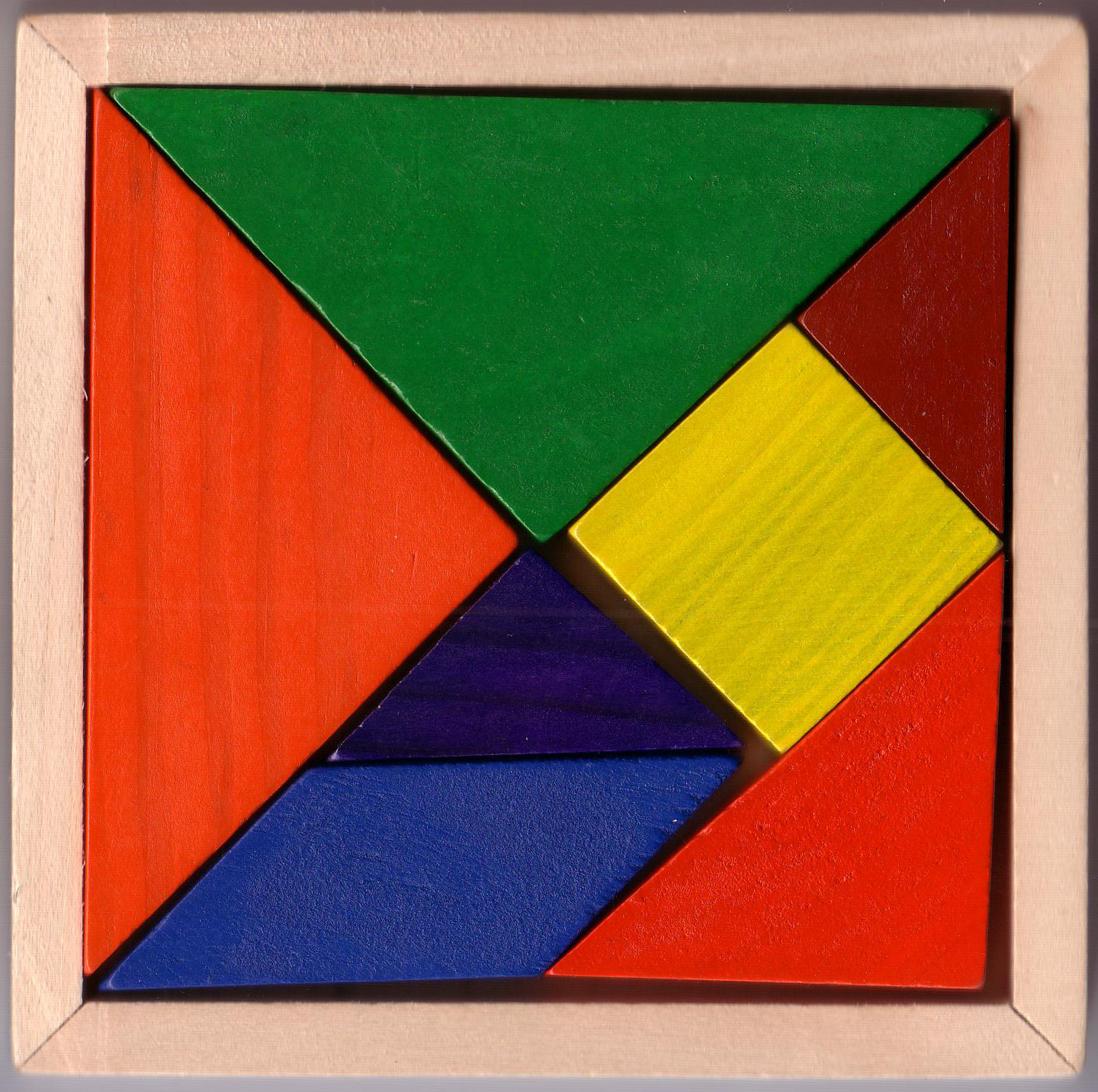 Tessellations have given rise to many types of tiling puzzle, from traditional jigsaw puzzles (with irregular pieces of wood or cardboard) and the tangram to more modern puzzles which often have a mathematical basis. For example, polyiamonds and
Tessellations have given rise to many types of tiling puzzle, from traditional jigsaw puzzles (with irregular pieces of wood or cardboard) and the tangram to more modern puzzles which often have a mathematical basis. For example, polyiamonds and
File:Tile 3,6.svg, Triangular tiling, one of the three regular tilings of the plane.
File:Academ Periodic tiling where eighteen triangles encircle each hexagon.svg, Snub hexagonal tiling, a
Tegula
(open-source software for exploring two-dimensional tilings of the plane, sphere and hyperbolic plane; includes databases containing millions of tilings)
(good bibliography, drawings of regular, semiregular and demiregular tessellations) *Dirk Frettlöh and Edmund Harriss.
Tilings Encyclopedia
(extensive information on substitution tilings, including drawings, people, and references)
Tessellations.org
(how-to guides, Escher tessellation gallery, galleries of tessellations by other artists, lesson plans, history) * (list of web resources including articles and galleries) {{Good article Tessellation, Symmetry Mosaic
surface
A surface, as the term is most generally used, is the outermost or uppermost layer of a physical object or space. It is the portion or region of the object that can first be perceived by an observer using the senses of sight and touch, and is t ...
, often a plane
Plane(s) most often refers to:
* Aero- or airplane, a powered, fixed-wing aircraft
* Plane (geometry), a flat, 2-dimensional surface
Plane or planes may also refer to:
Biology
* Plane (tree) or ''Platanus'', wetland native plant
* ''Planes' ...
, using one or more geometric shape
A shape or figure is a graphical representation of an object or its external boundary, outline, or external surface, as opposed to other properties such as color, texture, or material type.
A plane shape or plane figure is constrained to lie on ...
s, called ''tiles'', with no overlaps and no gaps. In mathematics
Mathematics is an area of knowledge that includes the topics of numbers, formulas and related structures, shapes and the spaces in which they are contained, and quantities and their changes. These topics are represented in modern mathematics ...
, tessellation can be generalized to higher dimensions
In physics and mathematics, the dimension of a mathematical space (or object) is informally defined as the minimum number of coordinates needed to specify any point within it. Thus, a line has a dimension of one (1D) because only one coord ...
and a variety of geometries.
A periodic tiling has a repeating pattern. Some special kinds include ''regular tilings
This article lists the regular polytopes and regular polytope compounds in Euclidean geometry, Euclidean, spherical geometry, spherical and hyperbolic geometry, hyperbolic spaces.
The Schläfli symbol describes every regular tessellation of an ' ...
'' with regular polygon
In Euclidean geometry, a regular polygon is a polygon that is Equiangular polygon, direct equiangular (all angles are equal in measure) and Equilateral polygon, equilateral (all sides have the same length). Regular polygons may be either convex p ...
al tiles all of the same shape, and ''semiregular tiling
Euclidean plane tilings by convex regular polygons have been widely used since antiquity. The first systematic mathematical treatment was that of Kepler in his ''Harmonices Mundi'' (Latin: ''The Harmony of the World'', 1619).
Notation of Eucli ...
s'' with regular tiles of more than one shape and with every corner identically arranged. The patterns formed by periodic tilings can be categorized into 17 wallpaper groups
A wallpaper is a mathematical object covering a whole Euclidean plane by repeating a motif indefinitely, in manner that certain isometries keep the drawing unchanged. To a given wallpaper there corresponds a group of such congruent transformatio ...
. A tiling that lacks a repeating pattern is called "non-periodic". An ''aperiodic tiling
An aperiodic tiling is a non-periodic tiling with the additional property that it does not contain arbitrarily large periodic regions or patches. A set of tile-types (or prototiles) is aperiodic if copies of these tiles can form only non- period ...
'' uses a small set of tile shapes that cannot form a repeating pattern. A ''tessellation of space
In geometry, a honeycomb is a ''space filling'' or ''close packing'' of polyhedral or higher-dimensional ''cells'', so that there are no gaps. It is an example of the more general mathematical ''tiling'' or ''tessellation'' in any number of dime ...
'', also known as a space filling or honeycomb, can be defined in the geometry of higher dimensions.
A real physical tessellation is a tiling made of materials such as cement
A cement is a binder, a chemical substance used for construction that sets, hardens, and adheres to other materials to bind them together. Cement is seldom used on its own, but rather to bind sand and gravel ( aggregate) together. Cement mix ...
ed ceramic
A ceramic is any of the various hard, brittle, heat-resistant and corrosion-resistant materials made by shaping and then firing an inorganic, nonmetallic material, such as clay, at a high temperature. Common examples are earthenware, porcelain ...
squares or hexagons. Such tilings may be decorative patterns
A pattern is a regularity in the world, in human-made design, or in abstract ideas. As such, the elements of a pattern repeat in a predictable manner. A geometric pattern is a kind of pattern formed of geometric shapes and typically repeated l ...
, or may have functions such as providing durable and water-resistant pavement, floor or wall coverings. Historically, tessellations were used in Ancient Rome
In modern historiography, ancient Rome refers to Roman civilisation from the founding of the city of Rome in the 8th century BC to the collapse of the Western Roman Empire in the 5th century AD. It encompasses the Roman Kingdom (753–509 B ...
and in Islamic art
Islamic art is a part of Islamic culture and encompasses the visual arts produced since the 7th century CE by people who lived within territories inhabited or ruled by Muslim populations. Referring to characteristic traditions across a wide ra ...
such as in the Moroccan architecture
Moroccan architecture refers to the architecture characteristic of Morocco throughout its history and up to modern times. The country's diverse geography and long history, marked by successive waves of settlers through both migration and military ...
and decorative geometric tiling of the Alhambra
The Alhambra (, ; ar, الْحَمْرَاء, Al-Ḥamrāʾ, , ) is a palace and fortress complex located in Granada, Andalusia, Spain. It is one of the most famous monuments of Islamic architecture and one of the best-preserved palaces of the ...
palace. In the twentieth century, the work of M. C. Escher
Maurits Cornelis Escher (; 17 June 1898 – 27 March 1972) was a Dutch graphic artist who made mathematically inspired woodcuts, lithographs, and mezzotints.
Despite wide popular interest, Escher was for most of his life neglected in t ...
often made use of tessellations, both in ordinary Euclidean geometry
Euclidean geometry is a mathematical system attributed to ancient Greek mathematics, Greek mathematician Euclid, which he described in his textbook on geometry: the ''Euclid's Elements, Elements''. Euclid's approach consists in assuming a small ...
and in hyperbolic geometry
In mathematics, hyperbolic geometry (also called Lobachevskian geometry or Bolyai– Lobachevskian geometry) is a non-Euclidean geometry. The parallel postulate of Euclidean geometry is replaced with:
:For any given line ''R'' and point ''P'' ...
, for artistic effect. Tessellations are sometimes employed for decorative effect in quilting
Quilting is the term given to the process of joining a minimum of three layers of fabric together either through stitching manually using a needle and thread, or mechanically with a sewing machine or specialised longarm quilting system. A ...
. Tessellations form a class of patterns in nature
Patterns in nature are visible regularities of form found in the natural world. These patterns recur in different contexts and can sometimes be modelled mathematically. Natural patterns include symmetries, trees, spirals, meanders, waves, foa ...
, for example in the arrays of hexagonal cells found in honeycomb
A honeycomb is a mass of Triangular prismatic honeycomb#Hexagonal prismatic honeycomb, hexagonal prismatic Beeswax, wax cells built by honey bees in their beehive, nests to contain their larvae and stores of honey and pollen.
beekeeping, Beekee ...
s.
History
Sumerians
Sumer () is the earliest known civilization in the historical region of southern Mesopotamia (south-central Iraq), emerging during the Chalcolithic and early Bronze Ages between the sixth and fifth millennium BC. It is one of the cradles of c ...
(about 4000 BC) in building wall decorations formed by patterns of clay tiles.
Decorative mosaic
A mosaic is a pattern or image made of small regular or irregular pieces of colored stone, glass or ceramic, held in place by plaster/mortar, and covering a surface. Mosaics are often used as floor and wall decoration, and were particularly pop ...
tilings made of small squared blocks called tessera
A tessera (plural: tesserae, diminutive ''tessella'') is an individual tile, usually formed in the shape of a square, used in creating a mosaic. It is also known as an abaciscus or abaculus.
Historical tesserae
The oldest known tesserae ...
e were widely employed in classical antiquity
Classical antiquity (also the classical era, classical period or classical age) is the period of cultural history between the 8th century BC and the 5th century AD centred on the Mediterranean Sea, comprising the interlocking civilizations of ...
, sometimes displaying geometric patterns.
In 1619 Johannes Kepler
Johannes Kepler (; ; 27 December 1571 – 15 November 1630) was a German astronomer, mathematician, astrologer, natural philosopher and writer on music. He is a key figure in the 17th-century Scientific Revolution, best known for his laws ...
made an early documented study of tessellations. He wrote about regular and semiregular tessellations in his ''Harmonices Mundi
''Harmonice Mundi (Harmonices mundi libri V)''The full title is ''Ioannis Keppleri Harmonices mundi libri V'' (''The Five Books of Johannes Kepler's The Harmony of the World''). (Latin: ''The Harmony of the World'', 1619) is a book by Johannes ...
''; he was possibly the first to explore and to explain the hexagonal structures of honeycomb and snowflakes
A snowflake is a single ice crystal that has achieved a sufficient size, and may have amalgamated with others, which falls through the Earth's atmosphere as snow.Knight, C.; Knight, N. (1973). Snow crystals. Scientific American, vol. 228, no. ...
.
 Some two hundred years later in 1891, the Russian crystallographer Yevgraf Fyodorov proved that every periodic tiling of the plane features one of seventeen different groups of isometries. Fyodorov's work marked the unofficial beginning of the mathematical study of tessellations. Other prominent contributors include
Some two hundred years later in 1891, the Russian crystallographer Yevgraf Fyodorov proved that every periodic tiling of the plane features one of seventeen different groups of isometries. Fyodorov's work marked the unofficial beginning of the mathematical study of tessellations. Other prominent contributors include Alexei Vasilievich Shubnikov
Alexei Vasilievich Shubnikov (russian: Алексей Васильевич Шубников; 29March 1887 – 27April 1970) was a Soviet crystallographer and mathematician. Shubnikov was the founding director of the Institute of Crystallograph ...
and Nikolai Belov (1964), and Heinrich Heesch
Heinrich Heesch (June 25, 1906 – July 26, 1995) was a German mathematician. He was born in Kiel and died in Hanover.
In Göttingen he worked on Group theory. In 1933 Heesch witnessed the National Socialist purges of university staff. Not wi ...
and Otto Kienzle (1963).
Etymology
In Latin, ''tessella'' is a small cubical piece ofclay
Clay is a type of fine-grained natural soil material containing clay minerals (hydrous aluminium phyllosilicates, e.g. kaolin, Al2 Si2 O5( OH)4).
Clays develop plasticity when wet, due to a molecular film of water surrounding the clay par ...
, stone
In geology, rock (or stone) is any naturally occurring solid mass or aggregate of minerals or mineraloid matter. It is categorized by the minerals included, its Chemical compound, chemical composition, and the way in which it is formed. Rocks ...
or glass
Glass is a non-crystalline, often transparent, amorphous solid that has widespread practical, technological, and decorative use in, for example, window panes, tableware, and optics. Glass is most often formed by rapid cooling (quenching) of ...
used to make mosaics. The word "tessella" means "small square" (from ''tessera'', square, which in turn is from the Greek word τέσσερα for ''four''). It corresponds to the everyday term ''tiling'', which refers to applications of tessellations, often made of glazed clay.
Overview
triangle
A triangle is a polygon with three Edge (geometry), edges and three Vertex (geometry), vertices. It is one of the basic shapes in geometry. A triangle with vertices ''A'', ''B'', and ''C'' is denoted \triangle ABC.
In Euclidean geometry, an ...
, square
In Euclidean geometry, a square is a regular quadrilateral, which means that it has four equal sides and four equal angles (90-degree angles, π/2 radian angles, or right angles). It can also be defined as a rectangle with two equal-length adj ...
and the regular hexagon
In geometry, a hexagon (from Ancient Greek, Greek , , meaning "six", and , , meaning "corner, angle") is a six-sided polygon. The total of the internal angles of any simple polygon, simple (non-self-intersecting) hexagon is 720°.
Regular hexa ...
. Any one of these three shapes can be duplicated infinitely to fill a plane
Plane(s) most often refers to:
* Aero- or airplane, a powered, fixed-wing aircraft
* Plane (geometry), a flat, 2-dimensional surface
Plane or planes may also refer to:
Biology
* Plane (tree) or ''Platanus'', wetland native plant
* ''Planes' ...
with no gaps.
Many other types of tessellation are possible under different constraints. For example, there are eight types of semi-regular tessellation, made with more than one kind of regular polygon but still having the same arrangement of polygons at every corner. Irregular tessellations can also be made from other shapes such as pentagons
In geometry, a pentagon (from the Greek language, Greek πέντε ''pente'' meaning ''five'' and γωνία ''gonia'' meaning ''angle'') is any five-sided polygon or 5-gon. The sum of the internal angles in a simple polygon, simple pentagon is ...
, polyominoes
A polyomino is a plane geometric figure formed by joining one or more equal squares edge to edge. It is a polyform whose cells are squares. It may be regarded as a finite subset of the regular square tiling.
Polyominoes have been used in po ...
and in fact almost any kind of geometric shape. The artist M. C. Escher
Maurits Cornelis Escher (; 17 June 1898 – 27 March 1972) was a Dutch graphic artist who made mathematically inspired woodcuts, lithographs, and mezzotints.
Despite wide popular interest, Escher was for most of his life neglected in t ...
is famous for making tessellations with irregular interlocking tiles, shaped like animals and other natural objects. If suitable contrasting colours are chosen for the tiles of differing shape, striking patterns are formed, and these can be used to decorate physical surfaces such as church floors.
 More formally, a tessellation or tiling is a
More formally, a tessellation or tiling is a cover
Cover or covers may refer to:
Packaging
* Another name for a lid
* Cover (philately), generic term for envelope or package
* Album cover, the front of the packaging
* Book cover or magazine cover
** Book design
** Back cover copy, part of co ...
of the Euclidean plane by a countable
In mathematics, a set is countable if either it is finite or it can be made in one to one correspondence with the set of natural numbers. Equivalently, a set is ''countable'' if there exists an injective function from it into the natural numbers; ...
number of closed sets, called ''tiles'', such that the tiles intersect only on their boundaries. These tiles may be polygons or any other shapes. Many tessellations are formed from a finite number of prototile
In the mathematical theory of tessellations, a prototile is one of the shapes of a tile in a tessellation.
Definition
A tessellation of the plane or of any other space is a cover of the space by closed shapes, called tiles, that have disjoint in ...
s in which all tiles in the tessellation are congruent
Congruence may refer to:
Mathematics
* Congruence (geometry), being the same size and shape
* Congruence or congruence relation, in abstract algebra, an equivalence relation on an algebraic structure that is compatible with the structure
* In mod ...
to the given prototiles. If a geometric shape can be used as a prototile to create a tessellation, the shape is said to ''tessellate'' or to ''tile the plane''. The Conway criterion
In the mathematical theory of tessellations, the Conway criterion, named for the English mathematician John Horton Conway, is a sufficient rule for when a prototile will tile the plane. It consists of the following requirements:Will It Tile? Try ...
is a sufficient but not necessary set of rules for deciding if a given shape tiles the plane periodically without reflections: some tiles fail the criterion but still tile the plane. No general rule has been found for determining if a given shape can tile the plane or not, which means there are many unsolved problems concerning tessellations.
Mathematically, tessellations can be extended to spaces other than the Euclidean plane. The Swiss
Swiss may refer to:
* the adjectival form of Switzerland
* Swiss people
Places
* Swiss, Missouri
* Swiss, North Carolina
*Swiss, West Virginia
* Swiss, Wisconsin
Other uses
*Swiss-system tournament, in various games and sports
*Swiss Internation ...
geometer
A geometer is a mathematician whose area of study is geometry.
Some notable geometers and their main fields of work, chronologically listed, are:
1000 BCE to 1 BCE
* Baudhayana (fl. c. 800 BC) – Euclidean geometry, geometric algebra
* ...
Ludwig Schläfli
Ludwig Schläfli (15 January 1814 – 20 March 1895) was a Swiss mathematician, specialising in geometry and complex analysis (at the time called function theory) who was one of the key figures in developing the notion of higher-dimensional space ...
pioneered this by defining ''polyschemes'', which mathematicians nowadays call polytopes
In elementary geometry, a polytope is a geometric object with flat sides (''faces''). Polytopes are the generalization of three-dimensional polyhedra to any number of dimensions. Polytopes may exist in any general number of dimensions as an - ...
. These are the analogues to polygons and polyhedra
In geometry, a polyhedron (plural polyhedra or polyhedrons; ) is a three-dimensional shape with flat polygonal faces, straight edges and sharp corners or vertices.
A convex polyhedron is the convex hull of finitely many points, not all on t ...
in spaces with more dimensions. He further defined the Schläfli symbol
In geometry, the Schläfli symbol is a notation of the form \ that defines regular polytopes and tessellations.
The Schläfli symbol is named after the 19th-century Swiss mathematician Ludwig Schläfli, who generalized Euclidean geometry to more ...
notation to make it easy to describe polytopes. For example, the Schläfli symbol for an equilateral triangle is , while that for a square is . The Schläfli notation makes it possible to describe tilings compactly. For example, a tiling of regular hexagons has three six-sided polygons at each vertex, so its Schläfli symbol is .
Other methods also exist for describing polygonal tilings. When the tessellation is made of regular polygons, the most common notation is the vertex configuration
In geometry, a vertex configurationCrystallography ...
, which is simply a list of the number of sides of the polygons around a vertex. The square tiling has a vertex configuration of 4.4.4.4, or 44. The tiling of regular hexagons is noted 6.6.6, or 63.
In mathematics
Introduction to tessellations
Mathematicians use some technical terms when discussing tilings. An ''edge
Edge or EDGE may refer to:
Technology Computing
* Edge computing, a network load-balancing system
* Edge device, an entry point to a computer network
* Adobe Edge, a graphical development application
* Microsoft Edge, a web browser developed by ...
'' is the intersection between two bordering tiles; it is often a straight line. A ''vertex
Vertex, vertices or vertexes may refer to:
Science and technology Mathematics and computer science
*Vertex (geometry), a point where two or more curves, lines, or edges meet
*Vertex (computer graphics), a data structure that describes the position ...
'' is the point of intersection of three or more bordering tiles. Using these terms, an ''isogonal'' or vertex-transitive
In geometry, a polytope (e.g. a polygon or polyhedron) or a tiling is isogonal or vertex-transitive if all its vertices are equivalent under the symmetries of the figure. This implies that each vertex is surrounded by the same kinds of face in ...
tiling is a tiling where every vertex point is identical; that is, the arrangement of polygon
In geometry, a polygon () is a plane figure that is described by a finite number of straight line segments connected to form a closed ''polygonal chain'' (or ''polygonal circuit''). The bounded plane region, the bounding circuit, or the two toge ...
s about each vertex is the same. The fundamental region
Given a topological space and a group acting on it, the images of a single point under the group action form an orbit of the action. A fundamental domain or fundamental region is a subset of the space which contains exactly one point from each o ...
is a shape such as a rectangle that is repeated to form the tessellation. For example, a regular tessellation of the plane with squares has a meeting of four squares at every vertex.
The sides of the polygons are not necessarily identical to the edges of the tiles. An edge-to-edge tiling is any polygonal tessellation where adjacent tiles only share one full side, i.e., no tile shares a partial side or more than one side with any other tile. In an edge-to-edge tiling, the sides of the polygons and the edges of the tiles are the same. The familiar "brick wall" tiling is not edge-to-edge because the long side of each rectangular brick is shared with two bordering bricks.
A ''normal tiling'' is a tessellation for which every tile is topologically
In mathematics, topology (from the Greek words , and ) is concerned with the properties of a geometric object that are preserved under continuous deformations, such as stretching, twisting, crumpling, and bending; that is, without closing ho ...
equivalent to a disk
Disk or disc may refer to:
* Disk (mathematics), a geometric shape
* Disk storage
Music
* Disc (band), an American experimental music band
* ''Disk'' (album), a 1995 EP by Moby
Other uses
* Disk (functional analysis), a subset of a vector sp ...
, the intersection of any two tiles is a connected set
In topology and related branches of mathematics, a connected space is a topological space that cannot be represented as the union of two or more disjoint non-empty open subsets. Connectedness is one of the principal topological properties that ...
or the empty set
In mathematics, the empty set is the unique set having no elements; its size or cardinality (count of elements in a set) is zero. Some axiomatic set theories ensure that the empty set exists by including an axiom of empty set, while in other ...
, and all tiles are uniformly bounded
In mathematics, a uniformly bounded family of functions is a family of bounded functions that can all be bounded by the same constant. This constant is larger than or equal to the absolute value of any value of any of the functions in the family.
...
. This means that a single circumscribing radius and a single inscribing radius can be used for all the tiles in the whole tiling; the condition disallows tiles that are pathologically long or thin.
 A is a tessellation in which all tiles are
A is a tessellation in which all tiles are congruent
Congruence may refer to:
Mathematics
* Congruence (geometry), being the same size and shape
* Congruence or congruence relation, in abstract algebra, an equivalence relation on an algebraic structure that is compatible with the structure
* In mod ...
; it has only one prototile. A particularly interesting type of monohedral tessellation is the spiral monohedral tiling. The first spiral monohedral tiling was discovered by Heinz Voderberg in 1936; the Voderberg tiling has a unit tile that is a nonconvex enneagon
In geometry, a nonagon () or enneagon () is a nine-sided polygon or 9-gon.
The name ''nonagon'' is a prefix hybrid formation, from Latin (''nonus'', "ninth" + ''gonon''), used equivalently, attested already in the 16th century in French ''nonogo ...
. The Hirschhorn tiling, published by Michael D. Hirschhorn and D. C. Hunt in 1985, is a pentagon tiling
In geometry, a pentagonal tiling is a tiling of the plane where each individual piece is in the shape of a pentagon.
A regular tiling, regular pentagonal tiling on the Euclidean plane is impossible because the internal angle of a Pentagon#Regular ...
using irregular pentagons: regular pentagons cannot tile the Euclidean plane as the internal angle
In geometry, an angle of a polygon is formed by two sides of the polygon that share an endpoint. For a simple (non-self-intersecting) polygon, regardless of whether it is convex or non-convex, this angle is called an interior angle (or ) if ...
of a regular pentagon, , is not a divisor of 2.
An isohedral tiling is a special variation of a monohedral tiling in which all tiles belong to the same transitivity class, that is, all tiles are transforms of the same prototile under the symmetry
Symmetry (from grc, συμμετρία "agreement in dimensions, due proportion, arrangement") in everyday language refers to a sense of harmonious and beautiful proportion and balance. In mathematics, "symmetry" has a more precise definit ...
group of the tiling. If a prototile admits a tiling, but no such tiling is isohedral, then the prototile is called anisohedral and forms anisohedral tiling
In geometry, a shape is said to be anisohedral if it admits a tiling, but no such tiling is isohedral (tile-transitive); that is, in any tiling by that shape there are two tiles that are not equivalent under any symmetry of the tiling. A tiling by ...
s.
A regular tessellation is a highly symmetric
Symmetry (from grc, συμμετρία "agreement in dimensions, due proportion, arrangement") in everyday language refers to a sense of harmonious and beautiful proportion and balance. In mathematics, "symmetry" has a more precise definiti ...
, edge-to-edge tiling made up of regular polygon
In Euclidean geometry, a regular polygon is a polygon that is Equiangular polygon, direct equiangular (all angles are equal in measure) and Equilateral polygon, equilateral (all sides have the same length). Regular polygons may be either convex p ...
s, all of the same shape. There are only three regular tessellations: those made up of equilateral triangle
In geometry, an equilateral triangle is a triangle in which all three sides have the same length. In the familiar Euclidean geometry, an equilateral triangle is also equiangular; that is, all three internal angles are also congruent to each othe ...
s, squares
In Euclidean geometry, a square is a regular quadrilateral, which means that it has four equal sides and four equal angles (90- degree angles, π/2 radian angles, or right angles). It can also be defined as a rectangle with two equal-length a ...
, or regular hexagon
In geometry, a hexagon (from Ancient Greek, Greek , , meaning "six", and , , meaning "corner, angle") is a six-sided polygon. The total of the internal angles of any simple polygon, simple (non-self-intersecting) hexagon is 720°.
Regular hexa ...
s. All three of these tilings are isogonal and monohedral.
 A semi-regular (or Archimedean) tessellation uses more than one type of regular polygon in an isogonal arrangement. There are eight semi-regular tilings (or nine if the mirror-image pair of tilings counts as two). These can be described by their
A semi-regular (or Archimedean) tessellation uses more than one type of regular polygon in an isogonal arrangement. There are eight semi-regular tilings (or nine if the mirror-image pair of tilings counts as two). These can be described by their vertex configuration
In geometry, a vertex configurationCrystallography ...
; for example, a semi-regular tiling using squares and regular octagons has the vertex configuration 4.82 (each vertex has one square and two octagons). Many non-edge-to-edge tilings of the Euclidean plane are possible, including the family of Pythagorean tiling
A Pythagorean tiling or two squares tessellation is a tiling of a Euclidean plane by squares of two different sizes, in which each square touches four squares of the other size on its four sides. Many proofs of the Pythagorean theorem are ...
s, tessellations that use two (parameterised) sizes of square, each square touching four squares of the other size. An edge tessellation
In geometry, an edge tessellation is a partition of the plane into non-overlapping polygons (a tessellation) with the property that the reflection of any of these polygons across any of its edges is another polygon in the tessellation.
All of the r ...
is one in which each tile can be reflected over an edge to take up the position of a neighbouring tile, such as in an array of equilateral or isosceles triangles.
Wallpaper groups
 Tilings with
Tilings with translational symmetry
In geometry, to translate a geometric figure is to move it from one place to another without rotating it. A translation "slides" a thing by .
In physics and mathematics, continuous translational symmetry is the invariance of a system of equatio ...
in two independent directions can be categorized by wallpaper group
A wallpaper is a mathematical object covering a whole Euclidean plane by repeating a motif indefinitely, in manner that certain isometries keep the drawing unchanged. To a given wallpaper there corresponds a group of such congruent transformatio ...
s, of which 17 exist. It has been claimed that all seventeen of these groups are represented in the Alhambra
The Alhambra (, ; ar, الْحَمْرَاء, Al-Ḥamrāʾ, , ) is a palace and fortress complex located in Granada, Andalusia, Spain. It is one of the most famous monuments of Islamic architecture and one of the best-preserved palaces of the ...
palace in Granada
Granada (,, DIN 31635, DIN: ; grc, Ἐλιβύργη, Elibýrgē; la, Illiberis or . ) is the capital city of the province of Granada, in the autonomous communities of Spain, autonomous community of Andalusia, Spain. Granada is located at the fo ...
, Spain
, image_flag = Bandera de España.svg
, image_coat = Escudo de España (mazonado).svg
, national_motto = ''Plus ultra'' (Latin)(English: "Further Beyond")
, national_anthem = (English: "Royal March")
, i ...
. Though this is disputed, the variety and sophistication of the Alhambra tilings have surprised modern researchers. Of the three regular tilings two are in the ''p6m'' wallpaper group and one is in ''p4m''. Tilings in 2D with translational symmetry in just one direction can be categorized by the seven frieze groups describing the possible frieze pattern
In mathematics, a frieze or frieze pattern is a two-dimensional design that repeats in one direction. Such patterns occur frequently in architecture and decorative art. Frieze patterns can be classified into seven types according to their symmetri ...
s. Orbifold notation
In geometry, orbifold notation (or orbifold signature) is a system, invented by the mathematician William Thurston and promoted by John Conway, for representing types of symmetry groups in two-dimensional spaces of constant curvature. The advanta ...
can be used to describe wallpaper groups of the Euclidean plane.
Aperiodic tilings
Penrose tiling
A Penrose tiling is an example of an aperiodic tiling. Here, a ''tiling'' is a covering of the plane by non-overlapping polygons or other shapes, and ''aperiodic'' means that shifting any tiling with these shapes by any finite distance, without r ...
s, which use two different quadrilateral prototiles, are the best known example of tiles that forcibly create non-periodic patterns. They belong to a general class of aperiodic tiling
An aperiodic tiling is a non-periodic tiling with the additional property that it does not contain arbitrarily large periodic regions or patches. A set of tile-types (or prototiles) is aperiodic if copies of these tiles can form only non- period ...
s, which use tiles that cannot tessellate periodically. The recursive process of substitution tiling In geometry, a tile substitution is a method for constructing highly ordered tilings. Most importantly, some tile substitutions generate aperiodic tilings, which are tilings whose prototiles do not admit any tiling with translational symmetry. The ...
is a method of generating aperiodic tilings. One class that can be generated in this way is the rep-tile
In the geometry of tessellations, a rep-tile or reptile is a shape that can be dissected into smaller copies of the same shape. The term was coined as a pun on animal reptiles by recreational mathematician Solomon W. Golomb and popularized by ...
s; these tilings have surprising self-replicating
Self-replication is any behavior of a dynamical system that yields construction of an identical or similar copy of itself. Biological cells, given suitable environments, reproduce by cell division. During cell division, DNA is replicated and ca ...
properties. Pinwheel tiling
In geometry, pinwheel tilings are non-periodic tilings defined by Charles Radin and based on a construction due to John Conway.
They are the first known non-periodic tilings to each have the property that their tiles appear in infinitely many or ...
s are non-periodic, using a rep-tile construction; the tiles appear in infinitely many orientations. It might be thought that a non-periodic pattern would be entirely without symmetry, but this is not so. Aperiodic tilings, while lacking in translational symmetry
In geometry, to translate a geometric figure is to move it from one place to another without rotating it. A translation "slides" a thing by .
In physics and mathematics, continuous translational symmetry is the invariance of a system of equatio ...
, do have symmetries of other types, by infinite repetition of any bounded patch of the tiling and in certain finite groups of rotations or reflections of those patches. A substitution rule, such as can be used to generate some Penrose patterns using assemblies of tiles called rhombs, illustrates scaling symmetry. A Fibonacci word
A Fibonacci word is a specific sequence of binary digits (or symbols from any two-letter alphabet). The Fibonacci word is formed by repeated concatenation in the same way that the Fibonacci numbers are formed by repeated addition.
It is a para ...
can be used to build an aperiodic tiling, and to study quasicrystal
A quasiperiodic crystal, or quasicrystal, is a structure that is ordered but not periodic. A quasicrystalline pattern can continuously fill all available space, but it lacks translational symmetry. While crystals, according to the classical cr ...
s, which are structures with aperiodic order.
Wang tile
Wang tiles (or Wang dominoes), first proposed by mathematician, logician, and philosopher Hao Wang in 1961, are a class of formal systems. They are modelled visually by square tiles with a color on each side. A set of such tiles is selected, an ...
s are squares coloured on each edge, and placed so that abutting edges of adjacent tiles have the same colour; hence they are sometimes called Wang dominoes
Dominoes is a family of tile-based games played with gaming pieces, commonly known as dominoes. Each domino is a rectangular tile, usually with a line dividing its face into two square ''ends''. Each end is marked with a number of spots (also ca ...
. A suitable set of Wang dominoes can tile the plane, but only aperiodically. This is known because any Turing machine
A Turing machine is a mathematical model of computation describing an abstract machine that manipulates symbols on a strip of tape according to a table of rules. Despite the model's simplicity, it is capable of implementing any computer algori ...
can be represented as a set of Wang dominoes that tile the plane if and only if the Turing machine does not halt. Since the halting problem
In computability theory, the halting problem is the problem of determining, from a description of an arbitrary computer program and an input, whether the program will finish running, or continue to run forever. Alan Turing proved in 1936 that a g ...
is undecidable, the problem of deciding whether a Wang domino set can tile the plane is also undecidable.
rotational symmetry
Rotational symmetry, also known as radial symmetry in geometry, is the property a shape has when it looks the same after some rotation by a partial turn. An object's degree of rotational symmetry is the number of distinct orientations in which i ...
; in 1704, Sébastien Truchet
Jean Truchet (1657 – 5 February 1729), known as Father Sébastian, was a French Dominican priest born in Lyon, who lived under the reign of Louis XIV. He was active in areas such as mathematics, hydraulics, graphics, typography, and for ...
used a square tile split into two triangles of contrasting colours. These can tile the plane either periodically or randomly.
Tessellations and colour
four colour theorem
In mathematics, the four color theorem, or the four color map theorem, states that no more than four colors are required to color the regions of any map so that no two adjacent regions have the same color. ''Adjacent'' means that two regions sha ...
states that for every tessellation of a normal Euclidean plane
In mathematics, the Euclidean plane is a Euclidean space of dimension two. That is, a geometric setting in which two real quantities are required to determine the position of each point ( element of the plane), which includes affine notions of ...
, with a set of four available colours, each tile can be coloured in one colour such that no tiles of equal colour meet at a curve of positive length. The colouring guaranteed by the four colour theorem does not generally respect the symmetries of the tessellation. To produce a colouring which does, it is necessary to treat the colours as part of the tessellation. Here, as many as seven colours may be needed, as in the picture at right.
Tessellations with polygons
quadrilateral
In geometry a quadrilateral is a four-sided polygon, having four edges (sides) and four corners (vertices). The word is derived from the Latin words ''quadri'', a variant of four, and ''latus'', meaning "side". It is also called a tetragon, ...
(even non-convex) can be used as a prototile to form a monohedral tessellation, often in more than one way. Copies of an arbitrary quadrilateral
In geometry a quadrilateral is a four-sided polygon, having four edges (sides) and four corners (vertices). The word is derived from the Latin words ''quadri'', a variant of four, and ''latus'', meaning "side". It is also called a tetragon, ...
can form a tessellation with translational symmetry and 2-fold rotational symmetry with centres at the midpoints of all sides. For an asymmetric quadrilateral this tiling belongs to wallpaper group p2. As fundamental domain
Given a topological space and a group acting on it, the images of a single point under the group action form an orbit of the action. A fundamental domain or fundamental region is a subset of the space which contains exactly one point from each o ...
we have the quadrilateral. Equivalently, we can construct a parallelogram
In Euclidean geometry, a parallelogram is a simple (non- self-intersecting) quadrilateral with two pairs of parallel sides. The opposite or facing sides of a parallelogram are of equal length and the opposite angles of a parallelogram are of equa ...
subtended by a minimal set of translation vectors, starting from a rotational centre. We can divide this by one diagonal, and take one half (a triangle) as fundamental domain. Such a triangle has the same area as the quadrilateral and can be constructed from it by cutting and pasting.
If only one shape of tile is allowed, tilings exists with convex ''N''-gons for ''N'' equal to 3, 4, 5 and 6. For , see Pentagonal tiling
In geometry, a pentagonal tiling is a tiling of the plane where each individual piece is in the shape of a pentagon.
A regular pentagonal tiling on the Euclidean plane is impossible because the internal angle of a regular pentagon, 108°, is not ...
, for , see Hexagonal tiling
In geometry, the hexagonal tiling or hexagonal tessellation is a regular tiling of the Euclidean plane, in which exactly three hexagons meet at each vertex. It has Schläfli symbol of or (as a truncated triangular tiling).
English mathemat ...
, for , see Heptagonal tiling
In geometry, a heptagonal tiling is a regular tiling of the hyperbolic plane. It is represented by Schläfli symbol of , having three regular heptagons around each vertex.
Images
Related polyhedra and tilings
This tiling is topologically r ...
and for , see octagonal tiling
In geometry, the octagonal tiling is a regular tiling of the hyperbolic plane. It is represented by Schläfli symbol of ', having three regular octagons around each vertex. It also has a construction as a truncated order-8 square tiling, t.
U ...
.
For results on tiling the plane with polyomino
A polyomino is a plane geometric figure formed by joining one or more equal squares edge to edge. It is a polyform whose cells are squares. It may be regarded as a finite subset of the regular square tiling.
Polyominoes have been used in pop ...
es, see Polyomino § Uses of polyominoes.
Voronoi tilings
Voronoi or Dirichlet tilings are tessellations where each tile is defined as the set of points closest to one of the points in a discrete set of defining points. (Think of geographical regions where each region is defined as all the points closest to a given city or post office.) The ''Voronoi cell'' for each defining point is a convex polygon. TheDelaunay triangulation
In mathematics and computational geometry, a Delaunay triangulation (also known as a Delone triangulation) for a given set P of discrete points in a general position is a triangulation DT(P) such that no point in P is inside the circumcircle o ...
is a tessellation that is the dual graph
In the mathematical discipline of graph theory, the dual graph of a plane graph is a graph that has a vertex for each face of . The dual graph has an edge for each pair of faces in that are separated from each other by an edge, and a self-loop ...
of a Voronoi tessellation. Delaunay triangulations are useful in numerical simulation, in part because among all possible triangulations of the defining points, Delaunay triangulations maximize the minimum of the angles formed by the edges. Voronoi tilings with randomly placed points can be used to construct random tilings of the plane.
Tessellations in higher dimensions
 Tessellation can be extended to three dimensions. Certain
Tessellation can be extended to three dimensions. Certain polyhedra
In geometry, a polyhedron (plural polyhedra or polyhedrons; ) is a three-dimensional shape with flat polygonal faces, straight edges and sharp corners or vertices.
A convex polyhedron is the convex hull of finitely many points, not all on t ...
can be stacked in a regular crystal pattern to fill (or tile) three-dimensional space, including the cube
In geometry, a cube is a three-dimensional solid object bounded by six square faces, facets or sides, with three meeting at each vertex. Viewed from a corner it is a hexagon and its net is usually depicted as a cross.
The cube is the only r ...
(the only Platonic polyhedron to do so), the rhombic dodecahedron
In geometry, the rhombic dodecahedron is a convex polyhedron with 12 congruent rhombic faces. It has 24 edges, and 14 vertices of 2 types. It is a Catalan solid, and the dual polyhedron of the cuboctahedron.
Properties
The rhombic dodecahedro ...
, the truncated octahedron
In geometry, the truncated octahedron is the Archimedean solid that arises from a regular octahedron by removing six pyramids, one at each of the octahedron's vertices. The truncated octahedron has 14 faces (8 regular hexagon, hexagons and 6 Squa ...
, and triangular, quadrilateral, and hexagonal prisms, among others. Any polyhedron that fits this criterion is known as a plesiohedron
In geometry, a plesiohedron is a special kind of space-filling polyhedron, defined as the Voronoi cell of a symmetric Delone set.
Three-dimensional Euclidean space can be completely filled by copies of any one of these shapes, with no overlaps. The ...
, and may possess between 4 and 38 faces. Naturally occurring rhombic dodecahedra are found as crystal
A crystal or crystalline solid is a solid material whose constituents (such as atoms, molecules, or ions) are arranged in a highly ordered microscopic structure, forming a crystal lattice that extends in all directions. In addition, macros ...
s of andradite
Andradite is a mineral species of the garnet group. It is a nesosilicate, with formula Ca3Fe2Si3O12.
Andradite includes three varieties:
* ''Melanite'': Black in color, referred to as "titanian andradite".garnet
Garnets () are a group of silicate minerals that have been used since the Bronze Age as gemstones and abrasives.
All species of garnets possess similar physical properties and crystal forms, but differ in chemical composition. The different s ...
) and fluorite
Fluorite (also called fluorspar) is the mineral form of calcium fluoride, CaF2. It belongs to the halide minerals. It crystallizes in isometric cubic habit, although octahedral and more complex isometric forms are not uncommon.
The Mohs sca ...
.
tetrahedra
In geometry, a tetrahedron (plural: tetrahedra or tetrahedrons), also known as a triangular pyramid, is a polyhedron composed of four triangular faces, six straight edges, and four vertex corners. The tetrahedron is the simplest of all the o ...
and six octahedra
In geometry, an octahedron (plural: octahedra, octahedrons) is a polyhedron with eight faces. The term is most commonly used to refer to the regular octahedron, a Platonic solid composed of eight equilateral triangles, four of which meet at ea ...
at each polyhedron vertex. However, there are many possible semiregular honeycombs in three dimensions. Uniform honeycombs can be constructed using the Wythoff construction
In geometry, a Wythoff construction, named after mathematician Willem Abraham Wythoff, is a method for constructing a uniform polyhedron or plane tiling. It is often referred to as Wythoff's kaleidoscopic construction.
Construction process
...
.
The Schmitt-Conway biprism is a convex polyhedron with the property of tiling space only aperiodically.
A Schwarz triangle
In geometry, a Schwarz triangle, named after Hermann Schwarz, is a spherical triangle that can be used to tile a sphere (spherical tiling), possibly overlapping, through reflections in its edges. They were classified in .
These can be defined mor ...
is a spherical triangle
Spherical trigonometry is the branch of spherical geometry that deals with the metrical relationships between the sides and angles of spherical triangles, traditionally expressed using trigonometric functions. On the sphere, geodesics are grea ...
that can be used to tile a sphere
A sphere () is a Geometry, geometrical object that is a solid geometry, three-dimensional analogue to a two-dimensional circle. A sphere is the Locus (mathematics), set of points that are all at the same distance from a given point in three ...
.
Tessellations in non-Euclidean geometries
 It is possible to tessellate in
It is possible to tessellate in non-Euclidean
In mathematics, non-Euclidean geometry consists of two geometries based on axioms closely related to those that specify Euclidean geometry. As Euclidean geometry lies at the intersection of metric geometry and affine geometry, non-Euclidean geo ...
geometries such as hyperbolic geometry
In mathematics, hyperbolic geometry (also called Lobachevskian geometry or Bolyai– Lobachevskian geometry) is a non-Euclidean geometry. The parallel postulate of Euclidean geometry is replaced with:
:For any given line ''R'' and point ''P'' ...
. A uniform tiling in the hyperbolic plane (which may be regular, quasiregular or semiregular) is an edge-to-edge filling of the hyperbolic plane, with regular polygon
In Euclidean geometry, a regular polygon is a polygon that is Equiangular polygon, direct equiangular (all angles are equal in measure) and Equilateral polygon, equilateral (all sides have the same length). Regular polygons may be either convex p ...
s as faces
The face is the front of an animal's head that features the eyes, nose and mouth, and through which animals express many of their emotions. The face is crucial for human identity, and damage such as scarring or developmental deformities may affe ...
; these are vertex-transitive
In geometry, a polytope (e.g. a polygon or polyhedron) or a tiling is isogonal or vertex-transitive if all its vertices are equivalent under the symmetries of the figure. This implies that each vertex is surrounded by the same kinds of face in ...
( transitive on its vertices), and isogonal (there is an isometry
In mathematics, an isometry (or congruence, or congruent transformation) is a distance-preserving transformation between metric spaces, usually assumed to be bijective. The word isometry is derived from the Ancient Greek: ἴσος ''isos'' mea ...
mapping any vertex onto any other).
A uniform honeycomb in hyperbolic space is a uniform tessellation of uniform polyhedral cells
Cell most often refers to:
* Cell (biology), the functional basic unit of life
Cell may also refer to:
Locations
* Monastic cell, a small room, hut, or cave in which a religious recluse lives, alternatively the small precursor of a monastery w ...
. In 3-dimensional hyperbolic space there are nine Coxeter group
In mathematics, a Coxeter group, named after H. S. M. Coxeter, is an abstract group that admits a formal description in terms of reflections (or kaleidoscopic mirrors). Indeed, the finite Coxeter groups are precisely the finite Euclidean refl ...
families of compact convex uniform honeycomb
In geometry, a convex uniform honeycomb is a uniform polytope, uniform tessellation which fills three-dimensional Euclidean space with non-overlapping convex polyhedron, convex uniform polyhedron, uniform polyhedral cells.
Twenty-eight such honey ...
s, generated as Wythoff construction
In geometry, a Wythoff construction, named after mathematician Willem Abraham Wythoff, is a method for constructing a uniform polyhedron or plane tiling. It is often referred to as Wythoff's kaleidoscopic construction.
Construction process
...
s, and represented by permutation
In mathematics, a permutation of a set is, loosely speaking, an arrangement of its members into a sequence or linear order, or if the set is already ordered, a rearrangement of its elements. The word "permutation" also refers to the act or proc ...
s of rings
Ring may refer to:
* Ring (jewellery), a round band, usually made of metal, worn as ornamental jewelry
* To make a sound with a bell, and the sound made by a bell
:(hence) to initiate a telephone connection
Arts, entertainment and media Film and ...
of the Coxeter diagram
Harold Scott MacDonald "Donald" Coxeter, (9 February 1907 – 31 March 2003) was a British and later also Canadian geometer. He is regarded as one of the greatest geometers of the 20th century.
Biography
Coxeter was born in Kensington to ...
s for each family.
In art
 In architecture, tessellations have been used to create decorative motifs since ancient times.
In architecture, tessellations have been used to create decorative motifs since ancient times. Mosaic
A mosaic is a pattern or image made of small regular or irregular pieces of colored stone, glass or ceramic, held in place by plaster/mortar, and covering a surface. Mosaics are often used as floor and wall decoration, and were particularly pop ...
tilings often had geometric patterns. Later civilisations also used larger tiles, either plain or individually decorated. Some of the most decorative were the Moorish
The term Moor, derived from the ancient Mauri, is an exonym first used by Christian Europeans to designate the Muslim inhabitants of the Maghreb, the Iberian Peninsula, Sicily and Malta during the Middle Ages.
Moors are not a distinct or se ...
wall tilings of Islamic architecture
Islamic architecture comprises the architectural styles of buildings associated with Islam. It encompasses both secular and religious styles from the early history of Islam to the present day. The Islamic world encompasses a wide geographic ar ...
, using Girih
''Girih'' ( fa, گره, "knot", also written ''gereh'') are decorative Islamic geometric patterns used in architecture and handicraft objects, consisting of angled lines that form an interlaced strapwork pattern.
''Girih'' decoration is beli ...
and Zellige
''Zellij'' ( ar, الزليج, translit=zillīj; also spelled zillij or zellige) is a style of mosaic tilework made from individually hand-chiseled tile pieces. The pieces were typically of different colours and fitted together to form various pa ...
tiles in buildings such as the Alhambra
The Alhambra (, ; ar, الْحَمْرَاء, Al-Ḥamrāʾ, , ) is a palace and fortress complex located in Granada, Andalusia, Spain. It is one of the most famous monuments of Islamic architecture and one of the best-preserved palaces of the ...
and La Mezquita.
Tessellations frequently appeared in the graphic art of M. C. Escher
Maurits Cornelis Escher (; 17 June 1898 – 27 March 1972) was a Dutch graphic artist who made mathematically inspired woodcuts, lithographs, and mezzotints.
Despite wide popular interest, Escher was for most of his life neglected in t ...
; he was inspired by the Moorish use of symmetry in places such as the Alhambra when he visited Spain
, image_flag = Bandera de España.svg
, image_coat = Escudo de España (mazonado).svg
, national_motto = ''Plus ultra'' (Latin)(English: "Further Beyond")
, national_anthem = (English: "Royal March")
, i ...
in 1936. Escher made four " Circle Limit" drawings of tilings that use hyperbolic geometry. For his woodcut
Woodcut is a relief printing technique in printmaking. An artist carves an image into the surface of a block of wood—typically with gouges—leaving the printing parts level with the surface while removing the non-printing parts. Areas that ...
"Circle Limit IV" (1960), Escher prepared a pencil and ink study showing the required geometry. Escher explained that "No single component of all the series, which from infinitely far away rise like rockets perpendicularly from the limit and are at last lost in it, ever reaches the boundary line."
Tessellated designs often appear on textiles, whether woven, stitched in or printed. Tessellation patterns have been used to design interlocking motifs of patch shapes in quilt
A quilt is a multi-layered textile, traditionally composed of two or more layers of fabric or fiber. Commonly three layers are used with a filler material. These layers traditionally include a woven cloth top, a layer of batting or wadding, a ...
s.
Tessellations are also a main genre in origami
) is the Japanese paper art, art of paper folding. In modern usage, the word "origami" is often used as an inclusive term for all folding practices, regardless of their culture of origin. The goal is to transform a flat square sheet of pape ...
(paper folding), where pleats are used to connect molecules such as twist folds together in a repeating fashion.
In manufacturing
Tessellation is used inmanufacturing industry
Manufacturing is the creation or production of goods with the help of equipment, labor, machines, tools, and chemical or biological processing or formulation. It is the essence of secondary sector of the economy. The term may refer to a rang ...
to reduce the wastage of material (yield losses) such as sheet metal
Sheet metal is metal formed into thin, flat pieces, usually by an industrial process. Sheet metal is one of the fundamental forms used in metalworking, and it can be cut and bent into a variety of shapes.
Thicknesses can vary significantly; ex ...
when cutting out shapes for objects like car door
A car or automobile is a motor vehicle with wheels. Most definitions of ''cars'' say that they run primarily on roads, seat one to eight people, have four wheels, and mainly transport people instead of goods.
The year 1886 is regarded as t ...
s or drinks can
A drink can (or beverage can) is a metal container designed to hold a fixed portion of liquid such as carbonated soft drinks, alcoholic drinks, fruit juices, teas, herbal teas, energy drinks, etc. Drink cans are made of aluminum (75% of w ...
s.
Tessellation is apparent in the mudcrack
Mudcracks (also known as mud cracks, desiccation cracks or cracked mud) are sedimentary structures formed as muddy sediment dries and contracts.Jackson, J.A., 1997, ''Glossary of Geology'' (4th ed.), American Geological Institute, Alexandria, VA, ...
-like cracking of thin film
A thin film is a layer of material ranging from fractions of a nanometer (monolayer) to several micrometers in thickness. The controlled synthesis of materials as thin films (a process referred to as deposition) is a fundamental step in many ap ...
s – with a degree of self-organisation
Self-organization, also called spontaneous order in the social sciences, is a process where some form of overall order arises from local interactions between parts of an initially disordered system. The process can be spontaneous when suff ...
being observed using micro
Micro may refer to:
Measurement
* micro- (μ), a metric prefix denoting a factor of 10−6
Places
* Micro, North Carolina, town in U.S.
People
* DJ Micro, (born Michael Marsicano) an American trance DJ and producer
*Chii Tomiya (都宮 ちい ...
and nanotechnologies
Nanotechnology, also shortened to nanotech, is the use of matter on an atomic, molecular, and Supramolecular complex, supramolecular scale for industrial purposes. The earliest, widespread description of nanotechnology referred to the particul ...
.
In nature
 The
The honeycomb
A honeycomb is a mass of Triangular prismatic honeycomb#Hexagonal prismatic honeycomb, hexagonal prismatic Beeswax, wax cells built by honey bees in their beehive, nests to contain their larvae and stores of honey and pollen.
beekeeping, Beekee ...
is a well-known example of tessellation in nature with its hexagonal cells.
 In botany, the term "tessellate" describes a checkered pattern, for example on a flower petal, tree bark, or fruit. Flowers including the
In botany, the term "tessellate" describes a checkered pattern, for example on a flower petal, tree bark, or fruit. Flowers including the fritillary
''Fritillaria'' (fritillaries) is a genus of spring flowering herbaceous bulbous perennial plants in the lily family (Liliaceae). The type species, ''Fritillaria meleagris'', was first described in Europe in 1571, while other species from the ...
and some species of ''Colchicum'' are characteristically tessellate.
Many patterns in nature
Patterns in nature are visible regularities of form found in the natural world. These patterns recur in different contexts and can sometimes be modelled mathematically. Natural patterns include symmetries, trees, spirals, meanders, waves, foa ...
are formed by cracks in sheets of materials. These patterns can be described by Gilbert tessellations, also known as random crack networks. The Gilbert tessellation is a mathematical model for the formation of mudcrack
Mudcracks (also known as mud cracks, desiccation cracks or cracked mud) are sedimentary structures formed as muddy sediment dries and contracts.Jackson, J.A., 1997, ''Glossary of Geology'' (4th ed.), American Geological Institute, Alexandria, VA, ...
s, needle-like crystal
A crystal or crystalline solid is a solid material whose constituents (such as atoms, molecules, or ions) are arranged in a highly ordered microscopic structure, forming a crystal lattice that extends in all directions. In addition, macros ...
s, and similar structures. The model, named after Edgar Gilbert, allows cracks to form starting from randomly scattered over the plane; each crack propagates in two opposite directions along a line through the initiation point, its slope chosen at random, creating a tessellation of irregular convex polygons. Basaltic lava flows often display Columnar basalt, columnar jointing as a result of Thermal expansion#Contraction effects (negative thermal expansion), contraction forces causing cracks as the lava cools. The extensive crack networks that develop often produce hexagonal columns of lava. One example of such an array of columns is the Giant's Causeway in Northern Ireland. Tessellated pavement, a characteristic example of which is found at Eaglehawk Neck, Tasmania, Eaglehawk Neck on the Tasman Peninsula of Tasmania, is a rare sedimentary rock formation where the rock has fractured into rectangular blocks.
Other natural patterns occur in foams; these are packed according to Plateau's laws, which require minimal surfaces. Such foams present a problem in how to pack cells as tightly as possible: in 1887, Lord Kelvin proposed a packing using only one solid, the bitruncated cubic honeycomb with very slightly curved faces. In 1993, Denis Weaire and Robert Phelan proposed the Weaire–Phelan structure, which uses less surface area to separate cells of equal volume than Kelvin's foam.
In puzzles and recreational mathematics
 Tessellations have given rise to many types of tiling puzzle, from traditional jigsaw puzzles (with irregular pieces of wood or cardboard) and the tangram to more modern puzzles which often have a mathematical basis. For example, polyiamonds and
Tessellations have given rise to many types of tiling puzzle, from traditional jigsaw puzzles (with irregular pieces of wood or cardboard) and the tangram to more modern puzzles which often have a mathematical basis. For example, polyiamonds and polyomino
A polyomino is a plane geometric figure formed by joining one or more equal squares edge to edge. It is a polyform whose cells are squares. It may be regarded as a finite subset of the regular square tiling.
Polyominoes have been used in pop ...
es are figures of regular triangles and squares, often used in tiling puzzles. Authors such as Henry Dudeney and Martin Gardner have made many uses of tessellation in recreational mathematics. For example, Dudeney invented the hinged dissection, while Gardner wrote about the rep-tile
In the geometry of tessellations, a rep-tile or reptile is a shape that can be dissected into smaller copies of the same shape. The term was coined as a pun on animal reptiles by recreational mathematician Solomon W. Golomb and popularized by ...
, a shape that can be dissection (geometry), dissected into smaller copies of the same shape. Inspired by Gardner's articles in Scientific American, the amateur mathematician Marjorie Rice found four new tessellations with pentagons. Squaring the square is the problem of tiling an integral square (one whose sides have integer length) using only other integral squares. An extension is squaring the plane, tiling it by squares whose sizes are all natural numbers without repetitions; James and Frederick Henle proved that this was possible.
Examples
semiregular tiling
Euclidean plane tilings by convex regular polygons have been widely used since antiquity. The first systematic mathematical treatment was that of Kepler in his ''Harmonices Mundi'' (Latin: ''The Harmony of the World'', 1619).
Notation of Eucli ...
of the plane
File:Tiling Dual Semiregular V3-3-3-3-6 Floret Pentagonal.svg, Floret pentagonal tiling, dual to a semiregular tiling and one of 15 monohedral pentagon tiling
In geometry, a pentagonal tiling is a tiling of the plane where each individual piece is in the shape of a pentagon.
A regular tiling, regular pentagonal tiling on the Euclidean plane is impossible because the internal angle of a Pentagon#Regular ...
s.
File:A variation on a tiling in the Alhambra of Spain.svg, All tiling elements are #Monohedral, identical pseudo‑triangles by disregarding their colors and ornaments.
File:Voderberg.png, The Voderberg tiling, a spiral, #Monohedral, monohedral tiling made of enneagon
In geometry, a nonagon () or enneagon () is a nine-sided polygon or 9-gon.
The name ''nonagon'' is a prefix hybrid formation, from Latin (''nonus'', "ninth" + ''gonon''), used equivalently, attested already in the 16th century in French ''nonogo ...
s.
File:Uniform tiling 433-t0.png, Alternated octagonal tiling, Alternated octagonal or tritetragonal tiling is a uniform tiling of the Hyperbolic geometry, hyperbolic plane.
File:Capital I tiling-4color.svg, Topology, Topological square tiling, isohedrally distorted into I shapes.
See also
* Discrete global grid * Space partitioning * Honeycomb (geometry)Footnotes
References
Sources
* * * * * *External links
Tegula
(open-source software for exploring two-dimensional tilings of the plane, sphere and hyperbolic plane; includes databases containing millions of tilings)
(good bibliography, drawings of regular, semiregular and demiregular tessellations) *Dirk Frettlöh and Edmund Harriss.
Tilings Encyclopedia
(extensive information on substitution tilings, including drawings, people, and references)
Tessellations.org
(how-to guides, Escher tessellation gallery, galleries of tessellations by other artists, lesson plans, history) * (list of web resources including articles and galleries) {{Good article Tessellation, Symmetry Mosaic