Michael F. Atiyah on:
[Wikipedia]
[Google]
[Amazon]
Sir Michael Francis Atiyah (; 22 April 1929 – 11 January 2019) was a British-Lebanese
 Atiyah was born on 22 April 1929 in Hampstead,
Atiyah was born on 22 April 1929 in Hampstead,
 Atiyah spent the academic year 1955–1956 at the Institute for Advanced Study, Princeton, then returned to
Atiyah spent the academic year 1955–1956 at the Institute for Advanced Study, Princeton, then returned to
 Atiyah collaborated with many mathematicians. His three main collaborations were with Raoul Bott on the Atiyah–Bott fixed-point theorem and many other topics, with
Atiyah collaborated with many mathematicians. His three main collaborations were with Raoul Bott on the Atiyah–Bott fixed-point theorem and many other topics, with
 Atiyah's early papers on algebraic geometry (and some general papers) are reprinted in the first volume of his collected works.
As an undergraduate Atiyah was interested in classical projective geometry, and wrote his first paper: a short note on twisted cubics. He started research under
Atiyah's early papers on algebraic geometry (and some general papers) are reprinted in the first volume of his collected works.
As an undergraduate Atiyah was interested in classical projective geometry, and wrote his first paper: a short note on twisted cubics. He started research under
 Atiyah's works on K-theory, including his book on K-theory are reprinted in volume 2 of his collected works.
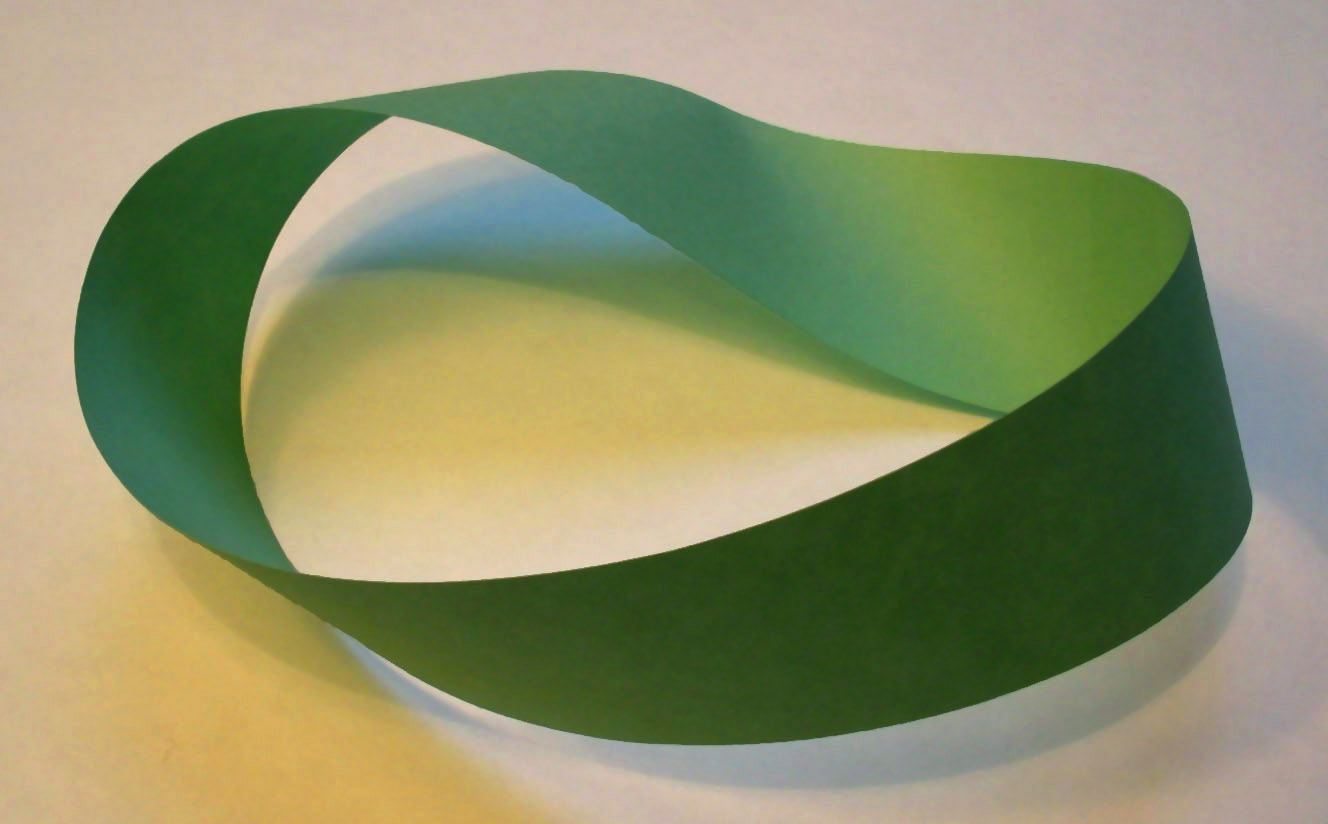
The simplest nontrivial example of a vector bundle is the Möbius band (pictured on the right): a strip of paper with a twist in it, which represents a rank 1 vector bundle over a circle (the circle in question being the centerline of the Möbius band). K-theory is a tool for working with higher-dimensional analogues of this example, or in other words for describing higher-dimensional twistings: elements of the K-group of a space are represented by vector bundles over it, so the Möbius band represents an element of the K-group of a circle.
Topological K-theory was discovered by Atiyah and Friedrich Hirzebruch who were inspired by Grothendieck's proof of the Grothendieck–Riemann–Roch theorem and Bott's work on the periodicity theorem. This paper only discussed the zeroth K-group; they shortly after extended it to K-groups of all degrees, giving the first (nontrivial) example of a generalized cohomology theory.
Several results showed that the newly introduced K-theory was in some ways more powerful than ordinary cohomology theory. Atiyah and Todd used K-theory to improve the lower bounds found using ordinary cohomology by Borel and Serre for the
Atiyah's works on K-theory, including his book on K-theory are reprinted in volume 2 of his collected works.
The simplest nontrivial example of a vector bundle is the Möbius band (pictured on the right): a strip of paper with a twist in it, which represents a rank 1 vector bundle over a circle (the circle in question being the centerline of the Möbius band). K-theory is a tool for working with higher-dimensional analogues of this example, or in other words for describing higher-dimensional twistings: elements of the K-group of a space are represented by vector bundles over it, so the Möbius band represents an element of the K-group of a circle.
Topological K-theory was discovered by Atiyah and Friedrich Hirzebruch who were inspired by Grothendieck's proof of the Grothendieck–Riemann–Roch theorem and Bott's work on the periodicity theorem. This paper only discussed the zeroth K-group; they shortly after extended it to K-groups of all degrees, giving the first (nontrivial) example of a generalized cohomology theory.
Several results showed that the newly introduced K-theory was in some ways more powerful than ordinary cohomology theory. Atiyah and Todd used K-theory to improve the lower bounds found using ordinary cohomology by Borel and Serre for the  The Atiyah–Hirzebruch spectral sequence relates the ordinary cohomology of a space to its generalized cohomology theory. (Atiyah and Hirzebruch used the case of K-theory, but their method works for all cohomology theories).
Atiyah showed that for a finite group ''G'', the K-theory of its classifying space, ''BG'', is isomorphic to the completion of its
The Atiyah–Hirzebruch spectral sequence relates the ordinary cohomology of a space to its generalized cohomology theory. (Atiyah and Hirzebruch used the case of K-theory, but their method works for all cohomology theories).
Atiyah showed that for a finite group ''G'', the K-theory of its classifying space, ''BG'', is isomorphic to the completion of its
 Atiyah's work on index theory is reprinted in volumes 3 and 4 of his collected works.
The index of a differential operator is closely related to the number of independent solutions (more precisely, it is the differences of the numbers of independent solutions of the differential operator and its adjoint). There are many hard and fundamental problems in mathematics that can easily be reduced to the problem of finding the number of independent solutions of some differential operator, so if one has some means of finding the index of a differential operator these problems can often be solved. This is what the Atiyah–Singer index theorem does: it gives a formula for the index of certain differential operators, in terms of topological invariants that look quite complicated but are in practice usually straightforward to calculate.
Several deep theorems, such as the Hirzebruch–Riemann–Roch theorem, are special cases of the Atiyah–Singer index theorem. In fact the index theorem gave a more powerful result, because its proof applied to all compact complex manifolds, while Hirzebruch's proof only worked for projective manifolds. There were also many new applications: a typical one is calculating the dimensions of the moduli spaces of instantons. The index theorem can also be run "in reverse": the index is obviously an integer, so the formula for it must also give an integer, which sometimes gives subtle integrality conditions on invariants of manifolds. A typical example of this is
Atiyah's work on index theory is reprinted in volumes 3 and 4 of his collected works.
The index of a differential operator is closely related to the number of independent solutions (more precisely, it is the differences of the numbers of independent solutions of the differential operator and its adjoint). There are many hard and fundamental problems in mathematics that can easily be reduced to the problem of finding the number of independent solutions of some differential operator, so if one has some means of finding the index of a differential operator these problems can often be solved. This is what the Atiyah–Singer index theorem does: it gives a formula for the index of certain differential operators, in terms of topological invariants that look quite complicated but are in practice usually straightforward to calculate.
Several deep theorems, such as the Hirzebruch–Riemann–Roch theorem, are special cases of the Atiyah–Singer index theorem. In fact the index theorem gave a more powerful result, because its proof applied to all compact complex manifolds, while Hirzebruch's proof only worked for projective manifolds. There were also many new applications: a typical one is calculating the dimensions of the moduli spaces of instantons. The index theorem can also be run "in reverse": the index is obviously an integer, so the formula for it must also give an integer, which sometimes gives subtle integrality conditions on invariants of manifolds. A typical example of this is  With Bott, Atiyah found an analogue of the
With Bott, Atiyah found an analogue of the  Atiyah, Bott and Vijay K. Patodi gave a new proof of the index theorem using the heat equation.
If the
Atiyah, Bott and Vijay K. Patodi gave a new proof of the index theorem using the heat equation.
If the  The fundamental solutions of linear hyperbolic partial differential equations often have Petrovsky lacunas: regions where they vanish identically. These were studied in 1945 by
The fundamental solutions of linear hyperbolic partial differential equations often have Petrovsky lacunas: regions where they vanish identically. These were studied in 1945 by
 Many of his papers on gauge theory and related topics are reprinted in volume 5 of his collected works. A common theme of these papers is the study of moduli spaces of solutions to certain non-linear partial differential equations, in particular the equations for instantons and monopoles. This often involves finding a subtle correspondence between solutions of two seemingly quite different equations. An early example of this which Atiyah used repeatedly is the Penrose transform, which can sometimes convert solutions of a non-linear equation over some real manifold into solutions of some linear holomorphic equations over a different complex manifold.
In a series of papers with several authors, Atiyah classified all instantons on 4-dimensional Euclidean space. It is more convenient to classify instantons on a sphere as this is compact, and this is essentially equivalent to classifying instantons on Euclidean space as this is conformally equivalent to a sphere and the equations for instantons are conformally invariant. With Hitchin and Singer he calculated the dimension of the moduli space of irreducible self-dual connections (instantons) for any principal bundle over a compact 4-dimensional Riemannian manifold (the Atiyah–Hitchin–Singer theorem). For example, the dimension of the space of SU2 instantons of rank ''k''>0 is 8''k''−3. To do this they used the Atiyah–Singer index theorem to calculate the dimension of the tangent space of the moduli space at a point; the tangent space is essentially the space of solutions of an elliptic differential operator, given by the linearization of the non-linear Yang–Mills equations. These moduli spaces were later used by Donaldson to construct his invariants of 4-manifolds.
Atiyah and Ward used the Penrose correspondence to reduce the classification of all instantons on the 4-sphere to a problem in algebraic geometry. With Hitchin he used ideas of Horrocks to solve this problem, giving the
Many of his papers on gauge theory and related topics are reprinted in volume 5 of his collected works. A common theme of these papers is the study of moduli spaces of solutions to certain non-linear partial differential equations, in particular the equations for instantons and monopoles. This often involves finding a subtle correspondence between solutions of two seemingly quite different equations. An early example of this which Atiyah used repeatedly is the Penrose transform, which can sometimes convert solutions of a non-linear equation over some real manifold into solutions of some linear holomorphic equations over a different complex manifold.
In a series of papers with several authors, Atiyah classified all instantons on 4-dimensional Euclidean space. It is more convenient to classify instantons on a sphere as this is compact, and this is essentially equivalent to classifying instantons on Euclidean space as this is conformally equivalent to a sphere and the equations for instantons are conformally invariant. With Hitchin and Singer he calculated the dimension of the moduli space of irreducible self-dual connections (instantons) for any principal bundle over a compact 4-dimensional Riemannian manifold (the Atiyah–Hitchin–Singer theorem). For example, the dimension of the space of SU2 instantons of rank ''k''>0 is 8''k''−3. To do this they used the Atiyah–Singer index theorem to calculate the dimension of the tangent space of the moduli space at a point; the tangent space is essentially the space of solutions of an elliptic differential operator, given by the linearization of the non-linear Yang–Mills equations. These moduli spaces were later used by Donaldson to construct his invariants of 4-manifolds.
Atiyah and Ward used the Penrose correspondence to reduce the classification of all instantons on the 4-sphere to a problem in algebraic geometry. With Hitchin he used ideas of Horrocks to solve this problem, giving the
 Many of the papers in the 6th volume of his collected works are surveys, obituaries, and general talks. Atiyah continued to publish subsequently, including several surveys, a popular book, and another paper with Segal on twisted K-theory.
One paper is a detailed study of the
Many of the papers in the 6th volume of his collected works are surveys, obituaries, and general talks. Atiyah continued to publish subsequently, including several surveys, a popular book, and another paper with Segal on twisted K-theory.
One paper is a detailed study of the
Jonathan Robbins
(called the Berry–Robbins problem), who asked if there is a map from the configuration space of ''n'' points in 3-space to the flag manifold of the unitary group. Atiyah gave an affirmative answer to this question, but felt his solution was too computational and studied a conjecture that would give a more natural solution. He also related the question to
 In 1966, when he was thirty-seven years old, he was awarded the Fields Medal, for his work in developing K-theory, a generalized Lefschetz fixed-point theorem and the Atiyah–Singer theorem, for which he also won the Abel Prize jointly with Isadore Singer in 2004.
Among other prizes he has received are the
In 1966, when he was thirty-seven years old, he was awarded the Fields Medal, for his work in developing K-theory, a generalized Lefschetz fixed-point theorem and the Atiyah–Singer theorem, for which he also won the Abel Prize jointly with Isadore Singer in 2004.
Among other prizes he has received are the
Michael Atiyah tells his life story
at Web of Stories
The celebrations of Michael Atiyah's 80th birthday in Edinburgh, 20-24 April 2009Mathematical descendants of Michael Atiyah
* * * * * * * * * * * *
List of works of Michael Atiyah
fro
''Celebratio Mathematica''
* * {{DEFAULTSORT:Atiyah, Michael 1929 births 2019 deaths People from Hampstead Alumni of Trinity College, Cambridge 20th-century British mathematicians 21st-century British mathematicians Academics of the University of Edinburgh Abel Prize laureates Algebraic geometers Michael British humanists British people of Lebanese descent British people of Scottish descent Differential geometers Fellows of the American Academy of Arts and Sciences Fellows of the American Mathematical Society Fellows of New College, Oxford Fellows of Pembroke College, Cambridge Fellows of the Academy of Medical Sciences (United Kingdom) Fellows of the Australian Academy of Science Foreign Fellows of the Indian National Science Academy Fellows of the Royal Society of Edinburgh Fields Medalists Institute for Advanced Study faculty Knights Bachelor Masters of Trinity College, Cambridge Fellows of the Royal Society Members of the French Academy of Sciences Foreign associates of the National Academy of Sciences Foreign Members of the Russian Academy of Sciences Members of the Order of Merit Members of the Norwegian Academy of Science and Letters People educated at Manchester Grammar School People associated with the University of Leicester Presidents of the Royal Society Recipients of the Copley Medal Royal Medal winners Savilian Professors of Geometry Topologists Victoria College, Alexandria alumni Royal Electrical and Mechanical Engineers soldiers Members of the Royal Swedish Academy of Sciences
mathematician
A mathematician is someone who uses an extensive knowledge of mathematics in their work, typically to solve mathematical problems.
Mathematicians are concerned with numbers, data, quantity, mathematical structure, structure, space, Mathematica ...
specialising in geometry
Geometry (; ) is, with arithmetic, one of the oldest branches of mathematics. It is concerned with properties of space such as the distance, shape, size, and relative position of figures. A mathematician who works in the field of geometry is c ...
. His contributions include the Atiyah–Singer index theorem and co-founding topological K-theory. He was awarded the Fields Medal in 1966 and the Abel Prize in 2004.
Life
Atiyah grew up in Sudan andEgypt
Egypt ( ar, مصر , ), officially the Arab Republic of Egypt, is a transcontinental country spanning the northeast corner of Africa and southwest corner of Asia via a land bridge formed by the Sinai Peninsula. It is bordered by the Med ...
but spent most of his academic life in the United Kingdom at the University of Oxford
The University of Oxford is a collegiate research university in Oxford, England. There is evidence of teaching as early as 1096, making it the oldest university in the English-speaking world and the world's second-oldest university in contin ...
and the University of Cambridge
, mottoeng = Literal: From here, light and sacred draughts.
Non literal: From this place, we gain enlightenment and precious knowledge.
, established =
, other_name = The Chancellor, Masters and Schola ...
and in the United States at the Institute for Advanced Study
The Institute for Advanced Study (IAS), located in Princeton, New Jersey, in the United States, is an independent center for theoretical research and intellectual inquiry. It has served as the academic home of internationally preeminent scholar ...
. He was the President of the Royal Society
The Royal Society, formally The Royal Society of London for Improving Natural Knowledge, is a learned society and the United Kingdom's national academy of sciences. The society fulfils a number of roles: promoting science and its benefits, r ...
(1990–1995), founding director of the Isaac Newton Institute
The Isaac Newton Institute for Mathematical Sciences is an international research institute for mathematics and its many applications at the University of Cambridge. It is named after one of the university's most illustrious figures, the mathema ...
(1990–1996), master of Trinity College, Cambridge
Trinity College is a constituent college of the University of Cambridge. Founded in 1546 by King Henry VIII, Trinity is one of the largest Cambridge colleges, with the largest financial endowment of any college at either Cambridge or Oxford. ...
(1990–1997), chancellor of the University of Leicester
, mottoeng = So that they may have life
, established =
, type = public research university
, endowment = £20.0 million
, budget = £326 million
, chancellor = David Willetts
, vice_chancellor = Nishan Canagarajah
, head_la ...
(1995–2005), and the President of the Royal Society of Edinburgh
The Royal Society of Edinburgh is Scotland's national academy of science and letters. It is a registered charity that operates on a wholly independent and non-partisan basis and provides public benefit throughout Scotland. It was established i ...
(2005–2008). From 1997 until his death, he was an honorary professor in the University of Edinburgh
The University of Edinburgh ( sco, University o Edinburgh, gd, Oilthigh Dhùn Èideann; abbreviated as ''Edin.'' in post-nominals) is a public research university based in Edinburgh, Scotland. Granted a royal charter by King James VI in 15 ...
.
Atiyah's mathematical collaborators included Raoul Bott, Friedrich Hirzebruch and Isadore Singer, and his students included Graeme Segal, Nigel Hitchin, Simon Donaldson, and Edward Witten. Together with Hirzebruch, he laid the foundations for topological K-theory, an important tool in algebraic topology
Algebraic topology is a branch of mathematics that uses tools from abstract algebra to study topological spaces. The basic goal is to find algebraic invariants that classify topological spaces up to homeomorphism, though usually most classif ...
, which, informally speaking, describes ways in which spaces can be twisted. His best known result, the Atiyah–Singer index theorem, was proved with Singer in 1963 and is used in counting the number of independent solutions to differential equations. Some of his more recent work was inspired by theoretical physics, in particular instantons and monopoles, which are responsible for some corrections in quantum field theory
In theoretical physics, quantum field theory (QFT) is a theoretical framework that combines classical field theory, special relativity, and quantum mechanics. QFT is used in particle physics to construct physical models of subatomic particles a ...
. He was awarded the Fields Medal in 1966 and the Abel Prize in 2004.
Early life and education
 Atiyah was born on 22 April 1929 in Hampstead,
Atiyah was born on 22 April 1929 in Hampstead, London
London is the capital and List of urban areas in the United Kingdom, largest city of England and the United Kingdom, with a population of just under 9 million. It stands on the River Thames in south-east England at the head of a estuary dow ...
, England, the son of Jean (née Levens) and Edward Atiyah. His mother was Scottish and his father was a Lebanese Orthodox Christian
Orthodoxy (from Greek: ) is adherence to correct or accepted creeds, especially in religion.
Orthodoxy within Christianity refers to acceptance of the doctrines defined by various creeds and ecumenical councils in Antiquity, but different Churche ...
. He had two brothers, Patrick (deceased) and Joe, and a sister, Selma (deceased). Atiyah went to primary school at the Diocesan school in Khartoum
Khartoum or Khartum ( ; ar, الخرطوم, Al-Khurṭūm, din, Kaartuɔ̈m) is the capital of Sudan. With a population of 5,274,321, its metropolitan area is the largest in Sudan. It is located at the confluence of the White Nile, flowing n ...
, Sudan (1934–1941), and to secondary school at Victoria College in Cairo
Cairo ( ; ar, القاهرة, al-Qāhirah, ) is the capital of Egypt and its largest city, home to 10 million people. It is also part of the largest urban agglomeration in Africa, the Arab world and the Middle East: The Greater Cairo met ...
and Alexandria
Alexandria ( or ; ar, ٱلْإِسْكَنْدَرِيَّةُ ; grc-gre, Αλεξάνδρεια, Alexándria) is the second largest city in Egypt, and the largest city on the Mediterranean coast. Founded in by Alexander the Great, Alexandr ...
(1941–1945); the school was also attended by European nobility displaced by the Second World War
World War II or the Second World War, often abbreviated as WWII or WW2, was a world war that lasted from 1939 to 1945. It involved the World War II by country, vast majority of the world's countries—including all of the great power ...
and some future leaders of Arab nations. He returned to England and Manchester Grammar School
The Manchester Grammar School (MGS) in Manchester, England, is the largest independent day school for boys in the United Kingdom. Founded in 1515 as a free grammar school next to Manchester Parish Church, it moved in 1931 to its present site at ...
for his HSC studies (1945–1947) and did his national service with the Royal Electrical and Mechanical Engineers (1947–1949). His undergraduate
Undergraduate education is education conducted after secondary education and before postgraduate education. It typically includes all postsecondary programs up to the level of a bachelor's degree. For example, in the United States, an entry-le ...
and postgraduate
Postgraduate or graduate education refers to academic or professional degrees, certificates, diplomas, or other qualifications pursued by post-secondary students who have earned an undergraduate ( bachelor's) degree.
The organization and str ...
studies took place at Trinity College, Cambridge
Trinity College is a constituent college of the University of Cambridge. Founded in 1546 by King Henry VIII, Trinity is one of the largest Cambridge colleges, with the largest financial endowment of any college at either Cambridge or Oxford. ...
(1949–1955). He was a doctoral student of William V. D. Hodge and was awarded a doctorate in 1955 for a thesis entitled ''Some Applications of Topological Methods in Algebraic Geometry''.
Atiyah was a member of the British Humanist Association.
During his time at Cambridge, he was president of The Archimedeans.
Career and research
 Atiyah spent the academic year 1955–1956 at the Institute for Advanced Study, Princeton, then returned to
Atiyah spent the academic year 1955–1956 at the Institute for Advanced Study, Princeton, then returned to Cambridge University
, mottoeng = Literal: From here, light and sacred draughts.
Non literal: From this place, we gain enlightenment and precious knowledge.
, established =
, other_name = The Chancellor, Masters and Schola ...
, where he was a research fellow and assistant lecturer (1957–1958), then a university lecturer and tutorial fellow
A fellow is a concept whose exact meaning depends on context.
In learned or professional societies, it refers to a privileged member who is specially elected in recognition of their work and achievements.
Within the context of higher education ...
at Pembroke College, Cambridge (1958–1961). In 1961, he moved to the University of Oxford
The University of Oxford is a collegiate research university in Oxford, England. There is evidence of teaching as early as 1096, making it the oldest university in the English-speaking world and the world's second-oldest university in contin ...
, where he was a reader and professor
Professor (commonly abbreviated as Prof.) is an academic rank at universities and other post-secondary education and research institutions in most countries. Literally, ''professor'' derives from Latin as a "person who professes". Professor ...
ial fellow at St Catherine's College (1961–1963). He became Savilian Professor of Geometry and a professorial fellow of New College, Oxford
New College is one of the constituent colleges of the University of Oxford in the United Kingdom. Founded in 1379 by William of Wykeham in conjunction with Winchester College as its feeder school, New College is one of the oldest colleges at ...
, from 1963 to 1969. He took up a three-year professorship at the Institute for Advanced Study in Princeton after which he returned to Oxford as a Royal Society
The Royal Society, formally The Royal Society of London for Improving Natural Knowledge, is a learned society and the United Kingdom's national academy of sciences. The society fulfils a number of roles: promoting science and its benefits, r ...
Research Professor and professorial fellow of St Catherine's College. He was president of the London Mathematical Society
The London Mathematical Society (LMS) is one of the United Kingdom's learned societies for mathematics (the others being the Royal Statistical Society (RSS), the Institute of Mathematics and its Applications (IMA), the Edinburgh Mathematical S ...
from 1974 to 1976.
Atiyah was president of the Pugwash Conferences on Science and World Affairs from 1997 to 2002. He also contributed to the foundation of the InterAcademy Panel on International Issues
The InterAcademy Partnership (IAP) is a global network consisting of over 140 national and regional member academies of science, engineering, and medicine. It was founded in 1993 as the InterAcademy Panel (IAP). In 2000, the IAP founded the ''Inte ...
, the Association of European Academies (ALLEA), and the European Mathematical Society
The European Mathematical Society (EMS) is a European organization dedicated to the development of mathematics in Europe. Its members are different mathematical societies in Europe, academic institutions and individual mathematicians. The current ...
(EMS).
Within the United Kingdom, he was involved in the creation of the Isaac Newton Institute for Mathematical Sciences in Cambridge and was its first director (1990–1996). He was President of the Royal Society (1990–1995), Master of Trinity College, Cambridge (1990–1997), Chancellor
Chancellor ( la, cancellarius) is a title of various official positions in the governments of many nations. The original chancellors were the of Roman courts of justice—ushers, who sat at the or lattice work screens of a basilica or law cou ...
of the University of Leicester
, mottoeng = So that they may have life
, established =
, type = public research university
, endowment = £20.0 million
, budget = £326 million
, chancellor = David Willetts
, vice_chancellor = Nishan Canagarajah
, head_la ...
(1995–2005), and president of the Royal Society of Edinburgh
The Royal Society of Edinburgh is Scotland's national academy of science and letters. It is a registered charity that operates on a wholly independent and non-partisan basis and provides public benefit throughout Scotland. It was established i ...
(2005–2008). From 1997 until his death in 2019 he was an honorary professor in the University of Edinburgh
The University of Edinburgh ( sco, University o Edinburgh, gd, Oilthigh Dhùn Èideann; abbreviated as ''Edin.'' in post-nominals) is a public research university based in Edinburgh, Scotland. Granted a royal charter by King James VI in 15 ...
. He was a Trustee of the James Clerk Maxwell Foundation.
Collaborations
 Atiyah collaborated with many mathematicians. His three main collaborations were with Raoul Bott on the Atiyah–Bott fixed-point theorem and many other topics, with
Atiyah collaborated with many mathematicians. His three main collaborations were with Raoul Bott on the Atiyah–Bott fixed-point theorem and many other topics, with Isadore M. Singer
Isadore Manuel Singer (May 3, 1924 – February 11, 2021) was an American mathematician. He was an Emeritus Institute Professor in the Department of Mathematics at the Massachusetts Institute of Technology and a Professor Emeritus of Mathematic ...
on the Atiyah–Singer index theorem, and with Friedrich Hirzebruch on topological K-theory, all of whom he met at the Institute for Advanced Study
The Institute for Advanced Study (IAS), located in Princeton, New Jersey, in the United States, is an independent center for theoretical research and intellectual inquiry. It has served as the academic home of internationally preeminent scholar ...
in Princeton in 1955. His other collaborators included; J. Frank Adams
John Frank Adams (5 November 1930 – 7 January 1989) was a British mathematician, one of the major contributors to homotopy theory.
Life
He was born in Woolwich, a suburb in south-east London, and attended Bedford School. He began research ...
( Hopf invariant problem), Jürgen Berndt (projective planes), Roger Bielawski (Berry–Robbins problem), Howard Donnelly (L-function
In mathematics, an ''L''-function is a meromorphic function on the complex plane, associated to one out of several categories of mathematical objects. An ''L''-series is a Dirichlet series, usually convergent on a half-plane, that may give ...
s), Vladimir G. Drinfeld (instantons), Johan L. Dupont (singularities of vector fields), Lars Gårding (hyperbolic differential equation
In mathematics, a hyperbolic partial differential equation of order n is a partial differential equation (PDE) that, roughly speaking, has a well-posed initial value problem for the first n-1 derivatives. More precisely, the Cauchy problem can be ...
s), Nigel J. Hitchin (monopoles), William V. D. Hodge (Integrals of the second kind), Michael Hopkins (K-theory), Lisa Jeffrey (topological Lagrangians), John D. S. Jones (Yang–Mills theory), Juan Maldacena (M-theory), Yuri I. Manin (instantons), Nick S. Manton (Skyrmions), Vijay K. Patodi (spectral asymmetry), A. N. Pressley (convexity), Elmer Rees (vector bundles), Wilfried Schmid (discrete series representations), Graeme Segal (equivariant K-theory), Alexander Shapiro (Clifford algebras), L. Smith (homotopy groups of spheres), Paul Sutcliffe
Paul Michael Sutcliffe is British mathematical physicist and mathematician, currently Professor of Theoretical Physics at the University of Durham. He specialises in the study of topological solitons.
He serves as the Project Director of the SPO ...
(polyhedra), David O. Tall (lambda rings), John A. Todd ( Stiefel manifolds), Cumrun Vafa (M-theory), Richard S. Ward (instantons) and Edward Witten (M-theory, topological quantum field theories).
His later research on gauge field theories
In physics, a gauge theory is a type of field theory in which the Lagrangian (and hence the dynamics of the system itself) does not change (is invariant) under local transformations according to certain smooth families of operations ( Lie group ...
, particularly Yang–Mills theory, stimulated important interactions between geometry
Geometry (; ) is, with arithmetic, one of the oldest branches of mathematics. It is concerned with properties of space such as the distance, shape, size, and relative position of figures. A mathematician who works in the field of geometry is c ...
and physics
Physics is the natural science that studies matter, its fundamental constituents, its motion and behavior through space and time, and the related entities of energy and force. "Physical science is that department of knowledge which rel ...
, most notably in the work of Edward Witten.
Atiyah's students included
Peter Braam 1987,
Simon Donaldson 1983,
K. David Elworthy 1967,
Howard Fegan 1977,
Eric Grunwald 1977,
Nigel Hitchin 1972,
Lisa Jeffrey 1991,
Frances Kirwan
Dame Frances Clare Kirwan, (born 21 August 1959) is a British mathematician, currently Savilian Professor of Geometry at the University of Oxford. Her fields of specialisation are algebraic and symplectic geometry.
Education
Kirwan was ed ...
1984,
Peter Kronheimer 1986,
Ruth Lawrence 1989,
George Lusztig 1971,
Jack Morava
Jack Johnson Morava is an American homotopy theorist at Johns Hopkins University.
Education
Of Czech and Appalachian descent, he was raised in Texas' lower Rio Grande valley. An early interest in topology was strongly encouraged by his paren ...
1968,
Michael Murray 1983,
Peter Newstead 1966,
Ian R. Porteous
Ian Robertson Porteous (9 October 1930 – 30 January 2011) was a Scottish mathematician at the University of Liverpool and an educator on Merseyside. He is best known for three books on geometry and modern algebra. In Liverpool he and Peter Gib ...
1961,
John Roe 1985,
Brian Sanderson 1963,
Rolph Schwarzenberger 1960,
Graeme Segal 1967,
David Tall 1966,
and Graham White 1982.
Other contemporary mathematicians who influenced Atiyah include Roger Penrose
Sir Roger Penrose (born 8 August 1931) is an English mathematician, mathematical physicist, philosopher of science and Nobel Laureate in Physics. He is Emeritus Rouse Ball Professor of Mathematics in the University of Oxford, an emeritus f ...
, Lars Hörmander, Alain Connes and Jean-Michel Bismut
Jean-Michel Bismut (born 26 February 1948) is a French mathematician who has been a professor at the Université Paris-Sud since 1981.
His mathematical career covers two apparently different branches of
mathematics: probability theory and diffe ...
. Atiyah said that the mathematician he most admired was Hermann Weyl
Hermann Klaus Hugo Weyl, (; 9 November 1885 – 8 December 1955) was a German mathematician, theoretical physicist and philosopher. Although much of his working life was spent in Zürich, Switzerland, and then Princeton, New Jersey, he is ass ...
, and that his favourite mathematicians from before the 20th century were Bernhard Riemann and William Rowan Hamilton
Sir William Rowan Hamilton LL.D, DCL, MRIA, FRAS (3/4 August 1805 – 2 September 1865) was an Irish mathematician, astronomer, and physicist. He was the Andrews Professor of Astronomy at Trinity College Dublin, and Royal Astronomer of Ire ...
.
The seven volumes of Atiyah's collected papers include most of his work, except for his commutative algebra textbook; the first five volumes are divided thematically and the sixth and seventh arranged by date.
Algebraic geometry (1952–1958)
 Atiyah's early papers on algebraic geometry (and some general papers) are reprinted in the first volume of his collected works.
As an undergraduate Atiyah was interested in classical projective geometry, and wrote his first paper: a short note on twisted cubics. He started research under
Atiyah's early papers on algebraic geometry (and some general papers) are reprinted in the first volume of his collected works.
As an undergraduate Atiyah was interested in classical projective geometry, and wrote his first paper: a short note on twisted cubics. He started research under W. V. D. Hodge
Sir William Vallance Douglas Hodge (; 17 June 1903 – 7 July 1975) was a British mathematician, specifically a geometer.
His discovery of far-reaching topological relations between algebraic geometry and differential geometry—an area now c ...
and won the Smith's prize for 1954 for a sheaf-theoretic approach to ruled surface
In geometry, a surface is ruled (also called a scroll) if through every point of there is a straight line that lies on . Examples include the plane, the lateral surface of a cylinder or cone, a conical surface with elliptical directri ...
s, which encouraged Atiyah to continue in mathematics, rather than switch to his other interests—architecture and archaeology.
His PhD thesis with Hodge was on a sheaf-theoretic approach to Solomon Lefschetz's theory of integrals of the second kind on algebraic varieties, and resulted in an invitation to visit the Institute for Advanced Study in Princeton for a year. While in Princeton he classified vector bundle
In mathematics, a vector bundle is a topological construction that makes precise the idea of a family of vector spaces parameterized by another space X (for example X could be a topological space, a manifold, or an algebraic variety): to ev ...
s on an elliptic curve (extending Alexander Grothendieck's classification of vector bundles on a genus 0 curve), by showing that any vector bundle is a sum of (essentially unique) indecomposable vector bundles, and then showing that the space of indecomposable vector bundles of given degree and positive dimension can be identified with the elliptic curve. He also studied double points on surfaces, giving the first example of a flop, a special birational transformation of 3-fold
In algebraic geometry, a 3-fold or threefold is a 3-dimensional algebraic variety.
The Mori program
In algebraic geometry, the minimal model program is part of the birational classification of algebraic varieties. Its goal is to construct a bira ...
s that was later heavily used in Shigefumi Mori's work on minimal models for 3-folds. Atiyah's flop can also be used to show that the universal marked family of K3 surfaces is not Hausdorff.
K theory (1959–1974)
 Atiyah's works on K-theory, including his book on K-theory are reprinted in volume 2 of his collected works.
The simplest nontrivial example of a vector bundle is the Möbius band (pictured on the right): a strip of paper with a twist in it, which represents a rank 1 vector bundle over a circle (the circle in question being the centerline of the Möbius band). K-theory is a tool for working with higher-dimensional analogues of this example, or in other words for describing higher-dimensional twistings: elements of the K-group of a space are represented by vector bundles over it, so the Möbius band represents an element of the K-group of a circle.
Topological K-theory was discovered by Atiyah and Friedrich Hirzebruch who were inspired by Grothendieck's proof of the Grothendieck–Riemann–Roch theorem and Bott's work on the periodicity theorem. This paper only discussed the zeroth K-group; they shortly after extended it to K-groups of all degrees, giving the first (nontrivial) example of a generalized cohomology theory.
Several results showed that the newly introduced K-theory was in some ways more powerful than ordinary cohomology theory. Atiyah and Todd used K-theory to improve the lower bounds found using ordinary cohomology by Borel and Serre for the
Atiyah's works on K-theory, including his book on K-theory are reprinted in volume 2 of his collected works.
The simplest nontrivial example of a vector bundle is the Möbius band (pictured on the right): a strip of paper with a twist in it, which represents a rank 1 vector bundle over a circle (the circle in question being the centerline of the Möbius band). K-theory is a tool for working with higher-dimensional analogues of this example, or in other words for describing higher-dimensional twistings: elements of the K-group of a space are represented by vector bundles over it, so the Möbius band represents an element of the K-group of a circle.
Topological K-theory was discovered by Atiyah and Friedrich Hirzebruch who were inspired by Grothendieck's proof of the Grothendieck–Riemann–Roch theorem and Bott's work on the periodicity theorem. This paper only discussed the zeroth K-group; they shortly after extended it to K-groups of all degrees, giving the first (nontrivial) example of a generalized cohomology theory.
Several results showed that the newly introduced K-theory was in some ways more powerful than ordinary cohomology theory. Atiyah and Todd used K-theory to improve the lower bounds found using ordinary cohomology by Borel and Serre for the James number
James is a common English language surname and given name:
* James (name), the typically masculine first name James
* James (surname), various people with the last name James
James or James City may also refer to:
People
* King James (disambigua ...
, describing when a map from a complex Stiefel manifold to a sphere has a cross section. (Adams
Adams may refer to:
* For persons, see Adams (surname)
Places United States
*Adams, California
*Adams, California, former name of Corte Madera, California
*Adams, Decatur County, Indiana
*Adams, Kentucky
*Adams, Massachusetts, a New England town ...
and Grant-Walker later showed that the bound found by Atiyah and Todd was best possible.) Atiyah and Hirzebruch used K-theory to explain some relations between Steenrod operation In algebraic topology, a Steenrod algebra was defined by to be the algebra of stable cohomology operations for mod p cohomology.
For a given prime number p, the Steenrod algebra A_p is the graded Hopf algebra over the field \mathbb_p of order p ...
s and Todd class In mathematics, the Todd class is a certain construction now considered a part of the theory in algebraic topology of characteristic classes. The Todd class of a vector bundle can be defined by means of the theory of Chern classes, and is enco ...
es that Hirzebruch had noticed a few years before. The original solution of the Hopf invariant one problem
In mathematics, in particular in algebraic topology, the Hopf invariant is a homotopy invariant of certain maps between n-spheres.
__TOC__ Motivation
In 1931 Heinz Hopf used Clifford parallels to construct the ''Hopf map''
:\eta\colon S^3 \to S^ ...
operations by J. F. Adams was very long and complicated, using secondary cohomology operations. Atiyah showed how primary operations in K-theory could be used to give a short solution taking only a few lines, and in joint work with Adams also proved analogues of the result at odd primes.
 The Atiyah–Hirzebruch spectral sequence relates the ordinary cohomology of a space to its generalized cohomology theory. (Atiyah and Hirzebruch used the case of K-theory, but their method works for all cohomology theories).
Atiyah showed that for a finite group ''G'', the K-theory of its classifying space, ''BG'', is isomorphic to the completion of its
The Atiyah–Hirzebruch spectral sequence relates the ordinary cohomology of a space to its generalized cohomology theory. (Atiyah and Hirzebruch used the case of K-theory, but their method works for all cohomology theories).
Atiyah showed that for a finite group ''G'', the K-theory of its classifying space, ''BG'', is isomorphic to the completion of its character ring In mathematics, especially in the area of algebra known as representation theory, the representation ring (or Green ring after J. A. Green) of a group is a ring formed from all the (isomorphism classes of the) finite-dimensional linear represe ...
:
:
The same year they proved the result for ''G'' any compact connected Lie group
In mathematics, a Lie group (pronounced ) is a group that is also a differentiable manifold. A manifold is a space that locally resembles Euclidean space, whereas groups define the abstract concept of a binary operation along with the addit ...
. Although soon the result could be extended to ''all'' compact Lie groups by incorporating results from Graeme Segal's thesis, that extension was complicated. However a simpler and more general proof was produced by introducing equivariant K-theory, ''i.e.'' equivalence classes of ''G''-vector bundles over a compact ''G''-space ''X''. It was shown that under suitable conditions the completion of the equivariant K-theory of ''X'' is isomorphic to the ordinary K-theory of a space, , which fibred over ''BG'' with fibre ''X'':
:
The original result then followed as a corollary by taking ''X'' to be a point: the left hand side reduced to the completion of ''R(G)'' and the right to ''K(BG)''. See Atiyah–Segal completion theorem for more details.
He defined new generalized homology and cohomology theories called bordism and cobordism, and pointed out that many of the deep results on cobordism of manifolds found by René Thom, C. T. C. Wall, and others could be naturally reinterpreted as statements about these cohomology theories. Some of these cohomology theories, in particular complex cobordism, turned out to be some of the most powerful cohomology theories known.
He introduced the J-group
In mathematics, the ''J''-homomorphism is a mapping from the homotopy groups of the special orthogonal groups to the homotopy groups of spheres. It was defined by , extending a construction of .
Definition
Whitehead's original homomorphism is defi ...
''J''(''X'') of a finite complex ''X'', defined as the group of stable fiber homotopy equivalence classes of sphere bundle In the mathematical field of topology, a sphere bundle is a fiber bundle in which the fibers are spheres S^n of some dimension ''n''. Similarly, in a disk bundle, the fibers are disks D^n. From a topological perspective, there is no difference betw ...
s; this was later studied in detail by J. F. Adams
John Frank Adams (5 November 1930 – 7 January 1989) was a British mathematician, one of the major contributors to homotopy theory.
Life
He was born in Woolwich, a suburb in south-east London, and attended Bedford School. He began research ...
in a series of papers, leading to the Adams conjecture.
With Hirzebruch he extended the Grothendieck–Riemann–Roch theorem to complex analytic embeddings, and in a related paper they showed that the Hodge conjecture for integral cohomology is false. The Hodge conjecture for rational cohomology is, as of 2008, a major unsolved problem.
The Bott periodicity theorem
In mathematics, the Bott periodicity theorem describes a periodicity in the homotopy groups of classical groups, discovered by , which proved to be of foundational significance for much further research, in particular in K-theory of stable co ...
was a central theme in Atiyah's work on K-theory, and he repeatedly returned to it, reworking the proof several times to understand it better. With Bott he worked out an elementary proof, and gave another version of it in his book. With Bott and Shapiro
Shapiro, and its variations such as Shapira, Schapiro, Schapira, Sapir, Sapira, Spira, Sapiro, Spiro (name)/Spyro (in Greek), Szapiro/Szpiro (in Polish) and Chapiro (in French), is a Jewish Ashkenazi surname.
Etymology
The surname is derived ...
he analysed the relation of Bott periodicity to the periodicity of Clifford algebras
In mathematics, a Clifford algebra is an algebra generated by a vector space with a quadratic form, and is a unital associative algebra. As -algebras, they generalize the real numbers, complex numbers, quaternions and several other hypercomp ...
; although this paper did not have a proof of the periodicity theorem, a proof along similar lines was shortly afterwards found by R. Wood. He found a proof of several generalizations using elliptic operators; this new proof used an idea that he used to give a particularly short and easy proof of Bott's original periodicity theorem.
Index theory (1963–1984)
 Atiyah's work on index theory is reprinted in volumes 3 and 4 of his collected works.
The index of a differential operator is closely related to the number of independent solutions (more precisely, it is the differences of the numbers of independent solutions of the differential operator and its adjoint). There are many hard and fundamental problems in mathematics that can easily be reduced to the problem of finding the number of independent solutions of some differential operator, so if one has some means of finding the index of a differential operator these problems can often be solved. This is what the Atiyah–Singer index theorem does: it gives a formula for the index of certain differential operators, in terms of topological invariants that look quite complicated but are in practice usually straightforward to calculate.
Several deep theorems, such as the Hirzebruch–Riemann–Roch theorem, are special cases of the Atiyah–Singer index theorem. In fact the index theorem gave a more powerful result, because its proof applied to all compact complex manifolds, while Hirzebruch's proof only worked for projective manifolds. There were also many new applications: a typical one is calculating the dimensions of the moduli spaces of instantons. The index theorem can also be run "in reverse": the index is obviously an integer, so the formula for it must also give an integer, which sometimes gives subtle integrality conditions on invariants of manifolds. A typical example of this is
Atiyah's work on index theory is reprinted in volumes 3 and 4 of his collected works.
The index of a differential operator is closely related to the number of independent solutions (more precisely, it is the differences of the numbers of independent solutions of the differential operator and its adjoint). There are many hard and fundamental problems in mathematics that can easily be reduced to the problem of finding the number of independent solutions of some differential operator, so if one has some means of finding the index of a differential operator these problems can often be solved. This is what the Atiyah–Singer index theorem does: it gives a formula for the index of certain differential operators, in terms of topological invariants that look quite complicated but are in practice usually straightforward to calculate.
Several deep theorems, such as the Hirzebruch–Riemann–Roch theorem, are special cases of the Atiyah–Singer index theorem. In fact the index theorem gave a more powerful result, because its proof applied to all compact complex manifolds, while Hirzebruch's proof only worked for projective manifolds. There were also many new applications: a typical one is calculating the dimensions of the moduli spaces of instantons. The index theorem can also be run "in reverse": the index is obviously an integer, so the formula for it must also give an integer, which sometimes gives subtle integrality conditions on invariants of manifolds. A typical example of this is Rochlin's theorem
In 4-dimensional topology, a branch of mathematics, Rokhlin's theorem states that if a smooth, closed 4-manifold ''M'' has a spin structure (or, equivalently, the second Stiefel–Whitney class w_2(M) vanishes), then the signature of its intersect ...
, which follows from the index theorem.
The index problem for elliptic differential operators was posed in 1959 by Gel'fand. He noticed the homotopy invariance of the index, and asked for a formula for it by means of topological invariants. Some of the motivating examples included the Riemann–Roch theorem
The Riemann–Roch theorem is an important theorem in mathematics, specifically in complex analysis and algebraic geometry, for the computation of the dimension of the space of meromorphic functions with prescribed zeros and allowed poles. It ...
and its generalization the Hirzebruch–Riemann–Roch theorem, and the Hirzebruch signature theorem. Hirzebruch and Borel had proved the integrality of the  genus of a spin manifold, and Atiyah suggested that this integrality could be explained if it were the index of the Dirac operator (which was rediscovered by Atiyah and Singer in 1961).
The first announcement of the Atiyah–Singer theorem was their 1963 paper. The proof sketched in this announcement was inspired by Hirzebruch's proof of the Hirzebruch–Riemann–Roch theorem and was never published by them, though it is described in the book by Palais. Their first published proof was more similar to Grothendieck's proof of the Grothendieck–Riemann–Roch theorem, replacing the cobordism theory of the first proof with K-theory, and they used this approach to give proofs of various generalizations in a sequence of papers from 1968 to 1971.
Instead of just one elliptic operator, one can consider a family of elliptic operators parameterized by some space ''Y''. In this case the index is an element of the K-theory of ''Y'', rather than an integer. If the operators in the family are real, then the index lies in the real K-theory of ''Y''. This gives a little extra information, as the map from the real K theory of ''Y'' to the complex K theory is not always injective.
 With Bott, Atiyah found an analogue of the
With Bott, Atiyah found an analogue of the Lefschetz fixed-point formula
In mathematics, the Lefschetz fixed-point theorem is a formula that counts the fixed points of a continuous mapping from a compact topological space X to itself by means of traces of the induced mappings on the homology groups of X. It is named ...
for elliptic operators, giving the Lefschetz number of an endomorphism of an elliptic complex in terms of a sum over the fixed points of the endomorphism. As special cases their formula included the Weyl character formula, and several new results about elliptic curves with complex multiplication, some of which were initially disbelieved by experts.
Atiyah and Segal combined this fixed point theorem with the index theorem as follows.
If there is a compact group action of a group ''G'' on the compact manifold ''X'', commuting with the elliptic operator, then one can replace ordinary K theory in the index theorem with equivariant K-theory.
For trivial groups ''G'' this gives the index theorem, and for a finite group ''G'' acting with isolated fixed points it gives the Atiyah–Bott fixed point theorem. In general it gives the index as a sum over fixed point submanifolds of the group ''G''.
Atiyah solved a problem asked independently by Hörmander and Gel'fand, about whether complex powers of analytic functions define distributions. Atiyah used Hironaka's resolution of singularities to answer this affirmatively. An ingenious and elementary solution was found at about the same time by J. Bernstein
Joseph Bernstein (sometimes spelled I. N. Bernshtein; he, יוס(י)ף נאומוביץ ברנשטיין; russian: Иосиф Наумович Бернштейн; born 18 April 1945) is a Soviet-born Israeli mathematician working at Tel Aviv Univ ...
, and discussed by Atiyah.
As an application of the equivariant index theorem, Atiyah and Hirzebruch showed that manifolds with effective circle actions have vanishing Â-genus
In mathematics, a genus of a multiplicative sequence is a ring homomorphism from the ring of smooth compact manifolds up to the equivalence of bounding a smooth manifold with boundary (i.e., up to suitable cobordism) to another ring, usually the ...
. (Lichnerowicz showed that if a manifold has a metric of positive scalar curvature then the Â-genus vanishes.)
With Elmer Rees, Atiyah studied the problem of the relation between topological and holomorphic vector bundles on projective space. They solved the simplest unknown case, by showing that all rank 2 vector bundles over projective 3-space have a holomorphic structure. Horrocks had previously found some non-trivial examples of such vector bundles, which were later used by Atiyah in his study of instantons on the 4-sphere.
 Atiyah, Bott and Vijay K. Patodi gave a new proof of the index theorem using the heat equation.
If the
Atiyah, Bott and Vijay K. Patodi gave a new proof of the index theorem using the heat equation.
If the manifold
In mathematics, a manifold is a topological space that locally resembles Euclidean space near each point. More precisely, an n-dimensional manifold, or ''n-manifold'' for short, is a topological space with the property that each point has a ...
is allowed to have boundary, then some restrictions must be put on the domain of the elliptic operator in order to ensure a finite index. These conditions can be local (like demanding that the sections in the domain vanish at the boundary) or more complicated global conditions (like requiring that the sections in the domain solve some differential equation). The local case was worked out by Atiyah and Bott, but they showed that many interesting operators (e.g., the signature operator) do not admit local boundary conditions. To handle these operators, Atiyah, Patodi and Singer introduced global boundary conditions equivalent to attaching a cylinder to the manifold along the boundary and then restricting the domain to those sections that are square integrable along the cylinder, and also introduced the Atiyah–Patodi–Singer eta invariant. This resulted in a series of papers on spectral asymmetry, which were later unexpectedly used in theoretical physics, in particular in Witten's work on anomalies.
 The fundamental solutions of linear hyperbolic partial differential equations often have Petrovsky lacunas: regions where they vanish identically. These were studied in 1945 by
The fundamental solutions of linear hyperbolic partial differential equations often have Petrovsky lacunas: regions where they vanish identically. These were studied in 1945 by I. G. Petrovsky
Ivan Georgievich Petrovsky (russian: Ива́н Гео́ргиевич Петро́вский) (18 January 1901 – 15 January 1973) (the family name is also transliterated as Petrovskii or Petrowsky) was a Soviet mathematician working mainly in ...
, who found topological conditions describing which regions were lacunas.
In collaboration with Bott and Lars Gårding, Atiyah wrote three papers updating and generalizing Petrovsky's work.
Atiyah showed how to extend the index theorem to some non-compact manifolds, acted on by a discrete group with compact quotient. The kernel of the elliptic operator is in general infinite-dimensional in this case, but it is possible to get a finite index using the dimension of a module over a von Neumann algebra; this index is in general real rather than integer valued. This version is called the ''L2 index theorem,'' and was used by Atiyah and Schmid to give a geometric construction, using square integrable harmonic spinors, of Harish-Chandra's discrete series representation
In mathematics, a discrete series representation is an irreducible unitary representation of a locally compact topological group ''G'' that is a subrepresentation of the left regular representation of ''G'' on L²(''G''). In the Plancherel mea ...
s of semisimple Lie groups. In the course of this work they found a more elementary proof of Harish-Chandra's fundamental theorem on the local integrability of characters of Lie groups.
With H. Donnelly and I. Singer, he extended Hirzebruch's formula (relating the signature defect at cusps of Hilbert modular surfaces to values of L-functions) from real quadratic fields to all totally real fields.
Gauge theory (1977–1985)
ADHM construction
In mathematical physics and gauge theory, the ADHM construction or monad construction is the construction of all instantons using methods of linear algebra by Michael Atiyah, Vladimir Drinfeld, Nigel Hitchin, Yuri I. Manin in their paper "Const ...
of all instantons on a sphere; Manin and Drinfeld found the same construction at the same time, leading to a joint paper by all four authors. Atiyah reformulated this construction using quaternion
In mathematics, the quaternion number system extends the complex numbers. Quaternions were first described by the Irish mathematician William Rowan Hamilton in 1843 and applied to mechanics in three-dimensional space. Hamilton defined a quat ...
s and wrote up a leisurely account of this classification of instantons on Euclidean space as a book.
Atiyah's work on instanton moduli spaces was used in Donaldson's work on Donaldson theory. Donaldson showed that the moduli space of (degree 1) instantons over a compact simply connected 4-manifold with positive definite intersection form can be compactified to give a cobordism between the manifold and a sum of copies of complex projective space. He deduced from this that the intersection form must be a sum of one-dimensional ones, which led to several spectacular applications to smooth 4-manifolds, such as the existence of non-equivalent smooth structures on 4-dimensional Euclidean space. Donaldson went on to use the other moduli spaces studied by Atiyah to define Donaldson invariants, which revolutionized the study of smooth 4-manifolds, and showed that they were more subtle than smooth manifolds in any other dimension, and also quite different from topological 4-manifolds. Atiyah described some of these results in a survey talk.
Green's functions for linear partial differential equations can often be found by using the Fourier transform
A Fourier transform (FT) is a mathematical transform that decomposes functions into frequency components, which are represented by the output of the transform as a function of frequency. Most commonly functions of time or space are transformed, ...
to convert this into an algebraic problem. Atiyah used a non-linear version of this idea. He used the Penrose transform to convert the Green's function for the conformally invariant Laplacian into a complex analytic object, which turned out to be essentially the diagonal embedding of the Penrose twistor space into its square. This allowed him to find an explicit formula for the conformally invariant Green's function on a 4-manifold.
In his paper with Jones, he studied the topology of the moduli space of SU(2) instantons over a 4-sphere. They showed that the natural map from this moduli space to the space of all connections induces epimorphisms of homology groups in a certain range of dimensions, and suggested that it might induce isomorphisms of homology groups in the same range of dimensions. This became known as the Atiyah–Jones conjecture, and was later proved by several mathematicians.
Harder and M. S. Narasimhan described the cohomology of the moduli spaces of stable vector bundles over Riemann surface
In mathematics, particularly in complex analysis, a Riemann surface is a connected one-dimensional complex manifold. These surfaces were first studied by and are named after Bernhard Riemann. Riemann surfaces can be thought of as deformed ve ...
s by counting the number of points of the moduli spaces over finite fields, and then using the Weil conjectures to recover the cohomology over the complex numbers.
Atiyah and R. Bott
Raoul Bott (September 24, 1923 – December 20, 2005) was a Hungarian-American mathematician known for numerous basic contributions to geometry in its broad sense. He is best known for his Bott periodicity theorem, the Morse–Bott functions whi ...
used Morse theory and the Yang–Mills equations over a Riemann surface
In mathematics, particularly in complex analysis, a Riemann surface is a connected one-dimensional complex manifold. These surfaces were first studied by and are named after Bernhard Riemann. Riemann surfaces can be thought of as deformed ve ...
to reproduce and extending the results of Harder and Narasimhan.
An old result due to Schur and Horn states that the set of possible diagonal vectors of an Hermitian matrix with given eigenvalues is the convex hull of all the permutations of the eigenvalues. Atiyah proved a generalization of this that applies to all compact symplectic manifolds acted on by a torus, showing that the image of the manifold under the moment map is a convex polyhedron, and with Pressley gave a related generalization to infinite-dimensional loop groups.
Duistermaat and Heckman found a striking formula, saying that the push-forward of the Liouville measure of a moment map In mathematics, specifically in symplectic geometry, the momentum map (or, by false etymology, moment map) is a tool associated with a Hamiltonian action of a Lie group on a symplectic manifold, used to construct conserved quantities for the actio ...
for a torus action is given exactly by the stationary phase approximation (which is in general just an asymptotic expansion rather than exact). Atiyah and Bott showed that this could be deduced from a more general formula in equivariant cohomology, which was a consequence of well-known localization theorems. Atiyah showed that the moment map was closely related to geometric invariant theory, and this idea was later developed much further by his student F. Kirwan. Witten shortly after applied the Duistermaat–Heckman formula to loop spaces and showed that this formally gave the Atiyah–Singer index theorem for the Dirac operator; this idea was lectured on by Atiyah.
With Hitchin he worked on magnetic monopoles, and studied their scattering using an idea of Nick Manton
Nicholas Stephen Manton (born 2 October 1952 in the City of Westminster)Autobiographical Memoir
p.7< ...
. His book with Hitchin gives a detailed description of their work on magnetic monopoles. The main theme of the book is a study of a moduli space of magnetic monopoles; this has a natural Riemannian metric, and a key point is that this metric is complete and hyperkähler. The metric is then used to study the scattering of two monopoles, using a suggestion of N. Manton that the geodesic flow on the moduli space is the low energy approximation to the scattering. For example, they show that a head-on collision between two monopoles results in 90-degree scattering, with the direction of scattering depending on the relative phases of the two monopoles. He also studied monopoles on hyperbolic space.
Atiyah showed that instantons in 4 dimensions can be identified with instantons in 2 dimensions, which are much easier to handle. There is of course a catch: in going from 4 to 2 dimensions the structure group of the gauge theory changes from a finite-dimensional group to an infinite-dimensional loop group. This gives another example where the moduli spaces of solutions of two apparently unrelated nonlinear partial differential equations turn out to be essentially the same.
Atiyah and Singer found that anomalies in quantum field theory could be interpreted in terms of index theory of the Dirac operator; this idea later became widely used by physicists.
p.7< ...
Later work (1986–2019)
 Many of the papers in the 6th volume of his collected works are surveys, obituaries, and general talks. Atiyah continued to publish subsequently, including several surveys, a popular book, and another paper with Segal on twisted K-theory.
One paper is a detailed study of the
Many of the papers in the 6th volume of his collected works are surveys, obituaries, and general talks. Atiyah continued to publish subsequently, including several surveys, a popular book, and another paper with Segal on twisted K-theory.
One paper is a detailed study of the Dedekind eta function
In mathematics, the Dedekind eta function, named after Richard Dedekind, is a modular form of weight 1/2 and is a function defined on the upper half-plane of complex numbers, where the imaginary part is positive. It also occurs in bosonic string ...
from the point of view of topology and the index theorem.
Several of his papers from around this time study the connections between quantum field theory, knots, and Donaldson theory. He introduced the concept of a topological quantum field theory, inspired by Witten's work and Segal's definition of a conformal field theory. His book “The Geometry and Physics of Knots” describes the new knot invariants found by Vaughan Jones and Edward Witten in terms of topological quantum field theories, and his paper with L. Jeffrey explains Witten's Lagrangian giving the Donaldson invariants.
He studied skyrmions with Nick Manton, finding a relation with magnetic monopoles and instantons, and giving a conjecture for the structure of the moduli space of two skyrmions as a certain subquotient of complex projective 3-space.
Several papers were inspired by a question oJonathan Robbins
(called the Berry–Robbins problem), who asked if there is a map from the configuration space of ''n'' points in 3-space to the flag manifold of the unitary group. Atiyah gave an affirmative answer to this question, but felt his solution was too computational and studied a conjecture that would give a more natural solution. He also related the question to
Nahm's equation In differential geometry and gauge theory (mathematics), gauge theory, the Nahm equations are a system of ordinary differential equations introduced by Werner Nahm in the context of the ''Nahm transform'' – an alternative to Richard S. Ward, W ...
, and introduced the Atiyah conjecture on configurations.
With Juan Maldacena and Cumrun Vafa, and E. Witten
Edward Witten (born August 26, 1951) is an American mathematical and theoretical physicist. He is a Professor Emeritus in the School of Natural Sciences at the Institute for Advanced Study in Princeton. Witten is a researcher in string theory, q ...
he described the dynamics of M-theory
M-theory is a theory in physics that unifies all consistent versions of superstring theory. Edward Witten first conjectured the existence of such a theory at a string theory conference at the University of Southern California in 1995. Witte ...
on manifolds with G2 holonomy. These papers seem to be the first time that Atiyah worked on exceptional Lie groups.
In his papers with M. Hopkins and G. Segal he returned to his earlier interest of K-theory, describing some twisted forms of K-theory with applications in theoretical physics.
In October 2016, he claimed a short proof of the non-existence of complex structures on the 6-sphere. His proof, like many predecessors, is considered flawed by the mathematical community, even after the proof was rewritten in a revised form.
At the 2018 Heidelberg Laureate Forum
Heidelberg (; Palatine German: ') is a city in the German state of Baden-Württemberg, situated on the river Neckar in south-west Germany. As of the 2016 census, its population was 159,914, of which roughly a quarter consisted of students.
...
, he claimed to have solved the Riemann hypothesis
In mathematics, the Riemann hypothesis is the conjecture that the Riemann zeta function has its zeros only at the negative even integers and complex numbers with real part . Many consider it to be the most important unsolved problem in pu ...
, Hilbert's eighth problem
Hilbert's eighth problem is one of David Hilbert's list of open mathematical problems posed in 1900. It concerns number theory, and in particular the Riemann hypothesis, although it is also concerned with the Goldbach Conjecture. The problem as st ...
, by contradiction using the fine-structure constant. Again, the proof did not hold up and the hypothesis remains one of the six unsolved Millennium Prize Problems in mathematics, as of 2022.
Bibliography
Books
This subsection lists all books written by Atiyah; it omits a few books that he edited. *. A classic textbook covering standard commutative algebra. *. Reprinted as . *. Reprinted as . *. Reprinted as . *. Reprinted as . *. *. *. *. *. *. First edition (1967) reprinted as . *. Reprinted as . *. * *. *.Selected papers
*. Reprinted in . *. Reprinted in . *. Reprinted in . *. Reprinted in . Formulation of the Atiyah "Conjecture" on the rationality of the L2-Betti numbers. *. An announcement of the index theorem. Reprinted in . *. This gives a proof using K theory instead of cohomology. Reprinted in . *. This reformulates the result as a sort of Lefschetz fixed point theorem, using equivariant K theory. Reprinted in . *. This paper shows how to convert from the K-theory version to a version using cohomology. Reprinted in . * This paper studies families of elliptic operators, where the index is now an element of the K-theory of the space parametrizing the family. Reprinted in . *. This studies families of real (rather than complex) elliptic operators, when one can sometimes squeeze out a little extra information. Reprinted in . *. This states a theorem calculating the Lefschetz number of an endomorphism of an elliptic complex. Reprinted in . * (reprinted in )and . Reprinted in . These give the proofs and some applications of the results announced in the previous paper. *; Reprinted in . *; . Reprinted in . *Awards and honours
 In 1966, when he was thirty-seven years old, he was awarded the Fields Medal, for his work in developing K-theory, a generalized Lefschetz fixed-point theorem and the Atiyah–Singer theorem, for which he also won the Abel Prize jointly with Isadore Singer in 2004.
Among other prizes he has received are the
In 1966, when he was thirty-seven years old, he was awarded the Fields Medal, for his work in developing K-theory, a generalized Lefschetz fixed-point theorem and the Atiyah–Singer theorem, for which he also won the Abel Prize jointly with Isadore Singer in 2004.
Among other prizes he has received are the Royal Medal
The Royal Medal, also known as The Queen's Medal and The King's Medal (depending on the gender of the monarch at the time of the award), is a silver-gilt medal, of which three are awarded each year by the Royal Society, two for "the most important ...
of the Royal Society
The Royal Society, formally The Royal Society of London for Improving Natural Knowledge, is a learned society and the United Kingdom's national academy of sciences. The society fulfils a number of roles: promoting science and its benefits, r ...
in 1968, the De Morgan Medal of the London Mathematical Society
The London Mathematical Society (LMS) is one of the United Kingdom's learned societies for mathematics (the others being the Royal Statistical Society (RSS), the Institute of Mathematics and its Applications (IMA), the Edinburgh Mathematical S ...
in 1980, the Antonio Feltrinelli Prize from the Accademia Nazionale dei Lincei in 1981, the King Faisal International Prize for Science
The King Faisal Foundation ( ar, مؤسسة الملك فيصل الخيرية; ''KFF''), is an international philanthropic organization established in 1976 with the intent of preserving and perpetuating King Faisal bin Abdulaziz's legacy. The fo ...
in 1987, the Copley Medal
The Copley Medal is an award given by the Royal Society, for "outstanding achievements in research in any branch of science". It alternates between the physical sciences or mathematics and the biological sciences. Given every year, the medal is t ...
of the Royal Society in 1988, the Benjamin Franklin Medal for Distinguished Achievement in the Sciences of the American Philosophical Society
The American Philosophical Society (APS), founded in 1743 in Philadelphia, is a scholarly organization that promotes knowledge in the sciences and humanities through research, professional meetings, publications, library resources, and communi ...
in 1993, the Jawaharlal Nehru Birth Centenary Medal
of the Indian National Science Academy
The Indian National Science Academy (INSA) is a national academy in New Delhi
New Delhi (, , ''Naī Dillī'') is the Capital city, capital of India and a part of the NCT Delhi, National Capital Territory of Delhi (NCT). New Delhi is t ...
in 1993, the President's Medal from the Institute of Physics
The Institute of Physics (IOP) is a UK-based learned society and professional body that works to advance physics education, research and application.
It was founded in 1874 and has a worldwide membership of over 20,000. The IOP is the Physica ...
in 2008, the Grande Médaille of the French Academy of Sciences
The French Academy of Sciences (French: ''Académie des sciences'') is a learned society, founded in 1666 by Louis XIV at the suggestion of Jean-Baptiste Colbert, to encourage and protect the spirit of French scientific research. It was at th ...
in 2010 and the Grand Officier of the French Légion d'honneur in 2011.
He was elected a foreign member of the National Academy of Sciences
The National Academy of Sciences (NAS) is a United States nonprofit, non-governmental organization. NAS is part of the National Academies of Sciences, Engineering, and Medicine, along with the National Academy of Engineering (NAE) and the Nat ...
, the American Academy of Arts and Sciences
The American Academy of Arts and Sciences (abbreviation: AAA&S) is one of the oldest learned societies in the United States. It was founded in 1780 during the American Revolution by John Adams, John Hancock, James Bowdoin, Andrew Oliver, ...
(1969), the Académie des Sciences
The French Academy of Sciences (French: ''Académie des sciences'') is a learned society, founded in 1666 by Louis XIV at the suggestion of Jean-Baptiste Colbert, to encourage and protect the spirit of French scientific research. It was at the ...
, the Akademie Leopoldina
The German National Academy of Sciences Leopoldina (german: Deutsche Akademie der Naturforscher Leopoldina – Nationale Akademie der Wissenschaften), short Leopoldina, is the national academy of Germany, and is located in Halle (Saale). Founded ...
, the Royal Swedish Academy
The Royal Swedish Academy of Sciences ( sv, Kungliga Vetenskapsakademien) is one of the royal academies of Sweden. Founded on 2 June 1739, it is an independent, non-governmental scientific organization that takes special responsibility for prom ...
, the Royal Irish Academy, the Royal Society of Edinburgh
The Royal Society of Edinburgh is Scotland's national academy of science and letters. It is a registered charity that operates on a wholly independent and non-partisan basis and provides public benefit throughout Scotland. It was established i ...
, the American Philosophical Society
The American Philosophical Society (APS), founded in 1743 in Philadelphia, is a scholarly organization that promotes knowledge in the sciences and humanities through research, professional meetings, publications, library resources, and communi ...
, the Indian National Science Academy
The Indian National Science Academy (INSA) is a national academy in New Delhi
New Delhi (, , ''Naī Dillī'') is the Capital city, capital of India and a part of the NCT Delhi, National Capital Territory of Delhi (NCT). New Delhi is t ...
, the Chinese Academy of Science
The Chinese Academy of Sciences (CAS); ), known by Academia Sinica in English until the 1980s, is the national academy of the People's Republic of China for natural sciences. It has historical origins in the Academia Sinica during the Republ ...
, the Australian Academy of Science
The Australian Academy of Science was founded in 1954 by a group of distinguished Australians, including Australian Fellows of the Royal Society of London. The first president was Sir Mark Oliphant. The academy is modelled after the Royal So ...
, the Russian Academy of Science, the Ukrainian Academy of Science
The National Academy of Sciences of Ukraine (NASU; uk, Національна академія наук України, ''Natsional’na akademiya nauk Ukrayiny'', abbr: NAN Ukraine) is a self-governing state-funded organization in Ukraine th ...
, the Georgian Academy of Science, the Venezuela Academy of Science
Venezuela (; ), officially the Bolivarian Republic of Venezuela ( es, link=no, República Bolivariana de Venezuela), is a country on the northern coast of South America, consisting of a continental landmass and many islands and islets in th ...
, the Norwegian Academy of Science and Letters
The Norwegian Academy of Science and Letters ( no, Det Norske Videnskaps-Akademi, DNVA) is a learned society based in Oslo, Norway. Its purpose is to support the advancement of science and scholarship in Norway.
History
The Royal Frederick Unive ...
, the Royal Spanish Academy of Science
The Spanish Royal Academy of Sciences ( Spanish: ''Real Academia de Ciencias Exactas, Físicas y Naturales'') is an academic institution and learned society that was founded in Madrid in 1847. It is dedicated to the study and research of mathe ...
, the Accademia dei Lincei and the Moscow Mathematical Society
The Moscow Mathematical Society (MMS) is a society of Moscow mathematicians aimed at the development of mathematics in Russia. It was created in 1864, and Victor Vassiliev is the current president.
History
The first meeting of the society wa ...
. In 2012, he became a fellow of the American Mathematical Society
The American Mathematical Society (AMS) is an association of professional mathematicians dedicated to the interests of mathematical research and scholarship, and serves the national and international community through its publications, meetings ...
. He was also appointed as a Honorary Fellow
A fellow is a concept whose exact meaning depends on context.
In learned or professional societies, it refers to a privileged member who is specially elected in recognition of their work and achievements.
Within the context of higher education ...
of the Royal Academy of Engineering in 1993.
Atiyah was awarded honorary degrees by the universities of Birmingham, Bonn, Chicago, Cambridge, Dublin, Durham, Edinburgh, Essex, Ghent, Helsinki, Lebanon, Leicester, London, Mexico, Montreal, Oxford, Reading, Salamanca, St. Andrews, Sussex, Wales, Warwick, the American University of Beirut, Brown University, Charles University in Prague, Harvard University, Heriot–Watt University, Hong Kong (Chinese University), Keele University, Queen's University (Canada), The Open University, University of Waterloo, Wilfrid Laurier University, Technical University of Catalonia, and UMIST.
Atiyah was made a Knight Bachelor
The title of Knight Bachelor is the basic rank granted to a man who has been knighted by the monarch but not inducted as a member of one of the organised orders of chivalry; it is a part of the British honours system. Knights Bachelor are t ...
in 1983 and made a member of the Order of Merit in 1992.
The Michael Atiyah building at the University of Leicester
, mottoeng = So that they may have life
, established =
, type = public research university
, endowment = £20.0 million
, budget = £326 million
, chancellor = David Willetts
, vice_chancellor = Nishan Canagarajah
, head_la ...
and the Michael Atiyah Chair in Mathematical Sciences at the American University of Beirut were named after him.
Personal life
Atiyah married Lily Brown on 30 July 1955, with whom he had three sons, John, David and Robin. Atiyah's eldest son John died on 24 June 2002 while on a walking holiday in thePyrenees
The Pyrenees (; es, Pirineos ; french: Pyrénées ; ca, Pirineu ; eu, Pirinioak ; oc, Pirenèus ; an, Pirineus) is a mountain range straddling the border of France and Spain. It extends nearly from its union with the Cantabrian Mountains to ...
with his wife Maj-Lis. Lily Atiyah died on 13 March 2018 at the age of 90.
Sir Michael Atiyah died on 11 January 2019, aged 89.
References
Sources
* * *. Reprinted in volume 1 of his collected works, p. 65–75, . On page 120 Gel'fand suggests that the index of an elliptic operator should be expressible in terms of topological data. * * *. This describes the original proof of the index theorem. (Atiyah and Singer never published their original proof themselves, but only improved versions of it.) *. *. *.External links
Michael Atiyah tells his life story
at Web of Stories
The celebrations of Michael Atiyah's 80th birthday in Edinburgh, 20-24 April 2009
* * * * * * * * * * * *
List of works of Michael Atiyah
fro
''Celebratio Mathematica''
* * {{DEFAULTSORT:Atiyah, Michael 1929 births 2019 deaths People from Hampstead Alumni of Trinity College, Cambridge 20th-century British mathematicians 21st-century British mathematicians Academics of the University of Edinburgh Abel Prize laureates Algebraic geometers Michael British humanists British people of Lebanese descent British people of Scottish descent Differential geometers Fellows of the American Academy of Arts and Sciences Fellows of the American Mathematical Society Fellows of New College, Oxford Fellows of Pembroke College, Cambridge Fellows of the Academy of Medical Sciences (United Kingdom) Fellows of the Australian Academy of Science Foreign Fellows of the Indian National Science Academy Fellows of the Royal Society of Edinburgh Fields Medalists Institute for Advanced Study faculty Knights Bachelor Masters of Trinity College, Cambridge Fellows of the Royal Society Members of the French Academy of Sciences Foreign associates of the National Academy of Sciences Foreign Members of the Russian Academy of Sciences Members of the Order of Merit Members of the Norwegian Academy of Science and Letters People educated at Manchester Grammar School People associated with the University of Leicester Presidents of the Royal Society Recipients of the Copley Medal Royal Medal winners Savilian Professors of Geometry Topologists Victoria College, Alexandria alumni Royal Electrical and Mechanical Engineers soldiers Members of the Royal Swedish Academy of Sciences