Killing vector field on:
[Wikipedia]
[Google]
[Amazon]
In
 The vector field on a circle that points clockwise and has the same length at each point is a Killing vector field, since moving each point on the circle along this vector field simply rotates the circle.
The vector field on a circle that points clockwise and has the same length at each point is a Killing vector field, since moving each point on the circle along this vector field simply rotates the circle.
 A toy example for a Killing vector field is on the upper half-plane equipped with the Poincaré metric . The pair is typically called the hyperbolic plane and has Killing vector field (using standard coordinates). This should be intuitively clear since the covariant derivative transports the metric along an integral curve generated by the vector field (whose image is parallel to the x-axis).
Furthermore, the metric is independent of from which we can immediately conclude that is a Killing field using one of the results below in this article.
The isometry group of the upper half-plane model (or rather, the component connected to the identity) is (see
A toy example for a Killing vector field is on the upper half-plane equipped with the Poincaré metric . The pair is typically called the hyperbolic plane and has Killing vector field (using standard coordinates). This should be intuitively clear since the covariant derivative transports the metric along an integral curve generated by the vector field (whose image is parallel to the x-axis).
Furthermore, the metric is independent of from which we can immediately conclude that is a Killing field using one of the results below in this article.
The isometry group of the upper half-plane model (or rather, the component connected to the identity) is (see
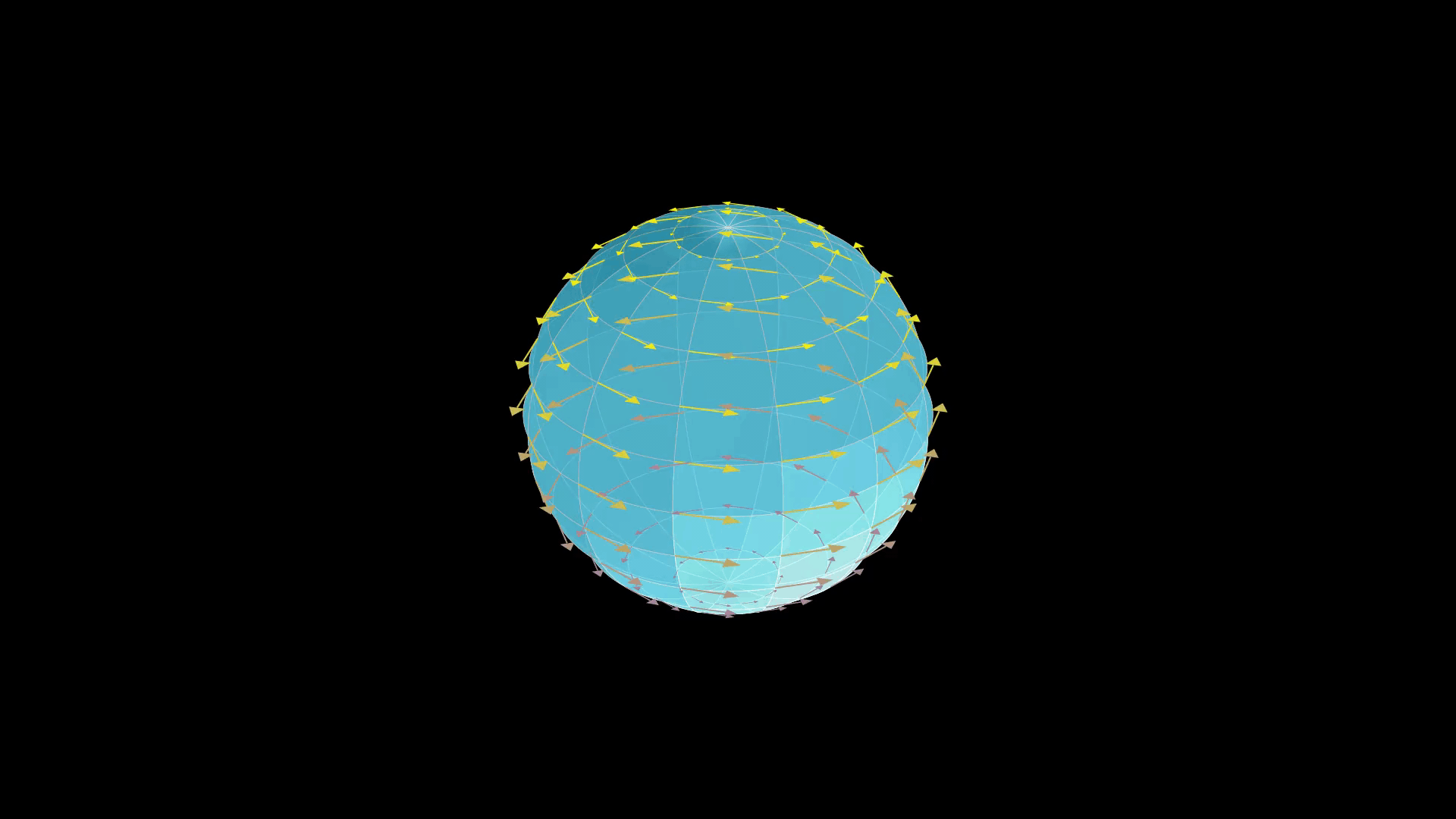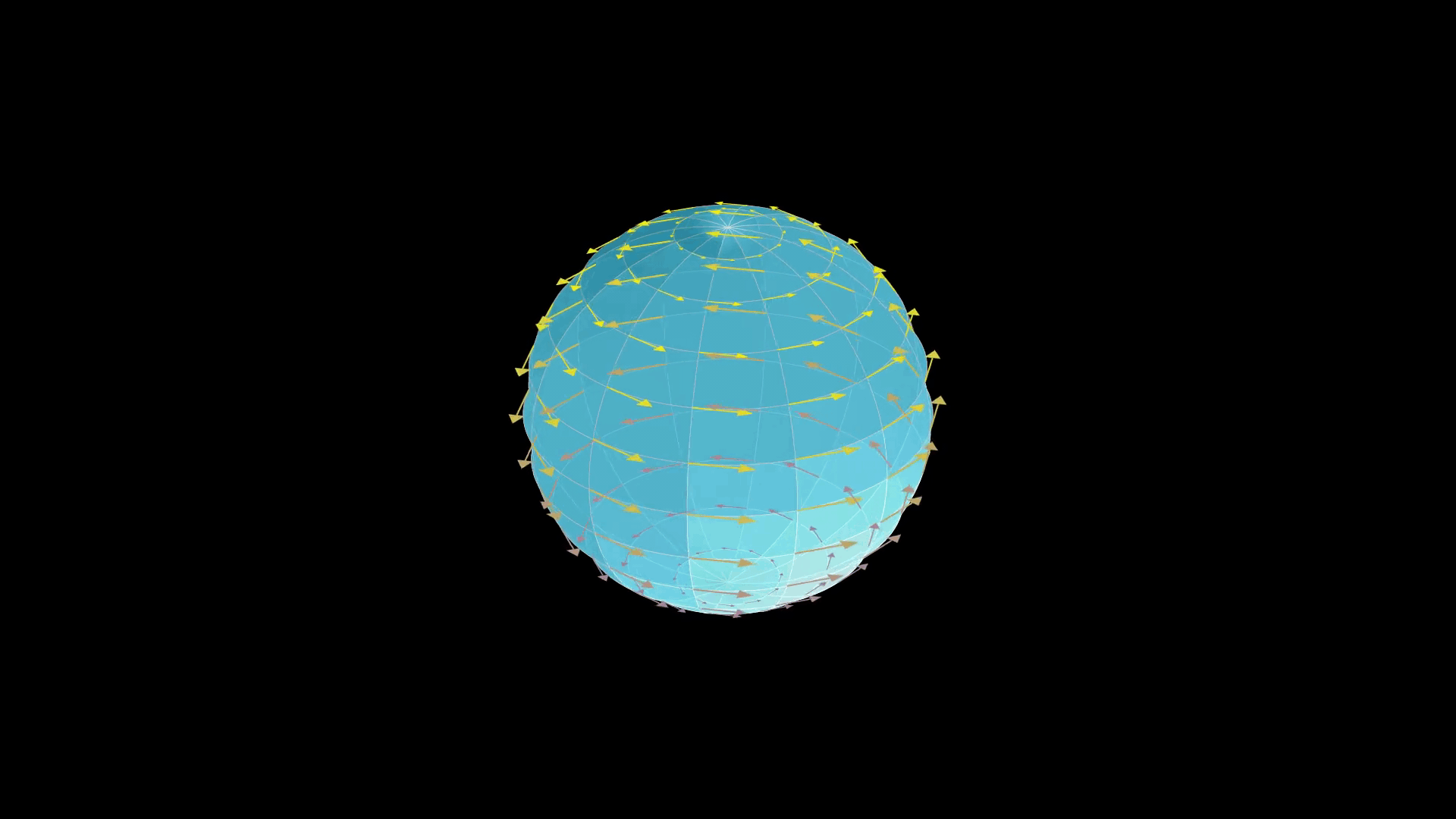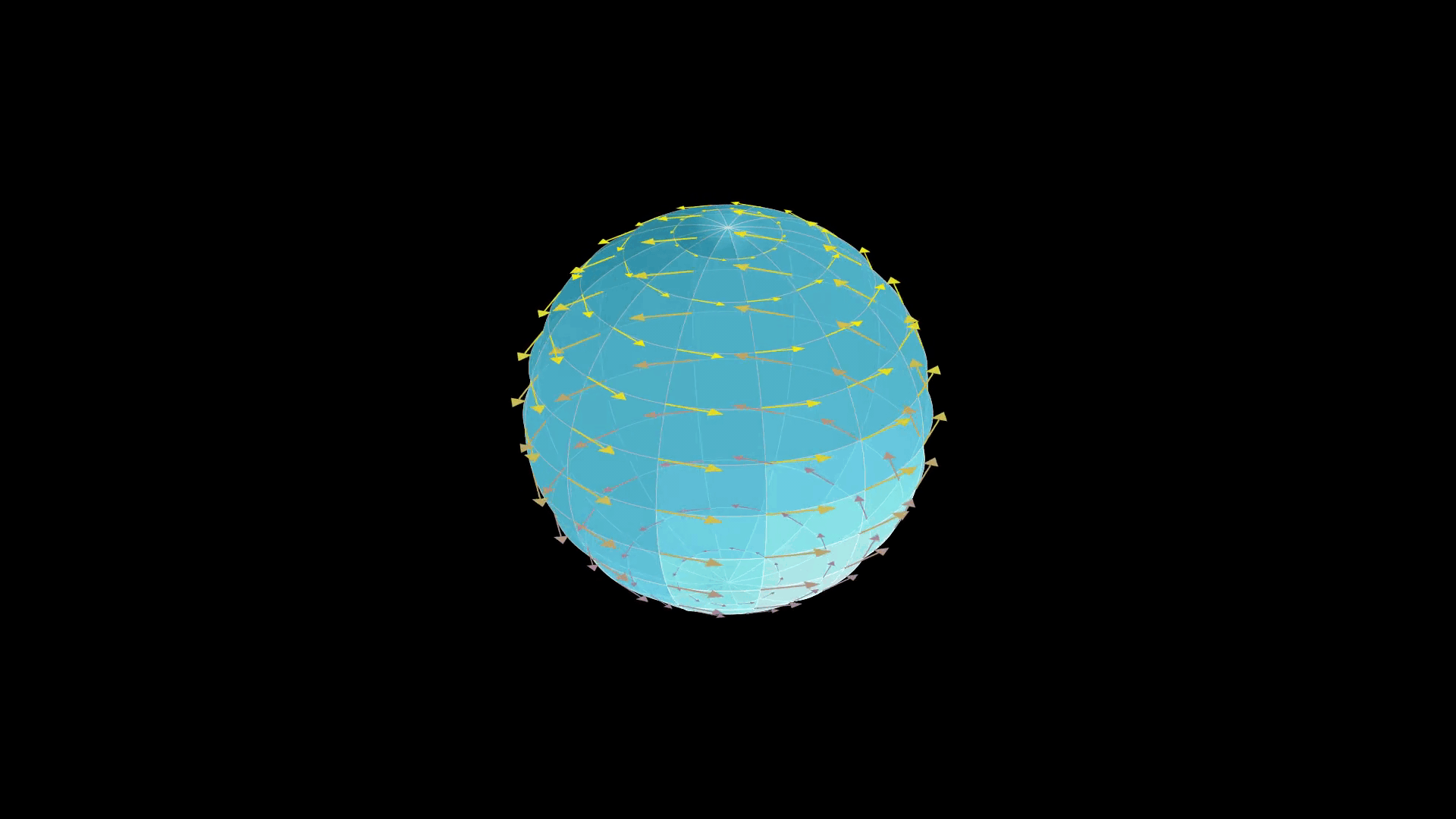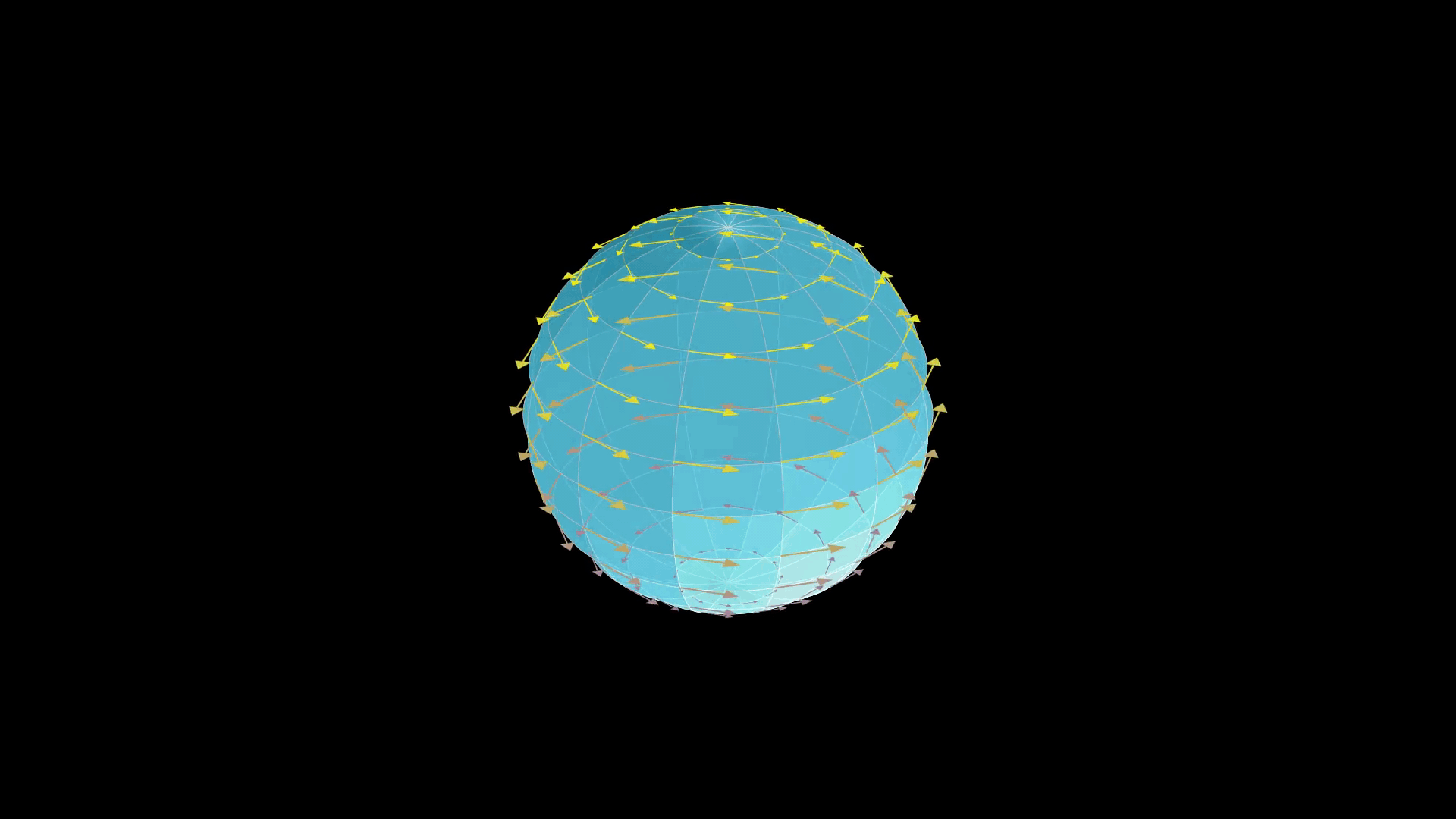 The Killing fields of the two-sphere , or more generally the -sphere should be "obvious" from ordinary intuition: spheres, having rotational symmetry, should possess Killing fields which generate rotations about any axis. That is, we expect to have symmetry under the action of the 3D rotation group SO(3).
When explicitly expressed in terms of the standard coordinate chart for , the Killing fields have a non-obvious structure that obscures their nature. This is articulated below. This "non-obvious" structure is generic to manifolds that are not spheres, and thus the 2-sphere provides a good toy model on which to explore the intuitive interpretation of Killing fields.
The conventional chart for the 2-sphere embedded in in Cartesian coordinates is given by
:
so that parametrises the height, and parametrises rotation about the -axis.
Pulling back the standard Cartesian metric to these coordinates gives the standard metric on the sphere,
:.
Intuitively, a rotation about any axis should be an isometry. In this chart, we can immediately write down the vector field which generates rotations about the -axis:
:
Note that in these coordinates, the metric components are all independent of , which is another way for us to see that is a Killing vector (see below).
Naïvely we might hope to find another Killing vector
:
The metric is dependent on , so we cannot immediately deduce that it is a Killing vector. In fact, explicitly evaluating Killing's equation reveals it is not a Killing field. Intuitively, the flow generated by moves points downwards. Near , points move apart, thus distorting the metric, and we can see it is not an isometry, and therefore not a Killing field.
Killing fields have the property that the Lie bracket of two Killing fields is still a Killing field. Thus, the Killing fields on a manifold ''M'' form a Lie subalgebra of vector fields on ''M''. For example, we may wish to compute the dimension of this algebra and its structure constants, and possibly identify the Lie algebra.
Since are a coordinate basis, their commutator vanishes. Unfortunately, as earlier mentioned, is not a Killing field. In these coordinates, it is difficult to guess other Killing fields.
We can realize the algebra of Killing fields by considering as an embedded manifold of , and work in orthonormal Cartesian coordinates where commutators will turn out to be straight-forward.
The generator is recognized as a rotation about the -axis
:
A second generator, rotations about the -axis, is
:
Commuting these two, one finds a third generator for rotations about the -axis
:
The algebra given by linear combinations of these three generators closes, and we have the relations
: We identify this as the Lie algebra
Expressing and in terms of spherical coordinates gives
:
and
:
We'd like to say these three Killing fields are a complete set of generators for the algebra. One way to see this is that as a submanifold of , its symmetries are a strict subset of those of . The symmetries of are generated by rotations and translations. This can be shown using Killing's equation. Translations do not preserve the unit sphere, but rotations do. Hence this is the complete set of generators of the algebra of Killing fields, which we can identify with the algebra of rotations.
The Killing fields of the two-sphere , or more generally the -sphere should be "obvious" from ordinary intuition: spheres, having rotational symmetry, should possess Killing fields which generate rotations about any axis. That is, we expect to have symmetry under the action of the 3D rotation group SO(3).
When explicitly expressed in terms of the standard coordinate chart for , the Killing fields have a non-obvious structure that obscures their nature. This is articulated below. This "non-obvious" structure is generic to manifolds that are not spheres, and thus the 2-sphere provides a good toy model on which to explore the intuitive interpretation of Killing fields.
The conventional chart for the 2-sphere embedded in in Cartesian coordinates is given by
:
so that parametrises the height, and parametrises rotation about the -axis.
Pulling back the standard Cartesian metric to these coordinates gives the standard metric on the sphere,
:.
Intuitively, a rotation about any axis should be an isometry. In this chart, we can immediately write down the vector field which generates rotations about the -axis:
:
Note that in these coordinates, the metric components are all independent of , which is another way for us to see that is a Killing vector (see below).
Naïvely we might hope to find another Killing vector
:
The metric is dependent on , so we cannot immediately deduce that it is a Killing vector. In fact, explicitly evaluating Killing's equation reveals it is not a Killing field. Intuitively, the flow generated by moves points downwards. Near , points move apart, thus distorting the metric, and we can see it is not an isometry, and therefore not a Killing field.
Killing fields have the property that the Lie bracket of two Killing fields is still a Killing field. Thus, the Killing fields on a manifold ''M'' form a Lie subalgebra of vector fields on ''M''. For example, we may wish to compute the dimension of this algebra and its structure constants, and possibly identify the Lie algebra.
Since are a coordinate basis, their commutator vanishes. Unfortunately, as earlier mentioned, is not a Killing field. In these coordinates, it is difficult to guess other Killing fields.
We can realize the algebra of Killing fields by considering as an embedded manifold of , and work in orthonormal Cartesian coordinates where commutators will turn out to be straight-forward.
The generator is recognized as a rotation about the -axis
:
A second generator, rotations about the -axis, is
:
Commuting these two, one finds a third generator for rotations about the -axis
:
The algebra given by linear combinations of these three generators closes, and we have the relations
: We identify this as the Lie algebra
Expressing and in terms of spherical coordinates gives
:
and
:
We'd like to say these three Killing fields are a complete set of generators for the algebra. One way to see this is that as a submanifold of , its symmetries are a strict subset of those of . The symmetries of are generated by rotations and translations. This can be shown using Killing's equation. Translations do not preserve the unit sphere, but rotations do. Hence this is the complete set of generators of the algebra of Killing fields, which we can identify with the algebra of rotations.
mathematics
Mathematics is an area of knowledge that includes the topics of numbers, formulas and related structures, shapes and the spaces in which they are contained, and quantities and their changes. These topics are represented in modern mathematics ...
, a Killing vector field (often called a Killing field), named after Wilhelm Killing, is a vector field on a Riemannian manifold
In differential geometry, a Riemannian manifold or Riemannian space , so called after the German mathematician Bernhard Riemann, is a real manifold, real, smooth manifold ''M'' equipped with a positive-definite Inner product space, inner product ...
(or pseudo-Riemannian manifold) that preserves the metric. Killing fields are the infinitesimal generators of isometries; that is, flow
Flow may refer to:
Science and technology
* Fluid flow, the motion of a gas or liquid
* Flow (geomorphology), a type of mass wasting or slope movement in geomorphology
* Flow (mathematics), a group action of the real numbers on a set
* Flow (psych ...
s generated by Killing fields are continuous isometries of the manifold
In mathematics, a manifold is a topological space that locally resembles Euclidean space near each point. More precisely, an n-dimensional manifold, or ''n-manifold'' for short, is a topological space with the property that each point has a n ...
. More simply, the flow generates a symmetry
Symmetry (from grc, συμμετρία "agreement in dimensions, due proportion, arrangement") in everyday language refers to a sense of harmonious and beautiful proportion and balance. In mathematics, "symmetry" has a more precise definit ...
, in the sense that moving each point of an object the same distance in the direction of the Killing vector will not distort distances on the object.
Definition
Specifically, a vector field ''X'' is a Killing field if the Lie derivative with respect to ''X'' of the metric ''g'' vanishes: : In terms of theLevi-Civita connection
In Riemannian or pseudo Riemannian geometry (in particular the Lorentzian geometry of general relativity), the Levi-Civita connection is the unique affine connection on the tangent bundle of a manifold (i.e. affine connection) that preserves th ...
, this is
:
for all vectors ''Y'' and ''Z''. In local coordinates, this amounts to the Killing equation
:
This condition is expressed in covariant form. Therefore, it is sufficient to establish it in a preferred coordinate system in order to have it hold in all coordinate systems.
Examples
Killing field on the circle
 The vector field on a circle that points clockwise and has the same length at each point is a Killing vector field, since moving each point on the circle along this vector field simply rotates the circle.
The vector field on a circle that points clockwise and has the same length at each point is a Killing vector field, since moving each point on the circle along this vector field simply rotates the circle.
Killing fields in flat space
Here we derive the Killing fields for general flat space. From Killing's equation and the Ricci identity for a covector , : (usingabstract index notation
Abstract index notation (also referred to as slot-naming index notation) is a mathematical notation for tensors and spinors that uses indices to indicate their types, rather than their components in a particular basis. The indices are mere placeho ...
) where is the Riemann curvature tensor, the following identity may be proven for a Killing field :
:
When the base manifold is flat space, that is, Euclidean space or possibly Pseudo-Euclidean space (as for Minkowski space), we can choose global flat coordinates such that in these coordinates, the Levi-Civita connection and hence Riemann curvature vanishes everywhere, giving
:
Integrating and imposing the Killing equation allows us to write the general solution to as
:
where is antisymmetric. By taking appropriate values of and , we get a basis for the generalised Poincaré algebra
Poincaré is a French surname. Notable people with the surname include:
* Henri Poincaré (1854–1912), French physicist, mathematician and philosopher of science
* Henriette Poincaré (1858-1943), wife of Prime Minister Raymond Poincaré
* Luci ...
of isometries of flat space:
:
:
These generate pseudo-rotations (rotations and boosts) and translations respectively. Intuitively these preserve the (pseudo)-metric at each point.
For (Pseudo-)Euclidean space of total dimension, in total there are generators, making flat space maximally symmetric. This number is generic for maximally symmetric spaces. Maximally symmetric spaces can be considered as sub-manifolds of flat space, arising as surfaces of constant proper distance
:
which have O(p,q) symmetry. If the submanifold has dimension , this group of symmetries has the expected dimension (as a Lie group
In mathematics, a Lie group (pronounced ) is a group that is also a differentiable manifold. A manifold is a space that locally resembles Euclidean space, whereas groups define the abstract concept of a binary operation along with the additio ...
).
Heuristically, we can derive the dimension of the Killing field algebra. Treating Killing's equation together with the identity as a system of second order differential equations for , we can determine the value of at any point given initial data at a point . The initial data specifies and , but Killing's equation imposes that the covariant derivative is antisymmetric. In total this is independent values of initial data.
For concrete examples, see below for examples of flat space (Minkowski space) and maximally symmetric spaces (sphere, hyperbolic space).
Killing fields on the hyperbolic plane
 A toy example for a Killing vector field is on the upper half-plane equipped with the Poincaré metric . The pair is typically called the hyperbolic plane and has Killing vector field (using standard coordinates). This should be intuitively clear since the covariant derivative transports the metric along an integral curve generated by the vector field (whose image is parallel to the x-axis).
Furthermore, the metric is independent of from which we can immediately conclude that is a Killing field using one of the results below in this article.
The isometry group of the upper half-plane model (or rather, the component connected to the identity) is (see
A toy example for a Killing vector field is on the upper half-plane equipped with the Poincaré metric . The pair is typically called the hyperbolic plane and has Killing vector field (using standard coordinates). This should be intuitively clear since the covariant derivative transports the metric along an integral curve generated by the vector field (whose image is parallel to the x-axis).
Furthermore, the metric is independent of from which we can immediately conclude that is a Killing field using one of the results below in this article.
The isometry group of the upper half-plane model (or rather, the component connected to the identity) is (see Poincaré half-plane model
In non-Euclidean geometry, the Poincaré half-plane model is the upper half-plane, denoted below as H = \, together with a metric, the Poincaré metric, that makes it a model of two-dimensional hyperbolic geometry.
Equivalently the Poincaré ha ...
), and the other two Killing fields may be derived from considering the action of the generators of on the upper half-plane. The other two generating Killing fields are dilatation and the special conformal transformation .
Killing fields on a 2-sphere
 The Killing fields of the two-sphere , or more generally the -sphere should be "obvious" from ordinary intuition: spheres, having rotational symmetry, should possess Killing fields which generate rotations about any axis. That is, we expect to have symmetry under the action of the 3D rotation group SO(3).
When explicitly expressed in terms of the standard coordinate chart for , the Killing fields have a non-obvious structure that obscures their nature. This is articulated below. This "non-obvious" structure is generic to manifolds that are not spheres, and thus the 2-sphere provides a good toy model on which to explore the intuitive interpretation of Killing fields.
The conventional chart for the 2-sphere embedded in in Cartesian coordinates is given by
:
so that parametrises the height, and parametrises rotation about the -axis.
Pulling back the standard Cartesian metric to these coordinates gives the standard metric on the sphere,
:.
Intuitively, a rotation about any axis should be an isometry. In this chart, we can immediately write down the vector field which generates rotations about the -axis:
:
Note that in these coordinates, the metric components are all independent of , which is another way for us to see that is a Killing vector (see below).
Naïvely we might hope to find another Killing vector
:
The metric is dependent on , so we cannot immediately deduce that it is a Killing vector. In fact, explicitly evaluating Killing's equation reveals it is not a Killing field. Intuitively, the flow generated by moves points downwards. Near , points move apart, thus distorting the metric, and we can see it is not an isometry, and therefore not a Killing field.
Killing fields have the property that the Lie bracket of two Killing fields is still a Killing field. Thus, the Killing fields on a manifold ''M'' form a Lie subalgebra of vector fields on ''M''. For example, we may wish to compute the dimension of this algebra and its structure constants, and possibly identify the Lie algebra.
Since are a coordinate basis, their commutator vanishes. Unfortunately, as earlier mentioned, is not a Killing field. In these coordinates, it is difficult to guess other Killing fields.
We can realize the algebra of Killing fields by considering as an embedded manifold of , and work in orthonormal Cartesian coordinates where commutators will turn out to be straight-forward.
The generator is recognized as a rotation about the -axis
:
A second generator, rotations about the -axis, is
:
Commuting these two, one finds a third generator for rotations about the -axis
:
The algebra given by linear combinations of these three generators closes, and we have the relations
: We identify this as the Lie algebra
Expressing and in terms of spherical coordinates gives
:
and
:
We'd like to say these three Killing fields are a complete set of generators for the algebra. One way to see this is that as a submanifold of , its symmetries are a strict subset of those of . The symmetries of are generated by rotations and translations. This can be shown using Killing's equation. Translations do not preserve the unit sphere, but rotations do. Hence this is the complete set of generators of the algebra of Killing fields, which we can identify with the algebra of rotations.
The Killing fields of the two-sphere , or more generally the -sphere should be "obvious" from ordinary intuition: spheres, having rotational symmetry, should possess Killing fields which generate rotations about any axis. That is, we expect to have symmetry under the action of the 3D rotation group SO(3).
When explicitly expressed in terms of the standard coordinate chart for , the Killing fields have a non-obvious structure that obscures their nature. This is articulated below. This "non-obvious" structure is generic to manifolds that are not spheres, and thus the 2-sphere provides a good toy model on which to explore the intuitive interpretation of Killing fields.
The conventional chart for the 2-sphere embedded in in Cartesian coordinates is given by
:
so that parametrises the height, and parametrises rotation about the -axis.
Pulling back the standard Cartesian metric to these coordinates gives the standard metric on the sphere,
:.
Intuitively, a rotation about any axis should be an isometry. In this chart, we can immediately write down the vector field which generates rotations about the -axis:
:
Note that in these coordinates, the metric components are all independent of , which is another way for us to see that is a Killing vector (see below).
Naïvely we might hope to find another Killing vector
:
The metric is dependent on , so we cannot immediately deduce that it is a Killing vector. In fact, explicitly evaluating Killing's equation reveals it is not a Killing field. Intuitively, the flow generated by moves points downwards. Near , points move apart, thus distorting the metric, and we can see it is not an isometry, and therefore not a Killing field.
Killing fields have the property that the Lie bracket of two Killing fields is still a Killing field. Thus, the Killing fields on a manifold ''M'' form a Lie subalgebra of vector fields on ''M''. For example, we may wish to compute the dimension of this algebra and its structure constants, and possibly identify the Lie algebra.
Since are a coordinate basis, their commutator vanishes. Unfortunately, as earlier mentioned, is not a Killing field. In these coordinates, it is difficult to guess other Killing fields.
We can realize the algebra of Killing fields by considering as an embedded manifold of , and work in orthonormal Cartesian coordinates where commutators will turn out to be straight-forward.
The generator is recognized as a rotation about the -axis
:
A second generator, rotations about the -axis, is
:
Commuting these two, one finds a third generator for rotations about the -axis
:
The algebra given by linear combinations of these three generators closes, and we have the relations
: We identify this as the Lie algebra
Expressing and in terms of spherical coordinates gives
:
and
:
We'd like to say these three Killing fields are a complete set of generators for the algebra. One way to see this is that as a submanifold of , its symmetries are a strict subset of those of . The symmetries of are generated by rotations and translations. This can be shown using Killing's equation. Translations do not preserve the unit sphere, but rotations do. Hence this is the complete set of generators of the algebra of Killing fields, which we can identify with the algebra of rotations.
Killing fields in Minkowski space
The Killing fields of Minkowski space are the 3 space translations, time translation, three generators of rotations (the little group) and the three generators of boosts. These are * Time and space translations *: * Vector fields generating three rotations, often called the ''J'' generators, *: * Vector fields generating three boosts, the ''K'' generators, *: The boosts and rotations generate the Lorentz group. Together with space-time translations, this forms the Lie algebra for the Poincaré group.Killing fields in general relativity
Killing fields are used to discuss isometries in general relativity (in which the geometry of spacetime as distorted bygravitational field
In physics, a gravitational field is a model used to explain the influences that a massive body extends into the space around itself, producing a force on another massive body. Thus, a gravitational field is used to explain gravitational phenome ...
s is viewed as a 4-dimensional pseudo-Riemannian manifold). In a static configuration, in which nothing changes with time, the time vector will be a Killing vector, and thus the Killing field will point in the direction of forward motion in time. For example, the Schwarzschild metric
In Einstein's theory of general relativity, the Schwarzschild metric (also known as the Schwarzschild solution) is an
exact solution to the Einstein field equations that describes the gravitational field outside a spherical mass, on the assumpti ...
has four Killing fields: the metric is independent of , hence is a time-like Killing field. The other three are the three generators of rotations discussed above. The Kerr metric for a rotating black hole has only two Killing fields: the time-like field, and a field generating rotations about the axis of rotation of the black hole.
de Sitter space and anti-de Sitter space are maximally symmetric spaces, with the -dimensional versions of each possessing Killing fields.
Killing field of a constant coordinate
If the metric coefficients in some coordinate basis are independent of one of the coordinates , then is a Killing vector, where is the Kronecker delta. To prove this, let us assume . Then and Now let us look at the Killing condition : and from . The Killing condition becomes : that is , which is true. * The physical meaning is, for example, that, if none of the metric coefficients is a function of time, the manifold must automatically have a time-like Killing vector. * In layman's terms, if an object doesn't transform or "evolve" in time (when time passes), time passing won't change the measures of the object. Formulated like this, the result sounds like a tautology, but one has to understand that the example is very much contrived: Killing fields apply also to much more complex and interesting cases. Conversely, if the metric admits a Killing field , then one can construct coordinates for which . These coordinates are constructed by taking a hypersurface such that is nowhere tangent to . Take coordinates on , then define local coordinates where denotes the parameter along the integral curve of based at on . In these coordinates, the Lie derivative reduces to the coordinate derivative, that is, : and by the definition of the Killing field the left-hand side vanishes.Properties
A Killing field is determined uniquely by a vector at some point and its gradient (i.e. allcovariant derivative
In mathematics, the covariant derivative is a way of specifying a derivative along tangent vectors of a manifold. Alternatively, the covariant derivative is a way of introducing and working with a connection on a manifold by means of a different ...
s of the field at the point).
The Lie bracket of two Killing fields is still a Killing field. The Killing fields on a manifold ''M'' thus form a Lie subalgebra of vector fields on ''M''. This is the Lie algebra of the isometry group of the manifold if ''M'' is complete
Complete may refer to:
Logic
* Completeness (logic)
* Completeness of a theory, the property of a theory that every formula in the theory's language or its negation is provable
Mathematics
* The completeness of the real numbers, which implies t ...
. A Riemannian manifold
In differential geometry, a Riemannian manifold or Riemannian space , so called after the German mathematician Bernhard Riemann, is a real manifold, real, smooth manifold ''M'' equipped with a positive-definite Inner product space, inner product ...
with a transitive group of isometries is a homogeneous space
In mathematics, particularly in the theories of Lie groups, algebraic groups and topological groups, a homogeneous space for a group ''G'' is a non-empty manifold or topological space ''X'' on which ''G'' acts transitively. The elements of ' ...
.
For compact manifolds
* Negative Ricci curvature implies there are no nontrivial (nonzero) Killing fields.
* Nonpositive Ricci curvature implies that any Killing field is parallel. i.e. covariant derivative along any vector field is identically zero.
* If the sectional curvature is positive and the dimension of ''M'' is even, a Killing field must have a zero.
The covariant divergence of every Killing vector field vanishes.
If is a Killing vector field and is a harmonic vector field, then is a harmonic function.
If is a Killing vector field and is a harmonic p-form, then
Geodesics
Each Killing vector corresponds to a quantity which is conserved along geodesics. This conserved quantity is the metric product between the Killing vector and the geodesic tangent vector. Along an affinely parametrized geodesic with tangent vector then given the Killing vector , the quantity is conserved: : This aids in analytically studying motions in a spacetime with symmetries.Stress-energy tensor
Given a conserved, symmetric tensor , that is, one satisfying and , which are properties typical of a stress-energy tensor, and a Killing vector , we can construct the conserved quantity satisfying :Cartan decomposition
As noted above, the Lie bracket of two Killing fields is still a Killing field. The Killing fields on a manifold thus form a Lie subalgebra of all vector fields on Selecting a point the algebra can be decomposed into two parts: : and : where is thecovariant derivative
In mathematics, the covariant derivative is a way of specifying a derivative along tangent vectors of a manifold. Alternatively, the covariant derivative is a way of introducing and working with a connection on a manifold by means of a different ...
. These two parts intersect trivially but do not in general split . For instance, if is a Riemannian homogeneous space, we have if and only if is a Riemannian symmetric space.
Intuitively, the isometries of locally define a submanifold of the total space, and the Killing fields show how to "slide along" that submanifold. They span the tangent space of that submanifold. The tangent space should have the same dimension as the isometries acting effectively at that point. That is, one expects Yet, in general, the number of Killing fields is larger than the dimension of that tangent space. How can this be? The answer is that the "extra" Killing fields are redundant. Taken all together, the fields provide an over-complete basis for the tangent space at any particular selected point; linear combinations can be made to vanish at that particular point. This was seen in the example of the Killing fields on a 2-sphere: there are 3 Killing fields; at any given point, two span the tangent space at that point, and the third one is a linear combination of the other two. Picking any two defines the remaining degenerate linear combinations define an orthogonal space
Cartan involution
The Cartan involution is defined as the mirroring or reversal of the direction of a geodesic. Its differential flips the direction of the tangents to a geodesic. It is a linear operator of norm one; it has two invariant subspaces, of eigenvalue +1 and −1. These two subspaces correspond to and respectively. This can be made more precise. Fixing a point consider a geodesic passing through , with The involution is defined as : This map is an involution, in that When restricted to geodesics along the Killing fields, it is also clearly an isometry. It is uniquely defined. Let be the group of isometries generated by the Killing fields. The function defined by : is a homomorphism of . Its infinitesimal is : The Cartan involution is a Lie algebra homomorphism, in that :