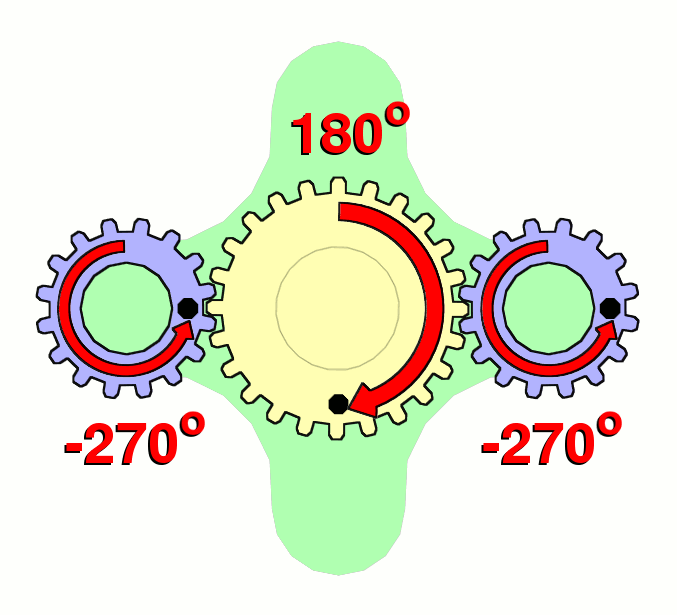
Epicyclic Carrier Locked on:
[Wikipedia]
[Google]
[Amazon]
 An epicyclic gear train (also known as a planetary gearset) consists of two
An epicyclic gear train (also known as a planetary gearset) consists of two
 Epicyclic gearing is also available which consists of a sun, a carrier, and two planets which mesh with each other. One planet meshes with the sun gear, while the second planet meshes with the ring gear. For this case, when the carrier is fixed, the ring gear rotates in the same direction as the sun gear, thus providing a reversal in direction compared to standard epicyclic gearing.
Epicyclic gearing is also available which consists of a sun, a carrier, and two planets which mesh with each other. One planet meshes with the sun gear, while the second planet meshes with the ring gear. For this case, when the carrier is fixed, the ring gear rotates in the same direction as the sun gear, thus providing a reversal in direction compared to standard epicyclic gearing.
 The overall gear ratio of a simple planetary gearset can be calculated using the following two equations, representing the sun-planet and planet-ring interactions respectively:
:
where
: are the angular velocities of the ''ring gear'', ''sun gear'', ''planetary gears'' , and ''carrier frame'' respectively, and
: are the number of teeth of the ''ring gear'', the ''sun gear'', and each ''planet gear'' respectively.
from which we can derive the following:
:
:
:
:
and
:
only if
In many epicyclic gearing systems, one of these three basic components is held stationary (hence set for whichever gear is stationary); one of the two remaining components is an ''input'', providing power to the system, while the last component is an ''output'', receiving power from the system. The ratio of input rotation to output rotation is dependent upon the number of teeth in each of the gears, and upon which component is held stationary.
Alternatively, in the special case where the number of teeth on each gear meets the relationship the equation can be re-written as the following:
:
where
: is the sun-to-planet gear ratio.
These relationships can be used to analyze any epicyclic system, including those, such as hybrid vehicle transmissions, where two of the components are used as ''inputs'' with the third providing ''output'' relative to the two inputs.
In one arrangement, the planetary carrier (green in the diagram above) is held stationary, and the sun gear (yellow) is used as input. In that case, the planetary gears simply rotate about their own axes (i.e., spin) at a rate determined by the number of teeth in each gear. If the sun gear has teeth, and each planet gear has teeth, then the ratio is equal to For instance, if the sun gear has 24 teeth, and each planet has 16 teeth, then the ratio is , or ; this means that one
The overall gear ratio of a simple planetary gearset can be calculated using the following two equations, representing the sun-planet and planet-ring interactions respectively:
:
where
: are the angular velocities of the ''ring gear'', ''sun gear'', ''planetary gears'' , and ''carrier frame'' respectively, and
: are the number of teeth of the ''ring gear'', the ''sun gear'', and each ''planet gear'' respectively.
from which we can derive the following:
:
:
:
:
and
:
only if
In many epicyclic gearing systems, one of these three basic components is held stationary (hence set for whichever gear is stationary); one of the two remaining components is an ''input'', providing power to the system, while the last component is an ''output'', receiving power from the system. The ratio of input rotation to output rotation is dependent upon the number of teeth in each of the gears, and upon which component is held stationary.
Alternatively, in the special case where the number of teeth on each gear meets the relationship the equation can be re-written as the following:
:
where
: is the sun-to-planet gear ratio.
These relationships can be used to analyze any epicyclic system, including those, such as hybrid vehicle transmissions, where two of the components are used as ''inputs'' with the third providing ''output'' relative to the two inputs.
In one arrangement, the planetary carrier (green in the diagram above) is held stationary, and the sun gear (yellow) is used as input. In that case, the planetary gears simply rotate about their own axes (i.e., spin) at a rate determined by the number of teeth in each gear. If the sun gear has teeth, and each planet gear has teeth, then the ratio is equal to For instance, if the sun gear has 24 teeth, and each planet has 16 teeth, then the ratio is , or ; this means that one
: : : : : :
where: — Torque of ring (annulus), — Torque of sun, — Torque of carrier. For all three, these are the torques applied to the mechanism (input torques). Output torques have the reverse sign of input torques. In the cases where gears are accelerating, or to account for friction, these equations must be modified.
 A spur gear differential is constructed from two identical coaxial epicyclic gear trains assembled with a single carrier such that their planet gears are engaged. This forms a planetary gear train with a fixed carrier train ratio ''R'' = −1.
In this case, the fundamental formula for the planetary gear train yields,
:
or
:
Thus, the angular velocity of the carrier of a spur gear differential is the average of the angular velocities of the sun and ring gears.
In discussing the spur gear differential, the use of the term ''ring gear'' is a convenient way to distinguish the sun gears of the two epicyclic gear trains. Ring gears are normally fixed in most applications as this arrangement will have a good reduction capacity. The second sun gear serves the same purpose as the ring gear of a simple planetary gear train but clearly does not have the internal gear mate that is typical of a ring gear.
A spur gear differential is constructed from two identical coaxial epicyclic gear trains assembled with a single carrier such that their planet gears are engaged. This forms a planetary gear train with a fixed carrier train ratio ''R'' = −1.
In this case, the fundamental formula for the planetary gear train yields,
:
or
:
Thus, the angular velocity of the carrier of a spur gear differential is the average of the angular velocities of the sun and ring gears.
In discussing the spur gear differential, the use of the term ''ring gear'' is a convenient way to distinguish the sun gears of the two epicyclic gear trains. Ring gears are normally fixed in most applications as this arrangement will have a good reduction capacity. The second sun gear serves the same purpose as the ring gear of a simple planetary gear train but clearly does not have the internal gear mate that is typical of a ring gear.
 Some epicyclic gear trains employ two planetary gears which mesh with each other. One of these planets meshes with the sun gear, the other planet meshes with the ring gear. This results in different ratios being generated by the planetary and also causes the sun gear to rotate in the same direction as the ring gear when the planet carrier is the stationary. The fundamental equation becomes:
:
where
which results in:
: when the carrier is locked,
: when the sun is locked,
: when the ring gear is locked.
Some epicyclic gear trains employ two planetary gears which mesh with each other. One of these planets meshes with the sun gear, the other planet meshes with the ring gear. This results in different ratios being generated by the planetary and also causes the sun gear to rotate in the same direction as the ring gear when the planet carrier is the stationary. The fundamental equation becomes:
:
where
which results in:
: when the carrier is locked,
: when the sun is locked,
: when the ring gear is locked.
 "Compound planetary gear" is a general concept and it refers to any planetary gears involving one or more of the following three types of structures: ''meshed-planet'' (there are at least two or more planets in mesh with each other in each planet train), ''stepped-planet'' (there exists a shaft connection between two planets in each planet train), and ''multi-stage structures'' (the system contains two or more planet sets).
Some designs use "stepped-planet" which have two differently-sized gears on either end of a common shaft. The small end engages the sun, while the large end engages the ring gear. This may be necessary to achieve smaller step changes in gear ratio when the overall package size is limited. Compound planets have "timing marks" (or "relative gear mesh phase" in technical term). The assembly conditions of compound planetary gears are more restrictive than simple planetary gears, and they must be assembled in the correct initial orientation relative to each other, or their teeth will not simultaneously engage the sun and ring gear at opposite ends of the planet, leading to very rough running and short life. In 2015, a traction based variant of the "stepped-planet" design was developed at the Delft University of Technology, which relies on compression of the stepped planet elements to achieve torque transmission. The use of traction elements eliminates the need to "timing marks" as well as the restrictive assembly conditions as typically found. Compound planetary gears can easily achieve larger transmission ratio with equal or smaller volume. For example, compound planets with teeth in a 2:1 ratio with a 50T ring gear would give the same effect as a 100T ring gear, but with half the actual diameter.
More planet and sun gear units can be placed in series in the same housing (where the output shaft of the first stage becomes the input shaft of the next stage) providing a larger (or smaller) gear ratio. This is the way most
"Compound planetary gear" is a general concept and it refers to any planetary gears involving one or more of the following three types of structures: ''meshed-planet'' (there are at least two or more planets in mesh with each other in each planet train), ''stepped-planet'' (there exists a shaft connection between two planets in each planet train), and ''multi-stage structures'' (the system contains two or more planet sets).
Some designs use "stepped-planet" which have two differently-sized gears on either end of a common shaft. The small end engages the sun, while the large end engages the ring gear. This may be necessary to achieve smaller step changes in gear ratio when the overall package size is limited. Compound planets have "timing marks" (or "relative gear mesh phase" in technical term). The assembly conditions of compound planetary gears are more restrictive than simple planetary gears, and they must be assembled in the correct initial orientation relative to each other, or their teeth will not simultaneously engage the sun and ring gear at opposite ends of the planet, leading to very rough running and short life. In 2015, a traction based variant of the "stepped-planet" design was developed at the Delft University of Technology, which relies on compression of the stepped planet elements to achieve torque transmission. The use of traction elements eliminates the need to "timing marks" as well as the restrictive assembly conditions as typically found. Compound planetary gears can easily achieve larger transmission ratio with equal or smaller volume. For example, compound planets with teeth in a 2:1 ratio with a 50T ring gear would give the same effect as a 100T ring gear, but with half the actual diameter.
More planet and sun gear units can be placed in series in the same housing (where the output shaft of the first stage becomes the input shaft of the next stage) providing a larger (or smaller) gear ratio. This is the way most
 Planetary gear trains provide high power density in comparison to standard parallel axis gear trains. They provide a reduction in volume, multiple kinematic combinations, purely torsional reactions, and coaxial shafting. Disadvantages include high bearing loads, constant lubrication requirements, inaccessibility, and design complexity.Smith, J. D., 1983, ''Gears and Their Vibration: A Basic Approach to Understanding Gear Noise''. Marcel Dekker, New York and MacMillan, London
The efficiency loss in a planetary gear train is typically about 3% per stage. This type of efficiency ensures that a high proportion (about 97%) of the energy being input is transmitted through the gearbox, rather than being wasted on mechanical losses inside the gearbox.
The load in a planetary gear train is shared among multiple planets; therefore, torque capability is greatly increased. The more planets in the system, the greater the load ability and the higher the torque density.
The planetary gear train also provides stability due to an even distribution of mass and increased rotational stiffness. Torque applied radially onto the gears of a planetary gear train is transferred radially by the gear, without lateral pressure on the gear teeth.
In a typical application, the drive power connects to the sun gear. The sun gear then drives the planetary gears assembled with the external gear ring to operate. The whole set of planetary gear system revolves on its own axis and along the external gear ring where the output shaft connected to the planetary carrier achieves the goal of speed reduction. A higher reduction ratio can be achieved by doubling the multiple staged gears and planetary gears which can operate within the same ring gear.
The method of motion of a planetary gear structure is different from traditional parallel gears. Traditional gears rely on a small number of contact points between two gears to transfer the driving force. In this case, all the loading is concentrated on a few contacting surfaces, making the gears wear quickly and sometimes crack. But the planetary speed reducer has multiple gear contacting surfaces with a larger area that can distribute the loading evenly around the central axis. Multiple gear surfaces share the load, including any instantaneous impact loading, evenly, which make them more resistant to damage from higher torque. The housing and bearing parts are also less likely to be damaged from high loading as only the planet carrier bearings experience significant lateral force from the transmission of torque, radial forces oppose each other and are balanced, and axial forces only arise when using helical gears.
Planetary gear trains provide high power density in comparison to standard parallel axis gear trains. They provide a reduction in volume, multiple kinematic combinations, purely torsional reactions, and coaxial shafting. Disadvantages include high bearing loads, constant lubrication requirements, inaccessibility, and design complexity.Smith, J. D., 1983, ''Gears and Their Vibration: A Basic Approach to Understanding Gear Noise''. Marcel Dekker, New York and MacMillan, London
The efficiency loss in a planetary gear train is typically about 3% per stage. This type of efficiency ensures that a high proportion (about 97%) of the energy being input is transmitted through the gearbox, rather than being wasted on mechanical losses inside the gearbox.
The load in a planetary gear train is shared among multiple planets; therefore, torque capability is greatly increased. The more planets in the system, the greater the load ability and the higher the torque density.
The planetary gear train also provides stability due to an even distribution of mass and increased rotational stiffness. Torque applied radially onto the gears of a planetary gear train is transferred radially by the gear, without lateral pressure on the gear teeth.
In a typical application, the drive power connects to the sun gear. The sun gear then drives the planetary gears assembled with the external gear ring to operate. The whole set of planetary gear system revolves on its own axis and along the external gear ring where the output shaft connected to the planetary carrier achieves the goal of speed reduction. A higher reduction ratio can be achieved by doubling the multiple staged gears and planetary gears which can operate within the same ring gear.
The method of motion of a planetary gear structure is different from traditional parallel gears. Traditional gears rely on a small number of contact points between two gears to transfer the driving force. In this case, all the loading is concentrated on a few contacting surfaces, making the gears wear quickly and sometimes crack. But the planetary speed reducer has multiple gear contacting surfaces with a larger area that can distribute the loading evenly around the central axis. Multiple gear surfaces share the load, including any instantaneous impact loading, evenly, which make them more resistant to damage from higher torque. The housing and bearing parts are also less likely to be damaged from high loading as only the planet carrier bearings experience significant lateral force from the transmission of torque, radial forces oppose each other and are balanced, and axial forces only arise when using helical gears.
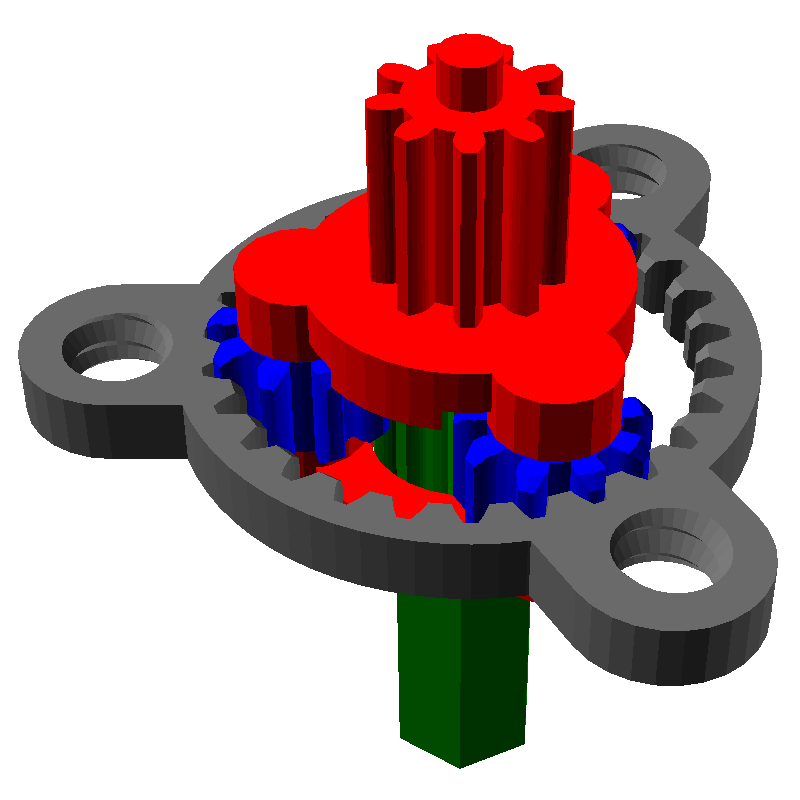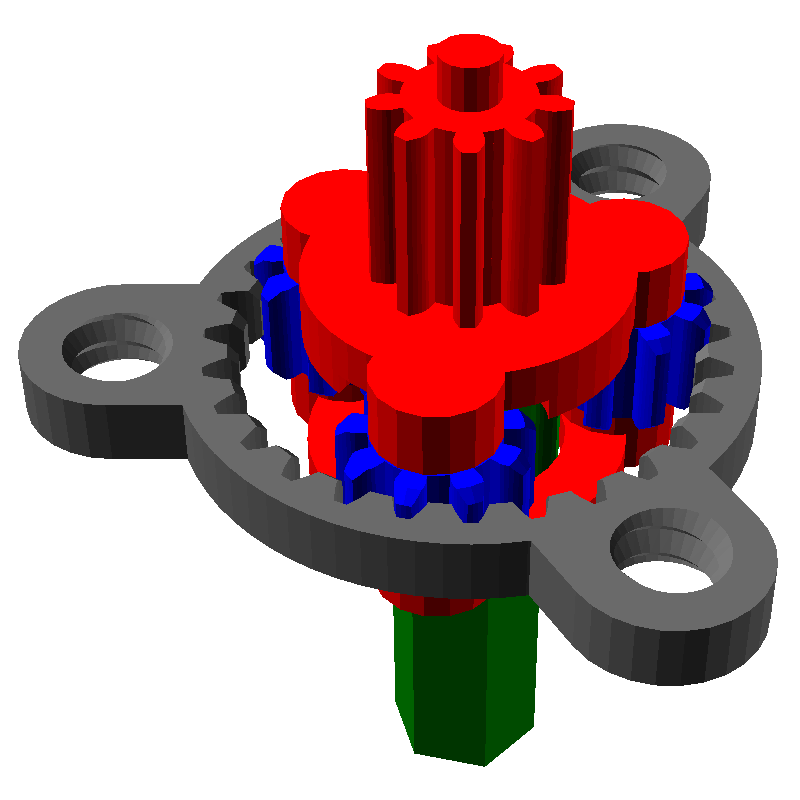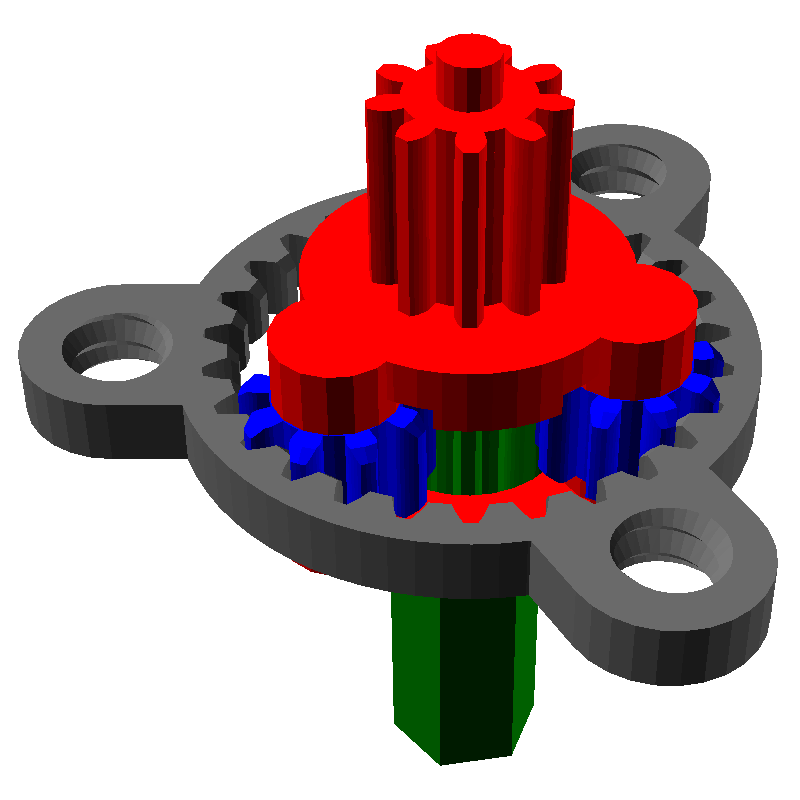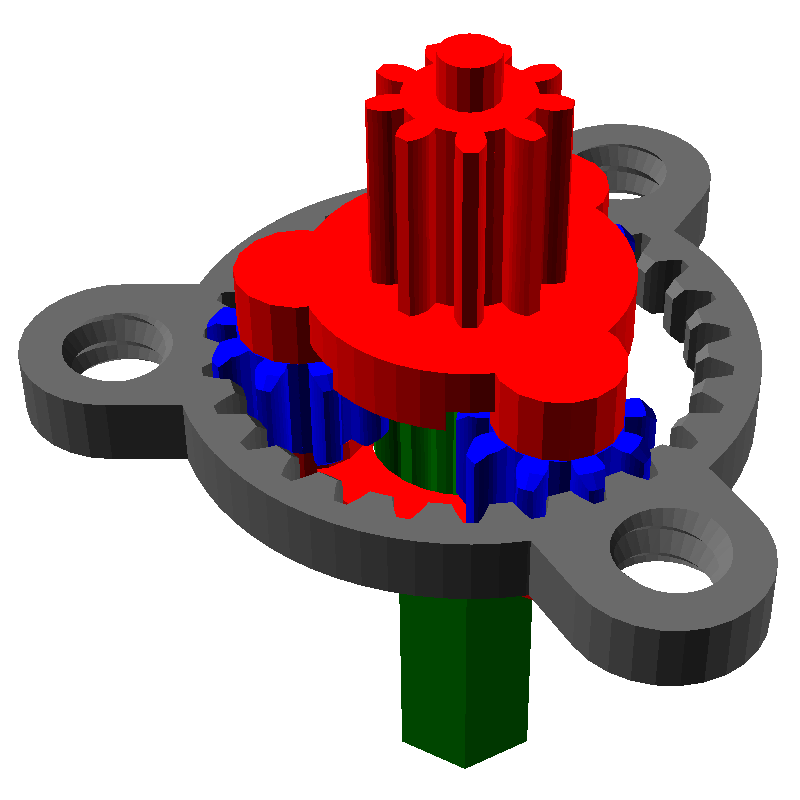 Planetary gears have become popular in
Planetary gears have become popular in
Kinematic Models for Design Digital Library (KMODDL)
movies and photos of hundreds of working mechanical-systems models at Cornell.
"Epicyclic gearing animation in SVG"Prius GearboxPlanetary GearboxShort Cuts for Analyzing Planetary Gearing
{{DEFAULTSORT:Epicyclic Gearing Gears
 An epicyclic gear train (also known as a planetary gearset) consists of two
An epicyclic gear train (also known as a planetary gearset) consists of two gear
A gear is a rotating circular machine part having cut teeth or, in the case of a cogwheel or gearwheel, inserted teeth (called ''cogs''), which mesh with another (compatible) toothed part to transmit (convert) torque and speed. The basic pr ...
s mounted so that the center of one gear revolves around the center of the other. A carrier connects the centers of the two gears and rotates the planet and sun gears mesh so that their pitch circle
This page lists the standard US nomenclature used in the description of mechanical gear construction and function, together with definitions of the terms. The terminology was established by the American Gear Manufacturers Association (AGMA), unde ...
s roll without slip. A point on the pitch circle of the planet gear traces an epicycloid
In geometry, an epicycloid is a plane curve produced by tracing the path of a chosen point on the circumference of a circle—called an ''epicycle''—which rolls without slipping around a fixed circle. It is a particular kind of roulette.
Equati ...
curve. In this simplified case, the sun gear is fixed and the planetary gear(s) roll around the sun gear.
An epicyclic gear train can be assembled so the planet gear rolls on the inside of the pitch circle of a fixed, outer gear ring, or ring gear, sometimes called an ''annular gear''. In this case, the curve traced by a point on the pitch circle of the planet is a hypocycloid
In geometry, a hypocycloid is a special plane curve generated by the trace of a fixed point on a small circle that rolls within a larger circle. As the radius of the larger circle is increased, the hypocycloid becomes more like the cycloid crea ...
.
The combination of epicycle gear trains with a planet engaging both a sun gear and a ring gear is called a ''planetary gear train''.J. J. Uicker, G. R. Pennock and J. E. Shigley, 2003, ''Theory of Machines and Mechanisms,'' Oxford University Press, New York.B. Paul, 1979, ''Kinematics and Dynamics of Planar Machinery'', Prentice Hall. In this case, the ring gear is usually fixed and the sun gear is driven.
Overview
''Epicyclic gearing'' or ''planetary gearing'' is agear
A gear is a rotating circular machine part having cut teeth or, in the case of a cogwheel or gearwheel, inserted teeth (called ''cogs''), which mesh with another (compatible) toothed part to transmit (convert) torque and speed. The basic pr ...
system consisting of one or more outer, or ''planet'', gears or pinion
A pinion is a round gear—usually the smaller of two meshed gears—used in several applications, including drivetrain and rack and pinion systems.
Applications
Drivetrain
Drivetrains usually feature a gear known as the pinion, which may ...
s, revolving about a central ''sun gear'' or ''sun wheel''. Typically, the planet gears are mounted on a movable arm or ''carrier'', which itself may rotate relative to the sun gear. Epicyclic gearing systems also incorporate the use of an outer ''ring'' gear or ''annulus'', which meshes with the planet gears. Planetary gears (or epicyclic gears) are typically classified as simple or compound planetary gears. Simple planetary gears have one sun, one ring, one carrier, and one planet set. Compound planetary gears involve one or more of the following three types of structures: meshed-planet (there are at least two more planets in mesh with each other in each planet train), stepped-planet (there exists a shaft connection between two planets in each planet
train), and multi-stage structures (the system contains two or more planet sets). Compared to simple planetary gears, compound planetary gears have the advantages of larger reduction ratio, higher torque-to-weight ratio, and more flexible configurations.
The axes of all gears are usually parallel, but for special cases like pencil sharpeners and differentials, they can be placed at an angle, introducing elements of bevel gear
Bevel gears are gears where the axes of the two shafts intersect and the tooth-bearing faces of the gears themselves are conically shaped. Bevel gears are most often mounted on shafts that are 90 degrees apart, but can be designed to work at oth ...
(see below). Further, the sun, planet carrier and ring axes are usually coaxial
In geometry, coaxial means that several three-dimensional linear or planar forms share a common axis. The two-dimensional analog is ''concentric''.
Common examples:
A coaxial cable is a three-dimensional linear structure. It has a wire conduc ...
.
 Epicyclic gearing is also available which consists of a sun, a carrier, and two planets which mesh with each other. One planet meshes with the sun gear, while the second planet meshes with the ring gear. For this case, when the carrier is fixed, the ring gear rotates in the same direction as the sun gear, thus providing a reversal in direction compared to standard epicyclic gearing.
Epicyclic gearing is also available which consists of a sun, a carrier, and two planets which mesh with each other. One planet meshes with the sun gear, while the second planet meshes with the ring gear. For this case, when the carrier is fixed, the ring gear rotates in the same direction as the sun gear, thus providing a reversal in direction compared to standard epicyclic gearing.
History
Around 500 BCE, the Greeks invented the idea of epicycles, of circles travelling on the circular orbits. With this theoryClaudius Ptolemy
Claudius Ptolemy (; grc-gre, Πτολεμαῖος, ; la, Claudius Ptolemaeus; AD) was a mathematician, astronomer, astrologer, geographer, and music theorist, who wrote about a dozen scientific treatises, three of which were of importance ...
in the ''Almagest
The ''Almagest'' is a 2nd-century Greek-language mathematical and astronomical treatise on the apparent motions of the stars and planetary paths, written by Claudius Ptolemy ( ). One of the most influential scientific texts in history, it canoni ...
'' in 148 CE was able to approximate planetary paths observed crossing the sky. The Antikythera Mechanism
The Antikythera mechanism ( ) is an Ancient Greece, Ancient Greek hand-powered orrery, described as the oldest example of an analogue computer used to predict astronomy, astronomical positions and eclipses decades in advance. It could also be ...
, circa 80 BCE, had gearing which was able to closely match the moon's elliptical path through the heavens, and even to correct for the nine-year precession
Precession is a change in the orientation of the rotational axis of a rotating body. In an appropriate reference frame it can be defined as a change in the first Euler angle, whereas the third Euler angle defines the rotation itself. In othe ...
of that path. (The Greeks interpreted the motion they saw, not as elliptical, but rather as epicyclic motion.)
In the 2nd century AD treatise ''The Mathematical Syntaxis'' (a.k.a. ''Almagest
The ''Almagest'' is a 2nd-century Greek-language mathematical and astronomical treatise on the apparent motions of the stars and planetary paths, written by Claudius Ptolemy ( ). One of the most influential scientific texts in history, it canoni ...
''), Claudius Ptolemy
Claudius Ptolemy (; grc-gre, Πτολεμαῖος, ; la, Claudius Ptolemaeus; AD) was a mathematician, astronomer, astrologer, geographer, and music theorist, who wrote about a dozen scientific treatises, three of which were of importance ...
used rotating deferent and epicycle
In the Hipparchian, Ptolemaic, and Copernican systems of astronomy, the epicycle (, meaning "circle moving on another circle") was a geometric model used to explain the variations in speed and direction of the apparent motion of the Moon, Sun ...
s that form epicyclic gear trains to predict the motions of the planets. Accurate predictions of the movement of the Sun, Moon, and the five planets, Mercury, Venus, Mars, Jupiter, and Saturn, across the sky assumed that each followed a trajectory traced by a point on the planet gear of an epicyclic gear train. This curve is called an epitrochoid
In geometry, an epitrochoid ( or ) is a roulette traced by a point attached to a circle of radius rolling around the outside of a fixed circle of radius , where the point is at a distance from the center of the exterior circle.
The parametric ...
.
Epicyclic gearing was used in the Antikythera Mechanism
The Antikythera mechanism ( ) is an Ancient Greece, Ancient Greek hand-powered orrery, described as the oldest example of an analogue computer used to predict astronomy, astronomical positions and eclipses decades in advance. It could also be ...
, circa 80 BCE, to adjust the displayed position of the moon for the ellipticity of its orbit, and even for its orbital apsidal precession
In celestial mechanics, apsidal precession (or apsidal advance) is the precession (gradual rotation) of the line connecting the apsides (line of apsides) of an astronomical body's orbit. The apsides are the orbital points closest (periapsi ...
. Two facing gears were rotated around slightly different centers; one drove the other, not with meshed teeth but with a pin inserted into a slot on the second. As the slot drove the second gear, the radius of driving would change, thus invoking a speeding up and slowing down of the driven gear in each revolution.
Richard of Wallingford
Richard of Wallingford (1292–1336) was an English mathematician, astronomer, horologist, and cleric who made major contributions to astronomy and horology while serving as abbot of St Albans Abbey in Hertfordshire.
Biography
Richard was bor ...
, an English abbot of St. Albans monastery, later described epicyclic gearing for an astronomical clock
An astronomical clock, horologium, or orloj is a clock with special mechanisms and dials to display astronomical information, such as the relative positions of the Sun, Moon, zodiacal constellations, and sometimes major planets.
Definition
...
in the 14th century. In 1588, Italian military engineer Agostino Ramelli
Agostino Ramelli (1531–ca. 1610) was an Italian engineer best known for writing and illustrating the book of engineering designs ''Le diverse et artificiose machine del Capitano Agostino Ramelli'', which contains, among others, his design ...
invented the bookwheel
The bookwheel (also written book wheel and sometimes called a reading wheel) is a type of rotating bookcase that allows one person to read multiple books in one location with ease. The books are rotated vertically similar to the motion of a water ...
, a vertically revolving bookstand containing epicyclic gearing with two levels of planetary gears to maintain proper orientation of the books.
French mathematician and engineer Desargues designed and constructed the first mill with epicycloidal teeth .
Requirements for Non-Interference
In order that the planet gear teeth mesh properly with both the sun and ring gears, assuming equally spaced planet gears, the following equation must be satisfied: where are the number of teeth of the ''ring gear'' and the ''sun gear'', respectively and is the number of planet gears in the assembly and is a whole number If one is to create an asymmetric carrier frame with non-equiangular planet gears, say to create some kind of mechanical vibration in the system, one must make the teething such that the above equation complies with the "imaginary gears". For example, in the case where a carrier frame is intended to contain planet gears spaced 0°, 50°, 120°, and 230°, one is to calculate as if there are actually 36 planetary gears (10° equiangular), rather than the four real ones.Gear speed ratios of conventional epicyclic gearing
Thegear ratio
A gear train is a mechanical system formed by mounting gears on a frame so the teeth of the gears engage.
Gear teeth are designed to ensure the pitch circles of engaging gears roll on each other without slipping, providing a smooth transmission ...
of an epicyclic gearing system is somewhat non-intuitive, particularly because there are several ways in which an input rotation can be converted into an output rotation. The four basic components of the epicyclic gear are:
* Sun gear: The central gear
* Carrier frame: Holds one or more ''planetary'' gear(s) symmetrically and separated, all meshed with the sun gear
* Planet gear(s): Usually two to four peripheral gears, all of the same size, that mesh between the ''sun gear'' and the ''ring gear''
* Ring gear or Annulus gear: An outer ring with inward-facing teeth that mesh with the planetary gear(s)
 The overall gear ratio of a simple planetary gearset can be calculated using the following two equations, representing the sun-planet and planet-ring interactions respectively:
:
where
: are the angular velocities of the ''ring gear'', ''sun gear'', ''planetary gears'' , and ''carrier frame'' respectively, and
: are the number of teeth of the ''ring gear'', the ''sun gear'', and each ''planet gear'' respectively.
from which we can derive the following:
:
:
:
:
and
:
only if
In many epicyclic gearing systems, one of these three basic components is held stationary (hence set for whichever gear is stationary); one of the two remaining components is an ''input'', providing power to the system, while the last component is an ''output'', receiving power from the system. The ratio of input rotation to output rotation is dependent upon the number of teeth in each of the gears, and upon which component is held stationary.
Alternatively, in the special case where the number of teeth on each gear meets the relationship the equation can be re-written as the following:
:
where
: is the sun-to-planet gear ratio.
These relationships can be used to analyze any epicyclic system, including those, such as hybrid vehicle transmissions, where two of the components are used as ''inputs'' with the third providing ''output'' relative to the two inputs.
In one arrangement, the planetary carrier (green in the diagram above) is held stationary, and the sun gear (yellow) is used as input. In that case, the planetary gears simply rotate about their own axes (i.e., spin) at a rate determined by the number of teeth in each gear. If the sun gear has teeth, and each planet gear has teeth, then the ratio is equal to For instance, if the sun gear has 24 teeth, and each planet has 16 teeth, then the ratio is , or ; this means that one
The overall gear ratio of a simple planetary gearset can be calculated using the following two equations, representing the sun-planet and planet-ring interactions respectively:
:
where
: are the angular velocities of the ''ring gear'', ''sun gear'', ''planetary gears'' , and ''carrier frame'' respectively, and
: are the number of teeth of the ''ring gear'', the ''sun gear'', and each ''planet gear'' respectively.
from which we can derive the following:
:
:
:
:
and
:
only if
In many epicyclic gearing systems, one of these three basic components is held stationary (hence set for whichever gear is stationary); one of the two remaining components is an ''input'', providing power to the system, while the last component is an ''output'', receiving power from the system. The ratio of input rotation to output rotation is dependent upon the number of teeth in each of the gears, and upon which component is held stationary.
Alternatively, in the special case where the number of teeth on each gear meets the relationship the equation can be re-written as the following:
:
where
: is the sun-to-planet gear ratio.
These relationships can be used to analyze any epicyclic system, including those, such as hybrid vehicle transmissions, where two of the components are used as ''inputs'' with the third providing ''output'' relative to the two inputs.
In one arrangement, the planetary carrier (green in the diagram above) is held stationary, and the sun gear (yellow) is used as input. In that case, the planetary gears simply rotate about their own axes (i.e., spin) at a rate determined by the number of teeth in each gear. If the sun gear has teeth, and each planet gear has teeth, then the ratio is equal to For instance, if the sun gear has 24 teeth, and each planet has 16 teeth, then the ratio is , or ; this means that one clockwise
Two-dimensional rotation can occur in two possible directions. Clockwise motion (abbreviated CW) proceeds in the same direction as a clock's hands: from the top to the right, then down and then to the left, and back up to the top. The opposite ...
turn of the sun gear produces 1.5 ''counterclockwise'' turns of each of the planet gear(s) about its axis.
Rotation of the planet gears can in turn drive the ring gear (not depicted in diagram), at a speed corresponding to the gear ratios: If the ring gear has teeth, then the ring will rotate by turns for each turn of the planetary gears. For instance, if the ring gear has 64 teeth, and the planets 16 teeth, one clockwise turn of a planet gear results in , or clockwise turns of the ring gear. Extending this case from the one above:
* One turn of the sun gear results in turns of the planets
* One turn of a planet gear results in turns of the ring gear
So, with the planetary carrier locked, one turn of the sun gear results in turns of the ring gear.
The ring gear may also be held fixed, with input provided to the planetary gear carrier; output rotation is then produced from the sun gear. This configuration will produce an increase in gear ratio, equal to
If the ring gear is held stationary and the sun gear is used as the input, the planet carrier will be the output. The gear ratio in this case will be which may also be written as This is the lowest gear ratio attainable with an epicyclic gear train. This type of gearing is sometimes used in tractors
A tractor is an engineering vehicle specifically designed to deliver a high tractive effort (or torque) at slow speeds, for the purposes of hauling a trailer or machinery such as that used in agriculture, mining or construction. Most common ...
and construction equipment to provide high torque to the drive wheels.
In bicycle hub gear
A hub gear, internal-gear hub, internally geared hub or just gear hub is a gear ratio changing system commonly used on bicycles that is implemented with planetary or epicyclic gears. The gears and lubricants are sealed within the shell of the h ...
s, the sun is usually stationary, being keyed to the axle or even machined directly onto it. The planetary gear carrier is used as input. In this case the gear ratio is simply given by The number of teeth in the planet gear is irrelevant.
Accelerations of standard epicyclic gearing
From the above formulae, we can also derive the accelerations of the sun, ring and carrier, which are: : : :Torque ratios of standard epicyclic gearing
In epicyclic gears, two speeds must be known, in order to determine the third speed. However, in a steady state condition, only one torque must be known, in order to determine the other two torques. The equations which determine torque are:: : : : : :
where: — Torque of ring (annulus), — Torque of sun, — Torque of carrier. For all three, these are the torques applied to the mechanism (input torques). Output torques have the reverse sign of input torques. In the cases where gears are accelerating, or to account for friction, these equations must be modified.
Fixed carrier train ratio
A convenient approach to determine the various speed ratios available in a planetary gear train begins by considering the speed ratio of the gear train when the carrier is held fixed. This is known as the fixed carrier train ratio. In the case of a simple planetary gear train formed by a carrier supporting a planet gear engaged with a sun and ring gear, the fixed carrier train ratio is computed as the speed ratio of thegear train
A gear train is a mechanical system formed by mounting gears on a frame so the teeth of the gears engage.
Gear teeth are designed to ensure the pitch circles of engaging gears roll on each other without slipping, providing a smooth transmission ...
formed by the sun, planet and ring gears on the fixed carrier. This is given by
:
In this calculation the planet gear is an idler gear.
The fundamental formula of the planetary gear train with a rotating carrier is obtained by recognizing that this formula remains true if the angular velocities of the sun, planet and ring gears are computed relative to the carrier angular velocity. This becomes,
:
This formula provides a simple way to determine the speed ratios for the simple planetary gear train under different conditions:
1. The carrier is held fixed, ωc=0,
::
2. The ring gear is held fixed, ωr=0,
::
3. The sun gear is held fixed, ωs=0,
::
Each of the speed ratios available to a simple planetary gear train can be obtained by using band brakes to hold and release the carrier, sun or ring gears as needed. This provides the basic structure for an automatic transmission
An automatic transmission (sometimes abbreviated to auto or AT) is a multi-speed transmission used in internal combustion engine-based motor vehicles that does not require any input from the driver to change forward gears under normal driving c ...
.
Spur gear differential
 A spur gear differential is constructed from two identical coaxial epicyclic gear trains assembled with a single carrier such that their planet gears are engaged. This forms a planetary gear train with a fixed carrier train ratio ''R'' = −1.
In this case, the fundamental formula for the planetary gear train yields,
:
or
:
Thus, the angular velocity of the carrier of a spur gear differential is the average of the angular velocities of the sun and ring gears.
In discussing the spur gear differential, the use of the term ''ring gear'' is a convenient way to distinguish the sun gears of the two epicyclic gear trains. Ring gears are normally fixed in most applications as this arrangement will have a good reduction capacity. The second sun gear serves the same purpose as the ring gear of a simple planetary gear train but clearly does not have the internal gear mate that is typical of a ring gear.
A spur gear differential is constructed from two identical coaxial epicyclic gear trains assembled with a single carrier such that their planet gears are engaged. This forms a planetary gear train with a fixed carrier train ratio ''R'' = −1.
In this case, the fundamental formula for the planetary gear train yields,
:
or
:
Thus, the angular velocity of the carrier of a spur gear differential is the average of the angular velocities of the sun and ring gears.
In discussing the spur gear differential, the use of the term ''ring gear'' is a convenient way to distinguish the sun gears of the two epicyclic gear trains. Ring gears are normally fixed in most applications as this arrangement will have a good reduction capacity. The second sun gear serves the same purpose as the ring gear of a simple planetary gear train but clearly does not have the internal gear mate that is typical of a ring gear.
Gear ratio of reversed epicyclic gearing
Compound planetary gears
 "Compound planetary gear" is a general concept and it refers to any planetary gears involving one or more of the following three types of structures: ''meshed-planet'' (there are at least two or more planets in mesh with each other in each planet train), ''stepped-planet'' (there exists a shaft connection between two planets in each planet train), and ''multi-stage structures'' (the system contains two or more planet sets).
Some designs use "stepped-planet" which have two differently-sized gears on either end of a common shaft. The small end engages the sun, while the large end engages the ring gear. This may be necessary to achieve smaller step changes in gear ratio when the overall package size is limited. Compound planets have "timing marks" (or "relative gear mesh phase" in technical term). The assembly conditions of compound planetary gears are more restrictive than simple planetary gears, and they must be assembled in the correct initial orientation relative to each other, or their teeth will not simultaneously engage the sun and ring gear at opposite ends of the planet, leading to very rough running and short life. In 2015, a traction based variant of the "stepped-planet" design was developed at the Delft University of Technology, which relies on compression of the stepped planet elements to achieve torque transmission. The use of traction elements eliminates the need to "timing marks" as well as the restrictive assembly conditions as typically found. Compound planetary gears can easily achieve larger transmission ratio with equal or smaller volume. For example, compound planets with teeth in a 2:1 ratio with a 50T ring gear would give the same effect as a 100T ring gear, but with half the actual diameter.
More planet and sun gear units can be placed in series in the same housing (where the output shaft of the first stage becomes the input shaft of the next stage) providing a larger (or smaller) gear ratio. This is the way most
"Compound planetary gear" is a general concept and it refers to any planetary gears involving one or more of the following three types of structures: ''meshed-planet'' (there are at least two or more planets in mesh with each other in each planet train), ''stepped-planet'' (there exists a shaft connection between two planets in each planet train), and ''multi-stage structures'' (the system contains two or more planet sets).
Some designs use "stepped-planet" which have two differently-sized gears on either end of a common shaft. The small end engages the sun, while the large end engages the ring gear. This may be necessary to achieve smaller step changes in gear ratio when the overall package size is limited. Compound planets have "timing marks" (or "relative gear mesh phase" in technical term). The assembly conditions of compound planetary gears are more restrictive than simple planetary gears, and they must be assembled in the correct initial orientation relative to each other, or their teeth will not simultaneously engage the sun and ring gear at opposite ends of the planet, leading to very rough running and short life. In 2015, a traction based variant of the "stepped-planet" design was developed at the Delft University of Technology, which relies on compression of the stepped planet elements to achieve torque transmission. The use of traction elements eliminates the need to "timing marks" as well as the restrictive assembly conditions as typically found. Compound planetary gears can easily achieve larger transmission ratio with equal or smaller volume. For example, compound planets with teeth in a 2:1 ratio with a 50T ring gear would give the same effect as a 100T ring gear, but with half the actual diameter.
More planet and sun gear units can be placed in series in the same housing (where the output shaft of the first stage becomes the input shaft of the next stage) providing a larger (or smaller) gear ratio. This is the way most automatic transmission
An automatic transmission (sometimes abbreviated to auto or AT) is a multi-speed transmission used in internal combustion engine-based motor vehicles that does not require any input from the driver to change forward gears under normal driving c ...
s work. In some cases multiple stages may even share the same ring gear which can be extended down the length of the transmission, or even be a structural part of the casing of smaller gearboxes.
During World War II
World War II or the Second World War, often abbreviated as WWII or WW2, was a world war that lasted from 1939 to 1945. It involved the vast majority of the world's countries—including all of the great powers—forming two opposin ...
, a special variation of epicyclic gearing was developed for portable radar
Radar is a detection system that uses radio waves to determine the distance (''ranging''), angle, and radial velocity of objects relative to the site. It can be used to detect aircraft, ships, spacecraft, guided missiles, motor vehicles, w ...
gear, where a very high reduction ratio in a small package was needed. This had two outer ring gears, each half the thickness of the other gears. One of these two ring gears was held fixed and had one tooth fewer than did the other. Therefore, several turns of the "sun" gear made the "planet" gears complete a single revolution, which in turn made the rotating ring gear rotate by a single tooth like a cycloidal drive
A cycloidal drive or cycloidal speed reducer is a mechanism for reducing the speed of an input shaft by a certain ratio. Cycloidal speed reducers are capable of relatively high ratios in compact sizes with very low backlash.
The input shaft dr ...
.
Power splitting
More than one member of a system can serve as output. As an example, the input is connected to the ring gear, the sun gear is connected to the output and the planet carrier is connected to the output through atorque converter
A torque converter is a type of fluid coupling that transfers rotating power from a prime mover, like an internal combustion engine, to a rotating driven load. In a vehicle with an automatic transmission, the torque converter connects the power ...
. Idler gears are used between sun gear and the planets to cause the sun gear to rotate in the same direction as the ring gear when the planet carrier is stationary. At low input speed, because of the load on the output, the sun will be stationary and the planet carrier will rotate in the direction of the ring gear. Given a high enough load, the turbine of the torque converter will remain stationary, the energy will be dissipated and the torque converter pump will slip. If the input speed is increased to overcome the load the converter turbine will turn the output shaft. Because the torque converter itself is a load on the planet carrier, a force will be exerted on the sun gear. Both the planet carrier and the sun gear extract energy from the system and apply it to the output shaft.
Advantages
 Planetary gear trains provide high power density in comparison to standard parallel axis gear trains. They provide a reduction in volume, multiple kinematic combinations, purely torsional reactions, and coaxial shafting. Disadvantages include high bearing loads, constant lubrication requirements, inaccessibility, and design complexity.Smith, J. D., 1983, ''Gears and Their Vibration: A Basic Approach to Understanding Gear Noise''. Marcel Dekker, New York and MacMillan, London
The efficiency loss in a planetary gear train is typically about 3% per stage. This type of efficiency ensures that a high proportion (about 97%) of the energy being input is transmitted through the gearbox, rather than being wasted on mechanical losses inside the gearbox.
The load in a planetary gear train is shared among multiple planets; therefore, torque capability is greatly increased. The more planets in the system, the greater the load ability and the higher the torque density.
The planetary gear train also provides stability due to an even distribution of mass and increased rotational stiffness. Torque applied radially onto the gears of a planetary gear train is transferred radially by the gear, without lateral pressure on the gear teeth.
In a typical application, the drive power connects to the sun gear. The sun gear then drives the planetary gears assembled with the external gear ring to operate. The whole set of planetary gear system revolves on its own axis and along the external gear ring where the output shaft connected to the planetary carrier achieves the goal of speed reduction. A higher reduction ratio can be achieved by doubling the multiple staged gears and planetary gears which can operate within the same ring gear.
The method of motion of a planetary gear structure is different from traditional parallel gears. Traditional gears rely on a small number of contact points between two gears to transfer the driving force. In this case, all the loading is concentrated on a few contacting surfaces, making the gears wear quickly and sometimes crack. But the planetary speed reducer has multiple gear contacting surfaces with a larger area that can distribute the loading evenly around the central axis. Multiple gear surfaces share the load, including any instantaneous impact loading, evenly, which make them more resistant to damage from higher torque. The housing and bearing parts are also less likely to be damaged from high loading as only the planet carrier bearings experience significant lateral force from the transmission of torque, radial forces oppose each other and are balanced, and axial forces only arise when using helical gears.
Planetary gear trains provide high power density in comparison to standard parallel axis gear trains. They provide a reduction in volume, multiple kinematic combinations, purely torsional reactions, and coaxial shafting. Disadvantages include high bearing loads, constant lubrication requirements, inaccessibility, and design complexity.Smith, J. D., 1983, ''Gears and Their Vibration: A Basic Approach to Understanding Gear Noise''. Marcel Dekker, New York and MacMillan, London
The efficiency loss in a planetary gear train is typically about 3% per stage. This type of efficiency ensures that a high proportion (about 97%) of the energy being input is transmitted through the gearbox, rather than being wasted on mechanical losses inside the gearbox.
The load in a planetary gear train is shared among multiple planets; therefore, torque capability is greatly increased. The more planets in the system, the greater the load ability and the higher the torque density.
The planetary gear train also provides stability due to an even distribution of mass and increased rotational stiffness. Torque applied radially onto the gears of a planetary gear train is transferred radially by the gear, without lateral pressure on the gear teeth.
In a typical application, the drive power connects to the sun gear. The sun gear then drives the planetary gears assembled with the external gear ring to operate. The whole set of planetary gear system revolves on its own axis and along the external gear ring where the output shaft connected to the planetary carrier achieves the goal of speed reduction. A higher reduction ratio can be achieved by doubling the multiple staged gears and planetary gears which can operate within the same ring gear.
The method of motion of a planetary gear structure is different from traditional parallel gears. Traditional gears rely on a small number of contact points between two gears to transfer the driving force. In this case, all the loading is concentrated on a few contacting surfaces, making the gears wear quickly and sometimes crack. But the planetary speed reducer has multiple gear contacting surfaces with a larger area that can distribute the loading evenly around the central axis. Multiple gear surfaces share the load, including any instantaneous impact loading, evenly, which make them more resistant to damage from higher torque. The housing and bearing parts are also less likely to be damaged from high loading as only the planet carrier bearings experience significant lateral force from the transmission of torque, radial forces oppose each other and are balanced, and axial forces only arise when using helical gears.
3D printing
 Planetary gears have become popular in
Planetary gears have become popular in 3D printing
3D printing or additive manufacturing is the Manufacturing, construction of a three-dimensional object from a computer-aided design, CAD model or a digital 3D modeling, 3D model. It can be done in a variety of processes in which material is ...
for a few different reasons. Planetary gear boxes can provide a large gear ratio in a small, light-weight package. Some people install such gearboxes to get more accurate 3D prints by gearing-down the movement of their stepper motors.
A geared-down motor must turn farther and faster in order to produce the same output movement in the 3D printer which is advantageous if it is not outweighed by the slower movement speed. If the stepper motor has to turn farther then it also has to take more steps to move the printer a given distance; therefore, the geared-down stepper motor has a smaller minimum step-size than the same stepper motor without a gearbox.
While there are many involved factors, planetary gearboxes may help produce very high quality 3D prints.
One popular use of 3D printed planetary gear systems is as toys for children. Since herringbone gear
A herringbone gear, a specific type of double helical gear, is a special type of gear that is a side-to-side (not face-to-face) combination of two Gear#Helical, helical gears of opposite Helix#Handedness, hands. From the top, each helical Groove ...
s are easy to 3D print, it has become very popular to 3D print a moving herringbone planetary gear system for teaching children how gears work. An advantage of herringbone gears is that they don't fall out of the ring and don't need a mounting plate, allowing the moving parts to be clearly seen.
Gallery
See also
* Hypocycloidal gearing *Antikythera mechanism
The Antikythera mechanism ( ) is an Ancient Greece, Ancient Greek hand-powered orrery, described as the oldest example of an analogue computer used to predict astronomy, astronomical positions and eclipses decades in advance. It could also be ...
– ancient mechanical astronomical computer
*Continuously variable transmission
A continuously variable transmission (CVT) is an automatic transmission that can change seamlessly through a continuous range of gear ratios. This contrasts with other transmissions that provide a limited number of gear ratios in fixed steps. T ...
(CVT)
*Cycloidal drive
A cycloidal drive or cycloidal speed reducer is a mechanism for reducing the speed of an input shaft by a certain ratio. Cycloidal speed reducers are capable of relatively high ratios in compact sizes with very low backlash.
The input shaft dr ...
*Epicycloid
In geometry, an epicycloid is a plane curve produced by tracing the path of a chosen point on the circumference of a circle—called an ''epicycle''—which rolls without slipping around a fixed circle. It is a particular kind of roulette.
Equati ...
*Ford Model T
The Ford Model T is an automobile that was produced by Ford Motor Company from October 1, 1908, to May 26, 1927. It is generally regarded as the first affordable automobile, which made car travel available to middle-class Americans. The relati ...
– had a 2 speed planetary transmission.
*Gearbox
Propulsion transmission is the mode of transmitting and controlling propulsion power of a machine. The term ''transmission'' properly refers to the whole drivetrain, including clutch, gearbox, prop shaft (for rear-wheel drive vehicles), differe ...
*Harmonic drive
Strain wave gearing (also known as harmonic gearing) is a type of mechanical gear system that uses a flexible spline with external teeth, which is deformed by a rotating elliptical plug to engage with the internal gear teeth of an outer spline. ...
*Hub gear
A hub gear, internal-gear hub, internally geared hub or just gear hub is a gear ratio changing system commonly used on bicycles that is implemented with planetary or epicyclic gears. The gears and lubricants are sealed within the shell of the h ...
, for bicycles, etc.
*NuVinci Continuously Variable Transmission
Internal CVP shift mechanism
Tilting ball variator schematic. The rotating input disk drives rotation of the balls, which drive the rotating output disk. The rotation axis of the balls can be changed in order to change the ratio of input to outp ...
*Ravigneaux planetary gearset
The Ravigneaux gearset is a double planetary gear set, invented by Pol Ravigneaux, who filed a patent application on July 28, 1949, in Neuilly-sur-Seine France. This planetary gear set, commonly used in automatic transmissions, is constructed from ...
*Lepelletier gear mechanism The name le Peletier (also spelled Lepeletier or Lepelletier) may refer to:
People
*Amédée Louis Michel le Peletier, comte de Saint-Fargeau (1770–1845), French entomologist
* Edmond Lepelletier (1846–1913), French journalist, poet and politi ...
*Rohloff Speedhub
The Rohloff Speedhub is an epicyclic
An epicyclic gear train (also known as a planetary gearset) consists of two gears mounted so that the center of one gear revolves around the center of the other. A carrier connects the centers of the t ...
– 14-ratio bicycle hub gearbox
*Simpson planetary gearset
The Simpson planetary gearset is a compound planetary gear train consisting of two planetary gearsets sharing a common sun gear. A Simpson gearset delivers three forward gears and one reverse, plus neutral, and is commonly employed in three and ...
*Sturmey Archer
Sturmey-Archer was a manufacturing company originally from Nottingham, England. It primarily produced bicycle hub gears, brakes and a great many other sundry bicycle components, most prominently during their heyday as a subsidiary of the Raleigh ...
– First major manufacturer of bicycle hubs using planetary gears
References
External links
Kinematic Models for Design Digital Library (KMODDL)
movies and photos of hundreds of working mechanical-systems models at Cornell.
"Epicyclic gearing animation in SVG"
{{DEFAULTSORT:Epicyclic Gearing Gears