|
Height
Height is measure of vertical distance, either vertical extent (how "tall" something or someone is) or vertical position (how "high" a point is). For example, "The height of that building is 50 m" or "The height of an airplane in-flight is about 10,000 m". For example, "Christopher Columbus is 5 foot 2 inches in vertical height." When the term is used to describe vertical position (of, e.g., an airplane) from sea level, height is more often called ''altitude''. Furthermore, if the point is attached to the Earth (e.g., a mountain peak), then altitude (height above sea level) is called ''elevation''. In a two-dimensional Cartesian space, height is measured along the vertical axis (''y'') between a specific point and another that does not have the same ''y''-value. If both points happen to have the same ''y''-value, then their relative height is zero. In the case of three-dimensional space, height is measured along the vertical ''z'' axis, describing a distance from (or "above") t ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Tree Height
:''This article outlines the basic procedures for measuring trees for scientific and champion tree purposes. It does not cover timber assessment for production purposes, which is focused on marketable wood volumes rather than overall tree size.'' Tree height is the vertical distance between the base of the tree and the tip of the highest branch on the tree, and is difficult to measure accurately. It is not the same as the length of the trunk. If a tree is leaning, the trunk length may be greater than the height of the tree. The base of the tree is where the projection of the pith (center) of the tree intersects the existing supporting surface upon which the tree is growing or where the seed sprouted. If the tree is growing on the side of a cliff, the base of the tree is at the point where the pith would intersect the cliff side. Roots extending down from that point would not add to the height of the tree. On a slope this base point is considered as halfway between the ground level a ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Height Function
A height function is a function that quantifies the complexity of mathematical objects. In Diophantine geometry, height functions quantify the size of solutions to Diophantine equations and are typically functions from a set of points on algebraic varieties (or a set of algebraic varieties) to the real numbers. For instance, the ''classical'' or ''naive height'' over the rational numbers is typically defined to be the maximum of the numerators and denominators of the coordinates (e.g. for the coordinates ), but in a logarithmic scale. Significance Height functions allow mathematicians to count objects, such as rational points, that are otherwise infinite in quantity. For instance, the set of rational numbers of naive height (the maximum of the numerator and denominator when expressed in lowest terms) below any given constant is finite despite the set of rational numbers being infinite. In this sense, height functions can be used to prove asymptotic results such as Baker's t ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
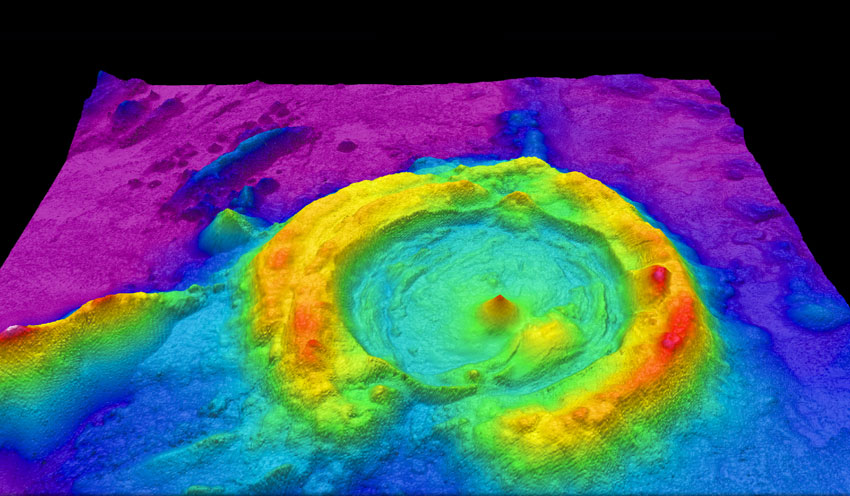
Height Demonstration Diagram
Height is measure of vertical distance, either vertical extent (how "tall" something or someone is) or vertical position (how "high" a point is). For example, "The height of that building is 50 m" or "The height of an airplane in-flight is about 10,000 m". For example, "Christopher Columbus is 5 foot 2 inches in vertical height." When the term is used to describe vertical position (of, e.g., an airplane) from sea level, height is more often called ''altitude''. Furthermore, if the point is attached to the Earth (e.g., a mountain peak), then altitude (height above sea level) is called ''elevation''. In a two-dimensional Cartesian space, height is measured along the vertical axis (''y'') between a specific point and another that does not have the same ''y''-value. If both points happen to have the same ''y''-value, then their relative height is zero. In the case of three-dimensional space, height is measured along the vertical ''z'' axis, describing a distance from (or "above") t ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Sea Level
Mean sea level (MSL, often shortened to sea level) is an average surface level of one or more among Earth's coastal bodies of water from which heights such as elevation may be measured. The global MSL is a type of vertical datuma standardised geodetic datumthat is used, for example, as a chart datum in cartography and marine navigation, or, in aviation, as the standard sea level at which atmospheric pressure is measured to calibrate altitude and, consequently, aircraft flight levels. A common and relatively straightforward mean sea-level standard is instead the midpoint between a mean low and mean high tide at a particular location. Sea levels can be affected by many factors and are known to have varied greatly over geological time scales. Current sea level rise is mainly caused by human-induced climate change. When temperatures rise, Glacier, mountain glaciers and the Ice sheet, polar ice caps melt, increasing the amount of water in water bodies. Because most of human settlem ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Vertical Position
Vertical position or vertical location, also known as vertical level or simply level, is a position along a vertical direction above or below a given vertical datum (reference level). Vertical distance or vertical separation is the distance between two vertical positions. Many vertical coordinates exist for expressing vertical position: depth, height, altitude, elevation, etc. Definitions The International Organization for Standardization (ISO), more specifically ISO 19111, offers the following two definitions: * ''depth'': "distance of a point from a chosen reference surface measured downward along a line perpendicular to that surface." * ''height'': "distance of a point from a chosen reference surface measured upward along a line perpendicular to that surface"; ISO 6709 (2008 version) makes the following additional definition: * ''altitude'': "height where the chosen reference surface is mean sea level" The International Civil Aviation Organization (ICAO) offers similar definit ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Elevation
The elevation of a geographic location is its height above or below a fixed reference point, most commonly a reference geoid, a mathematical model of the Earth's sea level as an equipotential gravitational surface (see Geodetic datum § Vertical datum). The term ''elevation'' is mainly used when referring to points on the Earth's surface, while ''altitude'' or ''geopotential height'' is used for points above the surface, such as an aircraft in flight or a spacecraft in orbit, and '' depth'' is used for points below the surface. Elevation is not to be confused with the distance from the center of the Earth. Due to the equatorial bulge, the summits of Mount Everest and Chimborazo have, respectively, the largest elevation and the largest geocentric distance. Aviation In aviation the term elevation or aerodrome elevation is defined by the ICAO as the highest point of the landing area. It is often measured in feet and can be found in approach charts of the aerodrome. It is n ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Tallest Mountain
This is a list of mountain peaks ordered by their topographic prominence. Terminology The prominence of a peak is the minimum height of climb to the summit on any route from a higher peak, or from sea level if there is no higher peak. The lowest point on that route is the col. For full definitions and explanations of ''topographic prominence'', ''key col'', and ''parent'', see topographic prominence. In particular, the different definitions of the parent of a peak are addressed at length in that article. ''Height'' on the other hand simply means elevation of the summit above sea level. Regarding parents, the ''prominence parent'' of peak A can be found by dividing the island or region in question into territories, by tracing the runoff from the key col (mountain pass) of every peak that is more prominent than peak A. The parent is the peak whose territory peak A resides in. The ''encirclement parent'' is found by tracing the contour below peak A's key col and picking the highe ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Altitude
Altitude or height (also sometimes known as depth) is a distance measurement, usually in the vertical or "up" direction, between a reference datum and a point or object. The exact definition and reference datum varies according to the context (e.g., aviation, geometry, geographical survey, sport, or atmospheric pressure). Although the term ''altitude'' is commonly used to mean the height above sea level of a location, in geography the term elevation is often preferred for this usage. Vertical distance measurements in the "down" direction are commonly referred to as depth. In aviation In aviation, the term altitude can have several meanings, and is always qualified by explicitly adding a modifier (e.g. "true altitude"), or implicitly through the context of the communication. Parties exchanging altitude information must be clear which definition is being used. Aviation altitude is measured using either mean sea level (MSL) or local ground level (above ground level, or ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Geodesy
Geodesy ( ) is the Earth science of accurately measuring and understanding Earth's figure (geometric shape and size), orientation in space, and gravity. The field also incorporates studies of how these properties change over time and equivalent measurements for other planets (known as '' planetary geodesy''). Geodynamical phenomena, including crustal motion, tides and polar motion, can be studied by designing global and national control networks, applying space geodesy and terrestrial geodetic techniques and relying on datums and coordinate systems. The job title is geodesist or geodetic surveyor. History Definition The word geodesy comes from the Ancient Greek word ''geodaisia'' (literally, "division of Earth"). It is primarily concerned with positioning within the temporally varying gravitational field. Geodesy in the German-speaking world is divided into "higher geodesy" ( or ), which is concerned with measuring Earth on the global scale, and "practical geodes ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Topographic Prominence
In topography, prominence (also referred to as autonomous height, relative height, and shoulder drop in US English, and drop or relative height in British English) measures the height of a mountain or hill's summit relative to the lowest contour line encircling it but containing no higher summit within it. It is a measure of the independence of a summit. A peak's ''key col'' (the highest col surrounding the peak) is a unique point on this contour line and the ''parent peak'' is some higher mountain, selected according to various criteria. Definitions The prominence of a peak may be defined as the least drop in height necessary in order to get from the summit to any higher terrain. This can be calculated for a given peak in the following way: for every path connecting the peak to higher terrain, find the lowest point on the path; the ''key col'' (or ''key Saddle point, saddle'', or ''linking col'', or ''link'') is defined as the highest of these points, along all connecting pat ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Circular Segment
In geometry, a circular segment (symbol: ), also known as a disk segment, is a region of a disk which is "cut off" from the rest of the disk by a secant or a chord. More formally, a circular segment is a region of two-dimensional space that is bounded by a circular arc (of less than π radians by convention) and by the circular chord connecting the endpoints of the arc. Formulae Let ''R'' be the radius of the arc which forms part of the perimeter of the segment, ''θ'' the central angle subtending the arc in radians, ''c'' the chord length, ''s'' the arc length, ''h'' the sagitta (height) of the segment, ''d'' the apothem of the segment, and ''a'' the area of the segment. Usually, chord length and height are given or measured, and sometimes the arc length as part of the perimeter, and the unknowns are area and sometimes arc length. These can't be calculated simply from chord length and height, so two intermediate quantities, the radius and central angle are usually calcula ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Width
Length is a measure of distance. In the International System of Quantities, length is a quantity with dimension distance. In most systems of measurement a base unit for length is chosen, from which all other units are derived. In the International System of Units (SI) system the base unit for length is the metre. Length is commonly understood to mean the most extended dimension of a fixed object. However, this is not always the case and may depend on the position the object is in. Various terms for the length of a fixed object are used, and these include height, which is vertical length or vertical extent, and width, breadth or depth. Height is used when there is a base from which vertical measurements can be taken. Width or breadth usually refer to a shorter dimension when length is the longest one. Depth is used for the third dimension of a three dimensional object. Length is the measure of one spatial dimension, whereas area is a measure of two dimensions (length square ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
