|
Triangle Congruence
In geometry, two figures or objects are congruent if they have the same shape and size, or if one has the same shape and size as the mirror image of the other. More formally, two sets of points are called congruent if, and only if, one can be transformed into the other by an isometry, i.e., a combination of rigid motions, namely a translation, a rotation, and a reflection. This means that either object can be repositioned and reflected (but not resized) so as to coincide precisely with the other object. Therefore two distinct plane figures on a piece of paper are congruent if they can be cut out and then matched up completely. Turning the paper over is permitted. In elementary geometry the word ''congruent'' is often used as follows. The word ''equal'' is often used in place of ''congruent'' for these objects. *Two line segments are congruent if they have the same length. *Two angles are congruent if they have the same measure. *Two circles are congruent if they have the sa ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Congruent Triangles
In geometry, two figures or objects are congruent if they have the same shape and size, or if one has the same shape and size as the mirror image of the other. More formally, two sets of points are called congruent if, and only if, one can be transformed into the other by an isometry, i.e., a combination of rigid motions, namely a translation, a rotation, and a reflection. This means that either object can be repositioned and reflected (but not resized) so as to coincide precisely with the other object. Therefore two distinct plane figures on a piece of paper are congruent if they can be cut out and then matched up completely. Turning the paper over is permitted. In elementary geometry the word ''congruent'' is often used as follows. The word ''equal'' is often used in place of ''congruent'' for these objects. *Two line segments are congruent if they have the same length. *Two angles are congruent if they have the same measure. *Two circles are congruent if they have the s ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Congruent Non-congruent Triangles
Congruence may refer to: Mathematics * Congruence (geometry), being the same size and shape * Congruence or congruence relation, in abstract algebra, an equivalence relation on an algebraic structure that is compatible with the structure * In modular arithmetic, having the same remainder when divided by a specified integer **Ramanujan's congruences, congruences for the partition function, , first discovered by Ramanujan in 1919 **Congruence subgroup, a subgroup defined by congruence conditions on the entries of a matrix group with integer entries **Congruence of squares, in number theory, a congruence commonly used in integer factorization algorithms * Matrix congruence, an equivalence relation between two matrices * Congruence (manifolds), in the theory of smooth manifolds, the set of integral curves defined by a nonvanishing vector field defined on the manifold * Congruence (general relativity), in general relativity, a congruence in a four-dimensional Lorentzian manifold that ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Congruence Of Triangles
{{disambiguation ...
Congruence of triangles may refer to: * Congruence (geometry)#Congruence of triangles * Solution of triangles Solution of triangles ( la, solutio triangulorum) is the main trigonometric problem of finding the characteristics of a triangle (angles and lengths of sides), when some of these are known. The triangle can be located on a plane or on a sphere. Appl ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Acronym
An acronym is a word or name formed from the initial components of a longer name or phrase. Acronyms are usually formed from the initial letters of words, as in ''NATO'' (''North Atlantic Treaty Organization''), but sometimes use syllables, as in ''Benelux'' (short for ''Belgium, the Netherlands, and Luxembourg''). They can also be a mixture, as in ''radar'' (''Radio Detection And Ranging''). Acronyms can be pronounced as words, like ''NASA'' and ''UNESCO''; as individual letters, like ''FBI'', ''TNT'', and ''ATM''; or as both letters and words, like '' JPEG'' (pronounced ') and ''IUPAC''. Some are not universally pronounced one way or the other and it depends on the speaker's preference or the context in which it is being used, such as '' SQL'' (either "sequel" or "ess-cue-el"). The broader sense of ''acronym''—the meaning of which includes terms pronounced as letters—is sometimes criticized, but it is the term's original meaning and is in common use. Dictionary and st ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Hyperbolic Geometry
In mathematics, hyperbolic geometry (also called Lobachevskian geometry or Bolyai– Lobachevskian geometry) is a non-Euclidean geometry. The parallel postulate of Euclidean geometry is replaced with: :For any given line ''R'' and point ''P'' not on ''R'', in the plane containing both line ''R'' and point ''P'' there are at least two distinct lines through ''P'' that do not intersect ''R''. (Compare the above with Playfair's axiom, the modern version of Euclid's parallel postulate.) Hyperbolic plane geometry is also the geometry of pseudospherical surfaces, surfaces with a constant negative Gaussian curvature. Saddle surfaces have negative Gaussian curvature in at least some regions, where they locally resemble the hyperbolic plane. A modern use of hyperbolic geometry is in the theory of special relativity, particularly the Minkowski model. When geometers first realised they were working with something other than the standard Euclidean geometry, they described their geomet ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Spherical Geometry
300px, A sphere with a spherical triangle on it. Spherical geometry is the geometry of the two-dimensional surface of a sphere. In this context the word "sphere" refers only to the 2-dimensional surface and other terms like "ball" or "solid sphere" are used for the surface together with its 3-dimensional interior. Long studied for its practical applications to navigation and astronomy, spherical geometry bears many similarities and relationships to, and important differences from, Euclidean plane geometry. The sphere has for the most part been studied as a part of 3-dimensional Euclidean geometry (often called solid geometry), the surface thought of as placed inside an ambient 3-d space. It can also be analyzed by "intrinsic" methods that only involve the surface itself, and do not refer to, or even assume the existence of, any surrounding space outside or inside the sphere. Because a sphere and a plane differ geometrically, (intrinsic) spherical geometry has some featu ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Similarity (geometry)
In Euclidean geometry, two objects are similar if they have the same shape, or one has the same shape as the mirror image of the other. More precisely, one can be obtained from the other by uniformly scaling (geometry), scaling (enlarging or reducing), possibly with additional translation (geometry), translation, rotation (mathematics), rotation and reflection (mathematics), reflection. This means that either object can be rescaled, repositioned, and reflected, so as to coincide precisely with the other object. If two objects are similar, each is congruence (geometry), congruent to the result of a particular uniform scaling of the other. For example, all circles are similar to each other, all squares are similar to each other, and all equilateral triangles are similar to each other. On the other hand, ellipses are not all similar to each other, rectangles are not all similar to each other, and isosceles triangles are not all similar to each other. If two angles of a triangle h ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Ambiguous Case
In trigonometry, the law of sines, sine law, sine formula, or sine rule is an equation relating the lengths of the sides of any triangle to the sines of its angles. According to the law, \frac \,=\, \frac \,=\, \frac \,=\, 2R, where , and are the lengths of the sides of a triangle, and , and are the opposite angles (see figure 2), while is the radius of the triangle's circumcircle. When the last part of the equation is not used, the law is sometimes stated using the reciprocals; \frac \,=\, \frac \,=\, \frac. The law of sines can be used to compute the remaining sides of a triangle when two angles and a side are known—a technique known as triangulation. It can also be used when two sides and one of the non-enclosed angles are known. In some such cases, the triangle is not uniquely determined by this data (called the ''ambiguous case'') and the technique gives two possible values for the enclosed angle. The law of sines is one of two trigonometric equations commonly a ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
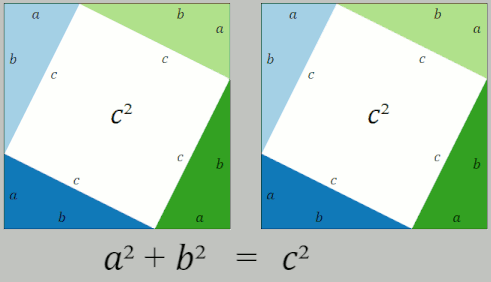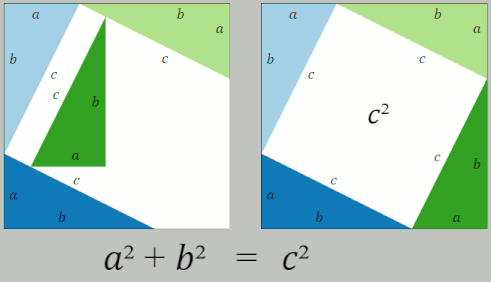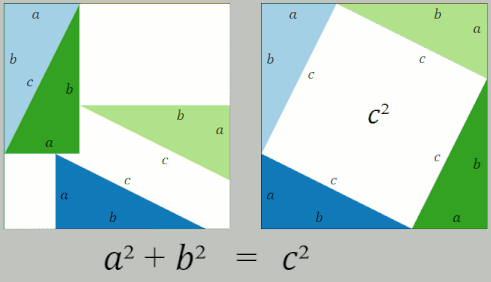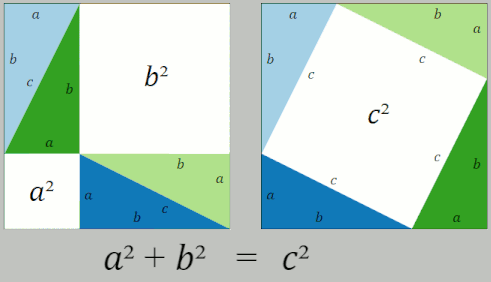
Pythagorean Theorem
In mathematics, the Pythagorean theorem or Pythagoras' theorem is a fundamental relation in Euclidean geometry between the three sides of a right triangle. It states that the area of the square whose side is the hypotenuse (the side opposite the right angle) is equal to the sum of the areas of the squares on the other two sides. This theorem can be written as an equation relating the lengths of the sides ''a'', ''b'' and the hypotenuse ''c'', often called the Pythagorean equation: :a^2 + b^2 = c^2 , The theorem is named for the Greek philosopher Pythagoras, born around 570 BC. The theorem has been proven numerous times by many different methods – possibly the most for any mathematical theorem. The proofs are diverse, including both geometric proofs and algebraic proofs, with some dating back thousands of years. When Euclidean space is represented by a Cartesian coordinate system in analytic geometry, Euclidean distance satisfies the Pythagorean relation: the squared dist ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
School Mathematics Study Group
The School Mathematics Study Group (SMSG) was an American academic think tank focused on the subject of reform in mathematics education. Directed by Edward G. Begle and financed by the National Science Foundation, the group was created in the wake of the Sputnik crisis in 1958 and tasked with creating and implementing mathematics curricula for primary and secondary education, which it did until its termination in 1977. The efforts of the SMSG yielded a reform in mathematics education known as New Math which was promulgated in a series of reports, culminating in a series published by Random House called the ''New Mathematical Library'' (Vol. 1 is Ivan Niven's ''Numbers: Rational and Irrational''). In the early years, SMSG also produced a set of draft textbooks in typewritten paperback format for elementary, middle and high school students. Perhaps the most authoritative collection of materials from the School Mathematics Study Group is now housed in the Archives of American M ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Theorem
In mathematics, a theorem is a statement that has been proved, or can be proved. The ''proof'' of a theorem is a logical argument that uses the inference rules of a deductive system to establish that the theorem is a logical consequence of the axioms and previously proved theorems. In the mainstream of mathematics, the axioms and the inference rules are commonly left implicit, and, in this case, they are almost always those of Zermelo–Fraenkel set theory with the axiom of choice, or of a less powerful theory, such as Peano arithmetic. A notable exception is Wiles's proof of Fermat's Last Theorem, which involves the Grothendieck universes whose existence requires the addition of a new axiom to the set theory. Generally, an assertion that is explicitly called a theorem is a proved result that is not an immediate consequence of other known theorems. Moreover, many authors qualify as ''theorems'' only the most important results, and use the terms ''lemma'', ''proposition'' and ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Thales Of Miletus
Thales of Miletus ( ; grc-gre, Θαλῆς; ) was a Greek mathematician, astronomer, statesman, and pre-Socratic philosopher from Miletus in Ionia, Asia Minor. He was one of the Seven Sages of Greece. Many, most notably Aristotle, regarded him as the first philosopher in the Greek tradition, and he is otherwise historically recognized as the first individual known to have entertained and engaged in scientific philosophy.Frank N. Magill''The Ancient World: Dictionary of World Biography'', Volume 1 Routledge, 2003 He is often referred to as the Father of Science. Thales is recognized for breaking from the use of mythology to explain the world and the universe, instead explaining natural objects and phenomena by offering naturalistic theories and hypotheses. Almost all the other pre-Socratic philosophers followed him in explaining nature as deriving from a unity of everything based on the existence of a single ultimate substance instead of using mythological explanations. Ar ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
.svg "Click for more on -> Ambiguous Case")
.PNG "Click for more on -> Theorem")
