|
Thomas Rotation
In theoretical physics, the composition of two non- collinear Lorentz boosts results in a Lorentz transformation that is not a pure boost but is the composition of a boost and a rotation. This rotation is called Thomas rotation, Thomas–Wigner rotation or Wigner rotation. The rotation was discovered and proved by Ludwik Silberstein in his 1914 book 'Relativity', rediscovered by Llewellyn Thomas in 1926, and rederived by Wigner in 1939. Wigner acknowledged Silberstein. If a sequence of non-collinear boosts returns an object to its initial velocity, then the sequence of Wigner rotations can combine to produce a net rotation called the Thomas precession. There are still ongoing discussions about the correct form of equations for the Thomas rotation in different reference systems with contradicting results. Goldstein: :''The spatial rotation resulting from the successive application of two non-collinear Lorentz transformations have been declared every bit as paradoxical as the m ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Wigner
Eugene Paul "E. P." Wigner ( hu, Wigner Jenő Pál, ; November 17, 1902 – January 1, 1995) was a Hungarian-American theoretical physicist who also contributed to mathematical physics. He received the Nobel Prize in Physics in 1963 "for his contributions to the theory of the atomic nucleus and the elementary particles, particularly through the discovery and application of fundamental symmetry principles". A graduate of the Technical University of Berlin, Wigner worked as an assistant to Karl Weissenberg and Richard Becker at the Kaiser Wilhelm Institute in Berlin, and David Hilbert at the University of Göttingen. Wigner and Hermann Weyl were responsible for introducing group theory into physics, particularly the theory of symmetry in physics. Along the way he performed ground-breaking work in pure mathematics, in which he authored a number of mathematical theorems. In particular, Wigner's theorem is a cornerstone in the mathematical formulation of quantum mechanics. He is also ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Nonlinear
In mathematics and science, a nonlinear system is a system in which the change of the output is not proportional to the change of the input. Nonlinear problems are of interest to engineers, biologists, physicists, mathematicians, and many other scientists because most systems are inherently nonlinear in nature. Nonlinear dynamical systems, describing changes in variables over time, may appear chaotic, unpredictable, or counterintuitive, contrasting with much simpler linear systems. Typically, the behavior of a nonlinear system is described in mathematics by a nonlinear system of equations, which is a set of simultaneous equations in which the unknowns (or the unknown functions in the case of differential equations) appear as variables of a polynomial of degree higher than one or in the argument of a function which is not a polynomial of degree one. In other words, in a nonlinear system of equations, the equation(s) to be solved cannot be written as a linear combination of the un ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Gyrovector Space
A gyrovector space is a mathematical concept proposed by Abraham A. Ungar for studying hyperbolic geometry in analogy to the way vector spaces are used in Euclidean geometry.Abraham A. Ungar (2005), "Analytic Hyperbolic Geometry: Mathematical Foundations and Applications", Published by World Scientific, , Ungar introduced the concept of gyrovectors that have addition based on gyrogroups instead of vectors which have addition based on groups. Ungar developed his concept as a tool for the formulation of special relativity as an alternative to the use of Lorentz transformations to represent compositions of velocities (also called boosts – "boosts" are aspects of relative velocities, and should not be conflated with "translations"). This is achieved by introducing "gyro operators"; two 3d velocity vectors are used to construct an operator, which acts on another 3d velocity. Name Gyrogroups are weakly associative group-like structures. Ungar proposed the term gyrogroup for ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Rotation Matrix
In linear algebra, a rotation matrix is a transformation matrix that is used to perform a rotation in Euclidean space. For example, using the convention below, the matrix :R = \begin \cos \theta & -\sin \theta \\ \sin \theta & \cos \theta \end rotates points in the plane counterclockwise through an angle with respect to the positive axis about the origin of a two-dimensional Cartesian coordinate system. To perform the rotation on a plane point with standard coordinates , it should be written as a column vector, and multiplied by the matrix : : R\mathbf = \begin \cos \theta & -\sin \theta \\ \sin \theta & \cos \theta \end \begin x \\ y \end = \begin x\cos\theta-y\sin\theta \\ x\sin\theta+y\cos\theta \end. If and are the endpoint coordinates of a vector, where is cosine and is sine, then the above equations become the trigonometric summation angle formulae. Indeed, a rotation matrix can be seen as the trigonometric summation angle formulae in matrix form. One w ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Symmetric Matrix
In linear algebra, a symmetric matrix is a square matrix that is equal to its transpose. Formally, Because equal matrices have equal dimensions, only square matrices can be symmetric. The entries of a symmetric matrix are symmetric with respect to the main diagonal. So if a_ denotes the entry in the ith row and jth column then for all indices i and j. Every square diagonal matrix is symmetric, since all off-diagonal elements are zero. Similarly in characteristic different from 2, each diagonal element of a skew-symmetric matrix must be zero, since each is its own negative. In linear algebra, a real symmetric matrix represents a self-adjoint operator represented in an orthonormal basis over a real inner product space. The corresponding object for a complex inner product space is a Hermitian matrix with complex-valued entries, which is equal to its conjugate transpose. Therefore, in linear algebra over the complex numbers, it is often assumed that a symmetric matrix refe ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
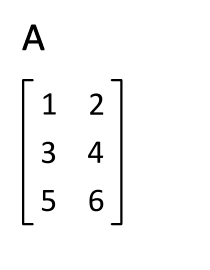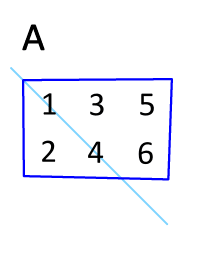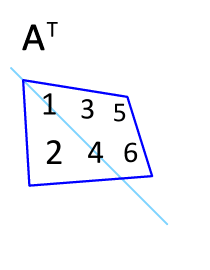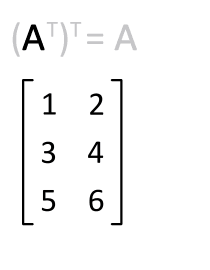
Matrix Transpose
In linear algebra, the transpose of a matrix is an operator which flips a matrix over its diagonal; that is, it switches the row and column indices of the matrix by producing another matrix, often denoted by (among other notations). The transpose of a matrix was introduced in 1858 by the British mathematician Arthur Cayley. In the case of a logical matrix representing a binary relation R, the transpose corresponds to the converse relation RT. Transpose of a matrix Definition The transpose of a matrix , denoted by , , , A^, , , or , may be constructed by any one of the following methods: # Reflect over its main diagonal (which runs from top-left to bottom-right) to obtain #Write the rows of as the columns of #Write the columns of as the rows of Formally, the -th row, -th column element of is the -th row, -th column element of : :\left mathbf^\operatorname\right = \left mathbf\right. If is an matrix, then is an matrix. In the case of square matrices, ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Column Vector
In linear algebra, a column vector with m elements is an m \times 1 matrix consisting of a single column of m entries, for example, \boldsymbol = \begin x_1 \\ x_2 \\ \vdots \\ x_m \end. Similarly, a row vector is a 1 \times n matrix for some n, consisting of a single row of n entries, \boldsymbol a = \begin a_1 & a_2 & \dots & a_n \end. (Throughout this article, boldface is used for both row and column vectors.) The transpose (indicated by T) of any row vector is a column vector, and the transpose of any column vector is a row vector: \begin x_1 \; x_2 \; \dots \; x_m \end^ = \begin x_1 \\ x_2 \\ \vdots \\ x_m \end and \begin x_1 \\ x_2 \\ \vdots \\ x_m \end^ = \begin x_1 \; x_2 \; \dots \; x_m \end. The set of all row vectors with ''n'' entries in a given field (such as the real numbers) forms an ''n''-dimensional vector space; similarly, the set of all column vectors with ''m'' entries forms an ''m''-dimensional vector space. The space of row vectors with ''n'' entries can b ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Block Matrix
In mathematics, a block matrix or a partitioned matrix is a matrix that is '' interpreted'' as having been broken into sections called blocks or submatrices. Intuitively, a matrix interpreted as a block matrix can be visualized as the original matrix with a collection of horizontal and vertical lines, which break it up, or partition it, into a collection of smaller matrices. Any matrix may be interpreted as a block matrix in one or more ways, with each interpretation defined by how its rows and columns are partitioned. This notion can be made more precise for an n by m matrix M by partitioning n into a collection \text, and then partitioning m into a collection \text. The original matrix is then considered as the "total" of these groups, in the sense that the (i, j) entry of the original matrix corresponds in a 1-to-1 way with some (s, t) offset entry of some (x,y), where x \in \text and y \in \text. Block matrix algebra arises in general from biproducts in categories of matrices ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Lorentz Boosts And Thomas Rotation 4
Lorentz is a name derived from the Roman surname, Laurentius, which means "from Laurentum". It is the German form of Laurence. Notable people with the name include: Given name * Lorentz Aspen (born 1978), Norwegian heavy metal pianist and keyboardist * Lorentz Dietrichson (1834–1917), Norwegian poet and historian of art and literature * Lorentz Eichstadt (1596–1660), German mathematician and astronomer * Lorentz Harboe Ree (1888–1962), Norwegian architect * Lorentz Lange (1783–1860), Norwegian judge and politician * Lorentz Reige (born 1990), Swedish dancer * Lorentz Reitan (born 1946), Norwegian musicologist Mononym * Lorentz (rapper), real name Lorentz Alexander, Swedish singer and rapper Surname * Dominique Lorentz, French investigative journalist who has written books on nuclear proliferation * Friedrich Lorentz, author of works on the Pomeranian language * Hendrik Antoon Lorentz (1853–1928), Dutch physicist and Nobel Prize winner * Hendrikus Albertus Lorentz (1871 ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Lorentz Boosts And Thomas Rotation 3
Lorentz is a name derived from the Roman surname, Laurentius, which means "from Laurentum". It is the German form of Laurence. Notable people with the name include: Given name * Lorentz Aspen (born 1978), Norwegian heavy metal pianist and keyboardist * Lorentz Dietrichson (1834–1917), Norwegian poet and historian of art and literature * Lorentz Eichstadt (1596–1660), German mathematician and astronomer * Lorentz Harboe Ree (1888–1962), Norwegian architect * Lorentz Lange (1783–1860), Norwegian judge and politician * Lorentz Reige (born 1990), Swedish dancer * Lorentz Reitan (born 1946), Norwegian musicologist Mononym * Lorentz (rapper), real name Lorentz Alexander, Swedish singer and rapper Surname * Dominique Lorentz, French investigative journalist who has written books on nuclear proliferation * Friedrich Lorentz, author of works on the Pomeranian language * Hendrik Antoon Lorentz (1853–1928), Dutch physicist and Nobel Prize winner * Hendrikus Albertus Lorentz (1871 ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Lorentz Boosts And Thomas Rotation 2
Lorentz is a name derived from the Roman surname, Laurentius, which means "from Laurentum". It is the German form of Laurence. Notable people with the name include: Given name * Lorentz Aspen (born 1978), Norwegian heavy metal pianist and keyboardist * Lorentz Dietrichson (1834–1917), Norwegian poet and historian of art and literature * Lorentz Eichstadt (1596–1660), German mathematician and astronomer * Lorentz Harboe Ree (1888–1962), Norwegian architect * Lorentz Lange (1783–1860), Norwegian judge and politician * Lorentz Reige (born 1990), Swedish dancer * Lorentz Reitan (born 1946), Norwegian musicologist Mononym * Lorentz (rapper), real name Lorentz Alexander, Swedish singer and rapper Surname * Dominique Lorentz, French investigative journalist who has written books on nuclear proliferation * Friedrich Lorentz, author of works on the Pomeranian language * Hendrik Antoon Lorentz (1853–1928), Dutch physicist and Nobel Prize winner * Hendrikus Albertus Lorentz (1871 ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Lorentz Boosts And Thomas Rotation 1
Lorentz is a name derived from the Roman surname, Laurentius, which means "from Laurentum". It is the German form of Laurence. Notable people with the name include: Given name * Lorentz Aspen (born 1978), Norwegian heavy metal pianist and keyboardist * Lorentz Dietrichson (1834–1917), Norwegian poet and historian of art and literature * Lorentz Eichstadt (1596–1660), German mathematician and astronomer * Lorentz Harboe Ree (1888–1962), Norwegian architect * Lorentz Lange (1783–1860), Norwegian judge and politician * Lorentz Reige (born 1990), Swedish dancer * Lorentz Reitan (born 1946), Norwegian musicologist Mononym * Lorentz (rapper), real name Lorentz Alexander, Swedish singer and rapper Surname * Dominique Lorentz, French investigative journalist who has written books on nuclear proliferation * Friedrich Lorentz, author of works on the Pomeranian language * Hendrik Antoon Lorentz (1853–1928), Dutch physicist and Nobel Prize winner * Hendrikus Albertus Lorentz (1871 ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |