|
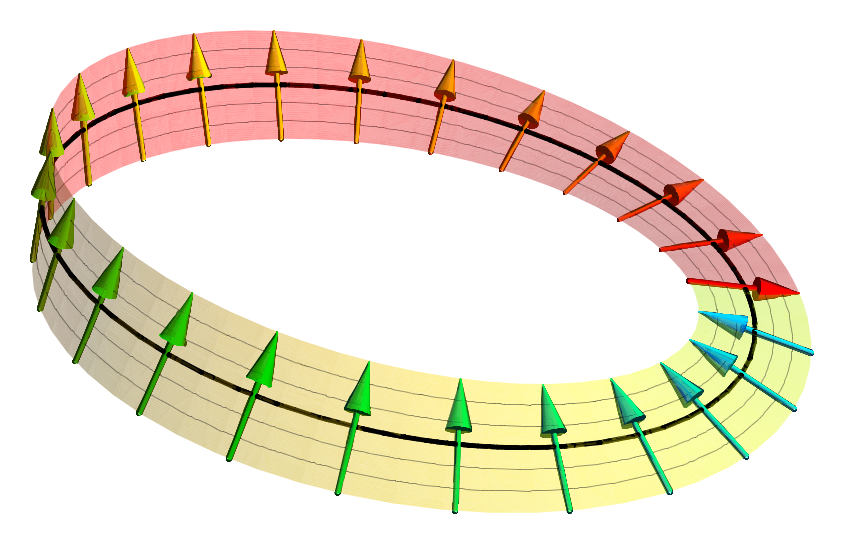
Plate Trick
In mathematics and physics, the plate trick, also known as Dirac's string trick (after Paul Dirac, who introduced and popularized it), the belt trick, or the Balinese cup trick (it appears in the Balinese candle dance), is any of several demonstrations of the idea that rotating an object with strings attached to it by 360 degrees does not return the system to its original state, while a second rotation of 360 degrees, a total rotation of 720 degrees, does. Mathematically, it is a demonstration of the theorem that SU(2) (which double-covers SO(3)) is simply connected. To say that SU(2) double-covers SO(3) essentially means that the unit quaternions represent the group of rotations twice over. A detailed, intuitive, yet semi-formal articulation can be found in the article on tangloids. Demonstrations Resting a small plate flat on the palm, it is possible to perform two rotations of one's hand while keeping the plate upright. After the first rotation of the hand, the arm will be tw ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Mathematics
Mathematics is a field of study that discovers and organizes methods, Mathematical theory, theories and theorems that are developed and Mathematical proof, proved for the needs of empirical sciences and mathematics itself. There are many areas of mathematics, which include number theory (the study of numbers), algebra (the study of formulas and related structures), geometry (the study of shapes and spaces that contain them), Mathematical analysis, analysis (the study of continuous changes), and set theory (presently used as a foundation for all mathematics). Mathematics involves the description and manipulation of mathematical object, abstract objects that consist of either abstraction (mathematics), abstractions from nature orin modern mathematicspurely abstract entities that are stipulated to have certain properties, called axioms. Mathematics uses pure reason to proof (mathematics), prove properties of objects, a ''proof'' consisting of a succession of applications of in ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Belt Gürtel
Belt or The Belt may refer to: Apparel * Belt (clothing), a leather or fabric band worn around the waist * Championship belt, a type of trophy used primarily in combat sports * Colored belts, such as a black belt or red belt, worn by martial arts practitioners to signify rank in the kyū ranking system Geology * Orogenic belt * Greenstone belt * A large-scale linear or curved array or belt of igneous rocks (e.g. Transscandinavian Igneous Belt) * A large-scale linear or curved array of mineral deposits (e.g. Bolivian tin belt) * Paired metamorphic belts Mechanical and vehicular * Belt (mechanical), a looped strip of material used to link multiple rotating shafts * Conveyor belt, a device for transporting goods along a fixed track * Belt manlift, a device for moving people between floors in a building or grain elevator. * Seat belt, a safety device in automobiles and on the plane * Timing belt, part of an internal combustion engine * Serpentine belt, move accessories of a comb ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Spinors
In geometry and physics, spinors (pronounced "spinner" IPA ) are elements of a complex numbers, complex vector space that can be associated with Euclidean space. A spinor transforms linearly when the Euclidean space is subjected to a slight (infinitesimal transformation, infinitesimal) rotation, but unlike Euclidean vector, geometric vectors and tensors, a spinor transforms to its negative when the space rotates through 360° (see picture). It takes a rotation of 720° for a spinor to go back to its original state. This property characterizes spinors: spinors can be viewed as the "square roots" of vectors (although this is inaccurate and may be misleading; they are better viewed as "square roots" of Section (fiber bundle), sections of vector bundles – in the case of the exterior algebra bundle of the cotangent bundle, they thus become "square roots" of differential forms). It is also possible to associate a substantially similar notion of spinor to Minkowski space, in which cas ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Rotation In Three Dimensions
Rotation or rotational/rotary motion is the circular movement of an object around a central line, known as an ''axis of rotation''. A plane figure can rotate in either a clockwise or counterclockwise sense around a perpendicular axis intersecting anywhere inside or outside the figure at a ''center of rotation''. A solid figure has an infinite number of possible axes and angles of rotation, including chaotic rotation (between arbitrary orientations), in contrast to rotation around a axis. The special case of a rotation with an internal axis passing through the body's own center of mass is known as a spin (or ''autorotation''). In that case, the surface intersection of the internal ''spin axis'' can be called a ''pole''; for example, Earth's rotation defines the geographical poles. A rotation around an axis completely external to the moving body is called a revolution (or ''orbit''), e.g. Earth's orbit around the Sun. The ends of the external ''axis of revolution'' can be ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Spin-1/2
In quantum mechanics, spin is an intrinsic property of all elementary particles. All known fermions, the particles that constitute ordinary matter, have a spin of . The spin number describes how many symmetrical facets a particle has in one full rotation; a spin of means that the particle must be rotated by two full turns (through 720°) before it has the same configuration as when it started. Particles with net spin include the proton, neutron, electron, neutrino, and quarks. The dynamics of spin- objects cannot be accurately described using classical physics; they are among the simplest systems whose description requires quantum mechanics. As such, the study of the behavior of spin- systems forms a central part of quantum mechanics. Stern–Gerlach experiment The necessity of introducing half-integer spin goes back experimentally to the results of the Stern–Gerlach experiment. A beam of atoms is run through a strong heterogeneous magnetic field, which then splits ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Tangloids
Tangloids is a mathematical game for two players created by Piet Hein to model the calculus of spinors. A description of the game appeared in the book ''"Martin Gardner's New Mathematical Diversions from Scientific American"'' by Martin Gardner from 1996 in a section on the mathematics of braiding.M. Gardner''Sphere Packing, Lewis Carroll, and Reversi: Martin Gardner's New Mathematical Diversions'', Cambridge University Press, September, 2009, Two flat blocks of wood each pierced with three small holes are joined with three parallel strings. Each player holds one of the blocks of wood. The first player holds one block of wood still, while the other player rotates the other block of wood for two full revolutions. The plane of rotation is perpendicular to the strings when not tangled. The strings now overlap each other. Then the first player tries to untangle the strings without rotating either piece of wood. Only translations (moving the pieces without rotating) are allowed. Af ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Orientation Entanglement
In mathematics and physics, the notion of orientation entanglement is sometimes used to develop intuition relating to the geometry of spinors or alternatively as a concrete realization of the failure of the special orthogonal groups to be simply connected. Elementary description Spatial vectors alone are not sufficient to describe fully the properties of rotations in space. Consider the following example. A coffee cup is suspended in a room by a pair of elastic rubber bands fixed to the walls of the room. The cup is rotated by its handle through a full twist of 360°, so that the handle is brought all the way around the central vertical axis of the cup and back to its original position. Note that after this rotation, the cup has been returned to its original orientation, but that its orientation with respect to the walls is ''twisted''. In other words, if we lower the coffee cup to the floor of the room, the two bands will coil around each other in one full twist of a double ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Spin–statistics Theorem
The spin–statistics theorem proves that the observed relationship between the intrinsic spin of a particle (angular momentum not due to the orbital motion) and the quantum particle statistics of collections of such particles is a consequence of the mathematics of quantum mechanics. According to the theorem, the many-body wave function for elementary particles with integer spin ( bosons) is symmetric under the exchange of any two particles, whereas for particles with half-integer spin ( fermions), the wave function is antisymmetric under such an exchange. A consequence of the theorem is that non-interacting particles with integer spin obey Bose–Einstein statistics, while those with half-integer spin obey Fermi–Dirac statistics. Background The statistics of indistinguishable particles is among the most fundamental of physical effects. The Pauli exclusion principle that every occupied quantum state contains at most one fermion controls the formation of matter. The basic bu ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Anti-twister Mechanism
The anti-twister or antitwister mechanism is a method of connecting a flexible link between two objects, one of which is rotating with respect to the other, in a way that prevents the link from becoming twisted. The link could be an electrical cable or a flexible conduit. This mechanism is intended as an alternative to the usual method of supplying electric power to a rotating device, the use of slip rings. The slip rings are attached to one part of the machine, and a set of fine metal brushes are attached to the other part. The brushes are kept in sliding contact with the slip rings, providing an electrical path between the two parts while allowing the parts to rotate about each other. However, this presents problems with smaller devices. Whereas with large devices minor fluctuations in the power provided through the brush mechanism are inconsequential, in the case of tiny electronic components, the brushing introduces unacceptable levels of noise (electronics), noise in the strea ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Breather
In physics, a breather is a nonlinear wave in which energy concentrates in a localized and oscillatory fashion. This contradicts with the expectations derived from the corresponding linear system for infinitesimal amplitudes, which tends towards an even distribution of initially localized energy. A discrete breather is a breather solution on a nonlinear lattice. The term breather originates from the characteristic that most breathers are localized in space and oscillate (as a breathe) in time. But also the opposite situation: oscillations in space and localized in time, is denoted as a breather. Overview A breather is a localized periodic solution of either continuous media equations or discrete lattice equations. The exactly solvable sine-Gordon equation and the focusing nonlinear Schrödinger equation Translated from ''Teoreticheskaya i Matematicheskaya Fizika'' 72(2): 183–196, August, 1987. are examples of one-dimensional partial differential equations that possess ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Classical Heisenberg Model
In statistical physics, the classical Heisenberg model, developed by Werner Heisenberg, is the n = 3 case of the ''n''-vector model, one of the models used to model ferromagnetism and other phenomena. Definition The classical Heisenberg model can be formulated as follows: take a d-dimensional lattice, and place a set of spins of unit length, :\vec_i \in \mathbb^3, , \vec_i, =1\quad (1), on each lattice node. The model is defined through the following Hamiltonian: : \mathcal = -\sum_ \mathcal_ \vec_i \cdot \vec_j\quad (2) where : \mathcal_ = \begin J & \mboxi, j\mbox \\ 0 & \mbox\end is a coupling between spins. Properties * The general mathematical formalism used to describe and solve the Heisenberg model and certain generalizations is developed in the article on the Potts model. * In the continuum limit the Heisenberg model (2) gives the following equation of motion :: \vec_=\vec\wedge \vec_. :This equation is called the continuous classical Heisenberg ferromagnet equatio ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Belt Buckle
A belt buckle is a buckle, a clasp for fastening two ends, such as of straps or a belt (clothing), belt, in which a device attached to one of the ends is fitted or coupled to the other. The word enters Middle English via Old French and the Latin ''buccula'' or "cheek-strap," as for a helmet. Belt buckles and other fixtures are used on a variety of belts, including Cingulum militare, cingula, Baldric#Roman balteus, baltea, baldrics and later waist-belts. Types Belt buckles go back at least to the Iron Age and a gold "great buckle" was among the items interred at Sutton Hoo. Primarily decorative "shield on tongue" buckles were common Anglo-Saxon grave goods at this time, elaborately decorated on the "shield" portion and associated only with men. One such buckle, found in a 7th-century grave at Finglesham Anglo-Saxon cemetery, Finglesham, Kent during excavations by Sonia Chadwick Hawkes in 1964 bears the image of a Nudity in combat, naked warrior standing between two spears ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |