|
Normal Score
The term normal score is used with two different meanings in statistics. One of them relates to creating a single value which can be treated as if it had arisen from a standard normal distribution (zero mean, unit variance). The second one relates to assigning alternative values to data points within a dataset, with the broad intention of creating data values than can be interpreted as being approximations for values that might have been observed had the data arisen from a standard normal distribution. The first meaning is as an alternative name for the standard score or z score, where values are standardised by subtracting the sample or estimated mean and dividing by the sample or other estimate of the standard deviation. Particularly in applications where the name "normal score" is used, there is usually a presumption that the value can be referred to a table of standard normal probabilities as a means of providing a significance test of some hypothesis, such as a difference in me ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Statistics
Statistics (from German: '' Statistik'', "description of a state, a country") is the discipline that concerns the collection, organization, analysis, interpretation, and presentation of data. In applying statistics to a scientific, industrial, or social problem, it is conventional to begin with a statistical population or a statistical model to be studied. Populations can be diverse groups of people or objects such as "all people living in a country" or "every atom composing a crystal". Statistics deals with every aspect of data, including the planning of data collection in terms of the design of surveys and experiments.Dodge, Y. (2006) ''The Oxford Dictionary of Statistical Terms'', Oxford University Press. When census data cannot be collected, statisticians collect data by developing specific experiment designs and survey samples. Representative sampling assures that inferences and conclusions can reasonably extend from the sample to the population as a whole. An ex ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
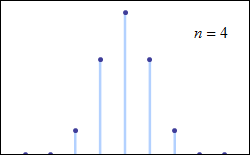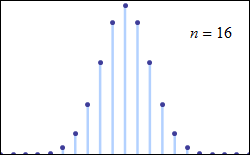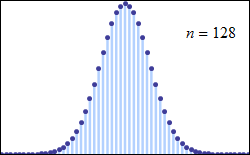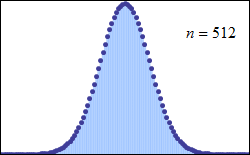
Standard Normal Distribution
In statistics, a normal distribution or Gaussian distribution is a type of continuous probability distribution for a real-valued random variable. The general form of its probability density function is : f(x) = \frac e^ The parameter \mu is the mean or expectation of the distribution (and also its median and mode), while the parameter \sigma is its standard deviation. The variance of the distribution is \sigma^2. A random variable with a Gaussian distribution is said to be normally distributed, and is called a normal deviate. Normal distributions are important in statistics and are often used in the natural and social sciences to represent real-valued random variables whose distributions are not known. Their importance is partly due to the central limit theorem. It states that, under some conditions, the average of many samples (observations) of a random variable with finite mean and variance is itself a random variable—whose distribution converges to a normal dist ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Standard Score
In statistics, the standard score is the number of standard deviations by which the value of a raw score (i.e., an observed value or data point) is above or below the mean value of what is being observed or measured. Raw scores above the mean have positive standard scores, while those below the mean have negative standard scores. It is calculated by subtracting the population mean from an individual raw score and then dividing the difference by the population standard deviation. This process of converting a raw score into a standard score is called standardizing or normalizing (however, "normalizing" can refer to many types of ratios; see normalization for more). Standard scores are most commonly called ''z''-scores; the two terms may be used interchangeably, as they are in this article. Other equivalent terms in use include z-values, normal scores, standardized variables and pull in high energy physics. Computing a z-score requires knowledge of the mean and standard ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Z Score
In statistics, the standard score is the number of standard deviations by which the value of a raw score (i.e., an observed value or data point) is above or below the mean value of what is being observed or measured. Raw scores above the mean have positive standard scores, while those below the mean have negative standard scores. It is calculated by subtracting the population mean from an individual raw score and then dividing the difference by the population standard deviation. This process of converting a raw score into a standard score is called standardizing or normalizing (however, "normalizing" can refer to many types of ratios; see normalization for more). Standard scores are most commonly called ''z''-scores; the two terms may be used interchangeably, as they are in this article. Other equivalent terms in use include z-values, normal scores, standardized variables and pull in high energy physics. Computing a z-score requires knowledge of the mean and standard de ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Significance Test
A statistical hypothesis test is a method of statistical inference used to decide whether the data at hand sufficiently support a particular hypothesis. Hypothesis testing allows us to make probabilistic statements about population parameters. History Early use While hypothesis testing was popularized early in the 20th century, early forms were used in the 1700s. The first use is credited to John Arbuthnot (1710), followed by Pierre-Simon Laplace (1770s), in analyzing the human sex ratio at birth; see . Modern origins and early controversy Modern significance testing is largely the product of Karl Pearson ( ''p''-value, Pearson's chi-squared test), William Sealy Gosset (Student's t-distribution), and Ronald Fisher ("null hypothesis", analysis of variance, "significance test"), while hypothesis testing was developed by Jerzy Neyman and Egon Pearson (son of Karl). Ronald Fisher began his life in statistics as a Bayesian (Zabell 1992), but Fisher soon grew disenchanted with the ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Ranking
A ranking is a relationship between a set of items such that, for any two items, the first is either "ranked higher than", "ranked lower than" or "ranked equal to" the second. In mathematics, this is known as a weak order or total preorder of objects. It is not necessarily a total order of objects because two different objects can have the same ranking. The rankings themselves are totally ordered. For example, materials are totally preordered by hardness, while degrees of hardness are totally ordered. If two items are the same in rank it is considered a tie. By reducing detailed measures to a sequence of ordinal numbers, rankings make it possible to evaluate complex information according to certain criteria. Thus, for example, an Internet search engine may rank the pages it finds according to an estimation of their relevance, making it possible for the user quickly to select the pages they are likely to want to see. Analysis of data obtained by ranking commonly requires no ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Order Statistic
In statistics, the ''k''th order statistic of a statistical sample is equal to its ''k''th-smallest value. Together with rank statistics, order statistics are among the most fundamental tools in non-parametric statistics and inference. Important special cases of the order statistics are the minimum and maximum value of a sample, and (with some qualifications discussed below) the sample median and other sample quantiles. When using probability theory to analyze order statistics of random samples from a continuous distribution, the cumulative distribution function is used to reduce the analysis to the case of order statistics of the uniform distribution. Notation and examples For example, suppose that four numbers are observed or recorded, resulting in a sample of size 4. If the sample values are :6, 9, 3, 8, the order statistics would be denoted :x_=3,\ \ x_=6,\ \ x_=8,\ \ x_=9,\, where the subscript enclosed in parentheses indicates the th order statistic of the ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Standard Normal Random Variable
In statistics, a normal distribution or Gaussian distribution is a type of continuous probability distribution for a real-valued random variable. The general form of its probability density function is : f(x) = \frac e^ The parameter \mu is the mean or expectation of the distribution (and also its median and mode), while the parameter \sigma is its standard deviation. The variance of the distribution is \sigma^2. A random variable with a Gaussian distribution is said to be normally distributed, and is called a normal deviate. Normal distributions are important in statistics and are often used in the natural and social sciences to represent real-valued random variables whose distributions are not known. Their importance is partly due to the central limit theorem. It states that, under some conditions, the average of many samples (observations) of a random variable with finite mean and variance is itself a random variable—whose distribution converges to a normal distributi ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Rankit
In statistics, rankits of a set of data are the expected values of the order statistics of a sample from the standard normal distribution the same size as the data. They are primarily used in the normal probability plot, a graphical technique for normality testing. Example This is perhaps most readily understood by means of an example. If an i.i.d. sample of six items is taken from a normally distributed population with expected value 0 and variance 1 (the standard normal distribution) and then sorted into increasing order, the expected values of the resulting order statistics are: :−1.2672, −0.6418, −0.2016, 0.2016, 0.6418, 1.2672. Suppose the numbers in a data set are : 65, 75, 16, 22, 43, 40. Then one may sort these and line them up with the corresponding rankits; in order they are : 16, 22, 40, 43, 65, 75, which yields the points: These points are then plotted as the vertical and horiz ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Normalization (statistics)
In statistics and applications of statistics, normalization can have a range of meanings. In the simplest cases, normalization of ratings means adjusting values measured on different scales to a notionally common scale, often prior to averaging. In more complicated cases, normalization may refer to more sophisticated adjustments where the intention is to bring the entire probability distributions of adjusted values into alignment. In the case of normalization of scores in educational assessment, there may be an intention to align distributions to a normal distribution. A different approach to normalization of probability distributions is quantile normalization, where the quantiles of the different measures are brought into alignment. In another usage in statistics, normalization refers to the creation of shifted and scaled versions of statistics, where the intention is that these normalized values allow the comparison of corresponding normalized values for different datasets i ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Normal Probability Plot
The normal probability plot is a graphical technique to identify substantive departures from normality. This includes identifying outliers, skewness, kurtosis, a need for transformations, and mixtures. Normal probability plots are made of raw data, residuals from model fits, and estimated parameters. In a normal probability plot (also called a "normal plot"), the sorted data are plotted vs. values selected to make the resulting image look close to a straight line if the data are approximately normally distributed. Deviations from a straight line suggest departures from normality. The plotting can be manually performed by using a special graph paper, called ''normal probability paper''. With modern computers normal plots are commonly made with software. The normal probability plot is a special case of the Q–Q probability plot for a normal distribution. The theoretical quantiles are generally chosen to approximate either the mean or the median of the corresponding ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Q–Q Plot
In statistics, a Q–Q plot (quantile-quantile plot) is a probability plot, a graphical method for comparing two probability distributions by plotting their '' quantiles'' against each other. A point on the plot corresponds to one of the quantiles of the second distribution (-coordinate) plotted against the same quantile of the first distribution (-coordinate). This defines a parametric curve where the parameter is the index of the quantile interval. If the two distributions being compared are similar, the points in the Q–Q plot will approximately lie on the identity line . If the distributions are linearly related, the points in the Q–Q plot will approximately lie on a line, but not necessarily on the line . Q–Q plots can also be used as a graphical means of estimating parameters in a location-scale family of distributions. A Q–Q plot is used to compare the shapes of distributions, providing a graphical view of how properties such as location, scale, and skewn ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |