|
Median
The median of a set of numbers is the value separating the higher half from the lower half of a Sample (statistics), data sample, a statistical population, population, or a probability distribution. For a data set, it may be thought of as the “middle" value. The basic feature of the median in describing data compared to the Arithmetic mean, mean (often simply described as the "average") is that it is not Skewness, skewed by a small proportion of extremely large or small values, and therefore provides a better representation of the center. Median income, for example, may be a better way to describe the center of the income distribution because increases in the largest incomes alone have no effect on the median. For this reason, the median is of central importance in robust statistics. Median is a 2-quantile; it is the value that partitions a set into two equal parts. Finite set of numbers The median of a finite list of numbers is the "middle" number, when those numbers are liste ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Mode (statistics)
In statistics, the mode is the value that appears most often in a set of data values. If is a discrete random variable, the mode is the value at which the probability mass function takes its maximum value (i.e., ). In other words, it is the value that is most likely to be sampled. Like the statistical mean and median, the mode is a way of expressing, in a (usually) single number, important information about a random variable or a population (statistics), population. The numerical value of the mode is the same as that of the mean and median in a normal distribution, and it may be very different in highly skewed distributions. The mode is not necessarily unique in a given discrete distribution since the probability mass function may take the same maximum value at several points , , etc. The most extreme case occurs in Uniform distribution (discrete), uniform distributions, where all values occur equally frequently. A mode of a continuous probability distribution is often conside ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Median Income
The median income is the income amount that divides a population into two groups, half having an income above that amount, and half having an income below that amount. It may differ from the mean (or average) income. Both of these are ways of understanding income distribution. Median income can be calculated by household income, by personal income, or for specific demographic groups. When taxes and mandatory contributions are subtracted from income, the result is called net or disposable income. The measurement of income from individuals and households, which is necessary to produce statistics such as the median, can pose challenges and yield results inconsistent with aggregate national accounts data. For example, an academic study on the Census income data claims that when correcting for underreporting, U.S. median gross household income was 15% higher in 2010 (table 3). Median equivalised disposable income (OECD) See also * Disposable household and per capita income *I ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Average
In colloquial, ordinary language, an average is a single number or value that best represents a set of data. The type of average taken as most typically representative of a list of numbers is the arithmetic mean the sum of the numbers divided by how many numbers are in the list. For example, the mean or average of the numbers 2, 3, 4, 7, and 9 (summing to 25) is 5. Depending on the context, the most representative statistics, statistic to be taken as the average might be another measure of central tendency, such as the mid-range, median, Mode (statistics), mode or geometric mean. For example, the average income, personal income is often given as the median the number below which are 50% of personal incomes and above which are 50% of personal incomes because the mean would be higher by including personal incomes from a few billionaires. General properties If all numbers in a list are the same number, then their average is also equal to this number. This property is shared by each o ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Quartile
In statistics, quartiles are a type of quantiles which divide the number of data points into four parts, or ''quarters'', of more-or-less equal size. The data must be ordered from smallest to largest to compute quartiles; as such, quartiles are a form of order statistic. The three quartiles, resulting in four data divisions, are as follows: * The first quartile (''Q''1) is defined as the 25th percentile where lowest 25% data is below this point. It is also known as the ''lower'' quartile. * The second quartile (''Q''2) is the median of a data set; thus 50% of the data lies below this point. * The third quartile (''Q''3) is the 75th percentile where lowest 75% data is below this point. It is known as the ''upper'' quartile, as 75% of the data lies below this point. Along with the minimum and maximum of the data (which are also quartiles), the three quartiles described above provide a five-number summary of the data. This summary is important in statistics because it provides infor ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Geometric Median
In geometry, the geometric median of a discrete point set in a Euclidean space is the point minimizing the sum of distances to the sample points. This generalizes the median, which has the property of minimizing the sum of distances or absolute differences for one-dimensional data. It is also known as the spatial median, Euclidean minisum point, Torricelli point, or 1-median. It provides a measure of central tendency in higher dimensions and it is a standard problem in facility location, i.e., locating a facility to minimize the cost of transportation. The geometric median is an important estimator of location in statistics, because it minimizes the sum of the ''L''2 distances of the samples. It is to be compared to the mean, which minimizes the sum of the ''squared'' ''L''2 distances; and to the coordinate-wise median which minimizes the sum of the ''L''1 distances. The more general ''k''-median problem asks for the location of ''k'' cluster centers minimizing the sum o ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
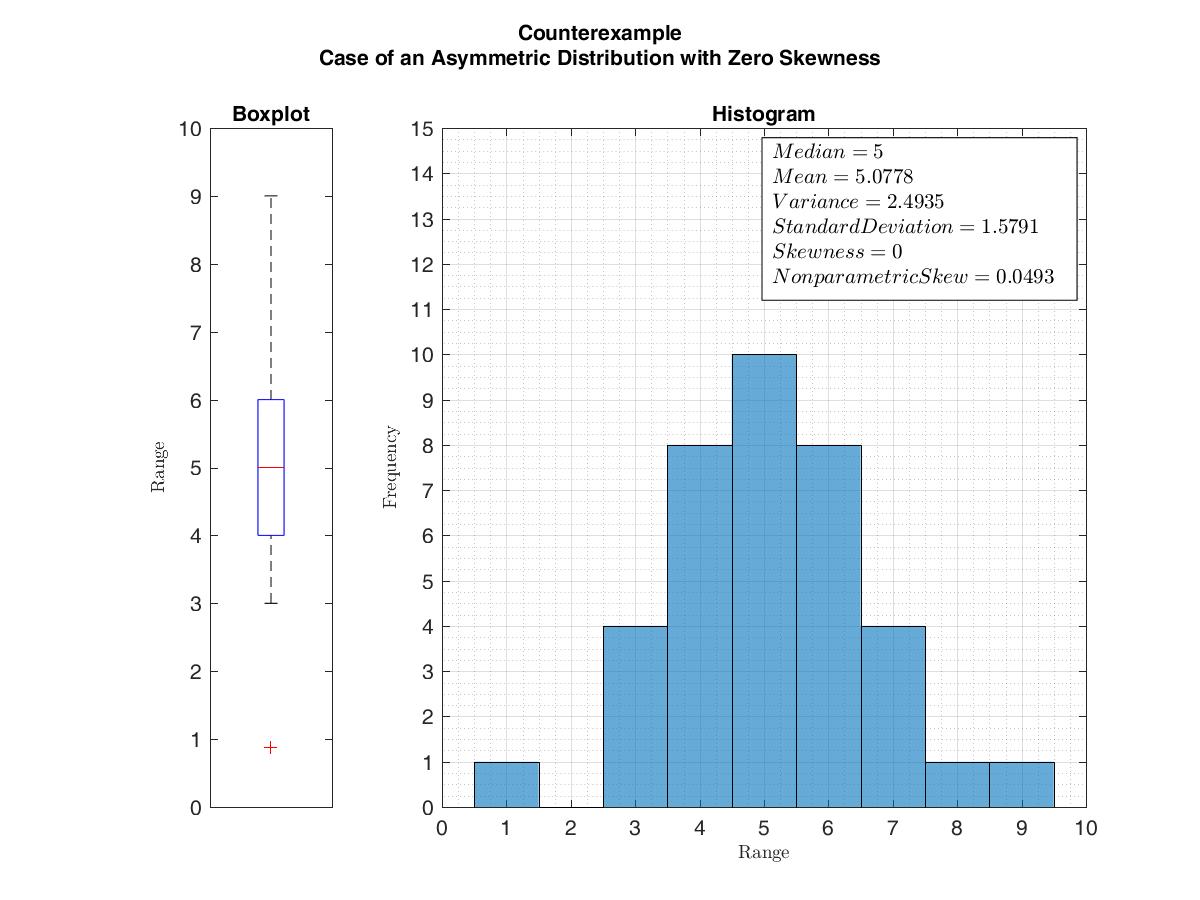
Skewness
In probability theory and statistics, skewness is a measure of the asymmetry of the probability distribution of a real-valued random variable about its mean. The skewness value can be positive, zero, negative, or undefined. For a unimodal distribution (a distribution with a single peak), negative skew commonly indicates that the ''tail'' is on the left side of the distribution, and positive skew indicates that the tail is on the right. In cases where one tail is long but the other tail is fat, skewness does not obey a simple rule. For example, a zero value in skewness means that the tails on both sides of the mean balance out overall; this is the case for a symmetric distribution but can also be true for an asymmetric distribution where one tail is long and thin, and the other is short but fat. Thus, the judgement on the symmetry of a given distribution by using only its skewness is risky; the distribution shape must be taken into account. Introduction Consider the two d ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Arithmetic Mean
In mathematics and statistics, the arithmetic mean ( ), arithmetic average, or just the ''mean'' or ''average'' is the sum of a collection of numbers divided by the count of numbers in the collection. The collection is often a set of results from an experiment, an observational study, or a Survey (statistics), survey. The term "arithmetic mean" is preferred in some contexts in mathematics and statistics because it helps to distinguish it from other types of means, such as geometric mean, geometric and harmonic mean, harmonic. Arithmetic means are also frequently used in economics, anthropology, history, and almost every other academic field to some extent. For example, per capita income is the arithmetic average of the income of a nation's Human population, population. While the arithmetic mean is often used to report central tendency, central tendencies, it is not a robust statistic: it is greatly influenced by outliers (Value (mathematics), values much larger or smaller than ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Skewness
In probability theory and statistics, skewness is a measure of the asymmetry of the probability distribution of a real-valued random variable about its mean. The skewness value can be positive, zero, negative, or undefined. For a unimodal distribution (a distribution with a single peak), negative skew commonly indicates that the ''tail'' is on the left side of the distribution, and positive skew indicates that the tail is on the right. In cases where one tail is long but the other tail is fat, skewness does not obey a simple rule. For example, a zero value in skewness means that the tails on both sides of the mean balance out overall; this is the case for a symmetric distribution but can also be true for an asymmetric distribution where one tail is long and thin, and the other is short but fat. Thus, the judgement on the symmetry of a given distribution by using only its skewness is risky; the distribution shape must be taken into account. Introduction Consider the two d ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Arithmetic Mean
In mathematics and statistics, the arithmetic mean ( ), arithmetic average, or just the ''mean'' or ''average'' is the sum of a collection of numbers divided by the count of numbers in the collection. The collection is often a set of results from an experiment, an observational study, or a Survey (statistics), survey. The term "arithmetic mean" is preferred in some contexts in mathematics and statistics because it helps to distinguish it from other types of means, such as geometric mean, geometric and harmonic mean, harmonic. Arithmetic means are also frequently used in economics, anthropology, history, and almost every other academic field to some extent. For example, per capita income is the arithmetic average of the income of a nation's Human population, population. While the arithmetic mean is often used to report central tendency, central tendencies, it is not a robust statistic: it is greatly influenced by outliers (Value (mathematics), values much larger or smaller than ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Trimmed Estimator
In statistics, a trimmed estimator is an estimator derived from another estimator by excluding some of the extreme values, a process called truncation. This is generally done to obtain a more robust statistic, and the extreme values are considered outliers. Trimmed estimators also often have higher efficiency for mixture distributions, and heavy-tailed distributions than the corresponding untrimmed estimator, at the cost of lower efficiency for other distributions, such as the normal distribution. Given an estimator, the x% trimmed version is obtained by discarding the x% lowest or highest observations or on both end: it is a statistic on the ''middle'' of the data. For instance, the 5% trimmed mean is obtained by taking the mean of the 5% to 95% range. In some cases a trimmed estimator discards a fixed number of points (such as maximum and minimum) instead of a percentage. Examples The median is the most trimmed statistic (nominally 50%), as it discards all but the most centra ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Central Tendency
In statistics, a central tendency (or measure of central tendency) is a central or typical value for a probability distribution.Weisberg H.F (1992) ''Central Tendency and Variability'', Sage University Paper Series on Quantitative Applications in the Social Sciences, p.2 Colloquially, measures of central tendency are often called '' averages.'' The term ''central tendency'' dates from the late 1920s. The most common measures of central tendency are the arithmetic mean, the median, and the mode. A middle tendency can be calculated for either a finite set of values or for a theoretical distribution, such as the normal distribution. Occasionally authors use central tendency to denote "the tendency of quantitative data to cluster around some central value."Upton, G.; Cook, I. (2008) ''Oxford Dictionary of Statistics'', OUP (entry for "central tendency")Dodge, Y. (2003) ''The Oxford Dictionary of Statistical Terms'', OUP for International Statistical Institute. (entry for "cent ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |