|
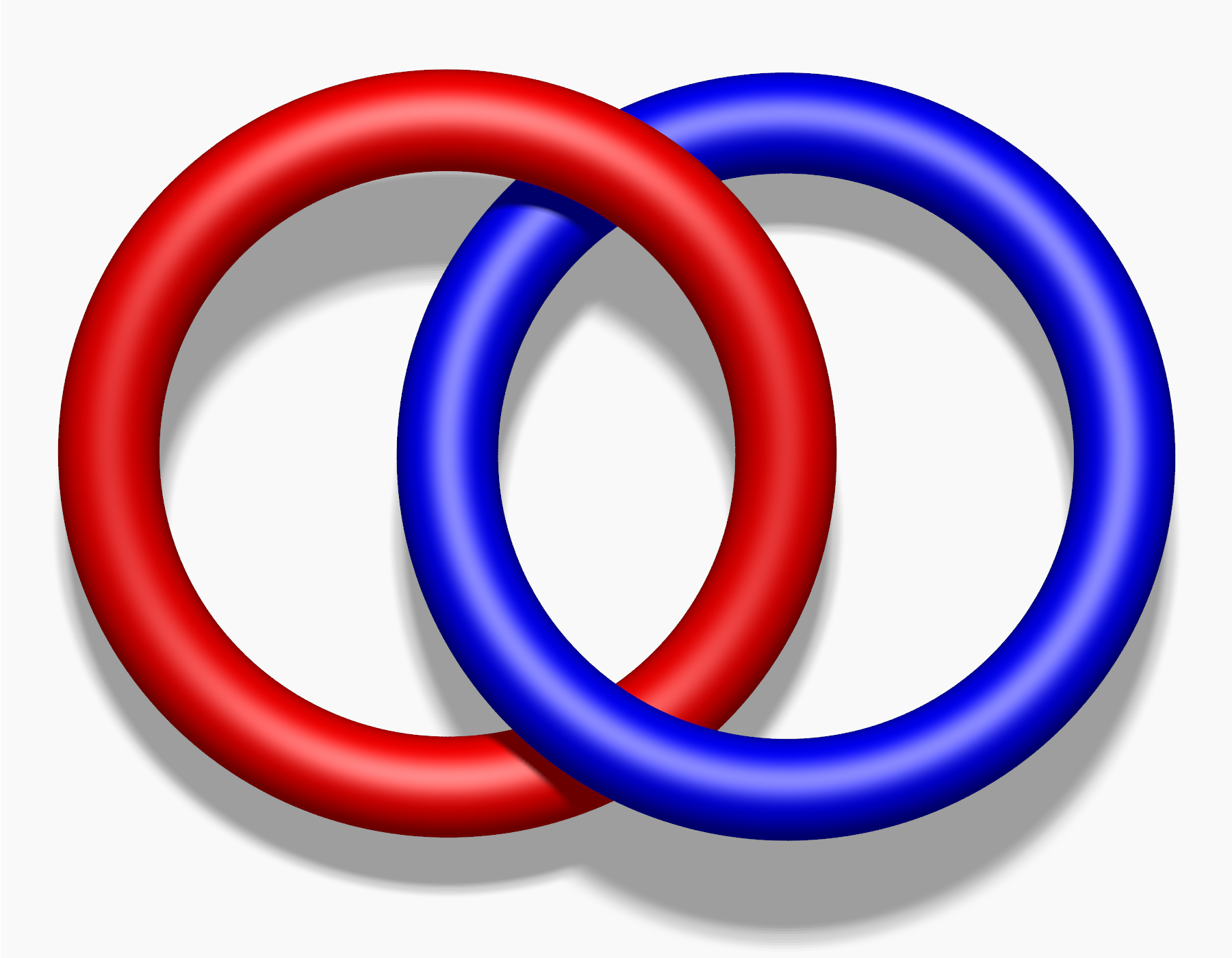
Hopfion
A hopfion is a topological soliton. It is a stable three-dimensional localised configuration of a three-component field \vec=(n_x,n_y,n_z) with a knotted topological structure. They are the three-dimensional counterparts of skyrmions, which exhibit similar topological properties in 2D. The soliton is mobile and stable: i.e. it is protected from a decay by an energy barrier. It can be deformed but always conserves an integer Hopf topological invariant. It is named after the German mathematician, Heinz Hopf. A model that supports hopfions was proposed as follows H= (\partial )^2 + (\epsilon_\cdot\partial_i \times \partial_j)^2 The terms of higher-order derivatives are required to stabilize the hopfions. Stable hopfions were predicted within various physical platforms, including Yang-Mills theory, superconductivity and magnetism. Experimental observation Hopfions have been observed experimentally in Ir/Co/Pt multilayers using X-ray magnetic circular dichroism and in the polar ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Hopfion
A hopfion is a topological soliton. It is a stable three-dimensional localised configuration of a three-component field \vec=(n_x,n_y,n_z) with a knotted topological structure. They are the three-dimensional counterparts of skyrmions, which exhibit similar topological properties in 2D. The soliton is mobile and stable: i.e. it is protected from a decay by an energy barrier. It can be deformed but always conserves an integer Hopf topological invariant. It is named after the German mathematician, Heinz Hopf. A model that supports hopfions was proposed as follows H= (\partial )^2 + (\epsilon_\cdot\partial_i \times \partial_j)^2 The terms of higher-order derivatives are required to stabilize the hopfions. Stable hopfions were predicted within various physical platforms, including Yang-Mills theory, superconductivity and magnetism. Experimental observation Hopfions have been observed experimentally in Ir/Co/Pt multilayers using X-ray magnetic circular dichroism and in the polar ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Skyrmions
In particle theory, the skyrmion () is a topologically stable field configuration of a certain class of non-linear sigma models. It was originally proposed as a model of the nucleon by (and named after) Tony Skyrme in 1961. As a topological soliton in the pion field, it has the remarkable property of being able to model, with reasonable accuracy, multiple low-energy properties of the nucleon, simply by fixing the nucleon radius. It has since found application in solid-state physics, as well as having ties to certain areas of string theory. Skyrmions as topological objects are important in solid-state physics, especially in the emerging technology of spintronics. A two-dimensional magnetic skyrmion, as a topological object, is formed, e.g., from a 3D effective-spin "hedgehog" (in the field of micromagnetics: out of a so-called " Bloch point" singularity of homotopy degree +1) by a stereographic projection, whereby the positive north-pole spin is mapped onto a far-off edge circle ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Heinz Hopf
Heinz Hopf (19 November 1894 – 3 June 1971) was a German mathematician who worked on the fields of topology and geometry. Early life and education Hopf was born in Gräbschen, Germany (now , part of Wrocław, Poland), the son of Elizabeth (née Kirchner) and Wilhelm Hopf. His father was born Jewish and converted to Protestantism a year after Heinz was born; his mother was from a Protestant family. Hopf attended Karl Mittelhaus higher boys' school from 1901 to 1904, and then entered the König-Wilhelm- Gymnasium in Breslau. He showed mathematical talent from an early age. In 1913 he entered the Silesian Friedrich Wilhelm University where he attended lectures by Ernst Steinitz, Adolf Kneser, Max Dehn, Erhard Schmidt, and Rudolf Sturm. When World War I broke out in 1914, Hopf eagerly enlisted. He was wounded twice and received the iron cross (first class) in 1918. After the war Hopf continued his mathematical education in Heidelberg (winter 1919/20 and summer 1920) and Berl ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Skyrmion
In particle theory, the skyrmion () is a topologically stable field configuration of a certain class of non-linear sigma models. It was originally proposed as a model of the nucleon by (and named after) Tony Skyrme in 1961. As a topological soliton in the pion field, it has the remarkable property of being able to model, with reasonable accuracy, multiple low-energy properties of the nucleon, simply by fixing the nucleon radius. It has since found application in solid-state physics, as well as having ties to certain areas of string theory. Skyrmions as topological objects are important in solid-state physics, especially in the emerging technology of spintronics. A two-dimensional magnetic skyrmion, as a topological object, is formed, e.g., from a 3D effective-spin "hedgehog" (in the field of micromagnetics: out of a so-called " Bloch point" singularity of homotopy degree +1) by a stereographic projection, whereby the positive north-pole spin is mapped onto a far-off edge circle of ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Topological Defect
A topological soliton occurs when two adjoining structures or spaces are in some way "out of phase" with each other in ways that make a seamless transition between them impossible. One of the simplest and most commonplace examples of a topological soliton occurs in old-fashioned coiled telephone handset cords, which are usually coiled clockwise. Years of picking up the handset can end up coiling parts of the cord in the opposite counterclockwise direction, and when this happens there will be a distinctive larger loop that separates the two directions of coiling. This odd looking transition loop, which is neither clockwise nor counterclockwise, is an excellent example of a topological soliton. No matter how complex the context, anything that qualifies as a topological soliton must at some level exhibit this same simple issue of reconciliation seen in the twisted phone cord example. Topological solitons arise with ease when creating the crystalline semiconductors used in modern elect ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Activation Energy
In chemistry and physics, activation energy is the minimum amount of energy that must be provided for compounds to result in a chemical reaction. The activation energy (''E''a) of a reaction is measured in joules per mole (J/mol), kilojoules per mole (kJ/mol) or kilocalories per mole (kcal/mol). Activation energy can be thought of as the magnitude of the potential barrier (sometimes called the energy barrier) separating minima of the potential energy surface pertaining to the initial and final thermodynamic state. For a chemical reaction to proceed at a reasonable rate, the temperature of the system should be high enough such that there exists an appreciable number of molecules with translational energy equal to or greater than the activation energy. The term "activation energy" was introduced in 1889 by the Swedish scientist Svante Arrhenius. Other uses Although less commonly used, activation energy also applies to nuclear reactions and various other physical phenomena. Te ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
X-ray Magnetic Circular Dichroism
X-ray magnetic circular dichroism (XMCD) is a difference spectrum of two X-ray absorption spectra (XAS) taken in a magnetic field, one taken with left circularly polarized light, and one with right circularly polarized light. By closely analyzing the difference in the XMCD spectrum, information can be obtained on the magnetic properties of the atom, such as its spin and orbital magnetic moment. Using XMCD magnetic moments below 10−5 µB can be observed. In the case of transition metals such as iron, cobalt, and nickel, the absorption spectra for XMCD are usually measured at the L-edge. This corresponds to the process in the iron case: with iron, a 2p electron is excited to a 3d state by an X-ray of about 700 eV. Because the 3d electron states are the origin of the magnetic properties of the elements, the spectra contain information on the magnetic properties. In rare-earth elements usually, the M4,5-edges are measured, corresponding to electron excitations from a 3d sta ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Hopf Fibration
In the mathematical field of differential topology, the Hopf fibration (also known as the Hopf bundle or Hopf map) describes a 3-sphere (a hypersphere in four-dimensional space) in terms of circles and an ordinary sphere. Discovered by Heinz Hopf in 1931, it is an influential early example of a fiber bundle. Technically, Hopf found a many-to-one continuous function (or "map") from the -sphere onto the -sphere such that each distinct ''point'' of the -sphere is mapped from a distinct great circle of the -sphere . Thus the -sphere is composed of fibers, where each fiber is a circle — one for each point of the -sphere. This fiber bundle structure is denoted :S^1 \hookrightarrow S^3 \xrightarrow S^2, meaning that the fiber space (a circle) is embedded in the total space (the -sphere), and (Hopf's map) projects onto the base space (the ordinary -sphere). The Hopf fibration, like any fiber bundle, has the important property that it is locally a product space. However it is ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Magnetism
Magnetism is the class of physical attributes that are mediated by a magnetic field, which refers to the capacity to induce attractive and repulsive phenomena in other entities. Electric currents and the magnetic moments of elementary particles give rise to a magnetic field, which acts on other currents and magnetic moments. Magnetism is one aspect of the combined phenomena of electromagnetism. The most familiar effects occur in ferromagnetic materials, which are strongly attracted by magnetic fields and can be magnetized to become permanent magnets, producing magnetic fields themselves. Demagnetizing a magnet is also possible. Only a few substances are ferromagnetic; the most common ones are iron, cobalt, and nickel and their alloys. The rare-earth metals neodymium and samarium are less common examples. The prefix ' refers to iron because permanent magnetism was first observed in lodestone, a form of natural iron ore called magnetite, Fe3O4. All substances exhibit some type of ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |