|
Heinz Hopf
Heinz Hopf (19 November 1894 – 3 June 1971) was a German mathematician who worked on the fields of topology and geometry. Early life and education Hopf was born in Gräbschen, Germany (now , part of Wrocław, Poland), the son of Elizabeth (née Kirchner) and Wilhelm Hopf. His father was born Jewish and converted to Protestantism a year after Heinz was born; his mother was from a Protestant family. Hopf attended Karl Mittelhaus higher boys' school from 1901 to 1904, and then entered the König-Wilhelm- Gymnasium in Breslau. He showed mathematical talent from an early age. In 1913 he entered the Silesian Friedrich Wilhelm University where he attended lectures by Ernst Steinitz, Adolf Kneser, Max Dehn, Erhard Schmidt, and Rudolf Sturm. When World War I broke out in 1914, Hopf eagerly enlisted. He was wounded twice and received the iron cross (first class) in 1918. After the war Hopf continued his mathematical education in Heidelberg (winter 1919/20 and summer 1920) and Berl ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Province Of Silesia
The Province of Silesia (german: Provinz Schlesien; pl, Prowincja Śląska; szl, Prowincyjŏ Ślōnskŏ) was a province of Prussia from 1815 to 1919. The Silesia region was part of the Prussian realm since 1740 and established as an official province in 1815, then became part of the German Empire in 1871. In 1919, as part of the Free State of Prussia within Weimar Germany, Silesia was divided into the provinces of Upper Silesia and Lower Silesia. Silesia was reunified briefly from 1 April 1938 to 27 January 1941 as a province of Nazi Germany before being divided back into Upper Silesia and Lower Silesia. Breslau (present-day Wrocław, Poland) was the provincial capital. Geography The territory on both sides of the Oder river formed the southeastern part of the Prussian kingdom. It comprised the bulk of the former Bohemian crown land of Upper and Lower Silesia as well as the adjacent County of Kladsko, which the Prussian King Frederick the Great had all conquered from the A ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Ernst Specker
Ernst Paul Specker (11 February 1920, Zurich – 10 December 2011, Zurich) was a Swiss mathematician. Much of his most influential work was on Quine's New Foundations, a set theory with a universal set, but he is most famous for the Kochen–Specker theorem in quantum mechanics, showing that certain types of hidden variable theories are impossible. He also proved the ordinal partition relation ω2 → (ω2,3)2, thereby solving a problem of Erdős. Specker received his Ph.D. in 1949 from ETH Zurich, where he remained throughout his professional career. See also * Specker sequence * Baer-Specker group References External links Biography at the University of St. Andrews (Aien aristeuein) , motto_lang = grc , mottoeng = Ever to ExcelorEver to be the Best , established = , type = Public research university Ancient university , endowment ... Ernst Specker (1920-2011) Martin Fürer, Janu ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Hopf Surface
In complex geometry, a Hopf surface is a compact complex surface obtained as a quotient of the complex vector space (with zero deleted) \Complex^2\setminus \ by a free action of a discrete group. If this group is the integers the Hopf surface is called primary, otherwise it is called secondary. (Some authors use the term "Hopf surface" to mean "primary Hopf surface".) The first example was found by , with the discrete group isomorphic to the integers, with a generator acting on \Complex^2 by multiplication by 2; this was the first example of a compact complex surface with no Kähler metric. Higher-dimensional analogues of Hopf surfaces are called Hopf manifolds. Invariants Hopf surfaces are surfaces of class VII and in particular all have Kodaira dimension -\infty, and all their plurigenera vanish. The geometric genus is 0. The fundamental group has a normal central infinite cyclic subgroup of finite index. The Hodge diamond is In particular the first Betti number is 1 and the s ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Hopf Manifold
In complex geometry, a Hopf manifold is obtained as a quotient of the complex vector space (with zero deleted) (^n\backslash 0) by a free action of the group \Gamma \cong of integers, with the generator \gamma of \Gamma acting by holomorphic contractions. Here, a ''holomorphic contraction'' is a map \gamma:\; ^n \to ^n such that a sufficiently big iteration \;\gamma^N maps any given compact subset of ^n onto an arbitrarily small neighbourhood of 0. Two-dimensional Hopf manifolds are called Hopf surfaces. Examples In a typical situation, \Gamma is generated by a linear contraction, usually a diagonal matrix q\cdot Id, with q\in a complex number, 0<, q, <1. Such manifold is called ''a classical Hopf manifold''. Properties A Hopf manifold isdiffeomorphic
In mathem ...
[...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
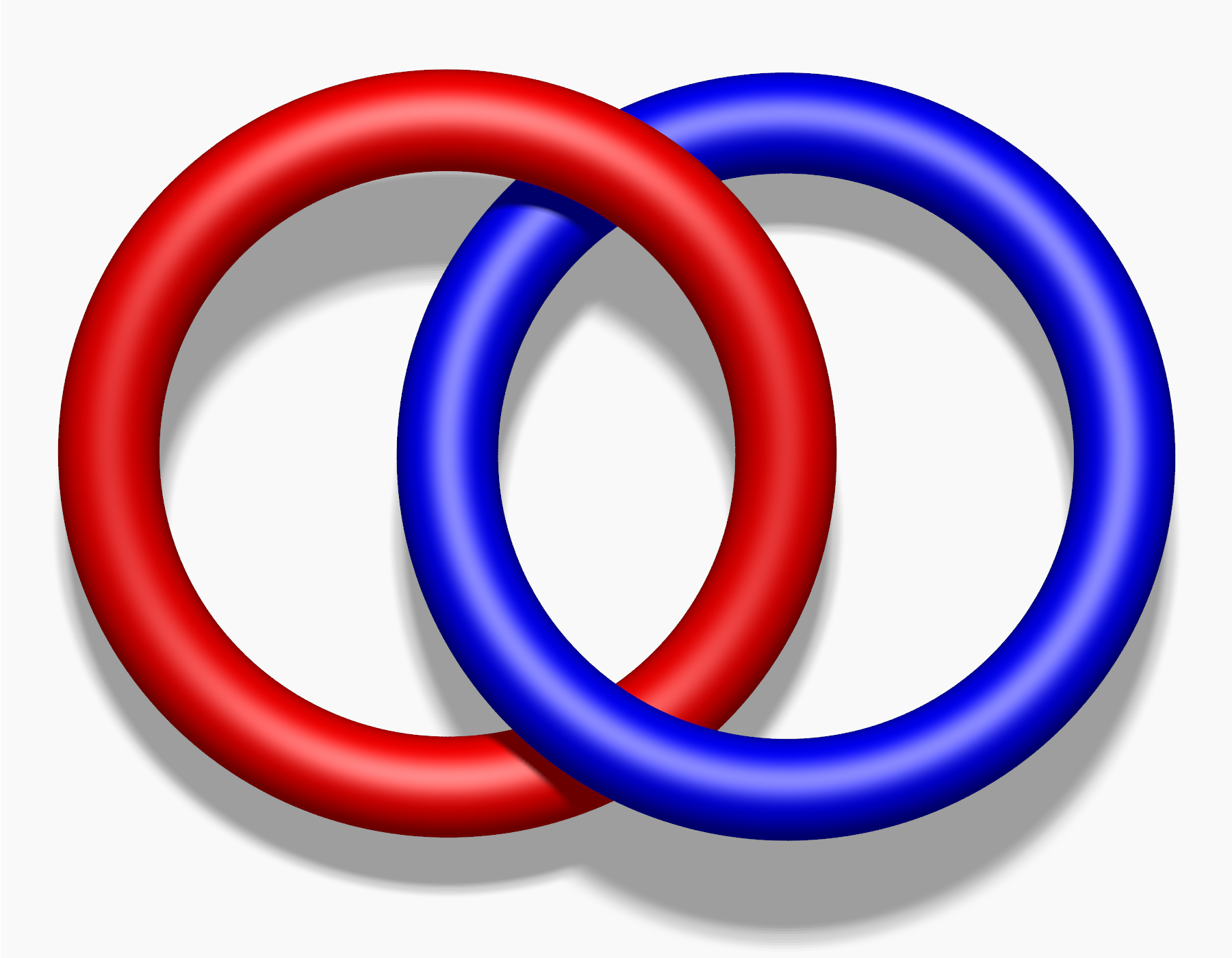
Hopf Link
In mathematical knot theory, the Hopf link is the simplest nontrivial link with more than one component. It consists of two circles linked together exactly once, and is named after Heinz Hopf. Geometric realization A concrete model consists of two unit circles in perpendicular planes, each passing through the center of the other.. See in particulap. 77 This model minimizes the ropelength of the link and until 2002 the Hopf link was the only link whose ropelength was known. The convex hull of these two circles forms a shape called an oloid. Properties Depending on the relative orientations of the two components the linking number of the Hopf link is ±1. The Hopf link is a (2,2)-torus link with the braid word :\sigma_1^2.\, The knot complement of the Hopf link is R × ''S''1 × ''S''1, the cylinder over a torus. This space has a locally Euclidean geometry, so the Hopf link is not a hyperbolic link. The knot group of the Hopf link (the fund ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Hopf Invariant
In mathematics, in particular in algebraic topology, the Hopf invariant is a homotopy invariant of certain maps between n-spheres. __TOC__ Motivation In 1931 Heinz Hopf used Clifford parallels to construct the ''Hopf map'' :\eta\colon S^3 \to S^2, and proved that \eta is essential, i.e., not homotopic to the constant map, by using the fact that the linking number of the circles :\eta^(x),\eta^(y) \subset S^3 is equal to 1, for any x \neq y \in S^2. It was later shown that the homotopy group \pi_3(S^2) is the infinite cyclic group generated by \eta. In 1951, Jean-Pierre Serre proved that the rational homotopy groups :\pi_i(S^n) \otimes \mathbb for an odd-dimensional sphere (n odd) are zero unless i is equal to 0 or ''n''. However, for an even-dimensional sphere (''n'' even), there is one more bit of infinite cyclic homotopy in degree 2n-1. Definition Let \phi \colon S^ \to S^n be a continuous map (assume n>1). Then we can form the cell complex : C_\phi = S^n \cup_\phi ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Hopf Fibration
In the mathematical field of differential topology, the Hopf fibration (also known as the Hopf bundle or Hopf map) describes a 3-sphere (a hypersphere in four-dimensional space) in terms of circles and an ordinary sphere. Discovered by Heinz Hopf in 1931, it is an influential early example of a fiber bundle. Technically, Hopf found a many-to-one continuous function (or "map") from the -sphere onto the -sphere such that each distinct ''point'' of the -sphere is mapped from a distinct great circle of the -sphere . Thus the -sphere is composed of fibers, where each fiber is a circle — one for each point of the -sphere. This fiber bundle structure is denoted :S^1 \hookrightarrow S^3 \xrightarrow S^2, meaning that the fiber space (a circle) is embedded in the total space (the -sphere), and (Hopf's map) projects onto the base space (the ordinary -sphere). The Hopf fibration, like any fiber bundle, has the important property that it is locally a product space. However it is ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Hopf Conjecture
In mathematics, Hopf conjecture may refer to one of several conjectural statements from differential geometry and topology attributed to Heinz Hopf. Positively or negatively curved Riemannian manifolds The Hopf conjecture is an open problem in global Riemannian geometry. It goes back to questions of Heinz Hopf from 1931. A modern formulation is: : ''A compact, even-dimensional Riemannian manifold with positive sectional curvature has positive Euler characteristic. A compact, (2''d'')-dimensional Riemannian manifold with negative sectional curvature has Euler characteristic of sign (-1)^d.'' For surfaces, these statements follow from the Gauss–Bonnet theorem. For four-dimensional manifolds, this follows from the finiteness of the fundamental group and Poincaré duality and Euler–Poincaré formula equating for 4-manifolds the Euler characteristic with b_0-b_1+b_2-b_3+b_4 and Synge's theorem, assuring that the orientation cover is simply connected, so that the Betti numbers ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Hopf Algebra
Hopf is a German surname. Notable people with the surname include: *Eberhard Hopf (1902–1983), Austrian mathematician *Hans Hopf (1916–1993), German tenor *Heinz Hopf (1894–1971), German mathematician *Heinz Hopf (actor) (1934–2001), Swedish actor *Ludwig Hopf (1884–1939), German physicist *Maria Hopf Maria Hopf (13 September 1913 – 24 August 2008) was a pioneering archaeobotanist, based at the RGZM, Mainz. Career Hopf studied botany from 1941–44, receiving her doctorate in 1947 on the subject of soil microbes. She then worked in phyto ... (1914-2008), German botanist and archaeologist {{surname, Hopf German-language surnames ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
H-space
In mathematics, an H-space is a homotopy-theoretic version of a generalization of the notion of topological group, in which the axioms on associativity and inverses are removed. Definition An H-space consists of a topological space , together with an element of and a continuous map , such that and the maps and are both homotopic to the identity map through maps sending to . This may be thought of as a pointed topological space together with a continuous multiplication for which the basepoint is an identity element up to basepoint-preserving homotopy. One says that a topological space is an H-space if there exists and such that the triple is an H-space as in the above definition. Alternatively, an H-space may be defined without requiring homotopies to fix the basepoint , or by requiring to be an exact identity, without any consideration of homotopy. In the case of a CW complex, all three of these definitions are in fact equivalent. Examples and properties The standard ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Almost Complex Manifold
In mathematics, an almost complex manifold is a smooth manifold equipped with a smooth linear complex structure on each tangent space. Every complex manifold is an almost complex manifold, but there are almost complex manifolds that are not complex manifolds. Almost complex structures have important applications in symplectic geometry. The concept is due to Charles Ehresmann and Heinz Hopf in the 1940s. Formal definition Let ''M'' be a smooth manifold. An almost complex structure ''J'' on ''M'' is a linear complex structure (that is, a linear map which squares to −1) on each tangent space of the manifold, which varies smoothly on the manifold. In other words, we have a smooth tensor field ''J'' of degree such that J^2=-1 when regarded as a vector bundle isomorphism J\colon TM\to TM on the tangent bundle. A manifold equipped with an almost complex structure is called an almost complex manifold. If ''M'' admits an almost complex structure, it must be even-dimensional. This ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Yoshie Katsurada
Yoshie Katsurada ( ja, 桂田 芳枝, 3 September 1911 – 10 May 1980) was a Japanese mathematician specializing in differential geometry. She became the first Japanese woman to earn a doctorate in mathematics, in 1950, and the first to obtain an imperial university professorship in mathematics, in 1967. Life Katsurada was born in Akaigawa, Hokkaido on 3 September 1911, a daughter of an elementary school principal. In high school in Otaru, she took special instruction in mathematics from a boys' mathematics instructor. Graduating from high school in 1929, she began auditing classes at the , a predecessor to the Tokyo University of Science, in 1931. She began working as an administrative assistant in the Hokkaido University Department of Mathematics in 1936. In 1938 she began study in mathematics at Tokyo Woman's Christian University, withdrawing in 1940 to transfer to Hokkaido University. She graduated from Hokkaido University in 1942, and in the same year became an assistant pr ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
