|
Gioseffe Zarlino
Gioseffo Zarlino (31 January or 22 March 1517 – 4 February 1590) was an Italian music theorist and composer of the Renaissance. He made a large contribution to the theory of counterpoint as well as to musical tuning. Life and career Zarlino was born in Chioggia, near Venice. His early education was with the Franciscans, and he later joined the order himself. In 1536 he was a singer at Chioggia Cathedral, and by 1539 he not only became a deacon, but also principal organist. In 1540 he was ordained, and in 1541 went to Venice to study with the famous contrapuntist and ''maestro di cappella'' of Saint Mark's, Adrian Willaert. In 1565, on the resignation of Cipriano de Rore, Zarlino took over the post of ''maestro di cappella'' of St. Mark's, one of the most prestigious musical positions in Italy, and held it until his death. While ''maestro di cappella'' he taught some of the principal figures of the Venetian school of composers, including Claudio Merulo, Girolamo Dirut ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Gioseffo Zarlino
Gioseffo Zarlino (31 January or 22 March 1517 – 4 February 1590) was an Italian music theorist and composer of the Renaissance. He made a large contribution to the theory of counterpoint as well as to musical tuning. Life and career Zarlino was born in Chioggia, near Venice. His early education was with the Franciscans, and he later joined the order himself. In 1536 he was a singer at Chioggia Cathedral, and by 1539 he not only became a deacon, but also principal organist. In 1540 he was ordained, and in 1541 went to Venice to study with the famous contrapuntist and ''maestro di cappella'' of Saint Mark's, Adrian Willaert. In 1565, on the resignation of Cipriano de Rore, Zarlino took over the post of ''maestro di cappella'' of St. Mark's, one of the most prestigious musical positions in Italy, and held it until his death. While ''maestro di cappella'' he taught some of the principal figures of the Venetian school of composers, including Claudio Merulo, Girolamo Diruta, a ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Vincenzo Galilei
Vincenzo Galilei (born 3 April 1520, Santa Maria a Monte, Italy died 2 July 1591, Florence, Italy) was an Italian lutenist, composer, and music theorist. His children included the astronomer and physicist Galileo Galilei and the lute virtuoso and composer Michelagnolo Galilei. Vincenzo was a figure in the musical life of the late Renaissance and contributed significantly to the musical revolution which demarcates the beginning of the Baroque era. In his study of pitch and string tension, Galilei produced perhaps the first non-linear mathematical description of a natural phenomenon known to history. Some credit him with directing the activity of his son away from pure, abstract mathematics and towards experimentation using mathematical quantitative description of the results, a direction of importance for the history of physics and natural science. Biography He was born in 1520 in Santa Maria a Monte, Pisa, Tuscany and began studying the lute at an early age. His mother was from ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Francesco Franceschi
Francesco Franceschi (died c. 1599) was a printer in the Italian Renaissance. His roots were in Siena, though the bulk of his work was done in Venice. Franceschi was known for the high quality of his engravings, which were done using metal plates rather than wooden, a common inexpensive alternative in the period. Evelyn Tribble describes in detail his 1565 edition of Ludovico Ariosto's '' Orlando Furioso'', which was influential for some English publishers, and which is heavily and ornately illustrated, including an engraving before every canto and an engraved frame surrounding the argument (Tribble 88). Franceschi was also known for printing music. According to the ''New Grove'', he printed the works of Gioseffo Zarlino Gioseffo Zarlino (31 January or 22 March 1517 – 4 February 1590) was an Italian music theorist and composer of the Renaissance. He made a large contribution to the theory of counterpoint as well as to musical tuning. Life and career Zarlin ... and ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Ptolemy's Intense Diatonic Scale
Ptolemy's intense diatonic scale, also known as the Ptolemaic sequence, justly tuned major scale, Ptolemy's tense diatonic scale, or the syntonous (or syntonic) diatonic scale, is a tuning for the diatonic scale proposed by Ptolemy, and corresponding with modern 5-limit just intonation.Chisholm, Hugh (1911). The Encyclopædia Britannica', Vol.28, p. 961. The Encyclopædia Britannica Company. This tuning was declared by Zarlino to be the only tuning that could be reasonably sung, it was also supported by Giuseppe Tartini, and is equivalent to Indian Gandhar tuning which features exactly the same intervals. It is produced through a tetrachord consisting of a greater tone (9:8), lesser tone (10:9), and just diatonic semitone (16:15). This is called Ptolemy's intense diatonic tetrachord (or "tense"), as opposed to Ptolemy's soft diatonic tetrachord (or "relaxed"), which is formed by 21:20, 10:9 and 8:7 intervals. Structure The structure of the intense diatonic scale is shown ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Boethius
Anicius Manlius Severinus Boethius, commonly known as Boethius (; Latin: ''Boetius''; 480 – 524 AD), was a Roman senator, consul, ''magister officiorum'', historian, and philosopher of the Early Middle Ages. He was a central figure in the translation of the Greek classics into Latin, a precursor to the Scholastic movement, and, along with Cassiodorus, one of the two leading Christian scholars of the 6th century. The local cult of Boethius in the Diocese of Pavia was sanctioned by the Sacred Congregation of Rites in 1883, confirming the diocese's custom of honouring him on the 23 October. Boethius was born in Rome a few years after the collapse of the Western Roman Empire. A member of the Anicii family, he was orphaned following the family's sudden decline and was raised by Quintus Aurelius Memmius Symmachus, a later consul. After mastering both Latin and Greek in his youth, Boethius rose to prominence as a statesman during the Ostrogothic Kingdom: becoming a senator by a ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Pythagoreanism
Pythagoreanism originated in the 6th century BC, based on and around the teachings and beliefs held by Pythagoras and his followers, the Pythagoreans. Pythagoras established the first Pythagorean community in the Ancient Greece, ancient Greek colony of Crotone, Kroton, in modern Calabria (Italy). Early Pythagorean communities spread throughout Magna Graecia. Pythagoras' death and disputes about his teachings led to the development of two philosophical traditions within Pythagoreanism. The ''akousmatikoi'' were superseded in the 4th century BC as a significant mendicant school of philosophy by the Cynicism (philosophy), Cynics. The ''mathēmatikoi'' philosophers were absorbed into the Platonic Academy, Platonic school in the 4th century BC. Following political instability in Magna Graecia, some Pythagorean philosophers fled to mainland Greece while others regrouped in Rhegium. By about 400 BC the majority of Pythagorean philosophers had left Italy. Pythagorean ideas exercised a m ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
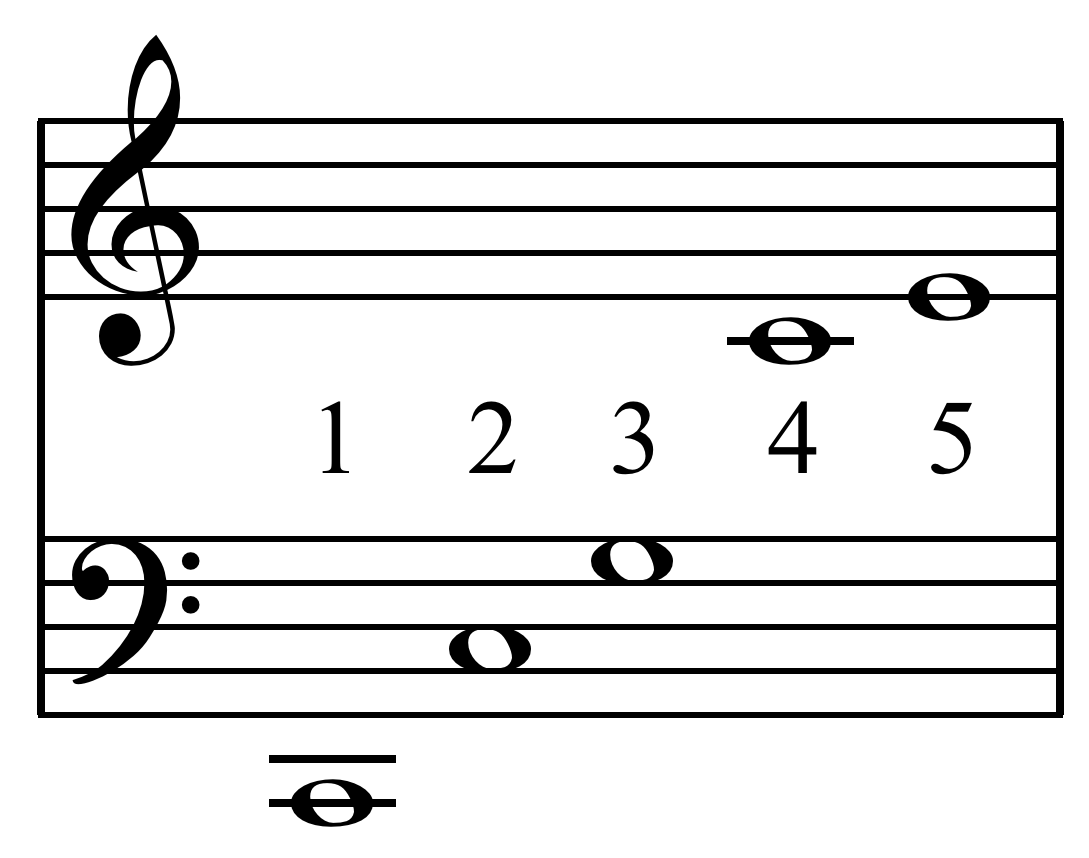
Just Intonation
In music, just intonation or pure intonation is the tuning of musical intervals Interval may refer to: Mathematics and physics * Interval (mathematics), a range of numbers ** Partially ordered set#Intervals, its generalization from numbers to arbitrary partially ordered sets * A statistical level of measurement * Interval e ... as whole number ratios (such as 3:2 or 4:3) of Frequency, frequencies. An interval (music), interval tuned in this way is said to be pure, and is called a just interval. Just intervals (and chords created by combining them) consist of tones from a single harmonic series (music), harmonic series of an implied fundamental frequency, fundamental. For example, in the diagram, if the notes G3 and C4 (labelled 3 and 4) are tuned as members of the harmonic series of the lowest C, their frequencies will be 3 and 4 times the fundamental frequency. The interval ratio between C4 and G3 is therefore 4:3, a just fourth (music), fourth. In Western musical practice ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Interval (music)
In music theory, an interval is a difference in pitch between two sounds. An interval may be described as horizontal, linear, or melodic if it refers to successively sounding tones, such as two adjacent pitches in a melody, and vertical or harmonic if it pertains to simultaneously sounding tones, such as in a chord. In Western music, intervals are most commonly differences between notes of a diatonic scale. Intervals between successive notes of a scale are also known as scale steps. The smallest of these intervals is a semitone. Intervals smaller than a semitone are called microtones. They can be formed using the notes of various kinds of non-diatonic scales. Some of the very smallest ones are called commas, and describe small discrepancies, observed in some tuning systems, between enharmonically equivalent notes such as C and D. Intervals can be arbitrarily small, and even imperceptible to the human ear. In physical terms, an interval is the ratio between two sonic freq ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Triad (music)
In music, a triad is a set of three notes (or "pitch classes") that can be stacked vertically in thirds.Ronald Pen, ''Introduction to Music'' (New York: McGraw-Hill, 1992): 81. . "A triad is a set of notes consisting of three notes built on successive intervals of a third. A triad can be constructed upon any note by adding alternating notes drawn from the scale.... In each case the note that forms the foundation pitch is called the ''root'', the middle tone of the triad is designated the ''third'' (because it is separated by the interval of a third from the root), and the top tone is referred to as the ''fifth'' (because it is a fifth away from the root)." Triads are the most common chords in Western music. When stacked in thirds, notes produce triads. The triad's members, from lowest-pitched tone to highest, are called: * the root **Note: Inversion does not change the root. (The third or fifth can be the lowest note.) * the third – its interval above the root being a minor thi ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Equal Temperament
An equal temperament is a musical temperament or tuning system, which approximates just intervals by dividing an octave (or other interval) into equal steps. This means the ratio of the frequencies of any adjacent pair of notes is the same, which gives an equal perceived step size as pitch is perceived roughly as the logarithm of frequency. In classical music and Western music in general, the most common tuning system since the 18th century has been twelve-tone equal temperament (also known as 12 equal temperament, 12-TET or 12-ET; informally abbreviated to twelve equal), which divides the octave into 12 parts, all of which are equal on a logarithmic scale, with a ratio equal to the 12th root of 2 ( ≈ 1.05946). That resulting smallest interval, the width of an octave, is called a semitone or half step. In Western countries the term ''equal temperament'', without qualification, generally means 12-TET. In modern times, 12-TET is usually tuned relative to a standard pitch of ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Meantone Temperament
Meantone temperament is a musical temperament, that is a tuning system, obtained by narrowing the fifths so that their ratio is slightly less than 3:2 (making them ''narrower'' than a perfect fifth), in order to push the thirds closer to pure. Meantone temperaments are constructed the same way as Pythagorean tuning, as a stack of equal fifths, but it is a ''temperament'' in that the fifths are not pure. Notable meantone temperaments Equal temperament, obtained by making all semitones the same size, each equal to one-twelfth of an octave (with ratio the 12th root of 2 to one (:1), narrows the fifths by about 2 cents or 1/12 of a Pythagorean comma, and produces thirds that are only slightly better than in Pythagorean tuning. Equal temperament is roughly the same as 1/11 comma meantone tuning. Quarter-comma meantone, which tempers the fifths by 1/4 of a syntonic comma, is the best known type of meantone temperament, and the term ''meantone temperament'' is often used to refer to ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Pietro Aron
Pietro Aron, also known as Pietro (or Piero) Aaron (c. 1480 – after 1545), was an Italian music theorist and composer. He was born in Florence and probably died in Bergamo (other sources state Florence or Venice). Biography Very little is known about Aron's early life but at least one source claims he may have been Jewish. He was educated in Italy. Aron was a self-taught musician. He claimed in his ''Toscanello in musica'' (1523) that he had been friends with Obrecht, Josquin, and Heinrich Isaac in Florence. If true, the time frame would have been most likely in 1487. Between 1515 and 1522, he was Church Cantor at the Cathedral of Imola. In 1516 he became a priest there. In February 1523 Aron went to Venice and became cantor of Rimini Cathedral, where he worked for Sebastiano Michiel, who was Grand Prior of the Knights of St. John of Jerusalem. In 1525, he was "maestro di casa" in a Venetian house. In 1536, after the death of Michiel, he joined a monastery in Bergamo where ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
