|
Diocles (mathematician)
Diocles ( grc-gre, Διοκλῆς; c. 240 BC – c. 180 BC) was a Greek mathematician and geometer. Life and work Although little is known about the life of Diocles, it is known that he was a contemporary of Apollonius and that he flourished sometime around the end of the 3rd century BC and the beginning of the 2nd century BC. Diocles is thought to be the first person to prove the focal property of the parabola. His name is associated with the geometric curve called the Cissoid of Diocles, which was used by Diocles to solve the problem of doubling the cube. The curve was alluded to by Proclus in his commentary on Euclid and attributed to Diocles by Geminus as early as the beginning of the 1st century. Fragments of a work by Diocles entitled ''On burning mirrors'' were preserved by Eutocius in his commentary of Archimedes' ''On the Sphere and the Cylinder'' and also survived in an Arabic translation of the lost Greek original titled ''Kitāb Dhiyūqlīs fī l-marāyā l-muḥ ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Hellenistic Greece
Hellenistic Greece is the historical period of the country following Classical Greece, between the death of Alexander the Great in 323 BC and the annexation of the classical Greek Achaean League heartlands by the Roman Republic. This culminated at the Battle of Corinth in 146 BC, a crushing Roman victory in the Peloponnese that led to the destruction of Corinth and ushered in the period of Roman Greece. Hellenistic Greece's definitive end was with the Battle of Actium in 31 BC, when the future emperor Augustus defeated Greek Ptolemaic queen Cleopatra VII and Mark Antony, the next year taking over Alexandria, the last great center of Hellenistic Greece. The Hellenistic period began with the wars of the Diadochi, armed contests among the former generals of Alexander the Great to carve up his empire in Europe, Asia, and North Africa. The wars lasted until 275 BC, witnessing the fall of both the Argead and Antipatrid dynasties of Macedonia in favor of the Antigonid dynasty. Th ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Archimedes
Archimedes of Syracuse (;; ) was a Greek mathematician, physicist, engineer, astronomer, and inventor from the ancient city of Syracuse in Sicily. Although few details of his life are known, he is regarded as one of the leading scientists in classical antiquity. Considered the greatest mathematician of ancient history, and one of the greatest of all time,* * * * * * * * * * Archimedes anticipated modern calculus and analysis by applying the concept of the infinitely small and the method of exhaustion to derive and rigorously prove a range of geometrical theorems. These include the area of a circle, the surface area and volume of a sphere, the area of an ellipse, the area under a parabola, the volume of a segment of a paraboloid of revolution, the volume of a segment of a hyperboloid of revolution, and the area of a spiral. Heath, Thomas L. 1897. ''Works of Archimedes''. Archimedes' other mathematical achievements include deriving an approximation of pi, defining and in ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Year Of Death Unknown
A year or annus is the orbital period of a planetary body, for example, the Earth, moving in its orbit around the Sun. Due to the Earth's axial tilt, the course of a year sees the passing of the seasons, marked by change in weather, the hours of daylight, and, consequently, vegetation and soil fertility. In temperate and subpolar regions around the planet, four seasons are generally recognized: spring, summer, autumn and winter. In tropical and subtropical regions, several geographical sectors do not present defined seasons; but in the seasonal tropics, the annual wet and dry seasons are recognized and tracked. A calendar year is an approximation of the number of days of the Earth's orbital period, as counted in a given calendar. The Gregorian calendar, or modern calendar, presents its calendar year to be either a common year of 365 days or a leap year of 366 days, as do the Julian calendars. For the Gregorian calendar, the average length of the calendar year (the mea ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Year Of Birth Unknown
A year or annus is the orbital period of a planetary body, for example, the Earth, moving in its orbit around the Sun. Due to the Earth's axial tilt, the course of a year sees the passing of the seasons, marked by change in weather, the hours of daylight, and, consequently, vegetation and soil fertility. In temperate and subpolar regions around the planet, four seasons are generally recognized: spring, summer, autumn and winter. In tropical and subtropical regions, several geographical sectors do not present defined seasons; but in the seasonal tropics, the annual wet and dry seasons are recognized and tracked. A calendar year is an approximation of the number of days of the Earth's orbital period, as counted in a given calendar. The Gregorian calendar, or modern calendar, presents its calendar year to be either a common year of 365 days or a leap year of 366 days, as do the Julian calendars. For the Gregorian calendar, the average length of the calendar year ( ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Ancient Greek Geometers
Ancient history is a time period from the beginning of writing and recorded human history to as far as late antiquity. The span of recorded history is roughly 5,000 years, beginning with the Sumerian cuneiform script. Ancient history covers all continents inhabited by humans in the period 3000 BCAD 500. The three-age system periodizes ancient history into the Stone Age, the Bronze Age, and the Iron Age, with recorded history generally considered to begin with the Bronze Age. The start and end of the three ages varies between world regions. In many regions the Bronze Age is generally considered to begin a few centuries prior to 3000 BC, while the end of the Iron Age varies from the early first millennium BC in some regions to the late first millennium AD in others. During the time period of ancient history, the world population was already exponentially increasing due to the Neolithic Revolution, which was in full progress. While in 10,000 BC, the world population stood at ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Burning Glass
A burning glass or burning lens is a large convex lens that can concentrate the sun's rays onto a small area, heating up the area and thus resulting in ignition of the exposed surface. Burning mirrors achieve a similar effect by using reflecting surfaces to focus the light. They were used in 18th-century chemical studies for burning materials in closed glass vessels where the products of combustion could be trapped for analysis. The burning glass was a useful contrivance in the days before electrical ignition was easily achieved. Historical development: from legend to science Burning glass technology has been known since antiquity, as described by Greek and Roman writers who recorded the use of lenses to start fires for various purposes. Pliny the Elder noted the use of glass vases filled with water to create a heat intense enough to ignite clothing, as well as convex lenses that were used to cauterize wounds. Plutarch refers to a burning mirror made of joined triangular metal mir ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Cubic Equation
In algebra, a cubic equation in one variable is an equation of the form :ax^3+bx^2+cx+d=0 in which is nonzero. The solutions of this equation are called roots of the cubic function defined by the left-hand side of the equation. If all of the coefficients , , , and of the cubic equation are real numbers, then it has at least one real root (this is true for all odd-degree polynomial functions). All of the roots of the cubic equation can be found by the following means: * algebraically, that is, they can be expressed by a cubic formula involving the four coefficients, the four basic arithmetic operations and th roots (radicals). (This is also true of quadratic (second-degree) and quartic (fourth-degree) equations, but not of higher-degree equations, by the Abel–Ruffini theorem.) * trigonometrically * numerical approximations of the roots can be found using root-finding algorithms such as Newton's method. The coefficients do not need to be real numbers. Much of what is ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
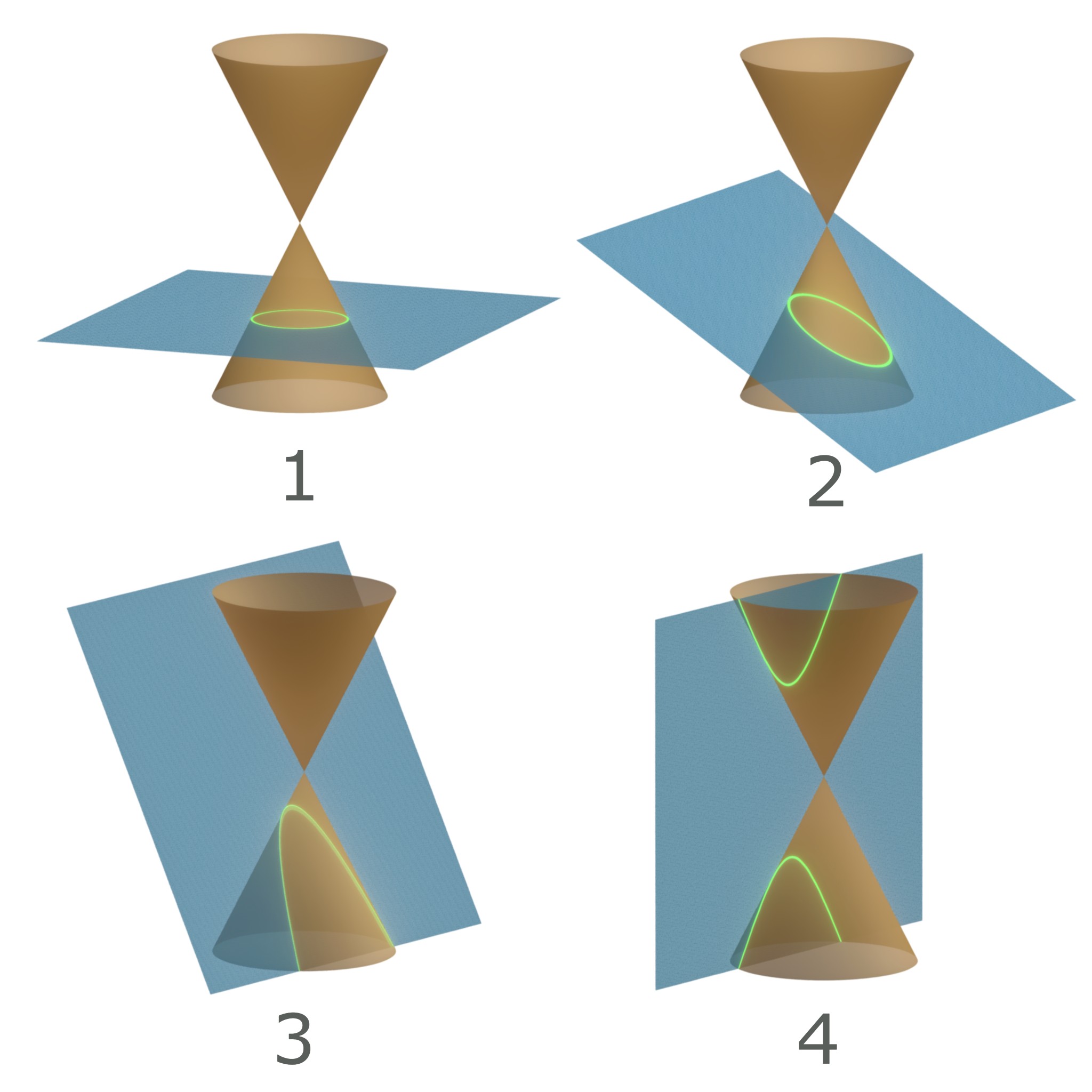
Conic Sections
In mathematics, a conic section, quadratic curve or conic is a curve obtained as the intersection of the surface of a cone with a plane. The three types of conic section are the hyperbola, the parabola, and the ellipse; the circle is a special case of the ellipse, though historically it was sometimes called a fourth type. The ancient Greek mathematicians studied conic sections, culminating around 200 BC with Apollonius of Perga's systematic work on their properties. The conic sections in the Euclidean plane have various distinguishing properties, many of which can be used as alternative definitions. One such property defines a non-circular conic to be the set of those points whose distances to some particular point, called a ''focus'', and some particular line, called a ''directrix'', are in a fixed ratio, called the ''eccentricity''. The type of conic is determined by the value of the eccentricity. In analytic geometry, a conic may be defined as a plane algebraic curve of deg ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Alhazen
Ḥasan Ibn al-Haytham, Latinized as Alhazen (; full name ; ), was a medieval mathematician, astronomer, and physicist of the Islamic Golden Age from present-day Iraq.For the description of his main fields, see e.g. ("He is one of the principal Arab mathematicians and, without any doubt, the best physicist.") , ("Ibn al-Ḥaytam was an eminent eleventh-century Arab optician, geometer, arithmetician, algebraist, astronomer, and engineer."), ("Ibn al-Haytham (d. 1039), known in the West as Alhazan, was a leading Arab mathematician, astronomer, and physicist. His optical compendium, Kitab al-Manazir, is the greatest medieval work on optics.") Referred to as "the father of modern optics", he made significant contributions to the principles of optics and visual perception in particular. His most influential work is titled '' Kitāb al-Manāẓir'' (Arabic: , "Book of Optics"), written during 1011–1021, which survived in a Latin edition. Ibn al-Haytham was an early propo ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Arabic
Arabic (, ' ; , ' or ) is a Semitic languages, Semitic language spoken primarily across the Arab world.Semitic languages: an international handbook / edited by Stefan Weninger; in collaboration with Geoffrey Khan, Michael P. Streck, Janet C. E.Watson; Walter de Gruyter GmbH & Co. KG, Berlin/Boston, 2011. Having emerged in the 1st century, it is named after the Arabs, Arab people; the term "Arab" was initially used to describe those living in the Arabian Peninsula, as perceived by geographers from ancient Greece. Since the 7th century, Arabic has been characterized by diglossia, with an opposition between a standard Prestige (sociolinguistics), prestige language—i.e., Literary Arabic: Modern Standard Arabic (MSA) or Classical Arabic—and diverse vernacular varieties, which serve as First language, mother tongues. Colloquial dialects vary significantly from MSA, impeding mutual intelligibility. MSA is only acquired through formal education and is not spoken natively. It is ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Eutocius
Eutocius of Ascalon (; el, Εὐτόκιος ὁ Ἀσκαλωνίτης; 480s – 520s) was a Palestinian-Greek mathematician who wrote commentaries on several Archimedean treatises and on the Apollonian ''Conics''. Life and work Little is known about the life of Eutocius. He was born in Ascalon, then in Palestina Prima. He lived during the reign of Justinian. Eutocius became head the school of philosophy in Athens following Ammonius and he was succeeded in this position by Olympiodorus, possibly as early as 525. He traveled to the greatest scientific centers of his time, including Alexandria, to conduct research on Archimedes' manuscripts. He wrote commentaries on Apollonius and on Archimedes. The surviving works of Eutocius are: *A Commentary on the first four books of the ''Conics'' of Apollonius. *Commentarieson: **the ''Sphere and Cylinder'' of Archimedes. **the ''Quadrature of the Circle'' of Archimedes (''In Archimedis circuli dimensionem'' in Latin). **the '' ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Mathematician
A mathematician is someone who uses an extensive knowledge of mathematics in their work, typically to solve mathematical problems. Mathematicians are concerned with numbers, data, quantity, structure, space, models, and change. History One of the earliest known mathematicians were Thales of Miletus (c. 624–c.546 BC); he has been hailed as the first true mathematician and the first known individual to whom a mathematical discovery has been attributed. He is credited with the first use of deductive reasoning applied to geometry, by deriving four corollaries to Thales' Theorem. The number of known mathematicians grew when Pythagoras of Samos (c. 582–c. 507 BC) established the Pythagorean School, whose doctrine it was that mathematics ruled the universe and whose motto was "All is number". It was the Pythagoreans who coined the term "mathematics", and with whom the study of mathematics for its own sake begins. The first woman mathematician recorded by history was Hypati ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
_by_Thomas_Degeorge.png "Click for more on -> Archimedes")
