|
Diatonic Set Theory
Diatonic set theory is a subdivision or application of musical set theory which applies the techniques and analysis of discrete mathematics to properties of the diatonic collection such as maximal evenness, Myhill's property, well formedness, the deep scale property, cardinality equals variety, and structure implies multiplicity. The name is something of a misnomer as the concepts involved usually apply much more generally, to any periodically repeating scale. Music theorists working in diatonic set theory include Eytan Agmon, Gerald J. Balzano, Norman Carey, David Clampitt, John Clough, Jay Rahn, and mathematician Jack Douthett. A number of key concepts were first formulated by David Rothenberg (the Rothenberg propriety), who published in the journal ''Mathematical Systems Theory'', and Erv Wilson, working entirely outside of the academic world. See also * Bisector *Diatonic and chromatic *Generic and specific intervals Further reading *Balzano, Gerald, "The Pitch Set as a Leve ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Set Theory (music)
Musical set theory provides concepts for categorizing musical objects and describing their relationships. Howard Hanson first elaborated many of the concepts for analyzing tonal music. Other theorists, such as Allen Forte, further developed the theory for analyzing atonal music, drawing on the twelve-tone theory of Milton Babbitt. The concepts of musical set theory are very general and can be applied to tonal and atonal styles in any equal temperament tuning system, and to some extent more generally than that. One branch of musical set theory deals with collections ( sets and permutations) of pitches and pitch classes (pitch-class set theory), which may be ordered or unordered, and can be related by musical operations such as transposition, melodic inversion, and complementation. Some theorists apply the methods of musical set theory to the analysis of rhythm as well. Mathematical set theory versus musical set theory Although musical set theory is often thought to involve ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Mathematical Systems Theory
Dynamical systems theory is an area of mathematics used to describe the behavior of complex dynamical systems, usually by employing differential equations or difference equations. When differential equations are employed, the theory is called ''continuous dynamical systems''. From a physical point of view, continuous dynamical systems is a generalization of classical mechanics, a generalization where the equations of motion are postulated directly and are not constrained to be Euler–Lagrange equations of a least action principle. When difference equations are employed, the theory is called ''discrete dynamical systems''. When the time variable runs over a set that is discrete over some intervals and continuous over other intervals or is any arbitrary time-set such as a Cantor set, one gets dynamic equations on time scales. Some situations may also be modeled by mixed operators, such as differential-difference equations. This theory deals with the long-term qualitative behavior ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
David Rothenberg
David Rothenberg (born 1962) is a professor of philosophy and music at the New Jersey Institute of Technology, with a special interest in animal sounds as music. He is also a composer and jazz musician whose books and recordings reflect a longtime interest in understanding other species such as singing insects by making music with them. Life and work Rothenberg graduated from Harvard and took his PhD from Boston University. Looking back at his high school years in the 1970s, Rothenberg told Claudia Dreifus of '' The New York Times'', "I was influenced by saxophonist Paul Winter's ''Common Ground'' album, which had his own compositions with whale and bird sounds mixed in. That got me interested in using music to learn more about the natural world." As an undergraduate at Harvard, Rothenberg created his own major to combine music with communication. He traveled in Europe after graduation, playing jazz clarinet. Listening to the recorded song of a hermit thrush, he heard ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
In Theory Only
''In Theory Only'' () was a peer-reviewed academic journal specializing in music theory Music theory is the study of the practices and possibilities of music. ''The Oxford Companion to Music'' describes three interrelated uses of the term "music theory". The first is the "rudiments", that are needed to understand music notation (ke ... and analysis. It began publication in 1975, under the auspices of graduate students in music theory at the University of Michigan School of Music, Theatre & Dance, making it the first of the graduate-student produced theory journals to debut in the U.S. (followed two years later by '' Indiana Theory Review'').David Carson Berry, "Schenkerian Theory in the United States: A Review of Its Establishment and a Survey of Current Research Topics," ''Zeitschrift der Gesellschaft für Musiktheorie'' 2/2–3 (2005), 111 (online version at http://www.gmth.de/zeitschrift/artikel/206.aspx). The journal initially employed the subtitle "Newsletter of the Mich ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Kraig Grady
Kraig Grady (born 1952) is a US-Australian composer/sound artist. He has composed and performed with an ensemble of microtonal instruments of his own design and also worked as a shadow puppeteer, tuning theorist, filmmaker, world music radio DJ and concert promoter. His works feature his own ensembles of acoustic instruments, including metallophones, marimbas, hammered dulcimers and reed organs tuned to microtonal just intonation scales. His compositions include accompaniments for silent films and shadow plays. An important influence in the development of Grady's music was Harry Partch, like Grady, a musician from the Southwest, and a composer of theatrical works in Just Intonation for self-built instruments. Many of his compositions use unusual meters of very extended lengths. Biography Born in Montebello, California in 1952, Grady began composing while still in his teens. After studies with Nicolas Slonimsky, Dean Drummond, Dorrance Stalvey and Byong-Kon Kim, he produced h ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Perspectives Of New Music
''Perspectives of New Music'' (PNM) is a peer-reviewed academic journal specializing in music theory and analysis. It was established in 1962 by Arthur Berger and Benjamin Boretz (who were its initial editors-in-chief). ''Perspectives'' was first published by the Princeton University Press, initially supported by the Fromm Music Foundation.David Carson Berry, "''Journal of Music Theory'' under Allen Forte's Editorship," ''Journal of Music Theory'' 50/1 (2006), 21, n49. The first issue was favorably reviewed in the ''Journal of Music Theory'', which observed that Berger and Boretz had produced "a first issue which sustains such a high quality of interest and cogency among its articles that one suspects the long delay preceding the yet-unborn Spring 1963 issue may reflect a scarcity of material up to their standard". However, as the journal's editorial "perspective" coalesced, Fromm became—in the words of David Gable—disenchanted with the "exclusive viewpoint hatcame to dominate" ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
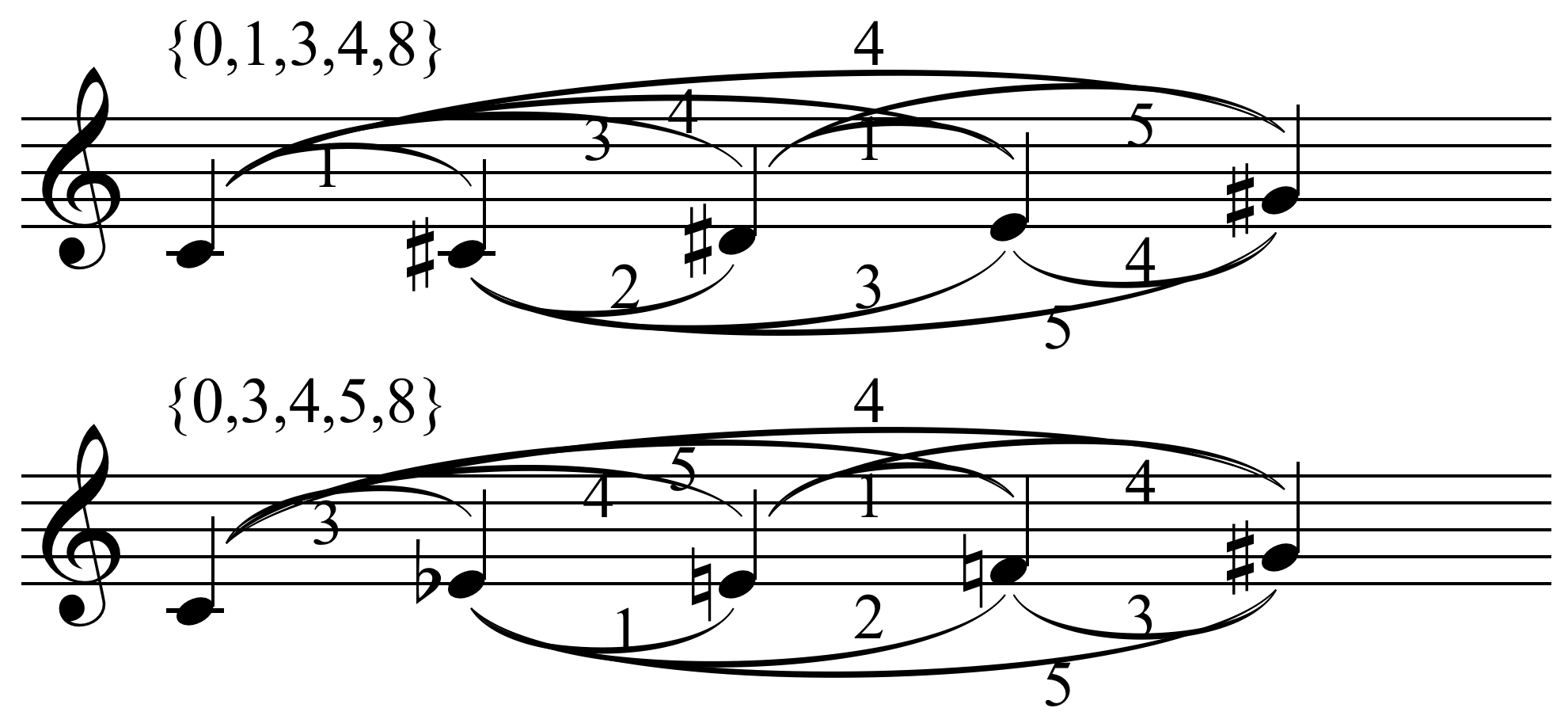
Generic And Specific Intervals
In diatonic set theory a generic interval is the number of scale steps between notes of a collection or scale. The largest generic interval is one less than the number of scale members. (Johnson 2003, p. 26) A specific interval is the clockwise distance between pitch classes on the chromatic circle ( interval class), in other words the number of half steps between notes. The largest specific interval is one less than the number of "chromatic" pitches. In twelve tone equal temperament the largest specific interval is 11. (Johnson 2003, p. 26) In the diatonic collection the generic interval is one less than the corresponding diatonic interval: * Adjacent intervals, seconds, are 1 * Thirds = 2 * Fourths = 3 * Fifths = 4 * Sixths = 5 * Sevenths = 6 The largest generic interval in the diatonic scale being 7 − 1 = 6. Myhill's property Myhill's property is the quality of musical scales or collections with exactly two specific intervals for every generic interva ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Diatonic And Chromatic
Diatonic and chromatic are terms in music theory that are most often used to characterize scales, and are also applied to musical instruments, intervals, chords, notes, musical styles, and kinds of harmony. They are very often used as a pair, especially when applied to contrasting features of the common practice music of the period 1600–1900. These terms may mean different things in different contexts. Very often, ''diatonic'' refers to musical elements derived from the modes and transpositions of the "white note scale" C–D–E–F–G–A–B. In some usages it includes all forms of heptatonic scale that are in common use in Western music (the major, and all forms of the minor). ''Chromatic'' most often refers to structures derived from the twelve-note chromatic scale, which consists of all semitones. Historically, however, it had other senses, referring in Ancient Greek music theory to a particular tuning of the tetrachord, and to a rhythmic notational convention in me ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Bisector (music)
In diatonic set theory, a bisector divides the octave approximately in half (the equal tempered tritone is exactly half the octave) and may be used in place of a generator to derive collections for which structure implies multiplicity is not true such as the ascending melodic minor, harmonic minor, and octatonic scales. Well formed generated collections generators and bisectors coincide, such as the perfect fifth (circle of fifths) in the diatonic collection. The term was introduced by Jay Rahn (1977), who considers any division between one and two thirds as approximately half (major third to minor sixth or 400 to 800 cents) and who applied the term only the equally spaced collections. Clough and Johnson both adapt the term to apply to generic scale steps. Rahn also uses ''aliquant bisector'' for bisectors which may be used to generate every note in a collection, in which case the bisector and the number of notes must be coprime. Bisectors may be used to produce the diatonic, h ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Erv Wilson
Ervin Wilson (June 11, 1928 – December 8, 2016) was a Mexico, Mexican/United States, American (dual citizen) music theory, music theorist. Early life Ervin Wilson was born in a remote area of northwest Chihuahua (state), Chihuahua, Mexico, where he lived until the age of fifteen. His mother taught him to play the reed organ and to read musical notation. He began to compose at an early age, but immediately discovered that some of the sounds he was hearing mentally could not be reproduced by the conventional intervals of the organ. As a teenager he began to read books on Indian music, developing an interest in concepts of raga. While he was in the Air Force in Japan, a chance meeting with a total stranger introduced him to Harmonic, musical harmonics, which changed the course of his life and work. Influenced by the work of Joseph Yasser, Wilson began to think of the musical scale as a living process—like a crystal or plant. He mentored many composers and instrument builders. Wo ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Rothenberg Propriety
In diatonic set theory, Rothenberg propriety is an important concept, lack of contradiction and ambiguity, in the general theory of musical scales which was introduced by David Rothenberg in a seminal series of papers in 1978. The concept was independently discovered in a more restricted context by Gerald Balzano, who termed it ''coherence''. "Rothenberg calls a scale 'strictly proper' if it possesses a generic ordering, 'proper' if it admits ambiguities but no contradictions, and 'improper' if it admits contradictions." A scale is strictly proper if all two step intervals are larger than any one step interval, all three step intervals are larger than any two step interval and so on. For instance with the diatonic scale, the one step intervals are the semitone (1) and tone (2), the two step intervals are the minor (3) and major (4) third, the three step intervals are the fourth (5) and tritone (6), the four step intervals are the fifth (7) and tritone (6), the five step inter ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Musical Analysis
Musical analysis is the study of musical structure in either compositions or performances. According to music theorist Ian Bent, music analysis "is the means of answering directly the question 'How does it work?'". The method employed to answer this question, and indeed exactly what is meant by the question, differs from analyst to analyst, and according to the purpose of the analysis. According to Bent, "its emergence as an approach and method can be traced back to the 1750s. However it existed as a scholarly tool, albeit an auxiliary one, from the Middle Ages onwards." The principle of analysis has been variously criticized, especially by composers, such as Edgard Varèse's claim that, "to explain by means of nalysisis to decompose, to mutilate the spirit of a work". Analyses Some analysts, such as Donald Tovey (whose '' Essays in Musical Analysis'' are among the most accessible musical analyses) have presented their analyses in prose. Others, such as Hans Keller (who devised ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
