|
ฤryabhaแนญฤซya
''Aryabhatiya'' (IAST: ') or ''Aryabhatiyam'' ('), a Indian astronomy, Sanskrit astronomical treatise, is the ''Masterpiece, magnum opus'' and only known surviving work of the 5th century Indian mathematics, Indian mathematician Aryabhata. Philosopher of astronomy Roger Billard estimates that the book was composed around 510 CE based on historical references it mentions. Structure and style Aryabhatiya is written in Sanskrit and divided into four sections; it covers a total of 121 verses describing different moralitus via a mnemonic writing style typical for such works in India (see definitions below): # Gitikapada (13 verses): large units of timeโKalpa (aeon), kalpa, manvantara, and Yuga Cycle, yugaโwhich present a cosmology different from earlier texts such as Lagadha's Vedanga Jyotisha (ca. 1st century BCE). There is also a table of [sine]s (jya), given in a single verse. The duration of the planetary revolutions during a mahayuga is given as 4.32 million years, using the ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Indian Mathematics
Indian mathematics emerged in the Indian subcontinent from 1200 BCE until the end of the 18th century. In the classical period of Indian mathematics (400 CE to 1200 CE), important contributions were made by scholars like Aryabhata, Brahmagupta, Bhaskara II, Varฤhamihira, and Madhava of Sangamagrama, Madhava. The Decimal, decimal number system in use today: "The measure of the genius of Indian civilisation, to which we owe our modern (number) system, is all the greater in that it was the only one in all history to have achieved this triumph. Some cultures succeeded, earlier than the Indian, in discovering one or at best two of the characteristics of this intellectual feat. But none of them managed to bring together into a complete and coherent system the necessary and sufficient conditions for a number-system with the same potential as our own." was first recorded in Indian mathematics. Indian mathematicians made early contributions to the study of the concept of 0 (number), ze ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Aryabhata Numeration
Aryabhata ( ISO 15919, ISO: ) or Aryabhata I (476โ550 Common Era, CE) was the first of the major mathematician-astronomers from the classical age of Indian mathematics and Indian astronomy. His works include the ''ฤryabhaแนญฤซya'' (which mentions that in 3600 ''Kali Yuga'', 499 CE, he was 23 years old) and the ''Arya-siddhanta''. For his explicit mention of the relativity of motion, he also qualifies as a major early physicist. Biography Name While there is a tendency to misspell his name as "Aryabhatta" by analogy with other names having the "bhatta" suffix, his name is properly spelled Aryabhata: every astronomical text spells his name thus, including Brahmagupta's references to him "in more than a hundred places by name". Furthermore, in most instances "Aryabhatta" would not fit the metre either. Time and place of birth Aryabhata mentions in the ''Aryabhatiya'' that he was 23 years old 3,600 years into the ''Kali Yuga'', but this is not to mean that the text was co ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Aryabhata
Aryabhata ( ISO: ) or Aryabhata I (476โ550 CE) was the first of the major mathematician-astronomers from the classical age of Indian mathematics and Indian astronomy. His works include the '' ฤryabhaแนญฤซya'' (which mentions that in 3600 '' Kali Yuga'', 499 CE, he was 23 years old) and the ''Arya- siddhanta''. For his explicit mention of the relativity of motion, he also qualifies as a major early physicist. Biography Name While there is a tendency to misspell his name as "Aryabhatta" by analogy with other names having the " bhatta" suffix, his name is properly spelled Aryabhata: every astronomical text spells his name thus, including Brahmagupta's references to him "in more than a hundred places by name". Furthermore, in most instances "Aryabhatta" would not fit the metre either. Time and place of birth Aryabhata mentions in the ''Aryabhatiya'' that he was 23 years old 3,600 years into the '' Kali Yuga'', but this is not to mean that the text was composed at that ti ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Indian Astronomy
Astronomy has a long history in the Indian subcontinent, stretching from History of India, pre-historic to History of India (1947โpresent), modern times. Some of the earliest roots of Indian astronomy can be dated to the period of Indus Valley civilisation or earlier. Astronomy later developed as a discipline of Vedanga, or one of the "auxiliary disciplines" associated with the study of the Vedas dating 1500 BCE or older. The oldest known text is the ''Vedanga Jyotisha'', dated to 1400โ1200 BCE (with the extant form possibly from 700 to 600 BCE). Indian astronomy was influenced by Greek astronomy beginning in the 4th century BCEHighlights of Astronomy, Volume 11B: As presented at the XXIIIrd General Assembly of the IAU, 1997. Johannes Andersen Springer, 31 January 1999 โ Science โ 616 pages. p. 72/ref>Babylon to Voyager and Beyond: A History of Planetary Astronomy. David Leverington. Cambridge University Press, 29 May 2010 โ Science โ 568 pages. p. 4/ref>Th ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Sanskrit
Sanskrit (; stem form ; nominal singular , ,) is a classical language belonging to the Indo-Aryan languages, Indo-Aryan branch of the Indo-European languages. It arose in northwest South Asia after its predecessor languages had Trans-cultural diffusion, diffused there from the northwest in the late Bronze Age#South Asia, Bronze Age. Sanskrit is the sacred language of Hinduism, the language of classical Hindu philosophy, and of historical texts of Buddhism and Jainism. It was a lingua franca, link language in ancient and medieval South Asia, and upon transmission of Hindu and Buddhist culture to Southeast Asia, East Asia and Central Asia in the early medieval era, it became a language of religion and high culture, and of the political elites in some of these regions. As a result, Sanskrit had a lasting effect on the languages of South Asia, Southeast Asia and East Asia, especially in their formal and learned vocabularies. Sanskrit generally connotes several Indo-Aryan languages# ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Kuแนญแนญaka
Kuแนญแนญaka is an algorithm for finding integer solutions of linear Diophantine equations. A linear Diophantine equation is an equation of the form ''ax'' + ''by'' = ''c'' where ''x'' and ''y'' are unknown quantities and ''a'', ''b'', and ''c'' are known quantities with integer values. The algorithm was originally invented by the Indian astronomer-mathematician ฤryabhaแนญa (476โ550 CE) and is described very briefly in his ฤryabhaแนญฤซya. ฤryabhaแนญa did not give the algorithm the name ''Kuแนญแนญaka'', and his description of the method was mostly obscure and incomprehensible. It was Bhฤskara I (c. 600 โ c. 680) who gave a detailed description of the algorithm with several examples from astronomy in his ''ฤryabhatiyabhฤแนฃya'', who gave the algorithm the name ''Kuแนญแนญaka''. In Sanskrit, the word Kuแนญแนญaka means ''pulverization'' (reducing to powder), and it indicates the nature of the algorithm. The algorithm in essence is a process where the coefficients in a given lin ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Description Of Kuttaka In Aryabhatiya
Description is any type of communication that aims to make vivid a place, object, person, group, or other physical entity. It is one of four rhetorical modes (also known as ''modes of discourse''), along with exposition, argumentation, and narration. Fiction writing Fiction writing specifically has modes such as action, exposition, description, dialogue, summary, and transition. Author Peter Selgin refers to ''methods'', including action, dialogue, thoughts, summary, scenes, and description. Description is the mode for transmitting a mental image of the particulars of a story. Together with dialogue, narration, exposition, and summarization, it is one of the most widely recognized of the fiction-writing modes. As stated in ''Writing from A to Z'', edited by Kirk Polking, it is more than the amassing of details; it is bringing something to life by carefully choosing and arranging words and phrases to produce the desired effect. Purple prose A purple patch is an over-written pa ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
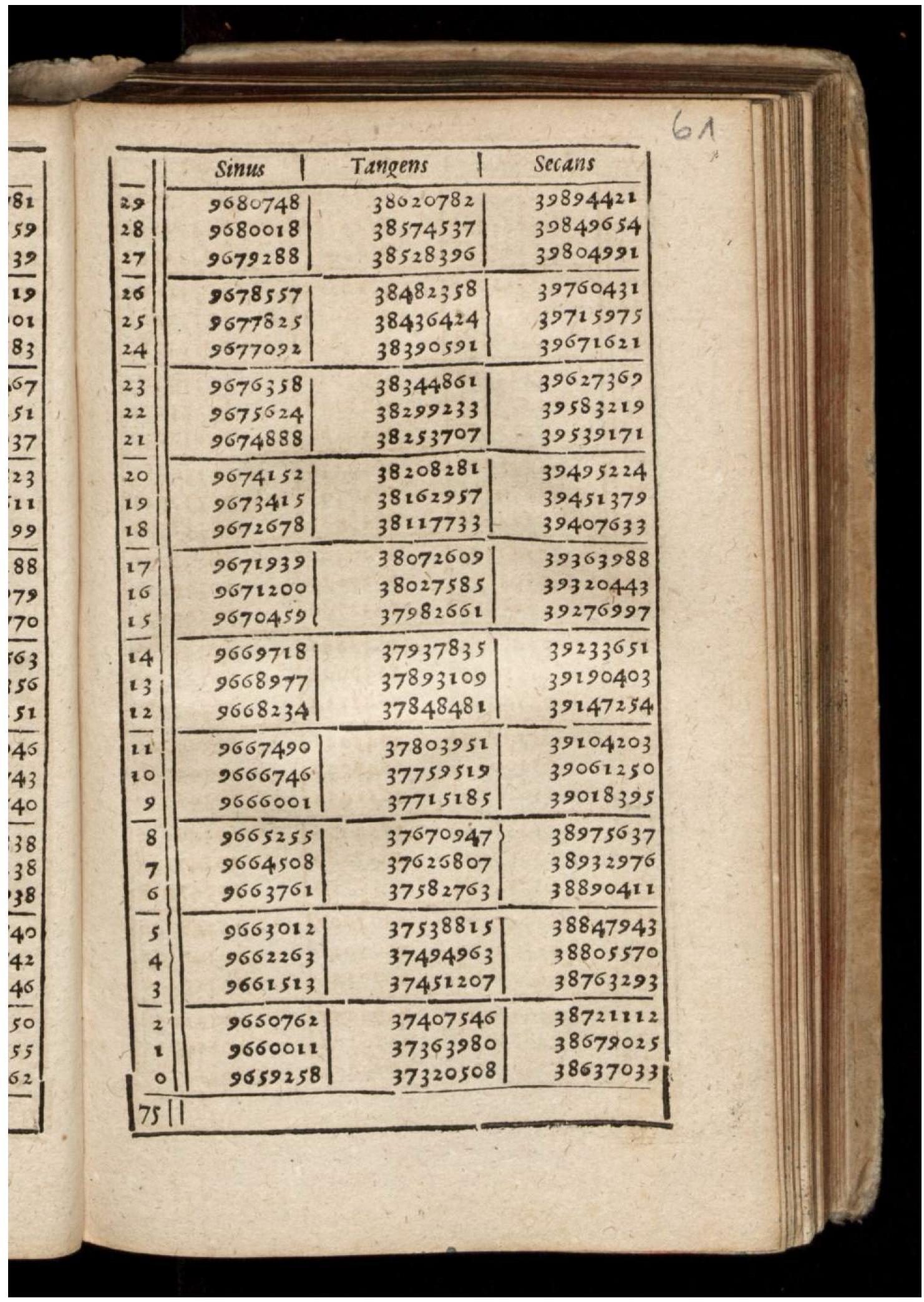
Trigonometric Tables
In mathematics, tables of trigonometric functions are useful in a number of areas. Before the existence of pocket calculators, trigonometric tables were essential for navigation, science and engineering. The calculation of mathematical tables was an important area of study, which led to the development of the first mechanical computing devices. Modern computers and pocket calculators now generate trigonometric function values on demand, using special libraries of mathematical code. Often, these libraries use pre-calculated tables internally, and compute the required value by using an appropriate interpolation method. Interpolation of simple look-up tables of trigonometric functions is still used in computer graphics, where only modest accuracy may be required and speed is often paramount. Another important application of trigonometric tables and generation schemes is for fast Fourier transform (FFT) algorithms, where the same trigonometric function values (called ''twiddle fa ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Brahman
In Hinduism, ''Brahman'' (; IAST: ''Brahman'') connotes the highest universal principle, the ultimate reality of the universe.P. T. Raju (2006), ''Idealistic Thought of India'', Routledge, , page 426 and Conclusion chapter part XII In the Vedic Upanishads, ''Brahman'' constitutes the fundamental reality that transcends the duality of existence and non-existence. It serves as the absolute ground from which time, space, and natural law emerge. It represents an unchanging, eternal principle that exists beyond all boundaries and constraints. Because it transcends all limitation, ''Brahman'' ultimately defies complete description or categorization through language. In major schools of Hindu philosophy, it is the non-physical, efficient, formal and final cause of all that exists.For dualism school of Hinduism, see: Francis X. Clooney (2010), ''Hindu God, Christian God: How Reason Helps Break Down the Boundaries between Religions'', Oxford University Press, , pages 51โ58, 11 ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Astronomical Constant
An astronomical constant is any of several physical constants used in astronomy. Formal sets of constants, along with recommended values, have been defined by the International Astronomical Union (IAU) several times: in 1964Resolution No.4 of thXIIth General Assembly of the International Astronomical Union Hamburg, 1964. and in 1976Resolution No. 1 on the recommendations of Commission 4 on ephemerides in thXVIth General Assembly of the International Astronomical Union Grenoble, 1976. (with an update in 1994). In 2009 the IAU adopted a new current set, and recognizing that new observations and techniques continuously provide better values for these constants, they decidedResolution B2 of thXXVIIth General Assembly of the International Astronomical Union Rio de Janeiro, 2009. to not fix these values, but have the Working Group on Numerical Standards continuously maintain a set of Current Best Estimates.IAU Division I Working Group on Numerical Standards for Fundamental Astronomy and As ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Earth's Rotation
Earth's rotation or Earth's spin is the rotation of planet Earth around its own Rotation around a fixed axis, axis, as well as changes in the orientation (geometry), orientation of the rotation axis in space. Earth rotates eastward, in prograde motion. As viewed from the northern polar star Polaris, Earth turns counterclockwise. The North Pole, also known as the Geographic North Pole or Terrestrial North Pole, is the point in the Northern Hemisphere where Earth's axis of rotation meets its surface. This point is distinct from Earth's north magnetic pole. The South Pole is the other point where Earth's axis of rotation intersects its surface, in Antarctica. Earth rotates once in about 24 hours with respect to the Sun, but once every 23 hours, 56 minutes and 4 seconds with respect to other distant stars (#Stellar and sidereal day, see below). Earth's rotation is slowing slightly with time; thus, a day was shorter in the past. This is due to the tidal acceleration, tidal effects ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Eccentricity (mathematics)
In mathematics, the eccentricity of a Conic section#Eccentricity, conic section is a non-negative real number that uniquely characterizes its shape. One can think of the eccentricity as a measure of how much a conic section deviates from being circular. In particular: * The eccentricity of a circle is 0. * The eccentricity of a non-circular ellipse is between 0 and 1. * The eccentricity of a parabola is 1. * The eccentricity of a hyperbola is greater than 1. * The eccentricity of a pair of Line (geometry), lines is \infty. Two conic sections with the same eccentricity are similarity (geometry), similar. Definitions Any conic section can be defined as the Locus (mathematics), locus of points whose distances to a point (the focus) and a line (the directrix) are in a constant ratio. That ratio is called the ''eccentricity'', commonly denoted as . The eccentricity can also be defined in terms of the intersection of a plane and a Cone (geometry), double-napped cone associated with ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |