Uniform Continuity on:
[Wikipedia]
[Google]
[Amazon]
 In
In
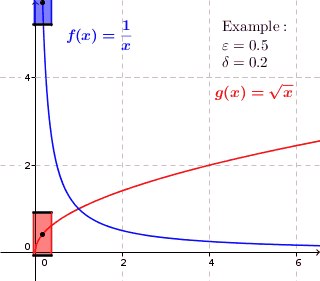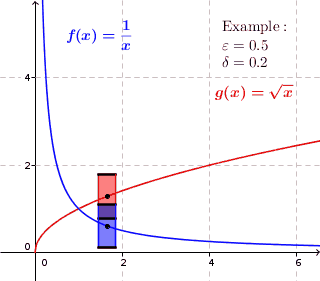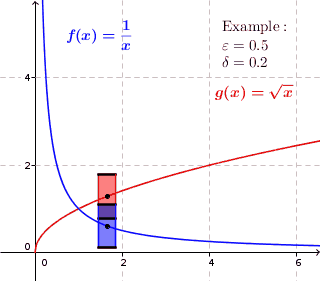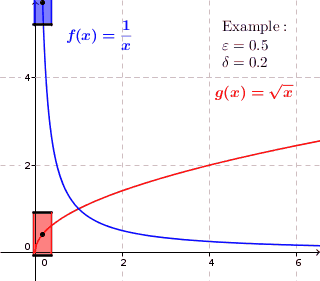
File:Gleichmäßig stetige Funktion.svg, For uniformly continuous functions, for each positive real number there is a positive real number such that when we draw a rectangle around each point of the graph with a width slightly less than and a height slightly less than , the graph lies completely inside the height of the rectangle.
File:Nicht gleichmäßig stetige Funktion.svg, For functions that are not uniformly continuous, there is a positive real number such that for every positive real number there is a point on the graph so that when we draw a rectangle with a height slightly less than and a width slightly less than around that point, there is a function value directly above or below the rectangle. There might be a graph point where the graph is completely inside the height of the rectangle but this is not true for every point of the graph.
 In
In mathematics
Mathematics is an area of knowledge that includes the topics of numbers, formulas and related structures, shapes and the spaces in which they are contained, and quantities and their changes. These topics are represented in modern mathematics ...
, a real function
Function or functionality may refer to:
Computing
* Function key, a type of key on computer keyboards
* Function model, a structured representation of processes in a system
* Function object or functor or functionoid, a concept of object-oriente ...
of real numbers is said to be uniformly continuous if there is a positive real number such that function values over any function domain interval of the size are as close to each other as we want. In other words, for a uniformly continuous real function of real numbers, if we want function value differences to be less than any positive real number , then there is a positive real number such that at any and in any function interval of the size .
The difference between uniform continuity and (ordinary) continuity is that, in uniform continuity there is a globally applicable (the size of a function domain interval over which function value differences are less than ) that depends on only , while in (ordinary) continuity there is a locally applicable that depends on the both and . So uniform continuity is a stronger continuity condition than continuity; a function that is uniformly continuous is continuous but a function that is continuous is not necessarily uniformly continuous. The concepts of uniform continuity and continuity can be expanded to functions defined between metric spaces
In mathematics, a metric space is a set together with a notion of ''distance'' between its elements, usually called points. The distance is measured by a function called a metric or distance function. Metric spaces are the most general settin ...
.
Continuous functions can fail to be uniformly continuous if they are unbounded on a bounded domain, such as on , or if their slopes become unbounded on an infinite domain, such as on the real (number) line. However, any Lipschitz map
In mathematical analysis, Lipschitz continuity, named after German mathematician Rudolf Lipschitz, is a strong form of uniform continuity for functions. Intuitively, a Lipschitz continuous function is limited in how fast it can change: there exis ...
between metric spaces is uniformly continuous, in particular any isometry
In mathematics, an isometry (or congruence, or congruent transformation) is a distance-preserving transformation between metric spaces, usually assumed to be bijective. The word isometry is derived from the Ancient Greek: ἴσος ''isos'' mea ...
(distance-preserving map).
Although continuity can be defined for functions between general topological spaces, defining uniform continuity requires more structure. The concept relies on comparing the sizes of neighbourhood
A neighbourhood (British English, Irish English, Australian English and Canadian English) or neighborhood (American English; see spelling differences) is a geographically localised community within a larger city, town, suburb or rural are ...
s of distinct points, so it requires a metric space, or more generally a uniform space
In the mathematical field of topology, a uniform space is a set with a uniform structure. Uniform spaces are topological spaces with additional structure that is used to define uniform properties such as completeness, uniform continuity and unifo ...
.
Definition for functions on metric spaces
For a function withmetric spaces
In mathematics, a metric space is a set together with a notion of ''distance'' between its elements, usually called points. The distance is measured by a function called a metric or distance function. Metric spaces are the most general settin ...
and , the following definitions of uniform continuity and (ordinary) continuity hold.
Definition of uniform continuity
* is called uniformly continuous if for everyreal number
In mathematics, a real number is a number that can be used to measure a ''continuous'' one-dimensional quantity such as a distance, duration or temperature. Here, ''continuous'' means that values can have arbitrarily small variations. Every real ...
there exists a real number such that for every with , we have . The set for each is a neighbourhood of and the set for each is a neighbourhood of by the definition of a neighbourhood in a metric space.
** If and are subsets of the real line
In elementary mathematics, a number line is a picture of a graduated straight line (geometry), line that serves as visual representation of the real numbers. Every point of a number line is assumed to correspond to a real number, and every real ...
, then and can be the standard one-dimensional Euclidean distance, yielding the following definition: for every real number there exists a real number such that for every , (where is a material conditional
The material conditional (also known as material implication) is an operation commonly used in logic. When the conditional symbol \rightarrow is interpreted as material implication, a formula P \rightarrow Q is true unless P is true and Q is ...
statement saying "if , then ").
* Equivalently, is said to be uniformly continuous if . Here quantifications (, , , and ) are used.
* Alternatively, is said to be uniformly continuous if there is a function of all positive real numbers , representing the maximum positive real number, such that for every if then . is a monotonically non-decreasing function.
Definition of (ordinary) continuity
* is called continuous if for everyreal number
In mathematics, a real number is a number that can be used to measure a ''continuous'' one-dimensional quantity such as a distance, duration or temperature. Here, ''continuous'' means that values can have arbitrarily small variations. Every real ...
there exists a real number such that for every with , we have . The set is a neighbourhood of . Thus, (ordinary) continuity is a local property of the function at the point .
* Equivalently, a function is said to be continuous if .
* Alternatively, a function is said to be continuous if there is a function of all positive real numbers and , representing the maximum positive real number, such that at each if satisfies then . At every , is a monotonically non-decreasing function.
Local continuity versus global uniform continuity
In the definitions, the difference between uniform continuity and continuity is that, in uniform continuity there is a globally applicable (the size of a neighbourhood in over which values of the metric for function values in are less than ) that depends on only while in continuity there is a locally applicable that depends on the both and . Continuity is a ''local'' property of a function — that is, a function is continuous, or not, at a particular point of the function domain , and this can be determined by looking at only the values of the function in an arbitrarily small neighbourhood of that point. When we speak of a function being continuous on an interval, we mean that the function is continuous at every point of the interval. In contrast, uniform continuity is a ''global'' property of , in the sense that the standard definition of uniform continuity refers to every point of . On the other hand, it is possible to give a definition that is ''local'' in terms of the natural extension (the characteristics of which at nonstandard points are determined by the global properties of ), although it is not possible to give a local definition of uniform continuity for an arbitrary hyperreal-valued function, seebelow
Below may refer to:
*Earth
*Ground (disambiguation)
*Soil
*Floor
*Bottom (disambiguation)
Bottom may refer to:
Anatomy and sex
* Bottom (BDSM), the partner in a BDSM who takes the passive, receiving, or obedient role, to that of the top or ...
.
A mathematical definition that a function is continuous on an interval and a definition that is uniformly continuous on are structurally similar as shown in the following.
Continuity of a function for metric spaces
In mathematics, a metric space is a set together with a notion of ''distance'' between its elements, usually called points. The distance is measured by a function called a metric or distance function. Metric spaces are the most general settin ...
and at every point '''' of an interval (i.e., continuity of on the interval ) is expressed by a formula starting with quantifications
: ,
(metrics and are and for for the set of real numbers ).
For uniform continuity, the order of the first, second, and third quantifications (, , and ) are rotated:
: .
Thus for continuity on the interval, one takes an arbitrary point of the interval'','' and then there must exist a distance ,
:
while for uniform continuity, a single must work uniformly for all points of the interval,
:
Properties
Every uniformly continuous function iscontinuous
Continuity or continuous may refer to:
Mathematics
* Continuity (mathematics), the opposing concept to discreteness; common examples include
** Continuous probability distribution or random variable in probability and statistics
** Continuous ...
, but the converse does not hold. Consider for instance the continuous function where is the set of real numbers. Given a positive real number , uniform continuity requires the existence of a positive real number such that for all with , we have . But
:
and as goes to be a higher and higher value, needs to be lower and lower to satisfy for positive real numbers and the given . This means that there is no specifiable (no matter how small it is) positive real number to satisfy the condition for to be uniformly continuous so is not uniformly continuous.
Any absolutely continuous
In calculus, absolute continuity is a smoothness property of functions that is stronger than continuity and uniform continuity. The notion of absolute continuity allows one to obtain generalizations of the relationship between the two central oper ...
function (over a compact interval) is uniformly continuous. On the other hand, the Cantor function
In mathematics, the Cantor function is an example of a function that is continuous, but not absolutely continuous. It is a notorious counterexample in analysis, because it challenges naive intuitions about continuity, derivative, and measure. Th ...
is uniformly continuous but not absolutely continuous.
The image of a totally bounded In topology and related branches of mathematics, total-boundedness is a generalization of compactness for circumstances in which a set is not necessarily closed. A totally bounded set can be covered by finitely many subsets of every fixed “size ...
subset under a uniformly continuous function is totally bounded. However, the image of a bounded subset of an arbitrary metric space under a uniformly continuous function need not be bounded: as a counterexample, consider the identity function from the integers endowed with the discrete metric
Discrete may refer to:
*Discrete particle or quantum in physics, for example in quantum theory
* Discrete device, an electronic component with just one circuit element, either passive or active, other than an integrated circuit
*Discrete group, a ...
to the integers endowed with the usual Euclidean metric
In mathematics, the Euclidean distance between two points in Euclidean space is the length of a line segment between the two points.
It can be calculated from the Cartesian coordinates of the points using the Pythagorean theorem, therefore oc ...
.
The Heine–Cantor theorem
In mathematics, the Heine–Cantor theorem, named after Eduard Heine and Georg Cantor, states that if f \colon M \to N is a continuous function between two metric spaces M and N, and M is compact, then f is uniformly continuous. An important speci ...
asserts that ''every continuous function on a compact set
In mathematics, specifically general topology, compactness is a property that seeks to generalize the notion of a closed and bounded subset of Euclidean space by making precise the idea of a space having no "punctures" or "missing endpoints", i ...
is uniformly continuous''. In particular, ''if a function is continuous on a closed bounded interval of the real line, it is uniformly continuous on that interval''. The Darboux integrability of continuous functions follows almost immediately from this theorem.
If a real-valued function is continuous on and exists (and is finite), then is uniformly continuous. In particular, every element of , the space of continuous functions on that vanish at infinity, is uniformly continuous. This is a generalization of the Heine-Cantor theorem mentioned above, since .
Examples and nonexamples
Examples
* Linear functions are the simplest examples of uniformly continuous functions. * Any continuous function on the interval is also uniformly continuous, since is a compact set. * Every function which is differentiable and has bounded derivative is uniformly continuous. * EveryLipschitz continuous
In mathematical analysis, Lipschitz continuity, named after German mathematician Rudolf Lipschitz, is a strong form of uniform continuity for functions. Intuitively, a Lipschitz continuous function is limited in how fast it can change: there exis ...
map between two metric spaces is uniformly continuous. More generally, every Hölder continuous Hölder:
* ''Hölder, Hoelder'' as surname
* Hölder condition
* Hölder's inequality
* Hölder mean
* Jordan–Hölder theorem In abstract algebra, a composition series provides a way to break up an algebraic structure, such as a group or a modul ...
function is uniformly continuous.
* The absolute value function is uniformly continuous, despite not being differentiable at . This shows uniformly continuous functions are not always differentiable.
* Despite being nowhere differentiable, the Weierstrass function
In mathematics, the Weierstrass function is an example of a real-valued function (mathematics), function that is continuous function, continuous everywhere but Differentiable function, differentiable nowhere. It is an example of a fractal curve ...
is uniformly continuous.
* Every member of a uniformly equicontinuous set of functions is uniformly continuous.
Nonexamples
* Functions that are unbounded on a bounded domain are not uniformly continuous. Thetangent function
In mathematics, the trigonometric functions (also called circular functions, angle functions or goniometric functions) are real functions which relate an angle of a right-angled triangle to ratios of two side lengths. They are widely used in all ...
is continuous on the interval but is ''not'' uniformly continuous on that interval, as it goes to infinity as .
* Functions that have slopes that become unbounded on an infinite domain cannot be uniformly continuous. The exponential function is continuous everywhere on the real line but is not uniformly continuous on the line, since its derivative tends to infinity as .
Visualization
For a uniformly continuous function, for every positive real number there is a positive real number such that two function values and have the maximum distance whenever and are within the maximum distance . Thus at each point of the graph, if we draw a rectangle with a height slightly less than and width a slightly less than around that point, then the graph lies completely within the height of the rectangle, i.e., the graph do not pass through the top or the bottom side of the rectangle. For functions that are not uniformly continuous, this isn't possible; for these functions, the graph might lie inside the height of the rectangle at some point on the graph but there is a point on the graph where the graph lies above or below the rectangle. (the graph penetrates the top or bottom side of the rectangle.)History
The first published definition of uniform continuity was by Heine in 1870, and in 1872 he published a proof that a continuous function on an open interval need not be uniformly continuous. The proofs are almost verbatim given byDirichlet
Johann Peter Gustav Lejeune Dirichlet (; 13 February 1805 – 5 May 1859) was a German mathematician who made deep contributions to number theory (including creating the field of analytic number theory), and to the theory of Fourier series and ...
in his lectures on definite integrals in 1854. The definition of uniform continuity appears earlier in the work of Bolzano where he also proved that continuous functions on an open interval do not need to be uniformly continuous. In addition he also states that a continuous function on a closed interval is uniformly continuous, but he does not give a complete proof.
Other characterizations
Non-standard analysis
Innon-standard analysis
The history of calculus is fraught with philosophical debates about the meaning and logical validity of fluxions or infinitesimal numbers. The standard way to resolve these debates is to define the operations of calculus using epsilon–delta ...
, a real-valued function '''' of a real variable is microcontinuous at a point '''' precisely if the difference is infinitesimal whenever '''' is infinitesimal. Thus '''' is continuous on a set '''' in precisely if is microcontinuous at every real point . Uniform continuity can be expressed as the condition that (the natural extension of) is microcontinuous not only at real points in , but at all points in its non-standard counterpart (natural extension) in . Note that there exist hyperreal-valued functions which meet this criterion but are not uniformly continuous, as well as uniformly continuous hyperreal-valued functions which do not meet this criterion, however, such functions cannot be expressed in the form for any real-valued function . (see non-standard calculus
In mathematics, nonstandard calculus is the modern application of infinitesimals, in the sense of nonstandard analysis, to infinitesimal calculus. It provides a rigorous justification for some arguments in calculus that were previously considered m ...
for more details and examples).
Cauchy continuity
For a function between metric spaces, uniform continuity implies Cauchy continuity . More specifically, let be a subset of . If a function is uniformly continuous then for every pair of sequences and such that : we have :Relations with the extension problem
Let be a metric space, a subset of '','' a complete metric space, and a continuous function. A question to answer: ''When can be extended to a continuous function on all of ?'' If '''' is closed in , the answer is given by theTietze extension theorem
In topology, the Tietze extension theorem (also known as the Tietze–Urysohn–Brouwer extension theorem) states that continuous functions on a closed subset of a normal topological space can be extended to the entire space, preserving boundedness ...
. So it is necessary and sufficient to extend '''' to the closure of in : that is, we may assume without loss of generality that '''' is dense in , and this has the further pleasant consequence that if the extension exists, it is unique. A sufficient condition for to extend to a continuous function is that it is Cauchy-continuous, i.e., the image under '''' of a Cauchy sequence remains Cauchy. If is complete (and thus the completion of ''''), then every continuous function from '''' to a metric space '''' is Cauchy-continuous. Therefore when '''' is complete, '''' extends to a continuous function if and only if '''' is Cauchy-continuous.
It is easy to see that every uniformly continuous function is Cauchy-continuous and thus extends to ''''. The converse does not hold, since the function is, as seen above, not uniformly continuous, but it is continuous and thus Cauchy continuous. In general, for functions defined on unbounded spaces like '''', uniform continuity is a rather strong condition. It is desirable to have a weaker condition from which to deduce extendability.
For example, suppose is a real number. At the precalculus level, the function can be given a precise definition only for rational values of '''' (assuming the existence of qth roots of positive real numbers, an application of the Intermediate Value Theorem
In mathematical analysis, the intermediate value theorem states that if f is a continuous function whose domain contains the interval , then it takes on any given value between f(a) and f(b) at some point within the interval.
This has two import ...
). One would like to extend to a function defined on all of . The identity
:
shows that '''' is not uniformly continuous on the set '''' of all rational numbers; however for any bounded interval '''' the restriction of '''' to is uniformly continuous, hence Cauchy-continuous, hence extends to a continuous function on ''''. But since this holds for every '''', there is then a unique extension of '''' to a continuous function on all of ''''.
More generally, a continuous function whose restriction to every bounded subset of '''' is uniformly continuous is extendable to '''', and the converse holds if '''' is locally compact In topology and related branches of mathematics, a topological space is called locally compact if, roughly speaking, each small portion of the space looks like a small portion of a compact space. More precisely, it is a topological space in which ev ...
.
A typical application of the extendability of a uniformly continuous function is the proof of the inverse Fourier transformation
A Fourier transform (FT) is a mathematical transform that decomposes functions into frequency components, which are represented by the output of the transform as a function of frequency. Most commonly functions of time or space are transformed, ...
formula. We first prove that the formula is true for test functions, there are densely many of them. We then extend the inverse map to the whole space using the fact that linear map is continuous; thus, uniformly continuous.
Generalization to topological vector spaces
In the special case of twotopological vector spaces
In mathematics, a topological vector space (also called a linear topological space and commonly abbreviated TVS or t.v.s.) is one of the basic structures investigated in functional analysis.
A topological vector space is a vector space that is als ...
and , the notion of uniform continuity of a map becomes: for any neighborhood of zero in , there exists a neighborhood of zero in such that implies
For linear transformation
In mathematics, and more specifically in linear algebra, a linear map (also called a linear mapping, linear transformation, vector space homomorphism, or in some contexts linear function) is a mapping V \to W between two vector spaces that pre ...
s , uniform continuity is equivalent to continuity. This fact is frequently used implicitly in functional analysis
Functional analysis is a branch of mathematical analysis, the core of which is formed by the study of vector spaces endowed with some kind of limit-related structure (e.g. Inner product space#Definition, inner product, Norm (mathematics)#Defini ...
to extend a linear map off a dense subspace of a Banach space
In mathematics, more specifically in functional analysis, a Banach space (pronounced ) is a complete normed vector space. Thus, a Banach space is a vector space with a metric that allows the computation of vector length and distance between vector ...
.
Generalization to uniform spaces
Just as the most natural and general setting for continuity istopological space
In mathematics, a topological space is, roughly speaking, a geometrical space in which closeness is defined but cannot necessarily be measured by a numeric distance. More specifically, a topological space is a set whose elements are called points ...
s, the most natural and general setting for the study of ''uniform'' continuity are the uniform space
In the mathematical field of topology, a uniform space is a set with a uniform structure. Uniform spaces are topological spaces with additional structure that is used to define uniform properties such as completeness, uniform continuity and unifo ...
s. A function between uniform spaces is called ''uniformly continuous'' if for every entourage
An entourage () is an informal group or band of people who are closely associated with a (usually) famous, notorious, or otherwise notable individual. The word can also refer to:
Arts and entertainment
* L'entourage, French hip hop / rap collecti ...
'''' in '''' there exists an entourage '''' in '''' such that for every in we have in .
In this setting, it is also true that uniformly continuous maps transform Cauchy sequences into Cauchy sequences.
Each compact Hausdorff space
In mathematics, specifically general topology, compactness is a property that seeks to generalize the notion of a closed and bounded subset of Euclidean space by making precise the idea of a space having no "punctures" or "missing endpoints", i ...
possesses exactly one uniform structure compatible with the topology. A consequence is a generalization of the Heine-Cantor theorem: each continuous function from a compact Hausdorff space to a uniform space is uniformly continuous.
See also
* *References
Further reading
* Chapter II is a comprehensive reference of uniform spaces. * * * * * *{{citation, last1=Rusnock, first1=P., last2=Kerr-Lawson, first2=A., title=Bolzano and uniform continuity, journal=Historia Mathematica, volume=32, year=2005, pages=303–311, number=3, doi=10.1016/j.hm.2004.11.003, doi-access=free Theory of continuous functions Calculus Mathematical analysis General topology