Spiral Of Archimedes on:
[Wikipedia]
[Google]
[Amazon]
The Archimedean spiral (also known as the arithmetic spiral) is a
spiral
In mathematics, a spiral is a curve which emanates from a point, moving farther away as it revolves around the point.
Helices
Two major definitions of "spiral" in the American Heritage Dictionary are:Greek mathematician
 Here is the modulus of the
Here is the modulus of the
 Given the parametrization in cartesian coordinates
the arc length from to is
or, equivalently:
The total length from to is therefore
The curvature is given by
Given the parametrization in cartesian coordinates
the arc length from to is
or, equivalently:
The total length from to is therefore
The curvature is given by
 The Archimedean spiral has the property that any ray from the origin intersects successive turnings of the spiral in points with a constant separation distance (equal to if is measured in radians), hence the name "arithmetic spiral". In contrast to this, in a logarithmic spiral these distances, as well as the distances of the intersection points measured from the origin, form a geometric progression.
The Archimedean spiral has two arms, one for and one for . The two arms are smoothly connected at the origin. Only one arm is shown on the accompanying graph. Taking the mirror image of this arm across the -axis will yield the other arm.
For large a point moves with well-approximated uniform acceleration along the Archimedean spiral while the spiral corresponds to the locations over time of a point moving away from a fixed point with a constant speed along a line which rotates with constant angular velocity (see contribution from Mikhail Gaichenkov).
As the Archimedean spiral grows, its evolute asymptotically approaches a circle with radius .
The Archimedean spiral has the property that any ray from the origin intersects successive turnings of the spiral in points with a constant separation distance (equal to if is measured in radians), hence the name "arithmetic spiral". In contrast to this, in a logarithmic spiral these distances, as well as the distances of the intersection points measured from the origin, form a geometric progression.
The Archimedean spiral has two arms, one for and one for . The two arms are smoothly connected at the origin. Only one arm is shown on the accompanying graph. Taking the mirror image of this arm across the -axis will yield the other arm.
For large a point moves with well-approximated uniform acceleration along the Archimedean spiral while the spiral corresponds to the locations over time of a point moving away from a fixed point with a constant speed along a line which rotates with constant angular velocity (see contribution from Mikhail Gaichenkov).
As the Archimedean spiral grows, its evolute asymptotically approaches a circle with radius .
 The Archimedean spiral has a variety of real-world applications.
The Archimedean spiral has a variety of real-world applications. 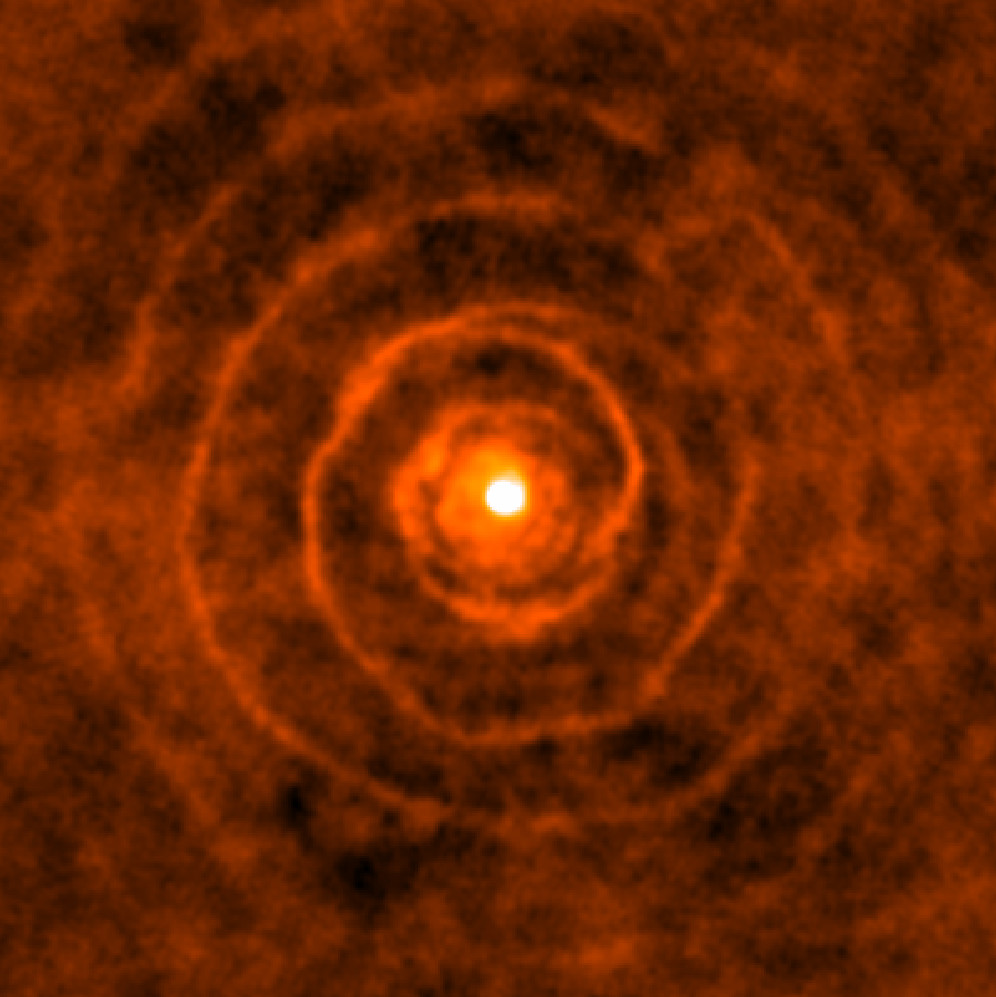 They are also used to model the pattern that occurs in a roll of paper or tape of constant thickness wrapped around a cylinder.
Many dynamic spirals (such as the Parker spiral of the solar wind, or the pattern made by a Catherine's wheel) are Archimedean. For instance, the star
They are also used to model the pattern that occurs in a roll of paper or tape of constant thickness wrapped around a cylinder.
Many dynamic spirals (such as the Parker spiral of the solar wind, or the pattern made by a Catherine's wheel) are Archimedean. For instance, the star
Jonathan Matt making the Archimedean spiral interesting - Video : The surprising beauty of Mathematics
-
Page with Java application to interactively explore the Archimedean spiral and its related curves
Online exploration using JSXGraph (JavaScript)
Archimedean spiral at "mathcurve"
{{DEFAULTSORT:Archimedean Spiral Squaring the circle Spirals
Archimedes
Archimedes of Syracuse (;; ) was a Greek mathematician, physicist, engineer, astronomer, and inventor from the ancient city of Syracuse in Sicily. Although few details of his life are known, he is regarded as one of the leading scientists ...
. It is the locus corresponding to the locations over time of a point moving away from a fixed point with a constant speed along a line that rotates with constant angular velocity
In physics, angular velocity or rotational velocity ( or ), also known as angular frequency vector,(UP1) is a pseudovector representation of how fast the angular position or orientation of an object changes with time (i.e. how quickly an objec ...
. Equivalently, in polar coordinates it can be described by the equation
with real numbers
In mathematics, a real number is a number that can be used to measure a ''continuous'' one-dimensional quantity such as a distance, duration or temperature. Here, ''continuous'' means that values can have arbitrarily small variations. Every real ...
and . Changing the parameter moves the centerpoint of the spiral outward from the origin (positive toward and negative toward ) essentially through a rotation of the spiral, while controls the distance between loops.
From the above equation, it can thus be stated: position of particle from point of start is proportional to angle as time elapses.
Archimedes described such a spiral in his book ''On Spirals
''On Spirals'' ( el, Περὶ ἑλίκων) is a treatise by Archimedes, written around 225 BC. Notably, Archimedes employed the Archimedean spiral in this book to square the circle and trisect an angle.
Contents
Preface
Archimedes begins ''O ...
''. Conon of Samos
Conon of Samos ( el, Κόνων ὁ Σάμιος, ''Konōn ho Samios''; c. 280 – c. 220 BC) was a Greek astronomer and mathematician. He is primarily remembered for naming the constellation Coma Berenices.
Life and work
Conon was born on Samos ...
was a friend of his and Pappus states that this spiral was discovered by Conon.
Derivation of general equation of spiral
A physical approach is used below to understand the notion of Archimedean spirals. Suppose a point object moves in the Cartesian system with a constant velocity directed parallel to the -axis, with respect to the -plane. Let at time , the object was at an arbitrary point . If the plane rotates with a constantangular velocity
In physics, angular velocity or rotational velocity ( or ), also known as angular frequency vector,(UP1) is a pseudovector representation of how fast the angular position or orientation of an object changes with time (i.e. how quickly an objec ...
about the -axis, then the velocity of the point with respect to -axis may be written as:
 Here is the modulus of the
Here is the modulus of the position vector
In geometry, a position or position vector, also known as location vector or radius vector, is a Euclidean vector that represents the position of a point ''P'' in space in relation to an arbitrary reference origin ''O''. Usually denoted x, r, or s ...
of the particle at any time , is the velocity component along the -axis and is the component along the -axis. The figure shown alongside explains this.
The above equations can be integrated by applying integration by parts, leading to the following parametric equations:
Squaring the two equations and then adding (and some small alterations) results in the Cartesian equation
(using the fact that and ) or
Its polar form is
Arc length and curvature
Characteristics
General Archimedean spiral
Sometimes the term ''Archimedean spiral'' is used for the more general group of spirals The normal Archimedean spiral occurs when . Other spirals falling into this group include the hyperbolic spiral (), Fermat's spiral (), and the lituus ().Applications
One method ofsquaring the circle
Squaring the circle is a problem in geometry first proposed in Greek mathematics. It is the challenge of constructing a square with the area of a circle by using only a finite number of steps with a compass and straightedge. The difficulty ...
, due to Archimedes, makes use of an Archimedean spiral. Archimedes also showed how the spiral can be used to trisect an angle
Angle trisection is a classical problem of straightedge and compass construction of ancient Greek mathematics. It concerns construction of an angle equal to one third of a given arbitrary angle, using only two tools: an unmarked straightedge a ...
. Both approaches relax the traditional limitations on the use of straightedge and compass in ancient Greek geometric proofs.
 The Archimedean spiral has a variety of real-world applications.
The Archimedean spiral has a variety of real-world applications. Scroll compressor
A scroll compressor (also called ''spiral compressor'', scroll pump and scroll vacuum pump) is a device for compressing air or refrigerant. It is used in air conditioning equipment, as an automobile supercharger (where it is known as a scroll- ...
s, used for compressing gases, have rotors that can be made from two interleaved Archimedean spirals, involutes of a circle of the same size that almost resemble Archimedean spirals, or hybrid curves.
Archimedean spirals can be found in spiral antenna
In microwave systems, a spiral antenna is a type of RF antenna. It is shaped as a two-arm spiral, or more arms may be used. Spiral antennas were first described in 1956. ''Logarithmic'' spiral antennas belong to the class of frequency independ ...
, which can be operated over a wide range of frequencies.
The coils of watch balance springs and the grooves of very early gramophone records form Archimedean spirals, making the grooves evenly spaced (although variable track spacing was later introduced to maximize the amount of music that could be cut onto a record).
Asking for a patient to draw an Archimedean spiral is a way of quantifying human tremor
A tremor is an involuntary, somewhat rhythmic, muscle contraction and relaxation involving oscillations or twitching movements of one or more body parts. It is the most common of all involuntary movements and can affect the hands, arms, eyes, fa ...
; this information helps in diagnosing neurological diseases.
Archimedean spirals are also used in digital light processing (DLP) projection systems to minimize the " rainbow effect", making it look as if multiple colors are displayed at the same time, when in reality red, green, and blue are being cycled extremely quickly. Additionally, Archimedean spirals are used in food microbiology to quantify bacterial concentration through a spiral platter.
 They are also used to model the pattern that occurs in a roll of paper or tape of constant thickness wrapped around a cylinder.
Many dynamic spirals (such as the Parker spiral of the solar wind, or the pattern made by a Catherine's wheel) are Archimedean. For instance, the star
They are also used to model the pattern that occurs in a roll of paper or tape of constant thickness wrapped around a cylinder.
Many dynamic spirals (such as the Parker spiral of the solar wind, or the pattern made by a Catherine's wheel) are Archimedean. For instance, the star LL Pegasi
LL Pegasi (AFGL 3068) is a Mira variable star surrounded by a pinwheel-shaped nebula, IRAS 23166+1655, thought to be a Protoplanetary nebula, preplanetary nebula. It is a binary system (astronomy), binary system that includes an extreme ...
shows an approximate Archimedean spiral in the dust clouds surrounding it, thought to be ejected matter from the star that has been shepherded into a spiral by another companion star as part of a double star system.
See also
* Archimedes' screw * Euler spiral * Fermat's spiral * Golden spiral * Hyperbolic spiral * List of spirals * Logarithmic spiral * Spiral of Theodorus * Triple spiral symbolReferences
External links
Jonathan Matt making the Archimedean spiral interesting - Video : The surprising beauty of Mathematics
-
TedX Talks
TED Conferences, LLC (Technology, Entertainment, Design) is an American-Canadian non-profit media organization that posts international talks online for free distribution under the slogan "ideas worth spreading". TED was founded by Richard Sau ...
, Green Farms
Green's Farms is the oldest neighborhood in the town of Westport, Connecticut, Westport in Fairfield County, Connecticut, Fairfield County, Connecticut, United States. It was listed as a census-designated place prior to the 2020 United States cens ...
*
*
Page with Java application to interactively explore the Archimedean spiral and its related curves
Online exploration using JSXGraph (JavaScript)
Archimedean spiral at "mathcurve"
{{DEFAULTSORT:Archimedean Spiral Squaring the circle Spirals
Spiral
In mathematics, a spiral is a curve which emanates from a point, moving farther away as it revolves around the point.
Helices
Two major definitions of "spiral" in the American Heritage Dictionary are:Articles with example R code