Many
mathematical problems have been stated but not yet solved. These problems come from many
areas of mathematics, such as
theoretical physics
Theoretical physics is a branch of physics that employs mathematical models and abstractions of physical objects and systems to rationalize, explain and predict natural phenomena. This is in contrast to experimental physics, which uses experi ...
,
computer science
Computer science is the study of computation, automation, and information. Computer science spans theoretical disciplines (such as algorithms, theory of computation, information theory, and automation) to practical disciplines (includin ...
,
algebra
Algebra () is one of the areas of mathematics, broad areas of mathematics. Roughly speaking, algebra is the study of mathematical symbols and the rules for manipulating these symbols in formulas; it is a unifying thread of almost all of mathem ...
,
analysis
Analysis ( : analyses) is the process of breaking a complex topic or substance into smaller parts in order to gain a better understanding of it. The technique has been applied in the study of mathematics and logic since before Aristotle (3 ...
,
combinatorics
Combinatorics is an area of mathematics primarily concerned with counting, both as a means and an end in obtaining results, and certain properties of finite structures. It is closely related to many other areas of mathematics and has many a ...
,
algebraic,
differential,
discrete
Discrete may refer to:
*Discrete particle or quantum in physics, for example in quantum theory
*Discrete device, an electronic component with just one circuit element, either passive or active, other than an integrated circuit
*Discrete group, a ...
and
Euclidean geometries,
graph theory
In mathematics, graph theory is the study of '' graphs'', which are mathematical structures used to model pairwise relations between objects. A graph in this context is made up of '' vertices'' (also called ''nodes'' or ''points'') which are conn ...
,
group theory
In abstract algebra, group theory studies the algebraic structures known as group (mathematics), groups.
The concept of a group is central to abstract algebra: other well-known algebraic structures, such as ring (mathematics), rings, field ...
,
model theory
In mathematical logic, model theory is the study of the relationship between formal theories (a collection of sentences in a formal language expressing statements about a mathematical structure), and their models (those structures in which the ...
,
number theory
Number theory (or arithmetic or higher arithmetic in older usage) is a branch of pure mathematics devoted primarily to the study of the integers and integer-valued functions. German mathematician Carl Friedrich Gauss (1777–1855) said, "Math ...
,
set theory
Set theory is the branch of mathematical logic that studies sets, which can be informally described as collections of objects. Although objects of any kind can be collected into a set, set theory, as a branch of mathematics, is mostly concer ...
,
Ramsey theory
Ramsey theory, named after the British mathematician and philosopher Frank P. Ramsey, is a branch of mathematics that focuses on the appearance of order in a substructure given a structure of a known size. Problems in Ramsey theory typically ask ...
,
dynamical system
In mathematics, a dynamical system is a system in which a function describes the time dependence of a point in an ambient space. Examples include the mathematical models that describe the swinging of a clock pendulum, the flow of water i ...
s, and
partial differential equation
In mathematics, a partial differential equation (PDE) is an equation which imposes relations between the various partial derivatives of a multivariable function.
The function is often thought of as an "unknown" to be solved for, similarly to ...
s. Some problems belong to more than one discipline and are studied using techniques from different areas. Prizes are often awarded for the solution to a long-standing problem, and some lists of unsolved problems, such as the
Millennium Prize Problems
The Millennium Prize Problems are seven well-known complex mathematical problems selected by the Clay Mathematics Institute in 2000. The Clay Institute has pledged a US$1 million prize for the first correct solution to each problem. According ...
, receive considerable attention.
This list is a composite of notable unsolved problems mentioned in previously published lists, including but not limited to lists considered authoritative. Although this list may never be comprehensive, the problems listed here vary widely in both difficulty and importance.
Lists of unsolved problems in mathematics
Various mathematicians and organizations have published and promoted lists of unsolved mathematical problems. In some cases, the lists have been associated with prizes for the discoverers of solutions.
Millennium Prize Problems
Of the original seven
Millennium Prize Problems
The Millennium Prize Problems are seven well-known complex mathematical problems selected by the Clay Mathematics Institute in 2000. The Clay Institute has pledged a US$1 million prize for the first correct solution to each problem. According ...
listed by the
Clay Mathematics Institute
The Clay Mathematics Institute (CMI) is a private, non-profit foundation dedicated to increasing and disseminating mathematical knowledge. Formerly based in Peterborough, New Hampshire, the corporate address is now in Denver, Colorado. CMI's sc ...
in 2000, six remain unsolved to date:
*
Birch and Swinnerton-Dyer conjecture
In mathematics, the Birch and Swinnerton-Dyer conjecture (often called the Birch–Swinnerton-Dyer conjecture) describes the set of rational solutions to equations defining an elliptic curve. It is an open problem in the field of number theory ...
*
Hodge conjecture
*
Navier–Stokes existence and smoothness
The Navier–Stokes existence and smoothness problem concerns the mathematical properties of solutions to the Navier–Stokes equations, a system of partial differential equations that describe the motion of a fluid in space. Solutions to the N ...
*
P versus NP
The P versus NP problem is a major unsolved problem in theoretical computer science. In informal terms, it asks whether every problem whose solution can be quickly verified can also be quickly solved.
The informal term ''quickly'', used above ...
*
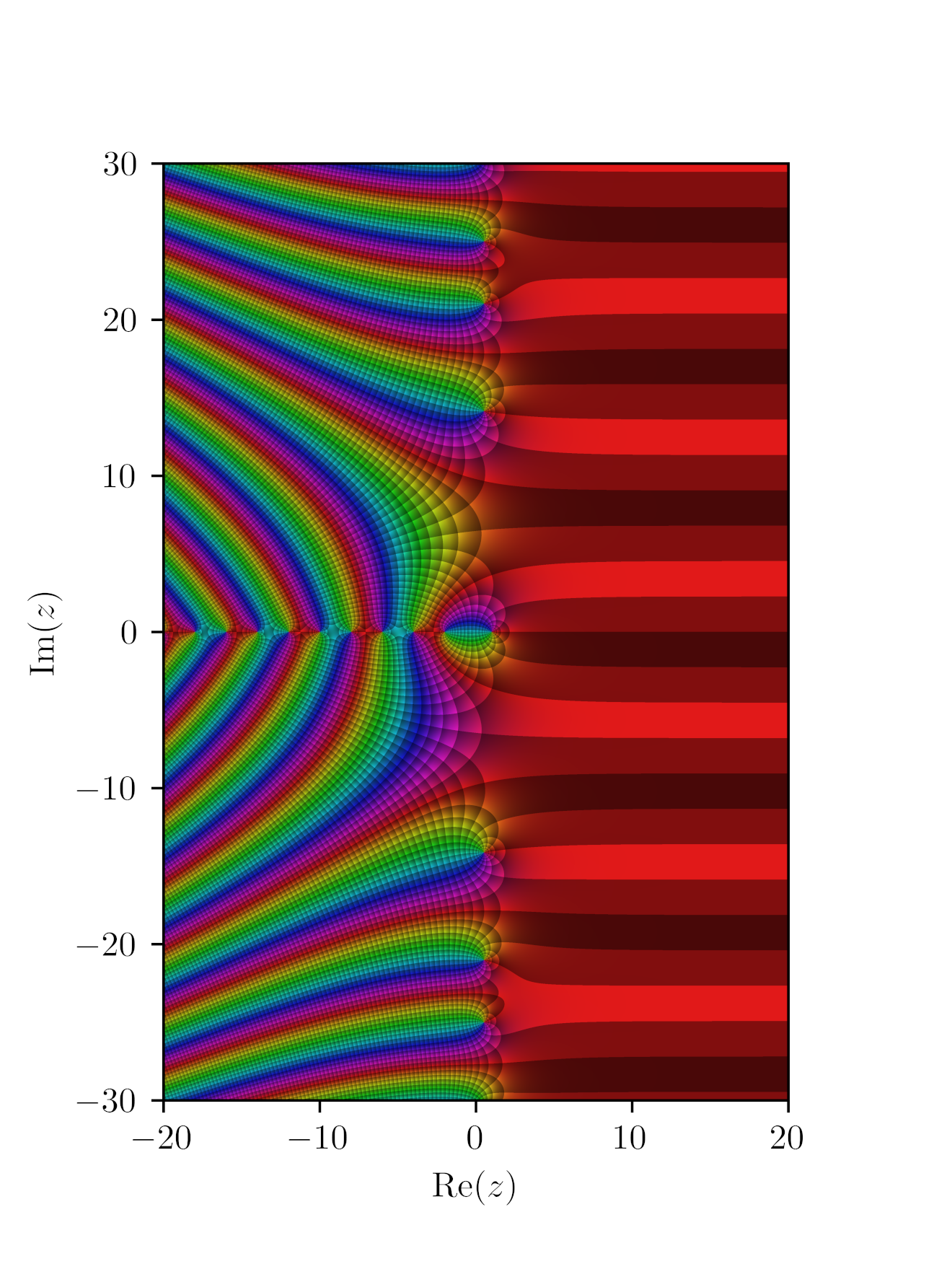
Riemann hypothesis
In mathematics, the Riemann hypothesis is the conjecture that the Riemann zeta function has its zeros only at the negative even integers and complex numbers with real part . Many consider it to be the most important unsolved problem in pu ...
*
Yang–Mills existence and mass gap
The seventh problem, the
Poincaré conjecture
In the mathematical field of geometric topology, the Poincaré conjecture (, , ) is a theorem about the characterization of the 3-sphere, which is the hypersphere that bounds the unit ball in four-dimensional space.
Originally conjectured b ...
, was solved by
Grigori Perelman
Grigori Yakovlevich Perelman ( rus, links=no, Григорий Яковлевич Перельман, p=ɡrʲɪˈɡorʲɪj ˈjakəvlʲɪvʲɪtɕ pʲɪrʲɪlʲˈman, a=Ru-Grigori Yakovlevich Perelman.oga; born 13 June 1966) is a Russian mathemati ...
in 2003. However, a generalization called the
smooth four-dimensional Poincaré conjecture—that is, whether a ''four''-dimensional topological sphere can have two or more inequivalent
smooth structures—is unsolved.
Unsolved problems
Algebra

*
Birch–Tate conjecture The Birch–Tate conjecture is a conjecture in mathematics (more specifically in algebraic K-theory) proposed by both Bryan John Birch and John Tate.
Statement
In algebraic K-theory, the group ''K''2 is defined as the center of the Steinberg g ...
on the relation between the order of the
center of the
Steinberg group of the
ring of integers
In mathematics, the ring of integers of an algebraic number field K is the ring of all algebraic integers contained in K. An algebraic integer is a root of a monic polynomial with integer coefficients: x^n+c_x^+\cdots+c_0. This ring is often d ...
of a
number field to the field's
Dedekind zeta function
In mathematics, the Dedekind zeta function of an algebraic number field ''K'', generally denoted ζ''K''(''s''), is a generalization of the Riemann zeta function (which is obtained in the case where ''K'' is the field of rational numbers Q). It ...
.
*
Bombieri–Lang conjectures on densities of rational points of
algebraic surfaces and
algebraic varieties
Algebraic varieties are the central objects of study in algebraic geometry, a sub-field of mathematics. Classically, an algebraic variety is defined as the set of solutions of a system of polynomial equations over the real or complex number
...
defined on
number fields
In mathematics, an algebraic number field (or simply number field) is an extension field K of the field of rational numbers such that the field extension K / \mathbb has finite degree (and hence is an algebraic field extension).
Thus K is a ...
and their
field extension
In mathematics, particularly in algebra, a field extension is a pair of fields E\subseteq F, such that the operations of ''E'' are those of ''F'' restricted to ''E''. In this case, ''F'' is an extension field of ''E'' and ''E'' is a subfield of ...
s.
*
Connes embedding problem in
Von Neumann algebra
In mathematics, a von Neumann algebra or W*-algebra is a *-algebra of bounded operators on a Hilbert space that is closed in the weak operator topology and contains the identity operator. It is a special type of C*-algebra.
Von Neumann a ...
theory
*
Crouzeix's conjecture
Crouzeix's conjecture is an unsolved (as of 2018) problem in matrix analysis. It was proposed by Michel Crouzeix in 2004, and it refines Crouzeix's theorem, which states:
: \, f(A)\, \le 11.08 \sup_ , f(z),
where the set W(A) is the field of ...
: the
matrix norm
In mathematics, a matrix norm is a vector norm in a vector space whose elements (vectors) are matrices (of given dimensions).
Preliminaries
Given a field K of either real or complex numbers, let K^ be the -vector space of matrices with m ...
of a complex function
applied to a complex matrix
is at most twice the
supremum
In mathematics, the infimum (abbreviated inf; plural infima) of a subset S of a partially ordered set P is a greatest element in P that is less than or equal to each element of S, if such an element exists. Consequently, the term ''greatest l ...
of
over the
field of values In the mathematical field of linear algebra and convex analysis, the numerical range or field of values of a complex n \times n matrix ''A'' is the set
:W(A) = \left\
where \mathbf^* denotes the conjugate transpose of the vector \mathbf. The ...
of
.
*
Eilenberg–Ganea conjecture: a group with
cohomological dimension 2 also has a 2-dimensional
Eilenberg–MacLane space
In mathematics, specifically algebraic topology, an Eilenberg–MacLane space Saunders Mac Lane originally spelt his name "MacLane" (without a space), and co-published the papers establishing the notion of Eilenberg–MacLane spaces under this nam ...
.
*
Farrell–Jones conjecture on whether certain
assembly maps are
isomorphisms.
**
Bost conjecture Bost may refer to:
Places
*Alternative name for Lashkargah, Afghanistan
** Boost Defenders, a cricket team from the region
** Bost Airport, near Lashkargah
*Bost, Allier, a commune in central France
People
*Bost (Μποστ) (1918–1995), pen na ...
: a specific case of the Farrell–Jones conjecture
*
Finite lattice representation problem
In mathematics, the finite lattice representation problem, or finite congruence lattice problem, asks whether every finite lattice is isomorphic to the congruence lattice of some finite algebra.
Background
A lattice is called algebraic if it ...
: is every finite
lattice isomorphic to the
congruence lattice of some finite
algebra
Algebra () is one of the areas of mathematics, broad areas of mathematics. Roughly speaking, algebra is the study of mathematical symbols and the rules for manipulating these symbols in formulas; it is a unifying thread of almost all of mathem ...
?
*
Goncharov conjecture on the
cohomology
In mathematics, specifically in homology theory and algebraic topology, cohomology is a general term for a sequence of abelian groups, usually one associated with a topological space, often defined from a cochain complex. Cohomology can be viewe ...
of certain
motivic complexes.
*
Green's conjecture: the
Clifford index of a non-
hyperelliptic curve is determined by the extent to which it, as a
canonical curve In mathematics, the canonical bundle of a non-singular algebraic variety V of dimension n over a field is the line bundle \,\!\Omega^n = \omega, which is the ''n''th exterior power of the cotangent bundle Ω on ''V''.
Over the complex numbers, ...
, has
linear syzygies.
*
Grothendieck–Katz p-curvature conjecture In mathematics, the Grothendieck–Katz p-curvature conjecture is a local-global principle for linear ordinary differential equations, related to differential Galois theory and in a loose sense analogous to the result in the Chebotarev density th ...
: a conjectured
local–global principle for
linear ordinary differential equations
Linearity is the property of a mathematical relationship (''function (mathematics), function'') that can be graph of a function, graphically represented as a straight Line (geometry), line. Linearity is closely related to ''Proportionality (mat ...
.
*
Hadamard conjecture
In mathematics, a Hadamard matrix, named after the French mathematician Jacques Hadamard, is a square matrix whose entries are either +1 or −1 and whose rows are mutually orthogonal. In geometric terms, this means that each pair of rows ...
: for every positive integer
, a
Hadamard matrix of order
exists.
**
Williamson conjecture In combinatorial mathematics, specifically in combinatorial design theory and combinatorial matrix theory the Williamson conjecture is that Williamson matrices of order n exist for all positive integers n.
Four symmetric and circulant matrices A, ...
: the problem of finding Williamson matrices, which can be used to construct Hadamard matrices.
*
Hadamard's maximal determinant problem: what is the largest
determinant
In mathematics, the determinant is a scalar value that is a function of the entries of a square matrix. It characterizes some properties of the matrix and the linear map represented by the matrix. In particular, the determinant is nonzero if ...
of a matrix with entries all equal to 1 or –1?
*
Hilbert's fifteenth problem: put
Schubert calculus on a rigorous foundation.
*
Hilbert's sixteenth problem
Hilbert's 16th problem was posed by David Hilbert at the Paris conference of the International Congress of Mathematicians in 1900, as part of his list of 23 problems in mathematics.
The original problem was posed as the ''Problem of the topolog ...
: what are the possible configurations of the
connected components of
M-curves?
*
Homological conjectures in commutative algebra In mathematics, homological conjectures have been a focus of research activity in commutative algebra since the early 1960s. They concern a number of interrelated (sometimes surprisingly so) conjectures relating various homological properties of a ...
*
Jacobson's conjecture
In abstract algebra, Jacobson's conjecture is an open problem in ring theory concerning the intersection of powers of the Jacobson radical of a Noetherian ring.
It has only been proven for special types of Noetherian rings, so far. Examples exist ...
: the intersection of all powers of the
Jacobson radical In mathematics, more specifically ring theory, the Jacobson radical of a ring R is the ideal consisting of those elements in R that annihilate all simple right R- modules. It happens that substituting "left" in place of "right" in the definitio ...
of a left-and-right
Noetherian ring
In mathematics, a Noetherian ring is a ring that satisfies the ascending chain condition on left and right ideals; if the chain condition is satisfied only for left ideals or for right ideals, then the ring is said left-Noetherian or right-Noethe ...
is precisely 0.
*
Kaplansky's conjectures
The mathematician Irving Kaplansky is notable for proposing numerous conjectures in several branches of mathematics, including a list of ten conjectures on Hopf algebras. They are usually known as Kaplansky's conjectures.
Group rings
Let be a fie ...
*
Köthe conjecture: if a ring has no
nil ideal In mathematics, more specifically ring theory, a left, right or two-sided ideal of a ring is said to be a nil ideal if each of its elements is nilpotent., p. 194
The nilradical of a commutative ring is an example of a nil ideal; in fact, it i ...
other than
, then it has no nil
one-sided ideal
In ring theory, a branch of abstract algebra, an ideal of a ring is a special subset of its elements. Ideals generalize certain subsets of the integers, such as the even numbers or the multiples of 3. Addition and subtraction of even number ...
other than
.
*
Monomial conjecture on
Noetherian local ring In abstract algebra, more specifically ring theory, local rings are certain rings that are comparatively simple, and serve to describe what is called "local behaviour", in the sense of functions defined on varieties or manifolds, or of algebraic ...
s
* Existence of
perfect cuboids and associated
cuboid conjectures
In mathematics, an Euler brick, named after Leonhard Euler, is a rectangular cuboid whose edges and face diagonals all have integer lengths. A primitive Euler brick is an Euler brick whose edge lengths are relatively prime. A perfect Euler brick ...
*
Pierce–Birkhoff conjecture: every piecewise-polynomial
is the maximum of a finite set of minimums of finite collections of polynomials.
*
Rota's basis conjecture: for matroids of rank
with
disjoint bases
, it is possible to create an
matrix whose rows are
and whose columns are also bases.
*
Sendov's conjecture: if a complex polynomial with degree at least
has all roots in the closed
unit disk
In mathematics, the open unit disk (or disc) around ''P'' (where ''P'' is a given point in the plane), is the set of points whose distance from ''P'' is less than 1:
:D_1(P) = \.\,
The closed unit disk around ''P'' is the set of points whose ...
, then each root is within distance
from some
critical point.
*
Serre's conjecture II: if
is a
simply connected
In topology, a topological space is called simply connected (or 1-connected, or 1-simply connected) if it is path-connected and every path between two points can be continuously transformed (intuitively for embedded spaces, staying within the spa ...
semisimple algebraic group over a perfect
field of
cohomological dimension at most
, then the
Galois cohomology In mathematics, Galois cohomology is the study of the group cohomology of Galois modules, that is, the application of homological algebra to modules for Galois groups. A Galois group ''G'' associated to a field extension ''L''/''K'' acts in a nat ...
set
is zero.
*
Serre's multiplicity conjectures In mathematics, Serre's multiplicity conjectures, named after Jean-Pierre Serre, are certain purely algebraic problems, in commutative algebra, motivated by the needs of algebraic geometry. Since André Weil's initial definition of intersection n ...
*
Uniform boundedness conjecture for rational points: do
algebraic curve
In mathematics, an affine algebraic plane curve is the zero set of a polynomial in two variables. A projective algebraic plane curve is the zero set in a projective plane of a homogeneous polynomial in three variables. An affine algebraic plane ...
s of
genus
Genus ( plural genera ) is a taxonomic rank used in the biological classification of living and fossil organisms as well as viruses. In the hierarchy of biological classification, genus comes above species and below family. In binomial n ...
over
number fields
In mathematics, an algebraic number field (or simply number field) is an extension field K of the field of rational numbers such that the field extension K / \mathbb has finite degree (and hence is an algebraic field extension).
Thus K is a ...
have at most some bounded number
of
-
rational point
In number theory and algebraic geometry, a rational point of an algebraic variety is a point whose coordinates belong to a given field. If the field is not mentioned, the field of rational numbers is generally understood. If the field is the fie ...
s?
*
Wild problems: problems involving classification of pairs of
matrices under simultaneous conjugation.
*
Zariski–Lipman conjecture
In mathematics, the Nakai conjecture is an unproven characterization of smooth algebraic varieties, conjectured by Japanese mathematician Yoshikazu Nakai in 1961.
It states that if ''V'' is a complex algebraic variety, such that its ring of diffe ...
: for a
complex algebraic variety
In algebraic geometry, a complex algebraic variety is an algebraic variety (in the scheme sense or otherwise) over the field of complex numbers. Parshin, Alexei N., and Igor Rostislavovich Shafarevich, eds. ''Algebraic Geometry III: Complex Algebr ...
with
coordinate ring
In algebraic geometry, an affine variety, or affine algebraic variety, over an algebraically closed field is the zero-locus in the affine space of some finite family of polynomials of variables with coefficients in that generate a prime ide ...
, if the
derivations of
are a
free module
In mathematics, a free module is a module that has a basis – that is, a generating set consisting of linearly independent elements. Every vector space is a free module, but, if the ring of the coefficients is not a division ring (not a fie ...
over
, then
is
smooth.
* Zauner's conjecture: do
SIC-POVMs exist in all dimensions?
*
Zilber–Pink conjecture
In mathematics, the Zilber–Pink conjecture is a far-reaching generalisation of many famous Diophantine conjectures and statements, such as André–Oort, Manin–Mumford, and Mordell–Lang. For algebraic tori and semiabelian varieties it wa ...
that if
is a mixed
Shimura variety or
semiabelian variety
In mathematics, particularly in algebraic geometry, complex analysis and algebraic number theory, an abelian variety is a projective algebraic variety that is also an algebraic group, i.e., has a group law that can be defined by regular functio ...
defined over
, and
is a subvariety, then
contains only finitely many atypical subvarieties.
Representation theory
*
Arthur's conjectures In mathematics, the Arthur conjectures are some conjectures about automorphic representation
In harmonic analysis and number theory, an automorphic form is a well-behaved function from a topological group ''G'' to the complex numbers (or comple ...
*
Dade's conjecture relating the numbers of
characters
Character or Characters may refer to:
Arts, entertainment, and media Literature
* ''Character'' (novel), a 1936 Dutch novel by Ferdinand Bordewijk
* ''Characters'' (Theophrastus), a classical Greek set of character sketches attributed to The ...
of
blocks of a finite group to the numbers of characters of blocks of local
subgroup
In group theory, a branch of mathematics, given a group ''G'' under a binary operation ∗, a subset ''H'' of ''G'' is called a subgroup of ''G'' if ''H'' also forms a group under the operation ∗. More precisely, ''H'' is a subgrou ...
s.
*
Demazure conjecture In mathematics, the Demazure conjecture is a conjecture about representations of algebraic groups over the integers made by . The conjecture implies that many of the results of his paper can be extended from complex algebraic groups to algebraic g ...
on
representation
Representation may refer to:
Law and politics
*Representation (politics), political activities undertaken by elected representatives, as well as other theories
** Representative democracy, type of democracy in which elected officials represent a ...
s of
algebraic group
In mathematics, an algebraic group is an algebraic variety endowed with a group structure which is compatible with its structure as an algebraic variety. Thus the study of algebraic groups belongs both to algebraic geometry and group theory.
...
s over the integers.
*
McKay conjecture: in a group
, the number of
irreducible complex characters of degree not divisible by a
prime number
A prime number (or a prime) is a natural number greater than 1 that is not a product of two smaller natural numbers. A natural number greater than 1 that is not prime is called a composite number. For example, 5 is prime because the only way ...
is equal to the number of irreducible complex characters of the
normalizer
In mathematics, especially group theory, the centralizer (also called commutant) of a subset ''S'' in a group ''G'' is the set of elements \mathrm_G(S) of ''G'' such that each member g \in \mathrm_G(S) commutes with each element of ''S'', ...
of any
Sylow -subgroup within
.
Notebook problems
* The Dniester Notebook () lists several hundred unsolved problems in algebra, particularly
ring theory
In algebra, ring theory is the study of rings—algebraic structures in which addition and multiplication are defined and have similar properties to those operations defined for the integers. Ring theory studies the structure of rings, their r ...
and
modulus theory.
* The Erlagol Notebook () lists unsolved problems in algebra and model theory.
Analysis

* The
Brennan conjecture: estimating the integral of powers of the moduli of the derivative of
conformal map
In mathematics, a conformal map is a function that locally preserves angles, but not necessarily lengths.
More formally, let U and V be open subsets of \mathbb^n. A function f:U\to V is called conformal (or angle-preserving) at a point u_0\in ...
s into the open unit disk, on certain subsets of
* The
four exponentials conjecture: the transcendence of at least one of four exponentials of combinations of irrationals
*
Goodman's conjecture
Goodman's conjecture on the coefficients of multivalent functions was proposed in complex analysis in 1948 by Adolph Winkler Goodman, an American mathematician.
Formulation
Let f(z)= \sum_^ be a p-valent function. The conjecture claims the fol ...
on the coefficients of
multivalent function
In mathematics, a multivalued function, also called multifunction, many-valued function, set-valued function, is similar to a function, but may associate several values to each input. More precisely, a multivalued function from a domain to a ...
s
*
Invariant subspace problem – does every
bounded operator
In functional analysis and operator theory, a bounded linear operator is a linear transformation L : X \to Y between topological vector spaces (TVSs) X and Y that maps bounded subsets of X to bounded subsets of Y.
If X and Y are normed vecto ...
on a complex
Banach space
In mathematics, more specifically in functional analysis, a Banach space (pronounced ) is a complete normed vector space. Thus, a Banach space is a vector space with a metric that allows the computation of vector length and distance between ve ...
send some non-trivial
closed subspace to itself?
* Kung–Traub conjecture on the optimal order of a multipoint iteration without memory
*
Lehmer's conjecture on the Mahler measure of non-cyclotomic polynomials
* The
Pompeiu problem In mathematics, the Pompeiu problem is a conjecture in integral geometry, named for Dimitrie Pompeiu, who posed the problem in 1929,
as follows. Suppose ''f'' is a nonzero continuous function defined on a Euclidean space, and ''K'' is a simply c ...
on the topology of domains for which some nonzero function has integrals that vanish over every congruent copy
*
Schanuel's conjecture on the transcendence degree of exponentials of linearly independent irrationals
[
* Vitushkin's conjecture on compact subsets of with analytic capacity
* Are (the ]Euler–Mascheroni constant
Euler's constant (sometimes also called the Euler–Mascheroni constant) is a mathematical constant usually denoted by the lowercase Greek letter gamma ().
It is defined as the limiting difference between the harmonic series and the natural ...
),, Catalan's constant
In mathematics, Catalan's constant , is defined by
: G = \beta(2) = \sum_^ \frac = \frac - \frac + \frac - \frac + \frac - \cdots,
where is the Dirichlet beta function. Its numerical value is approximately
:
It is not known whether is irra ...
, or Khinchin's constant In number theory, Aleksandr Yakovlevich Khinchin proved that for almost all real numbers ''x'', coefficients ''a'i'' of the continued fraction expansion of ''x'' have a finite geometric mean that is independent of the value of ''x'' and is kno ...
rational, algebraic irrational, or transcendental
Transcendence, transcendent, or transcendental may refer to:
Mathematics
* Transcendental number, a number that is not the root of any polynomial with rational coefficients
* Algebraic element or transcendental element, an element of a field exten ...
? What is the irrationality measure of each of these numbers?
* What is the exact value of Landau's constants, including Bloch's constant
In complex analysis, a branch of mathematics, Bloch's theorem describes the behaviour of holomorphic functions defined on the unit disk. It gives a lower bound on the size of a disk in which an inverse to a holomorphic function exists. It is named ...
?
* How are suspended infinite-infinitesimals paradoxes justified?
* Regularity of solutions of Euler equations
* Convergence of Flint Hills series
In mathematics, a series is, roughly speaking, a description of the operation of addition, adding infinitely many quantities, one after the other, to a given starting quantity. The study of series is a major part of calculus and its generalizat ...
* Regularity of solutions of Vlasov–Maxwell equations The Vlasov equation is a differential equation describing time evolution of the distribution function of plasma consisting of charged particles with long-range interaction, e.g. Coulomb. The equation was first suggested for description of plasma by ...
Combinatorics
* The 1/3–2/3 conjecture
In order theory, a branch of mathematics, the 1/3–2/3 conjecture states that, if one is comparison sorting a set of items then, no matter what comparisons may have already been performed, it is always possible to choose the next comparison in su ...
– does every finite partially ordered set
In mathematics, especially order theory, a partially ordered set (also poset) formalizes and generalizes the intuitive concept of an ordering, sequencing, or arrangement of the elements of a set. A poset consists of a set together with a binar ...
that is not totally ordered contain two elements ''x'' and ''y'' such that the probability that ''x'' appears before ''y'' in a random linear extension
In order theory, a branch of mathematics, a linear extension of a partial order is a total order (or linear order) that is compatible with the partial order. As a classic example, the lexicographic order of totally ordered sets is a linear ext ...
is between 1/3 and 2/3?
* The Dittert conjecture The Dittert conjecture, or Dittert–Hajek conjecture, is a mathematical hypothesis (in combinatorics) concerning the maximum achieved by a particular function \phi of matrices with real, nonnegative entries satisfying a summation condition. The con ...
concerning the maximum achieved by a particular function of matrices with real, nonnegative entries satisfying a summation condition
* Problems in Latin squares – open questions concerning Latin squares
* The lonely runner conjecture
In number theory, specifically the study of Diophantine approximation, the lonely runner conjecture is a conjecture about the long-term behavior of runners on a circular track. It states that n runners on a track of unit length, with constant spe ...
– if runners with pairwise distinct speeds run round a track of unit length, will every runner be "lonely" (that is, be at least a distance from each other runner) at some time?
* No-three-in-line problem – how many points can be placed in the grid so that no three of them lie on a line?
* Rudin's conjecture Rudin's conjecture is a mathematical hypothesis (in additive combinatorics and elementary number theory
Number theory (or arithmetic or higher arithmetic in older usage) is a branch of pure mathematics devoted primarily to the study of the int ...
on the number of squares in finite arithmetic progression
An arithmetic progression or arithmetic sequence () is a sequence of numbers such that the difference between the consecutive terms is constant. For instance, the sequence 5, 7, 9, 11, 13, 15, . . . is an arithmetic progression with a common differ ...
s
* The sunflower conjecture
The common sunflower (''Helianthus annuus'') is a large annual forb of the genus ''Helianthus'' grown as a crop for its edible oily seeds. Apart from cooking oil production, it is also used as livestock forage (as a meal or a silage plant), a ...
: can the number of size sets required for the existence of a sunflower of sets be bounded by an exponential function in for every fixed ?
* Frankl's union-closed sets conjecture – for any family of sets closed under sums there exists an element (of the underlying space) belonging to half or more of the sets
* Give a combinatorial interpretation of the Kronecker coefficients
* The values of the Dedekind numbers for
* The values of the Ramsey numbers, particularly
* The values of the Van der Waerden numbers
* Finding a function to model n-step self-avoiding walk
In mathematics, a self-avoiding walk (SAW) is a sequence of moves on a lattice (a lattice path) that does not visit the same point more than once. This is a special case of the graph theoretical notion of a path. A self-avoiding polygon (S ...
s
Dynamical systems
 * Arnold–Givental conjecture and Arnold conjecture – relating symplectic geometry to Morse theory.
* Berry–Tabor conjecture in
* Arnold–Givental conjecture and Arnold conjecture – relating symplectic geometry to Morse theory.
* Berry–Tabor conjecture in quantum chaos
Quantum chaos is a branch of physics which studies how chaotic classical dynamical systems can be described in terms of quantum theory. The primary question that quantum chaos seeks to answer is: "What is the relationship between quantum mech ...
* Banach's problem – is there an ergodic system with simple Lebesgue spectrum?
* Birkhoff conjecture – if a billiard table
A billiard table or billiards table is a bounded table on which cue sports are played. In the modern era, all billiards tables (whether for carom billiards, pool, pyramid or snooker) provide a flat surface usually made of quarried slate, ...
is strictly convex and integrable, is its boundary necessarily an ellipse?
* Collatz conjecture
The Collatz conjecture is one of the most famous unsolved problems in mathematics. The conjecture asks whether repeating two simple arithmetic operations will eventually transform every positive integer into 1. It concerns sequences of intege ...
(''aka'' the conjecture)
* Eden's conjecture that the supremum
In mathematics, the infimum (abbreviated inf; plural infima) of a subset S of a partially ordered set P is a greatest element in P that is less than or equal to each element of S, if such an element exists. Consequently, the term ''greatest l ...
of the local Lyapunov dimension In the mathematics of dynamical systems, the concept of Lyapunov dimension was suggested by Kaplan and Yorke for estimating the Hausdorff dimension of attractors.
Further the concept has been developed and rigorously justified in a number of paper ...
s on the global attractor
In the mathematical field of dynamical systems, an attractor is a set of states toward which a system tends to evolve, for a wide variety of starting conditions of the system. System values that get close enough to the attractor values remain ...
is achieved on a stationary point or an unstable periodic orbit embedded into the attractor.
* Eremenko's conjecture: every component of the escaping set of an entire transcendental
Transcendence, transcendent, or transcendental may refer to:
Mathematics
* Transcendental number, a number that is not the root of any polynomial with rational coefficients
* Algebraic element or transcendental element, an element of a field exten ...
function is unbounded.
* Fatou conjecture In mathematics, the Fatou conjecture, named after Pierre Fatou, states that a quadratic family of maps from the complex plane to itself is hyperbolic for an open dense set of parameters.
References
*
Dynamical systems
Conjectures
{{math-stub ...
that a quadratic family of maps from the complex plane
In mathematics, the complex plane is the plane formed by the complex numbers, with a Cartesian coordinate system such that the -axis, called the real axis, is formed by the real numbers, and the -axis, called the imaginary axis, is formed by th ...
to itself is hyperbolic for an open dense set of parameters.
* Furstenberg conjecture – is every invariant and ergodic
In mathematics, ergodicity expresses the idea that a point of a moving system, either a dynamical system or a stochastic process, will eventually visit all parts of the space that the system moves in, in a uniform and random sense. This implies t ...
measure for the action on the circle either Lebesgue or atomic?
* Kaplan–Yorke conjecture on the dimension of an attractor
In the mathematical field of dynamical systems, an attractor is a set of states toward which a system tends to evolve, for a wide variety of starting conditions of the system. System values that get close enough to the attractor values remain ...
in terms of its Lyapunov exponent
In mathematics, the Lyapunov exponent or Lyapunov characteristic exponent of a dynamical system is a quantity that characterizes the rate of separation of infinitesimally close trajectories. Quantitatively, two trajectories in phase space with in ...
s
* Margulis Margulis is a surname that, like its variants, is derived from the Ashkenazi Hebrew pronunciation of the Hebrew word (Israeli Hebrew ), meaning 'pearl.' Notable people and characters with the name include:
* Berl Broder (born Margulis), Broder sing ...
conjecture – measure classification for diagonalizable actions in higher-rank groups.
* MLC conjecture – is the Mandelbrot set locally connected?
* Many problems concerning an outer billiard, for example showing that outer billiards relative to almost every convex polygon have unbounded orbits.
* Quantum unique ergodicity conjecture on the distribution of large-frequency eigenfunction
In mathematics, an eigenfunction of a linear operator ''D'' defined on some function space is any non-zero function f in that space that, when acted upon by ''D'', is only multiplied by some scaling factor called an eigenvalue. As an equation, ...
s of the Laplacian
In mathematics, the Laplace operator or Laplacian is a differential operator given by the divergence of the gradient of a scalar function on Euclidean space. It is usually denoted by the symbols \nabla\cdot\nabla, \nabla^2 (where \nabla is ...
on a negatively-curved manifold
In mathematics, a manifold is a topological space that locally resembles Euclidean space near each point. More precisely, an n-dimensional manifold, or ''n-manifold'' for short, is a topological space with the property that each point has a ...
* Rokhlin's multiple mixing problem – are all strongly mixing systems also strongly 3-mixing?
* Weinstein conjecture In mathematics, the Weinstein conjecture refers to a general existence problem for periodic orbits of Hamiltonian or Reeb vector flows. More specifically, the conjecture claims that on a compact contact manifold, its Reeb vector field should carry ...
– does a regular compact contact type
In mathematics, more precisely in symplectic geometry, a hypersurface \Sigma of a symplectic manifold (M,\omega) is said to be of contact type if there is 1-form \alpha such that j^(\omega)=d\alpha and (\Sigma,\alpha) is a contact manifold, where ...
level set
In mathematics, a level set of a real-valued function of real variables is a set where the function takes on a given constant value , that is:
: L_c(f) = \left\~,
When the number of independent variables is two, a level set is cal ...
of a Hamiltonian on a symplectic manifold
In differential geometry, a subject of mathematics, a symplectic manifold is a smooth manifold, M , equipped with a closed nondegenerate differential 2-form \omega , called the symplectic form. The study of symplectic manifolds is called s ...
carry at least one periodic orbit of the Hamiltonian flow?
* Does every positive integer generate a juggler sequence terminating at 1?
* Lyapunov function: Lyapunov's second method for stability – For what classes of ODEs
Odes may refer to:
*The plural of ode, a type of poem
* ''Odes'' (Horace), a collection of poems by the Roman author Horace, circa 23 BCE
* Odes of Solomon, a pseudepigraphic book of the Bible
* Book of Odes (Bible), a Deuterocanonical book of ...
, describing dynamical systems, does the Lyapunov’s second method formulated in the classical and canonically generalized forms define the necessary and sufficient conditions for the (asymptotical) stability of motion?
* Is every reversible cellular automaton in three or more dimensions locally reversible?
Games and puzzles
Combinatorial games
* Is there a non-terminating game of beggar-my-neighbour
Beggar-my-neighbour, also known as Strip Jack naked, Beat your neighbour out of doors, or Beat Jack out of doors, or Beat Your Neighbour is a simple card game. It is somewhat similar in nature to the children's card game War, and has spawned a ...
?
* Sudoku
Sudoku (; ja, 数独, sūdoku, digit-single; originally called Number Place) is a logic-based, combinatorics, combinatorial number-placement puzzle. In classic Sudoku, the objective is to fill a 9 × 9 grid with digits so that each co ...
:
** How many puzzles have exactly one solution?Tic-tac-toe variants
Tic-tac-toe is an instance of an m,n,k-game, where two players alternate taking turns on an ''m''×''n'' board until one of them gets ''k'' in a row. Harary's generalized tic-tac-toe is an even broader generalization. The game can also be gene ...
:
**Given a width of tic-tac-toe board, what is the smallest dimension such that X is guaranteed a winning strategy?
* What is the Turing completeness
In computability theory, a system of data-manipulation rules (such as a computer's instruction set, a programming language, or a cellular automaton) is said to be Turing-complete or computationally universal if it can be used to simulate any Tu ...
status of all unique elementary cellular automata?
Games with imperfect information
* Rendezvous problem
The rendezvous dilemma is a logical dilemma, typically formulated in this way:
:Two people have a date in a park they have never been to before. Arriving separately in the park, they are both surprised to discover that it is a huge area and conseq ...
Geometry
Algebraic geometry
* Abundance conjecture: if the canonical bundle In mathematics, the canonical bundle of a non-singular algebraic variety V of dimension n over a field is the line bundle \,\!\Omega^n = \omega, which is the ''n''th exterior power of the cotangent bundle Ω on ''V''.
Over the complex numbers, ...
of a projective variety
In algebraic geometry, a projective variety over an algebraically closed field ''k'' is a subset of some projective ''n''-space \mathbb^n over ''k'' that is the zero-locus of some finite family of homogeneous polynomials of ''n'' + 1 variables ...
with Kawamata log terminal singularities is nef
Nef or NEF may refer to:
Businesses and organizations
* National Energy Foundation, a British charity
* National Enrichment Facility, an American uranium enrichment plant
* New Economics Foundation, a British think-tank
* Near East Foundation, ...
, then it is semiample.
* Bass conjecture In mathematics, especially algebraic geometry, the Bass conjecture says that certain algebraic ''K''-groups are supposed to be finitely generated. The conjecture was proposed by Hyman Bass.
Statement of the conjecture
Any of the following equivale ...
on the finite generation of certain algebraic K-groups.
* Deligne conjecture: any one of numerous named for Pierre Deligne
Pierre René, Viscount Deligne (; born 3 October 1944) is a Belgian mathematician. He is best known for work on the Weil conjectures, leading to a complete proof in 1973. He is the winner of the 2013 Abel Prize, 2008 Wolf Prize, 1988 Crafoord Pr ...
.
* Dixmier conjecture
In algebra the Dixmier conjecture, asked by Jacques Dixmier in 1968, is the conjecture that any endomorphism of a Weyl algebra is an automorphism.
Tsuchimoto in 2005, and independently Belov-Kanel and Kontsevich in 2007, showed that the Dixm ...
: any endomorphism
In mathematics, an endomorphism is a morphism from a mathematical object to itself. An endomorphism that is also an isomorphism is an automorphism. For example, an endomorphism of a vector space is a linear map , and an endomorphism of a ...
of a Weyl algebra is an automorphism.
* Fröberg conjecture In algebraic geometry, the Fröberg conjecture is a conjecture about the possible Hilbert function
In commutative algebra, the Hilbert function, the Hilbert polynomial, and the Hilbert series of a graded commutative algebra finitely generated over ...
on the Hilbert functions of a set of forms.
* Fujita conjecture regarding the line bundle constructed from a positive
Positive is a property of positivity and may refer to:
Mathematics and science
* Positive formula, a logical formula not containing negation
* Positive number, a number that is greater than 0
* Plus sign, the sign "+" used to indicate a posi ...
holomorphic line bundle In mathematics, a holomorphic vector bundle is a complex vector bundle over a complex manifold such that the total space is a complex manifold and the projection map is holomorphic. Fundamental examples are the holomorphic tangent bundle of ...
on a compact
Compact as used in politics may refer broadly to a pact or treaty; in more specific cases it may refer to:
* Interstate compact
* Blood compact, an ancient ritual of the Philippines
* Compact government, a type of colonial rule utilized in British ...
complex manifold
In differential geometry and complex geometry, a complex manifold is a manifold with an atlas of charts to the open unit disc in \mathbb^n, such that the transition maps are holomorphic.
The term complex manifold is variously used to mean a ...
and the canonical line bundle of
* Hartshorne's conjectures
* Jacobian conjecture: if a polynomial mapping over a characteristic-0 field has a constant nonzero Jacobian determinant, then it has a regular
The term regular can mean normal or in accordance with rules. It may refer to:
People
* Moses Regular (born 1971), America football player
Arts, entertainment, and media Music
* "Regular" (Badfinger song)
* Regular tunings of stringed instrum ...
(i.e. with polynomial components) inverse function.
* Manin conjecture on the distribution of rational point
In number theory and algebraic geometry, a rational point of an algebraic variety is a point whose coordinates belong to a given field. If the field is not mentioned, the field of rational numbers is generally understood. If the field is the fie ...
s of bounded height
Height is measure of vertical distance, either vertical extent (how "tall" something or someone is) or vertical position (how "high" a point is).
For example, "The height of that building is 50 m" or "The height of an airplane in-flight is ab ...
in certain subsets of Fano varieties
* Maulik–Nekrasov–Okounkov–Pandharipande conjecture on an equivalence between Gromov–Witten theory and Donaldson–Thomas theory
* Nagata's conjecture on curves
In mathematics, the Nagata conjecture on curves, named after Masayoshi Nagata, governs the minimal degree required for a plane algebraic curve to pass through a collection of very general points with prescribed multiplicities.
History
Nagata arri ...
, specifically the minimal degree required for a plane algebraic curve
In mathematics, an affine algebraic plane curve is the zero set of a polynomial in two variables. A projective algebraic plane curve is the zero set in a projective plane of a homogeneous polynomial in three variables. An affine algebraic plane ...
to pass through a collection of very general points with prescribed multiplicities.
* Nagata–Biran conjecture In mathematics, the Nagata–Biran conjecture, named after Masayoshi Nagata and Paul Biran, is a generalisation of Nagata's conjecture on curves to arbitrary polarised surfaces.
Statement
Let ''X'' be a smooth algebraic surface and ''L'' be an am ...
that if is a smooth algebraic surface and is an ample line bundle on of degree , then for sufficiently large , the Seshadri constant satisfies .
* Nakai conjecture
In mathematics, the Nakai conjecture is an unproven characterization of smooth algebraic varieties, conjectured by Japanese mathematician Yoshikazu Nakai in 1961.
It states that if ''V'' is a complex algebraic variety, such that its ring of differ ...
: if a complex algebraic variety
In algebraic geometry, a complex algebraic variety is an algebraic variety (in the scheme sense or otherwise) over the field of complex numbers. Parshin, Alexei N., and Igor Rostislavovich Shafarevich, eds. ''Algebraic Geometry III: Complex Algebr ...
has a ring of differential operators generated by its contained derivations, then it must be smooth.
* Parshin's conjecture In mathematics, more specifically in algebraic geometry, Parshin's conjecture (also referred to as the Beilinson–Parshin conjecture) states that for any smooth morphism, smooth projective variety ''X'' defined over a finite field, the higher algeb ...
: the higher algebraic K-groups of any smooth projective variety
In algebraic geometry, a projective variety over an algebraically closed field ''k'' is a subset of some projective ''n''-space \mathbb^n over ''k'' that is the zero-locus of some finite family of homogeneous polynomials of ''n'' + 1 variables ...
defined over a finite field
In mathematics, a finite field or Galois field (so-named in honor of Évariste Galois) is a field that contains a finite number of elements. As with any field, a finite field is a set on which the operations of multiplication, addition, subt ...
must vanish up to torsion.
* Section conjecture on splittings of group homomorphism
In mathematics, given two groups, (''G'', ∗) and (''H'', ·), a group homomorphism from (''G'', ∗) to (''H'', ·) is a function ''h'' : ''G'' → ''H'' such that for all ''u'' and ''v'' in ''G'' it holds that
: h(u*v) = h(u) \cdot h(v)
...
s from fundamental group
In the mathematical field of algebraic topology, the fundamental group of a topological space is the group of the equivalence classes under homotopy of the loops contained in the space. It records information about the basic shape, or holes, of ...
s of complete smooth curves over finitely-generated fields to the Galois group
In mathematics, in the area of abstract algebra known as Galois theory, the Galois group of a certain type of field extension is a specific group associated with the field extension. The study of field extensions and their relationship to the po ...
of .
* Standard conjectures In mathematics, the standard conjectures about algebraic cycles are several conjectures describing the relationship of algebraic cycles and Weil cohomology theory, Weil cohomology theories. One of the original applications of these conjectures, envi ...
on algebraic cycles
* Tate conjecture on the connection between algebraic cycle In mathematics, an algebraic cycle on an algebraic variety ''V'' is a formal linear combination of subvarieties of ''V''. These are the part of the algebraic topology of ''V'' that is directly accessible by algebraic methods. Understanding the al ...
s on algebraic varieties
Algebraic varieties are the central objects of study in algebraic geometry, a sub-field of mathematics. Classically, an algebraic variety is defined as the set of solutions of a system of polynomial equations over the real or complex number
...
and Galois representations
In mathematics, a Galois module is a ''G''-module, with ''G'' being the Galois group of some extension of fields. The term Galois representation is frequently used when the ''G''-module is a vector space over a field or a free module over a ring i ...
on étale cohomology groups.
* Virasoro conjecture: a certain generating function
In mathematics, a generating function is a way of encoding an infinite sequence of numbers () by treating them as the coefficients of a formal power series. This series is called the generating function of the sequence. Unlike an ordinary ser ...
encoding the Gromov–Witten invariant
In mathematics, specifically in symplectic topology and algebraic geometry, Gromov–Witten (GW) invariants are rational numbers that, in certain situations, count pseudoholomorphic curves meeting prescribed conditions in a given symplectic man ...
s of a smooth projective variety
In algebraic geometry, a projective variety over an algebraically closed field ''k'' is a subset of some projective ''n''-space \mathbb^n over ''k'' that is the zero-locus of some finite family of homogeneous polynomials of ''n'' + 1 variables ...
is fixed by an action of half of the Virasoro algebra
In mathematics, the Virasoro algebra (named after the physicist Miguel Ángel Virasoro) is a complex Lie algebra and the unique central extension of the Witt algebra. It is widely used in two-dimensional conformal field theory and in string t ...
.
* Zariski multiplicity conjecture on the topological equisingularity and equimultiplicity of varieties at singular points
* Are infinite sequences of flips possible in dimensions greater than 3?
* Resolution of singularities
In algebraic geometry, the problem of resolution of singularities asks whether every algebraic variety ''V'' has a resolution, a non-singular variety ''W'' with a proper birational map ''W''→''V''. For varieties over fields of characteri ...
in characteristic
Covering and packing
* Borsuk's problem on upper and lower bounds for the number of smaller-diameter subsets needed to cover a bounded
Boundedness or bounded may refer to:
Economics
* Bounded rationality, the idea that human rationality in decision-making is bounded by the available information, the cognitive limitations, and the time available to make the decision
* Bounded e ...
''n''-dimensional set.
* The covering problem of Rado: if the union of finitely many axis-parallel squares has unit area, how small can the largest area covered by a disjoint subset of squares be?
* The Erdős–Oler conjecture: when is a triangular number
A triangular number or triangle number counts objects arranged in an equilateral triangle. Triangular numbers are a type of figurate number, other examples being square numbers and cube numbers. The th triangular number is the number of dots i ...
, packing circles in an equilateral triangle requires a triangle of the same size as packing circles
* The kissing number problem for dimensions other than 1, 2, 3, 4, 8 and 24
* Reinhardt's conjecture
The smoothed octagon is a region in the plane found by Karl Reinhardt in 1934 and conjectured by him to have the ''lowest'' maximum packing density of the plane of all centrally symmetric convex shapes. It was also independently discovered by K ...
: the smoothed octagon has the lowest maximum packing density of all centrally-symmetric convex plane sets
* Sphere packing
In geometry, a sphere packing is an arrangement of non-overlapping spheres within a containing space. The spheres considered are usually all of identical size, and the space is usually three- dimensional Euclidean space. However, sphere pack ...
problems, including the density of the densest packing in dimensions other than 1, 2, 3, 8 and 24, and its asymptotic behavior for high dimensions.
* Square packing in a square: what is the asymptotic growth rate of wasted space?
* Ulam's packing conjecture about the identity of the worst-packing convex solid
Differential geometry
* The spherical Bernstein's problem, a generalization of Bernstein's problem
* Carathéodory conjecture: any convex, closed, and twice-differentiable surface in three-dimensional Euclidean space
Euclidean space is the fundamental space of geometry, intended to represent physical space. Originally, that is, in Euclid's ''Elements'', it was the three-dimensional space of Euclidean geometry, but in modern mathematics there are Euclidean sp ...
admits at least two umbilical points.
* Cartan–Hadamard conjecture: can the classical isoperimetric inequality
In mathematics, the isoperimetric inequality is a geometric inequality involving the perimeter of a set and its volume. In n-dimensional space \R^n the inequality lower bounds the surface area or perimeter \operatorname(S) of a set S\subset\R^n ...
for subsets of Euclidean space be extended to spaces of nonpositive curvature, known as Cartan–Hadamard manifolds?
* Chern's conjecture (affine geometry) Chern's conjecture for affinely flat manifolds was proposed by Shiing-Shen Chern in 1955 in the field of affine geometry. As of 2018, it remains an unsolved mathematical problem.
Chern's conjecture states that the Euler characteristic of a compact ...
that the Euler characteristic
In mathematics, and more specifically in algebraic topology and polyhedral combinatorics, the Euler characteristic (or Euler number, or Euler–Poincaré characteristic) is a topological invariant, a number that describes a topological spac ...
of a compact
Compact as used in politics may refer broadly to a pact or treaty; in more specific cases it may refer to:
* Interstate compact
* Blood compact, an ancient ritual of the Philippines
* Compact government, a type of colonial rule utilized in British ...
affine manifold vanishes.
* Chern's conjecture for hypersurfaces in spheres, a number of closely-related conjectures.
* Closed curve problem: find (explicit) necessary and sufficient conditions that determine when, given two periodic functions with the same period, the integral curve is closed.
* The filling area conjecture, that a hemisphere has the minimum area among shortcut-free surfaces in Euclidean space whose boundary forms a closed curve of given length
* The Hopf conjectures relating the curvature and Euler characteristic of higher-dimensional Riemannian manifolds
* Yau's conjecture: a closed Riemannian 3-manifold
In mathematics, a 3-manifold is a space that locally looks like Euclidean 3-dimensional space. A 3-manifold can be thought of as a possible shape of the universe. Just as a sphere looks like a plane to a small enough observer, all 3-manifolds ...
has an infinite number of smooth closed immersed minimal surfaces.
* Yau's conjecture on the first eigenvalue that the first eigenvalue
In linear algebra, an eigenvector () or characteristic vector of a linear transformation is a nonzero vector that changes at most by a scalar factor when that linear transformation is applied to it. The corresponding eigenvalue, often denot ...
for the Laplace–Beltrami operator
In differential geometry, the Laplace–Beltrami operator is a generalization of the Laplace operator to functions defined on submanifolds in Euclidean space and, even more generally, on Riemannian and pseudo-Riemannian manifolds. It is named ...
on an embedded minimal hypersurface of is .
Discrete geometry
 * The Hadwiger conjecture on covering ''n''-dimensional convex bodies with at most 2''n'' smaller copies
* Solving the
* The Hadwiger conjecture on covering ''n''-dimensional convex bodies with at most 2''n'' smaller copies
* Solving the happy ending problem
In mathematics, the "happy ending problem" (so named by Paul Erdős because it led to the marriage of George Szekeres and Esther Klein) is the following statement:
This was one of the original results that led to the development of Ramsey t ...
for arbitrary
*Improving lower and upper bounds for the Heilbronn triangle problem.
* Kalai's 3''d'' conjecture on the least possible number of faces of centrally symmetric polytopes.[.]
* The Kobon triangle problem
The Kobon triangle problem is an unsolved problem in combinatorial geometry first stated by Kobon Fujimura (1903-1983). The problem asks for the largest number ''N''(''k'') of nonoverlapping triangles whose sides lie on an arrangement of ''k'' ...
on triangles in line arrangements
* The Kusner conjecture: at most points can be equidistant in spaces
* The McMullen problem
The McMullen problem is an open problem in discrete geometry named after Peter McMullen.
Statement
In 1972, David G. Larman wrote about the following problem:
Larman credited the problem to a private communication by Peter McMullen.
Equivalent f ...
on projectively transforming sets of points into convex position
*Opaque forest problem
In discrete geometry, an opaque set is a system of curves or other set in the plane that blocks all lines of sight across a polygon, circle, or other shape. Opaque sets have also been called barriers, beam detectors, opaque covers, or (in cases ...
on finding opaque set
In discrete geometry, an opaque set is a system of curves or other set in the plane that blocks all lines of sight across a polygon, circle, or other shape. Opaque sets have also been called barriers, beam detectors, opaque covers, or (in cases ...
s for various planar shapes
* How many unit distances can be determined by a set of points in the Euclidean plane?
* Finding matching upper and lower bounds for ''k''-sets and halving lines
* Tripod packing: how many tripods can have their apexes packed into a given cube?
Euclidean geometry
* The Atiyah conjecture on configurations on the invertibility of a certain -by- matrix depending on points in
* Bellman's lost in a forest problem – find the shortest route that is guaranteed to reach the boundary of a given shape, starting at an unknown point of the shape with unknown orientation
* Borromean rings
In mathematics, the Borromean rings are three simple closed curves in three-dimensional space that are topologically linked and cannot be separated from each other, but that break apart into two unknotted and unlinked loops when any one of the ...
— are there three unknotted space curves, not all three circles, which cannot be arranged to form this link?
* Danzer's problem and Conway's dead fly problem – do Danzer sets of bounded density or bounded separation exist?
* Dissection into orthoschemes – is it possible for simplices of every dimension?
* Ehrhart's volume conjecture: a convex body in dimensions containing a single lattice point in its interior as its center of mass cannot have volume greater than
* The – does there exist a two-dimensional shape that forms the prototile for an aperiodic tiling
An aperiodic tiling is a non-periodic tiling with the additional property that it does not contain arbitrarily large periodic regions or patches. A set of tile-types (or prototiles) is aperiodic if copies of these tiles can form only non- peri ...
, but not for any periodic tiling?
* Falconer's conjecture In geometric measure theory, Falconer's conjecture, named after Kenneth Falconer, is an unsolved problem concerning the sets of Euclidean distances between points in compact d-dimensional spaces. Intuitively, it states that a set of points that is ...
: sets of Hausdorff dimension greater than in must have a distance set of nonzero Lebesgue measure
In measure theory, a branch of mathematics, the Lebesgue measure, named after French mathematician Henri Lebesgue, is the standard way of assigning a measure to subsets of ''n''-dimensional Euclidean space. For ''n'' = 1, 2, or 3, it coincides ...
* The values of the Hermite constants for dimensions other than 1–8 and 24
* Inscribed square problem, also known as Toeplitz' conjecture and the square peg problem – does every Jordan curve
In mathematics, a curve (also called a curved line in older texts) is an object similar to a line, but that does not have to be straight.
Intuitively, a curve may be thought of as the trace left by a moving point. This is the definition tha ...
have an inscribed square?Hausdorff dimension
In mathematics, Hausdorff dimension is a measure of ''roughness'', or more specifically, fractal dimension, that was first introduced in 1918 by mathematician Felix Hausdorff. For instance, the Hausdorff dimension of a single point is zero, o ...
and Minkowski dimension equal to ?
* The Kelvin problem on minimum-surface-area partitions of space into equal-volume cells, and the optimality of the Weaire–Phelan structure
In geometry, the Weaire–Phelan structure is a three-dimensional structure representing an idealised foam of equal-sized bubbles, with two different shapes. In 1993, Denis Weaire and Robert Phelan found that this structure was a better solution ...
as a solution to the Kelvin problem
* Lebesgue's universal covering problem
Lebesgue's universal covering problem is an unsolved problem in geometry that asks for the convex shape of smallest area that can cover every planar set of diameter one. The diameter of a set by definition is the least upper bound of the distance ...
on the minimum-area convex shape in the plane that can cover any shape of diameter one
* Mahler's conjecture on the product of the volumes of a centrally symmetric convex body
In mathematics, a convex body in n-dimensional Euclidean space \R^n is a compact convex set with non- empty interior.
A convex body K is called symmetric if it is centrally symmetric with respect to the origin; that is to say, a point x lies in ...
and its polar.
* Moser's worm problem – what is the smallest area of a shape that can cover every unit-length curve in the plane?
* The moving sofa problem – what is the largest area of a shape that can be maneuvered through a unit-width L-shaped corridor?
* Does every convex polyhedron have Rupert's property?convex polyhedron
A convex polytope is a special case of a polytope, having the additional property that it is also a convex set contained in the n-dimensional Euclidean space \mathbb^n. Most texts. use the term "polytope" for a bounded convex polytope, and the w ...
have a net, or simple edge-unfolding?
* Is there a non-convex polyhedron without self-intersections with more than seven faces, all of which share an edge with each other?
* The Thomson problem – what is the minimum energy configuration of mutually-repelling particles on a unit sphere?
* Convex uniform 5-polytopes – find and classify the complete set of these shapes
Graph theory
Graph coloring and labeling
 * Cereceda's conjecture on the diameter of the space of colorings of degenerate graphs
* The
* Cereceda's conjecture on the diameter of the space of colorings of degenerate graphs
* The Erdős–Faber–Lovász conjecture
In graph theory, the Erdős–Faber–Lovász conjecture is a problem about graph coloring, named after Paul Erdős, Vance Faber, and László Lovász, who formulated it in 1972.. It says:
:If complete graphs, each having exactly vertices, have ...
on coloring unions of cliques
* The Gyárfás–Sumner conjecture
In graph theory, the Gyárfás–Sumner conjecture asks whether, for every tree T and complete graph K, the graphs with neither T nor K as induced subgraphs can be properly colored using only a constant number of colors. Equivalently, it asks whet ...
on χ-boundedness of graphs with a forbidden induced tree
* The Hadwiger conjecture relating coloring to clique minors
* The Hadwiger–Nelson problem
In geometric graph theory, the Hadwiger–Nelson problem, named after Hugo Hadwiger and Edward Nelson, asks for the minimum number of colors required to color the plane such that no two points at distance 1 from each other have the same color ...
on the chromatic number of unit distance graphs
* Jaeger's Petersen-coloring conjecture: every bridgeless cubic graph has a cycle-continuous mapping to the Petersen graph
* The list coloring conjecture: for every graph, the list chromatic index equals the chromatic index
* The total coloring conjecture of Behzad and Vizing that the total chromatic number is at most two plus the maximum degree
Graph drawing
* The Albertson conjecture: the crossing number can be lower-bounded by the crossing number of a complete graph
In the mathematical field of graph theory, a complete graph is a simple undirected graph in which every pair of distinct vertices is connected by a unique edge. A complete digraph is a directed graph in which every pair of distinct vertices ...
with the same chromatic number
In graph theory, graph coloring is a special case of graph labeling; it is an assignment of labels traditionally called "colors" to elements of a graph subject to certain constraints. In its simplest form, it is a way of coloring the vertices o ...
* Conway's thrackle conjecture A thrackle is an embedding of a graph in the plane, such that each edge is a Jordan arc
and every pair of edges meet exactly once. Edges may either meet at a common endpoint, or, if they have no endpoints in common, at a point in their interiors. ...
that thrackle A thrackle is an embedding of a graph in the plane, such that each edge is a Jordan arc
and every pair of edges meet exactly once. Edges may either meet at a common endpoint, or, if they have no endpoints in common, at a point in their interiors. ...
s cannot have more edges than vertices
* Harborth's conjecture: every planar graph can be drawn with integer edge lengths
* Negami's conjecture
In graph theory, a planar cover of a finite graph ''G'' is a finite covering graph of ''G'' that is itself a planar graph. Every graph that can be embedded into the projective plane has a planar cover; an unsolved conjecture of Seiya Negami state ...
on projective-plane embeddings of graphs with planar covers
* The strong Papadimitriou–Ratajczak conjecture: every polyhedral graph has a convex greedy embedding
* Turán's brick factory problem
In the mathematics of graph drawing, Turán's brick factory problem asks for the minimum number of crossings in a drawing of a complete bipartite graph. The problem is named after Pál Turán, who formulated it while being forced to work in a ...
– Is there a drawing of any complete bipartite graph with fewer crossings than the number given by Zarankiewicz?
* Universal point sets of subquadratic size for planar graphs
Paths and cycles in graphs
* Barnette's conjecture
Barnette's conjecture is an unsolved problem in graph theory, a branch of mathematics, concerning Hamiltonian cycles in graphs. It is named after David W. Barnette, a professor emeritus at the University of California, Davis; it states that ev ...
: every cubic bipartite three-connected planar graph has a Hamiltonian cycle
* Gilbert–Pollack conjecture on the Steiner ratio of the Euclidean plane that the Steiner ratio is
* Chvátal's toughness conjecture, that there is a number such that every -tough graph is Hamiltonian
* The cycle double cover conjecture
In graph-theoretic mathematics, a cycle double cover is a collection of cycles in an undirected graph that together include each edge of the graph exactly twice. For instance, for any polyhedral graph, the faces of a convex polyhedron that repre ...
: every bridgeless graph has a family of cycles that includes each edge twice
* The Erdős–Gyárfás conjecture on cycles with power-of-two lengths in cubic graphs
* The linear arboricity
In graph theory, a branch of mathematics, the linear arboricity of an undirected graph is the smallest number of linear forests its edges can be partitioned into. Here, a linear forest is an acyclic graph with maximum degree two; that is, it is a ...
conjecture on decomposing graphs into disjoint unions of paths according to their maximum degree
* The Lovász conjecture on Hamiltonian paths in symmetric graphs
* The Oberwolfach problem
The Oberwolfach problem is an unsolved problem in mathematics that may be formulated either as a problem of scheduling seating assignments for diners,
or more abstractly as a problem in graph theory, on the edge cycle covers of complete graphs. ...
on which 2-regular graphs have the property that a complete graph on the same number of vertices can be decomposed into edge-disjoint copies of the given graph.
* Szymanski's conjecture
In mathematics, Szymanski's conjecture, named after , states that every permutation
In mathematics, a permutation of a set is, loosely speaking, an arrangement of its members into a sequence or linear order, or if the set is already ordered, a ...
: every permutation
In mathematics, a permutation of a set is, loosely speaking, an arrangement of its members into a sequence or linear order, or if the set is already ordered, a rearrangement of its elements. The word "permutation" also refers to the act or p ...
on the -dimensional doubly-directed
Director may refer to:
Literature
* ''Director'' (magazine), a British magazine
* ''The Director'' (novel), a 1971 novel by Henry Denker
* ''The Director'' (play), a 2000 play by Nancy Hasty
Music
* Director (band), an Irish rock band
* ''D ...
hypercube graph
In graph theory, the hypercube graph is the graph formed from the vertices and edges of an -dimensional hypercube. For instance, the cube graph is the graph formed by the 8 vertices and 12 edges of a three-dimensional cube.
has vertices, e ...
can be routed with edge-disjoint paths.
Word-representation of graphs
*Are there any graphs on ''n'' vertices whose representation
Representation may refer to:
Law and politics
*Representation (politics), political activities undertaken by elected representatives, as well as other theories
** Representative democracy, type of democracy in which elected officials represent a ...
requires more than floor(''n''/2) copies of each letter?planar graph
In graph theory, a planar graph is a graph that can be embedded in the plane, i.e., it can be drawn on the plane in such a way that its edges intersect only at their endpoints. In other words, it can be drawn in such a way that no edges cro ...
sword-representable graph
In the mathematical field of graph theory, a word-representable graph is a graph that can be characterized by a word (or sequence) whose entries alternate in a prescribed way. In particular, if the vertex set of the graph is ''V'', one should be a ...
s in terms of (induced) forbidden subgraphs.bipartite graph
In the mathematical field of graph theory, a bipartite graph (or bigraph) is a graph whose vertices can be divided into two disjoint and independent sets U and V, that is every edge connects a vertex in U to one in V. Vertex sets U and V ar ...
s, crown graphs require longest word-representants?line graph
In the mathematical discipline of graph theory, the line graph of an undirected graph is another graph that represents the adjacencies between edges of . is constructed in the following way: for each edge in , make a vertex in ; for every ...
of a non- word-representable graph always non- word-representable?
Miscellaneous graph theory
* Babai's problem
Babai's problem is a problem in algebraic graph theory first proposed in 1979 by László Babai.
Babai's problem
Let G be a finite group, let \operatorname(G) be the set of all irreducible characters of G, let \Gamma=\operatorname(G,S) be the ...
: which groups are Babai invariant groups?
* Brouwer's conjecture In the mathematical field of spectral graph theory, Brouwer's conjecture is a conjecture by Andries Brouwer on upper bounds for the intermediate sums of the eigenvalues of the Laplacian
In mathematics, the Laplace operator or Laplacian is a di ...
on upper bounds for sums of eigenvalues
In linear algebra, an eigenvector () or characteristic vector of a linear transformation is a nonzero vector that changes at most by a scalar factor when that linear transformation is applied to it. The corresponding eigenvalue, often denoted b ...
of Laplacians of graphs in terms of their number of edges
* Conway's 99-graph problem: does there exist a strongly regular graph
In graph theory, a strongly regular graph (SRG) is defined as follows. Let be a regular graph with vertices and degree . is said to be strongly regular if there are also integers and such that:
* Every two adjacent vertices have comm ...
with parameters (99,14,1,2)?
* Degree diameter problem: given two positive integers , what is the largest graph of diameter such that all vertices have degrees at most ?
* The Erdős–Hajnal conjecture on large cliques or independent sets in graphs with a forbidden induced subgraph
* The GNRS conjecture
In theoretical computer science and metric geometry, the GNRS conjecture connects the theory of graph minors, the stretch factor of embeddings, and the approximation ratio of multi-commodity flow problems. It is named after Anupam Gupta, Ilan ...
on whether minor-closed graph families have embeddings with bounded distortion
* Graham's pebbling conjecture
Graph pebbling is a mathematical game played on a graph with zero or more pebbles on each of its vertices. 'Game play' is composed of a series of pebbling moves. A pebbling move on a graph consists of choosing a vertex with at least two pebbles, ...
on the pebbling number of Cartesian products of graphs
* The implicit graph conjecture on the existence of implicit representations for slowly-growing hereditary families of graphs
* Jørgensen's conjecture that every 6-vertex-connected ''K''6-minor-free graph is an apex graph
* Meyniel's conjecture that cop number is
* Does a Moore graph with girth 5 and degree 57 exist?
* What is the largest possible pathwidth of an -vertex cubic graph
In the mathematical field of graph theory, a cubic graph is a graph in which all vertices have degree three. In other words, a cubic graph is a 3-regular graph. Cubic graphs are also called trivalent graphs.
A bicubic graph is a cubic bipa ...
?
* The reconstruction conjecture
Informally, the reconstruction conjecture in graph theory says that graphs are determined uniquely by their subgraphs. It is due to KellyKelly, P. J.A congruence theorem for trees ''Pacific J. Math.'' 7 (1957), 961–968. and Ulam.Ulam, S. M. ...
and new digraph reconstruction conjecture
The reconstruction conjecture of Stanisław Ulam is one of the best-known open problems in graph theory. Using the terminology of Frank Harary it can be stated as follows: If ''G'' and ''H'' are two graphs on at least three vertices and ƒ is a b ...
on whether a graph is uniquely determined by its vertex-deleted subgraphs.
* Ryser's conjecture relating the maximum matching size and minimum transversal size in hypergraph
In mathematics, a hypergraph is a generalization of a graph in which an edge can join any number of vertices. In contrast, in an ordinary graph, an edge connects exactly two vertices.
Formally, an undirected hypergraph H is a pair H = (X,E) ...
s
* The second neighborhood problem: does every oriented graph contain a vertex for which there are at least as many other vertices at distance two as at distance one?
* Sidorenko's conjecture on homomorphism densities of graphs in graphons
* Do there exist infinitely many strongly regular geodetic graphs, or any strongly regular geodetic graphs that are not Moore graphs?
* Sumner's conjecture
Sumner's conjecture (also called Sumner's universal tournament conjecture) states that every orientation of every n-vertex tree is a subgraph of every (2n-2)-vertex tournament.
David Sumner, a graph theorist at the University of South Carolina ...
: does every -vertex tournament contain as a subgraph every -vertex oriented tree?
* Tutte's conjectures:
** every bridgeless graph has a nowhere-zero 5-flow
** every Petersen
Petersen is a common Danish patronymic surname, meaning ''"son of Peter"''. There are other spellings. Petersen may refer to:
People In arts and entertainment
* Adolf Dahm-Petersen, Norwegian voice specialist
* Anja Petersen, German operatic ...
- minor-free bridgeless graph has a nowhere-zero 4-flow
* Vizing's conjecture
In graph theory, Vizing's conjecture concerns a relation between the domination number and the cartesian product of graphs.
This conjecture was first stated by , and states that, if denotes the minimum number of vertices in a dominating set fo ...
on the domination number of cartesian products of graphs
* Woodall's conjecture that the minimum number of edges in a dicut of a directed graph
In mathematics, and more specifically in graph theory, a directed graph (or digraph) is a graph that is made up of a set of vertices connected by directed edges, often called arcs.
Definition
In formal terms, a directed graph is an ordered pai ...
is equal to the maximum number of disjoint dijoins
* Zarankiewicz problem
The Zarankiewicz problem, an unsolved problem in mathematics, asks for the largest possible number of edges in a bipartite graph that has a given number of vertices and has no complete bipartite subgraphs of a given size.. Reprint of 1978 Academi ...
: how many edges can there be in a bipartite graph
In the mathematical field of graph theory, a bipartite graph (or bigraph) is a graph whose vertices can be divided into two disjoint and independent sets U and V, that is every edge connects a vertex in U to one in V. Vertex sets U and V ar ...
on a given number of vertices with no complete bipartite subgraphs of a given size?
Group theory
 * Andrews–Curtis conjecture: every balanced
* Andrews–Curtis conjecture: every balanced presentation
A presentation conveys information from a speaker to an audience. Presentations are typically demonstrations, introduction, lecture, or speech meant to inform, persuade, inspire, motivate, build goodwill, or present a new idea/product. Present ...
of the trivial group
In mathematics, a trivial group or zero group is a group consisting of a single element. All such groups are isomorphic, so one often speaks of the trivial group. The single element of the trivial group is the identity element and so it is usual ...
can be transformed into a trivial presentation by a sequence of Nielsen transformations on relators and conjugations of relators
* Guralnick–Thompson conjecture on the composition factors of groups in genus-0 systems
* Herzog–Schönheim conjecture: if a finite system of left coset
In mathematics, specifically group theory, a subgroup of a group may be used to decompose the underlying set of into disjoint, equal-size subsets called cosets. There are ''left cosets'' and ''right cosets''. Cosets (both left and right) ...
s of subgroups of a group form a partition of , then the finite indices of said subgroups cannot be distinct.
* The inverse Galois problem: is every finite group the Galois group of a Galois extension of the rationals?
* Problems in loop theory and quasigroup theory In mathematics, especially abstract algebra, loop (algebra), loop theory and quasigroup theory are active research areas with many open problems. As in other areas of mathematics, such problems are often made public at professional conferences and ...
consider generalizations of groups
* Are there an infinite number of Leinster group
In mathematics, a Leinster group is a finite group whose order (group theory), order equals the sum of the orders of its proper subgroup, proper normal subgroups.
The Leinster groups are named after Tom Leinster, a mathematician at the University ...
s?
* Does generalized moonshine exist?
* For which positive integers ''m'', ''n'' is the free Burnside group finite? In particular, is finite?
* Is every finitely presented periodic group finite?
* Is every group surjunctive
In mathematics, a surjunctive group is a group such that every injective cellular automaton with the group elements as its cells is also surjective. Surjunctive groups were introduced by . It is unknown whether every group is surjunctive.
Definit ...
?
Notebook problems
* The Kourovka Notebook is a collection of unsolved problems in group theory, first published in 1965 and updated many times since.
Model theory and formal languages
* The Cherlin–Zilber conjecture
In model theory, a stable group is a group that is stable in the sense of stability theory.
An important class of examples is provided by groups of finite Morley rank (see below).
Examples
*A group of finite Morley rank is an abstract group ''G' ...
: A simple group whose first-order theory is stable in is a simple algebraic group over an algebraically closed field.
* Generalized star height problem: can all regular language
In theoretical computer science and formal language theory, a regular language (also called a rational language) is a formal language that can be defined by a regular expression, in the strict sense in theoretical computer science (as opposed to ...
s be expressed using generalized regular expressions with limited nesting depths of Kleene star
In mathematical logic and computer science, the Kleene star (or Kleene operator or Kleene closure) is a unary operation, either on sets of strings or on sets of symbols or characters. In mathematics,
it is more commonly known as the free monoid ...
s?
* For which number fields does Hilbert's tenth problem hold?
* Kueker's conjecture
* The main gap conjecture, e.g. for uncountable first order theories, for AECs, and for -saturated models of a countable theory.[Shelah S, ''Classification Theory'', North-Holland, 1990]
* Shelah's categoricity conjecture for : If a sentence is categorical above the Hanf number then it is categorical in all cardinals above the Hanf number.theory
A theory is a rational type of abstract thinking about a phenomenon, or the results of such thinking. The process of contemplative and rational thinking is often associated with such processes as observational study or research. Theories may ...
of the real number
In mathematics, a real number is a number that can be used to measurement, measure a ''continuous'' one-dimensional quantity such as a distance, time, duration or temperature. Here, ''continuous'' means that values can have arbitrarily small var ...
s with the exponential function
The exponential function is a mathematical function denoted by f(x)=\exp(x) or e^x (where the argument is written as an exponent). Unless otherwise specified, the term generally refers to the positive-valued function of a real variable, ...
decidable?
* The universality problem for C-free graphs: For which finite sets C of graphs does the class of C-free countable graphs have a universal member under strong embeddings?
* The universality spectrum problem: Is there a first-order theory whose universality spectrum is minimum?
* Vaught conjecture: the number of countable
In mathematics, a set is countable if either it is finite or it can be made in one to one correspondence with the set of natural numbers. Equivalently, a set is ''countable'' if there exists an injective function from it into the natural number ...
models of a first-order complete theory in a countable language
Language is a structured system of communication. The structure of a language is its grammar and the free components are its vocabulary. Languages are the primary means by which humans communicate, and may be conveyed through a variety of ...
is either finite, , or .
* Assume K is the class of models of a countable first order theory omitting countably many types
Type may refer to:
Science and technology Computing
* Typing, producing text via a keyboard, typewriter, etc.
* Data type, collection of values used for computations.
* File type
* TYPE (DOS command), a command to display contents of a file.
* Ty ...
. If K has a model of cardinality does it have a model of cardinality continuum?
* Do the Henson graphs have the finite model property?
* Does a finitely presented homogeneous structure for a finite relational language have finitely many reduct
In universal algebra and in model theory, a reduct of an algebraic structure is obtained by omitting some of the operations and relations of that structure. The opposite of "reduct" is "expansion."
Definition
Let ''A'' be an algebraic structure ...
s?
* Does there exist an o-minimal first order theory with a trans-exponential (rapid growth) function?
* If the class of atomic models of a complete first order theory is categorical in the , is it categorical in every cardinal?
* Is every infinite, minimal field of characteristic zero algebraically closed? (Here, "minimal" means that every definable subset of the structure is finite or co-finite.)
* Is the Borel monadic theory of the real order (BMTO) decidable? Is the monadic theory of well-ordering (MTWO) consistently decidable?
* Is the theory of the field of Laurent series over decidable? of the field of polynomials over ?
* Is there a logic L which satisfies both the Beth property and Δ-interpolation, is compact but does not satisfy the interpolation property?
* Determine the structure of Keisler's order.
Probability theory
* Ibragimov–Iosifescu conjecture for φ-mixing sequences
Number theory
General
 * ''n'' conjecture: a generalization of the ''abc'' conjecture to more than three integers.
** ''abc'' conjecture: for any , is true for only finitely many positive such that .
**
* ''n'' conjecture: a generalization of the ''abc'' conjecture to more than three integers.
** ''abc'' conjecture: for any , is true for only finitely many positive such that .
** Szpiro's conjecture
In number theory, Szpiro's conjecture relates to the conductor and the discriminant of an elliptic curve. In a slightly modified form, it is equivalent to the well-known ''abc'' conjecture. It is named for Lucien Szpiro, who formulated it in ...
: for any , there is some constant such that, for any elliptic curve defined over with minimal discriminant and conductor , we have .
* Hardy–Littlewood zeta-function conjectures
* Hilbert's eleventh problem
Hilbert's eleventh problem is one of David Hilbert's list of open mathematical problems posed at the Second International Congress of Mathematicians in Paris in 1900. A furthering of the theory of quadratic forms, he stated the problem as follows: ...
: classify quadratic form
In mathematics, a quadratic form is a polynomial with terms all of degree two ("form" is another name for a homogeneous polynomial). For example,
:4x^2 + 2xy - 3y^2
is a quadratic form in the variables and . The coefficients usually belong to ...
s over algebraic number field
In mathematics, an algebraic number field (or simply number field) is an extension field K of the field of rational numbers such that the field extension K / \mathbb has finite degree (and hence is an algebraic field extension).
Thus K is a ...
s.
* Hilbert's ninth problem: find the most general reciprocity law
In mathematics, a reciprocity law is a generalization of the law of quadratic reciprocity to arbitrary monic irreducible polynomials f(x) with integer coefficients. Recall that first reciprocity law, quadratic reciprocity, determines when an ir ...
for the norm residues of -th order in a general algebraic number field
In mathematics, an algebraic number field (or simply number field) is an extension field K of the field of rational numbers such that the field extension K / \mathbb has finite degree (and hence is an algebraic field extension).
Thus K is a ...
, where is a power of a prime.
* Hilbert's twelfth problem: extend the Kronecker–Weber theorem on Abelian extensions of to any base number field.
* Grand Riemann hypothesis: do the nontrivial zeros of all automorphic L-functions lie on the critical line with real ?
**Generalized Riemann hypothesis
The Riemann hypothesis is one of the most important conjectures in mathematics. It is a statement about the zeros of the Riemann zeta function. Various geometrical and arithmetical objects can be described by so-called global L-function, ''L''-func ...
: do the nontrivial zeros of all Dirichlet L-function
In mathematics, a Dirichlet ''L''-series is a function of the form
:L(s,\chi) = \sum_^\infty \frac.
where \chi is a Dirichlet character and ''s'' a complex variable with real part greater than 1. It is a special case of a Dirichlet series. ...
s lie on the critical line with real ?
***Riemann hypothesis
In mathematics, the Riemann hypothesis is the conjecture that the Riemann zeta function has its zeros only at the negative even integers and complex numbers with real part . Many consider it to be the most important unsolved problem in pu ...
: do the nontrivial zeros of the Riemann zeta function lie on the critical line with real ?
*André–Oort conjecture
In mathematics, the André–Oort conjecture is a problem in Diophantine geometry, a branch of number theory, that can be seen as a non-abelian analogue of the Manin–Mumford conjecture, which is now a theorem (and is actually proven in several g ...
: is every irreducible component of the Zariski closure of a set of special points in a Shimura variety a special subvariety
A subvariety (Latin: ''subvarietas'') in botanical nomenclature
Botanical nomenclature is the formal, scientific naming of plants. It is related to, but distinct from Alpha taxonomy, taxonomy. Plant taxonomy is concerned with grouping and class ...
?
* Beilinson's conjectures
* Brocard's problem: are there any integer solutions to other than ?
* Carmichael's totient function conjecture: do all values of Euler's totient function
In number theory, Euler's totient function counts the positive integers up to a given integer that are relatively prime to . It is written using the Greek letter phi as \varphi(n) or \phi(n), and may also be called Euler's phi function. In ...
have multiplicity greater than ?
* Casas-Alvero conjecture
In mathematics, the Casas-Alvero conjecture is an open problem about polynomials which have factors in common with their derivatives, proposed by Eduardo Casas-Alvero in 2001. Formal statement
Let ''f'' be a polynomial of degree ''d'' defined ove ...
: if a polynomial of degree defined over a field of characteristic has a factor in common with its first through -th derivative, then must be the -th power of a linear polynomial?
* Catalan–Dickson conjecture on aliquot sequences: no aliquot sequence
In mathematics, an aliquot sequence is a sequence of positive integers in which each term is the sum of the proper divisors of the previous term. If the sequence reaches the number 1, it ends, since the sum of the proper divisors of 1 is 0.
Def ...
s are infinite but non-repeating.
* Congruent number problem (a corollary to Birch and Swinnerton-Dyer conjecture
In mathematics, the Birch and Swinnerton-Dyer conjecture (often called the Birch–Swinnerton-Dyer conjecture) describes the set of rational solutions to equations defining an elliptic curve. It is an open problem in the field of number theory ...
, per Tunnell's theorem): determine precisely what rational numbers are congruent numbers.
* Erdős–Moser problem: is the only solution to the Erdős–Moser equation?
* Erdős–Straus conjecture: for every , there are positive integers such that .
* Erdős–Ulam problem: is there a dense set
In topology and related areas of mathematics, a subset ''A'' of a topological space ''X'' is said to be dense in ''X'' if every point of ''X'' either belongs to ''A'' or else is arbitrarily "close" to a member of ''A'' — for instance, the ...
of points in the plane all at rational distances from one-another?
* Exponent pair conjecture: for all , is the pair an exponent pair In mathematics, van der Corput's method generates estimates for exponential sums. The method applies two processes, the van der Corput processes A and B which relate the sums into simpler sums which are easier to estimate.
The processes apply to e ...
?
* The Gauss circle problem
In mathematics, the Gauss circle problem is the problem of determining how many integer lattice points there are in a circle centered at the origin and with radius r. This number is approximated by the area of the circle, so the real problem i ...
: how far can the number of integer points in a circle centered at the origin be from the area of the circle?
* Goormaghtigh conjecture on solutions to where and .
* Grimm's conjecture In number theory, Grimm's conjecture (named after Carl Albert Grimm, 1 April 1926 – 2 January 2018) states that to each element of a set of consecutive composite numbers one can assign a distinct prime that divides it. It was first published in '' ...
: each element of a set of consecutive composite number
A composite number is a positive integer that can be formed by multiplying two smaller positive integers. Equivalently, it is a positive integer that has at least one divisor other than 1 and itself. Every positive integer is composite, prime, ...
s can be assigned a distinct prime number
A prime number (or a prime) is a natural number greater than 1 that is not a product of two smaller natural numbers. A natural number greater than 1 that is not prime is called a composite number. For example, 5 is prime because the only way ...
that divides it.
* Hall's conjecture In mathematics, Hall's conjecture is an open question, , on the differences between perfect squares and perfect cubes. It asserts that a perfect square ''y''2 and a perfect cube ''x''3 that are not equal must lie a substantial distance apart. This q ...
: for any , there is some constant such that either or .
* Hilbert–Pólya conjecture In mathematics, the Hilbert–Pólya conjecture states that the non-trivial zeros of the Riemann zeta function correspond to eigenvalues of a self-adjoint operator. It is a possible approach to the Riemann hypothesis, by means of spectral theory. ...
: the nontrivial zeros of the Riemann zeta function correspond to eigenvalues
In linear algebra, an eigenvector () or characteristic vector of a linear transformation is a nonzero vector that changes at most by a scalar factor when that linear transformation is applied to it. The corresponding eigenvalue, often denoted b ...
of a self-adjoint operator
In mathematics, a self-adjoint operator on an infinite-dimensional complex vector space ''V'' with inner product \langle\cdot,\cdot\rangle (equivalently, a Hermitian operator in the finite-dimensional case) is a linear map ''A'' (from ''V'' to ...
.
* Keating–Snaith conjecture concerning the asymptotics of an integral involving the Riemann zeta function
*Lehmer's totient problem
In mathematics, Lehmer's totient problem asks whether there is any composite number ''n'' such that Euler's totient function φ(''n'') divides ''n'' − 1. This is an unsolved problem.
It is known that φ(''n'') = ''n'' − ...
: if divides , must be prime?
* Leopoldt's conjecture: a p-adic analogue of the regulator of an algebraic number field
In mathematics, an algebraic number field (or simply number field) is an extension field K of the field of rational numbers such that the field extension K / \mathbb has finite degree (and hence is an algebraic field extension).
Thus K is a ...
does not vanish.
* Lindelöf hypothesis that for all ,
** The density hypothesis for zeroes of the Riemann zeta function
* Littlewood conjecture: for any two real numbers , , where is the distance from to the nearest integer.
* Mahler's 3/2 problem that no real number has the property that the fractional parts of are less than for all positive integers .
* Montgomery's pair correlation conjecture: the normalized pair correlation function
A correlation function is a function that gives the statistical correlation between random variables, contingent on the spatial or temporal distance between those variables. If one considers the correlation function between random variables re ...
between pairs of zeros of the Riemann zeta function is the same as the pair correlation function of random Hermitian matrices.
* Newman's conjecture: the partition function satisfies any arbitrary congruence infinitely often.
* Pillai's conjecture: for any , the equation has finitely many solutions when are not both .
* Piltz divisor problem on bounding
** Dirichlet's divisor problem: the specific case of the Piltz divisor problem for
* Ramanujan–Petersson conjecture In mathematics, the Ramanujan conjecture, due to , states that Ramanujan's tau function given by the Fourier coefficients of the cusp form of weight
:\Delta(z)= \sum_\tau(n)q^n=q\prod_\left (1-q^n \right)^ = q-24q^2+252q^3- 1472q^4 + 4830q^5- ...
: a number of related conjectures that are generalizations of the original conjecture.
* Sato–Tate conjecture: also a number of related conjectures that are generalizations of the original conjecture.
* Scholz conjecture: the length of the shortest addition chain producing is at most plus the length of the shortest addition chain producing .
* Do Siegel zeros exist?
* Singmaster's conjecture: is there a finite upper bound on the multiplicities of the entries greater than 1 in Pascal's triangle
In mathematics, Pascal's triangle is a triangular array of the binomial coefficients that arises in probability theory, combinatorics, and algebra. In much of the Western world, it is named after the French mathematician Blaise Pascal, althoug ...
?
* The uniqueness conjecture for Markov numbers that every Markov number is the largest number in exactly one normalized solution to the Markov Diophantine equation
In mathematics, a Diophantine equation is an equation, typically a polynomial equation in two or more unknowns with integer coefficients, such that the only solutions of interest are the integer ones. A linear Diophantine equation equates to ...
.
* Vojta's conjecture on heights of points on algebraic varieties
Algebraic varieties are the central objects of study in algebraic geometry, a sub-field of mathematics. Classically, an algebraic variety is defined as the set of solutions of a system of polynomial equations over the real or complex number
...
over algebraic number field
In mathematics, an algebraic number field (or simply number field) is an extension field K of the field of rational numbers such that the field extension K / \mathbb has finite degree (and hence is an algebraic field extension).
Thus K is a ...
s.
* Are there infinitely many perfect number
In number theory, a perfect number is a positive integer that is equal to the sum of its positive divisors, excluding the number itself. For instance, 6 has divisors 1, 2 and 3 (excluding itself), and 1 + 2 + 3 = 6, so 6 is a perfect number.
T ...
s?
*Do any odd perfect number
In number theory, a perfect number is a positive integer that is equal to the sum of its positive divisors, excluding the number itself. For instance, 6 has divisors 1, 2 and 3 (excluding itself), and 1 + 2 + 3 = 6, so 6 is a perfect number.
Th ...
s exist?
*Do quasiperfect numbers exist?
*Do any non-power of 2 almost perfect numbers exist?
*Are there 65, 66, or 67 idoneal numbers?
* Are there any pairs of amicable numbers which have opposite parity?
* Are there any pairs of betrothed numbers which have same parity?
* Are there any pairs of relatively prime
In mathematics, two integers and are coprime, relatively prime or mutually prime if the only positive integer that is a divisor of both of them is 1. Consequently, any prime number that divides does not divide , and vice versa. This is equival ...
amicable numbers?
* Are there infinitely many amicable numbers?
* Are there infinitely many betrothed numbers?
* Are there infinitely many Giuga number A Giuga number is a composite number ''n'' such that for each of its distinct prime factors ''p'i'' we have p_i , \left( - 1\right), or equivalently such that for each of its distinct prime factors ''p'i'' we have p_i^2 , (n - p_i).
The Gi ...
s?
* Does every rational number
In mathematics, a rational number is a number that can be expressed as the quotient or fraction of two integers, a numerator and a non-zero denominator . For example, is a rational number, as is every integer (e.g. ). The set of all ra ...
with an odd denominator have an odd greedy expansion?
* Do any Lychrel numbers exist?
* Do any odd noncototients exist?
* Do any odd weird numbers exist?
* Do any Taxicab(5, 2, n) exist for ''n'' > 1?
* Is there a covering system with odd distinct moduli?
* Is a normal number
In mathematics, a real number is said to be simply normal in an integer base b if its infinite sequence of digits is distributed uniformly in the sense that each of the b digit values has the same natural density 1/b. A number is said to ...
(i.e., is each digit 0–9 equally frequent)?
* Is 10 a solitary number?
* Can a 3×3 magic square
In recreational mathematics, a square array of numbers, usually positive integers, is called a magic square if the sums of the numbers in each row, each column, and both main diagonals are the same. The 'order' of the magic square is the number ...
be constructed from 9 distinct perfect square numbers?
* Which integers can be written as the sum of three perfect cubes?
* Can every integer be written as a sum of four perfect cubes?
* Find the value of the De Bruijn–Newman constant
The de Bruijn–Newman constant, denoted by Λ and named after Nicolaas Govert de Bruijn and Charles M. Newman, is a mathematical constant defined via the zeros of a certain function ''H''(''λ'', ''z''), where ''λ'' is a real parameter ...
.
Additive number theory
* Beal's conjecture: for all integral solutions to where , all three numbers must share some prime factor.
* Erdős conjecture on arithmetic progressions
Erdős' conjecture on arithmetic progressions, often referred to as the Erdős–Turán conjecture, is a conjecture in arithmetic combinatorics (not to be confused with the Erdős–Turán conjecture on additive bases). It states that if the sum ...
that if the sum of the reciprocals of the members of a set of positive integers diverges, then the set contains arbitrarily long arithmetic progression
An arithmetic progression or arithmetic sequence () is a sequence of numbers such that the difference between the consecutive terms is constant. For instance, the sequence 5, 7, 9, 11, 13, 15, . . . is an arithmetic progression with a common differ ...
s.
* Erdős–Turán conjecture on additive bases: if is an additive basis In additive number theory, an additive basis is a set S of natural numbers with the property that, for some finite number k, every natural number can be expressed as a sum of k or fewer elements of S. That is, the sumset of k copies of S consists of ...
of order , then the number of ways that positive integers can be expressed as the sum of two numbers in must tend to infinity as tends to infinity.
* Fermat–Catalan conjecture: there are finitely many distinct solutions to the equation with being positive coprime integers
In mathematics, two integers and are coprime, relatively prime or mutually prime if the only positive integer that is a divisor of both of them is 1. Consequently, any prime number that divides does not divide , and vice versa. This is equival ...
and being positive integers satisfying .
* Gilbreath's conjecture on consecutive applications of the unsigned forward difference operator to the sequence of prime number
A prime number (or a prime) is a natural number greater than 1 that is not a product of two smaller natural numbers. A natural number greater than 1 that is not prime is called a composite number. For example, 5 is prime because the only way ...
s.
* Goldbach's conjecture: every even natural number greater than is the sum of two prime number
A prime number (or a prime) is a natural number greater than 1 that is not a product of two smaller natural numbers. A natural number greater than 1 that is not prime is called a composite number. For example, 5 is prime because the only way ...
s.
* Lander, Parkin, and Selfridge conjecture: if the sum of -th powers of positive integers is equal to a different sum of -th powers of positive integers, then .
* Lemoine's conjecture: all odd integers greater than can be represented as the sum of an odd prime number
A prime number (or a prime) is a natural number greater than 1 that is not a product of two smaller natural numbers. A natural number greater than 1 that is not prime is called a composite number. For example, 5 is prime because the only way ...
and an even semiprime
In mathematics, a semiprime is a natural number that is the product of exactly two prime numbers. The two primes in the product may equal each other, so the semiprimes include the squares of prime numbers.
Because there are infinitely many prime ...
.
* Minimum overlap problem In number theory and set theory, the minimum overlap problem is a problem proposed by Hungarian mathematician Paul Erdős in 1955.
Formal statement of the problem
Let and be two complementary subsets, a splitting of the set of natural number ...
of estimating the minimum possible maximum number of times a number appears in the termwise difference of two equally large sets partitioning the set
* Pollock's conjectures
* Skolem problem: can an algorithm determine if a constant-recursive sequence
In mathematics and theoretical computer science, a constant-recursive sequence is an infinite sequence of numbers where each number in the sequence is equal to a fixed linear combination of one or more of its immediate predecessors. A consta ...
contains a zero?
* The values of ''g''(''k'') and ''G''(''k'') in Waring's problem
In number theory, Waring's problem asks whether each natural number ''k'' has an associated positive integer ''s'' such that every natural number is the sum of at most ''s'' natural numbers raised to the power ''k''. For example, every natural numb ...
* Do the Ulam numbers have a positive density?
* Determine growth rate of ''r''''k''(''N'') (see Szemerédi's theorem
In arithmetic combinatorics, Szemerédi's theorem is a result concerning arithmetic progressions in subsets of the integers. In 1936, Erdős and Turán conjectured that every set of integers ''A'' with positive natural density contains a ''k''-t ...
)
Algebraic number theory
* Class number problem: are there infinitely many real quadratic number fields with unique factorization?
* Fontaine–Mazur conjecture: actually numerous conjectures, all proposed by Jean-Marc Fontaine and Barry Mazur
Barry Charles Mazur (; born December 19, 1937) is an American mathematician and the Gerhard Gade University Professor at Harvard University. His contributions to mathematics include his contributions to Wiles's proof of Fermat's Last Theorem in ...
.
* Gan–Gross–Prasad conjecture: a restriction
Restriction, restrict or restrictor may refer to:
Science and technology
* restrict, a keyword in the C programming language used in pointer declarations
* Restriction enzyme, a type of enzyme that cleaves genetic material
Mathematics and log ...
problem in representation theory of real or p-adic Lie groups.
* Greenberg's conjectures
Greenberg's conjecture is either of two conjectures in algebraic number theory proposed by Ralph Greenberg. Both are still unsolved as of 2021.
Invariants conjecture
The first conjecture was proposed in 1976 and concerns Iwasawa invariants. Thi ...
* Hermite's problem Hermite's problem is an open problem in mathematics posed by Charles Hermite in 1848. He asked for a way of expressing real numbers as sequences of natural numbers, such that the sequence is eventually periodic precisely when the original number is ...
: is it possible, for any natural number , to assign a sequence of natural number
In mathematics, the natural numbers are those numbers used for counting (as in "there are ''six'' coins on the table") and ordering (as in "this is the ''third'' largest city in the country").
Numbers used for counting are called '' cardinal ...
s to each real number
In mathematics, a real number is a number that can be used to measurement, measure a ''continuous'' one-dimensional quantity such as a distance, time, duration or temperature. Here, ''continuous'' means that values can have arbitrarily small var ...
such that the sequence for is eventually periodic if and only if is algebraic of degree ?
* Kummer–Vandiver conjecture: primes do not divide the class number of the maximal real subfield of the -th cyclotomic field
In number theory, a cyclotomic field is a number field obtained by adjoining a complex root of unity to , the field of rational numbers.
Cyclotomic fields played a crucial role in the development of modern algebra and number theory because o ...
.
* Lang and Trotter's conjecture on supersingular primes that the number of supersingular primes less than a constant is within a constant multiple of
* Selberg's 1/4 conjecture: the eigenvalues
In linear algebra, an eigenvector () or characteristic vector of a linear transformation is a nonzero vector that changes at most by a scalar factor when that linear transformation is applied to it. The corresponding eigenvalue, often denoted b ...
of the Laplace operator
In mathematics, the Laplace operator or Laplacian is a differential operator given by the divergence of the gradient of a scalar function on Euclidean space. It is usually denoted by the symbols \nabla\cdot\nabla, \nabla^2 (where \nabla is t ...
on Maass wave form
In mathematics, Maass forms or Maass wave forms are studied in the theory of automorphic forms. Maass forms are complex-valued smooth functions of the upper half plane, which transform in a similar way under the operation of a discrete subgroup \ ...
s of congruence subgroups are at least .
* Stark conjectures (including Brumer–Stark conjecture)
* Characterize all algebraic number fields that have some power basis
In mathematics, an algebraic number field (or simply number field) is an extension field K of the field of rational numbers such that the field extension K / \mathbb has finite degree (and hence is an algebraic field extension).
Thus K is a ...
.
Computational number theory
* Can integer factorization be done in polynomial time
In computer science, the time complexity is the computational complexity that describes the amount of computer time it takes to run an algorithm. Time complexity is commonly estimated by counting the number of elementary operations performed by ...
?
Prime numbers
 *
* Agoh–Giuga conjecture In number theory the Agoh–Giuga conjecture on the Bernoulli numbers ''B'k'' postulates that ''p'' is a prime number if and only if
:pB_ \equiv -1 \pmod p.
It is named after Takashi Agoh and Giuseppe Giuga.
Equivalent formulation
The conje ...
on the Bernoulli number
In mathematics, the Bernoulli numbers are a sequence of rational numbers which occur frequently in analysis. The Bernoulli numbers appear in (and can be defined by) the Taylor series expansions of the tangent and hyperbolic tangent functions ...
s that is prime if and only if
* Agrawal's conjecture that given coprime positive integers and , if , then either is prime or
* Artin's conjecture on primitive roots that if an integer is neither a perfect square nor , then it is a primitive root modulo infinitely many prime number
A prime number (or a prime) is a natural number greater than 1 that is not a product of two smaller natural numbers. A natural number greater than 1 that is not prime is called a composite number. For example, 5 is prime because the only way ...
s
* Brocard's conjecture
In number theory, Brocard's conjecture is the conjecture that there are at least four prime numbers between (''p'n'')2 and (''p'n''+1)2, where ''p'n'' is the ''n''th prime number, for every ''n'' ≥ 2. The conjecture is named after ...
: there are always at least prime number
A prime number (or a prime) is a natural number greater than 1 that is not a product of two smaller natural numbers. A natural number greater than 1 that is not prime is called a composite number. For example, 5 is prime because the only way ...
s between consecutive squares of prime numbers, aside from and .
* Bunyakovsky conjecture
The Bunyakovsky conjecture (or Bouniakowsky conjecture) gives a criterion for a polynomial f(x) in one variable with integer coefficients to give infinitely many prime values in the sequencef(1), f(2), f(3),\ldots. It was stated in 1857 by the ...
: if an integer-coefficient polynomial has a positive leading coefficient, is irreducible over the integers, and has no common factors over all where is a positive integer, then is prime infinitely often.
* Catalan's Mersenne conjecture: some Catalan–Mersenne number
In mathematics, a double Mersenne number is a Mersenne number of the form
:M_ = 2^-1
where ''p'' is prime.
Examples
The first four terms of the sequence of double Mersenne numbers areChris Caldwell''Mersenne Primes: History, Theorems and Lis ...
is composite and thus all Catalan–Mersenne numbers are composite after some point.
* Dickson's conjecture In number theory, a branch of mathematics, Dickson's conjecture is the conjecture stated by that for a finite set of linear forms , , ..., with , there are infinitely many positive integers for which they are all prime, unless there is a congruen ...
: for a finite set of linear forms with each , there are infinitely many for which all forms are prime
A prime number (or a prime) is a natural number greater than 1 that is not a product of two smaller natural numbers. A natural number greater than 1 that is not prime is called a composite number. For example, 5 is prime because the only way ...
, unless there is some congruence
Congruence may refer to:
Mathematics
* Congruence (geometry), being the same size and shape
* Congruence or congruence relation, in abstract algebra, an equivalence relation on an algebraic structure that is compatible with the structure
* In mod ...
condition preventing it.
* Dubner's conjecture
Many mathematical problems have been stated but not yet solved. These problems come from many areas of mathematics, such as theoretical physics, computer science, algebra, analysis, combinatorics, algebraic, differential, discrete and Euclid ...
: every even number greater than is the sum of two primes
A prime number (or a prime) is a natural number greater than 1 that is not a product of two smaller natural numbers. A natural number greater than 1 that is not prime is called a composite number. For example, 5 is prime because the only ways ...
which both have twins
Twins are two offspring produced by the same pregnancy.MedicineNet > Definition of TwinLast Editorial Review: 19 June 2000 Twins can be either ''monozygotic'' ('identical'), meaning that they develop from one zygote, which splits and forms two em ...
.
* Elliott–Halberstam conjecture
In number theory, the Elliott–Halberstam conjecture is a conjecture about the distribution of prime numbers in arithmetic progressions. It has many applications in sieve theory. It is named for Peter D. T. A. Elliott and Heini Halberstam, who ...
on the distribution of prime number
A prime number (or a prime) is a natural number greater than 1 that is not a product of two smaller natural numbers. A natural number greater than 1 that is not prime is called a composite number. For example, 5 is prime because the only way ...
s in arithmetic progression
An arithmetic progression or arithmetic sequence () is a sequence of numbers such that the difference between the consecutive terms is constant. For instance, the sequence 5, 7, 9, 11, 13, 15, . . . is an arithmetic progression with a common differ ...
s.
* Erdős–Mollin–Walsh conjecture: no three consecutive numbers are all powerful.
* Feit–Thompson conjecture: for all distinct prime number
A prime number (or a prime) is a natural number greater than 1 that is not a product of two smaller natural numbers. A natural number greater than 1 that is not prime is called a composite number. For example, 5 is prime because the only way ...
s and , does not divide
* Fortune's conjecture that no Fortunate number is composite.
* The Gaussian moat
In number theory, the Gaussian moat problem asks whether it is possible to find an infinite sequence of distinct Gaussian prime numbers such that the difference between consecutive numbers in the sequence is bounded. More colorfully, if one imagin ...
problem: is it possible to find an infinite sequence of distinct Gaussian prime number
In number theory, a Gaussian integer is a complex number whose real and imaginary parts are both integers. The Gaussian integers, with ordinary addition and multiplication of complex numbers, form an integral domain, usually written as \mathbf /m ...
s such that the difference between consecutive numbers in the sequence is bounded?
* Gillies' conjecture on the distribution of prime
A prime number (or a prime) is a natural number greater than 1 that is not a product of two smaller natural numbers. A natural number greater than 1 that is not prime is called a composite number. For example, 5 is prime because the only way ...
divisors of Mersenne numbers
In mathematics, a Mersenne prime is a prime number that is one less than a power of two. That is, it is a prime number of the form for some integer . They are named after Marin Mersenne, a French Minim friar, who studied them in the early 1 ...
.
* Goldbach conjecture
Goldbach's conjecture is one of the oldest and best-known unsolved problems in number theory and all of mathematics. It states that every even natural number greater than 2 is the sum of two prime numbers.
The conjecture has been shown to hold ...
: all even natural number
In mathematics, the natural numbers are those numbers used for counting (as in "there are ''six'' coins on the table") and ordering (as in "this is the ''third'' largest city in the country").
Numbers used for counting are called '' cardinal ...
s greater than are the sum of two prime number
A prime number (or a prime) is a natural number greater than 1 that is not a product of two smaller natural numbers. A natural number greater than 1 that is not prime is called a composite number. For example, 5 is prime because the only way ...
s.
* Landau's problems
At the 1912 International Congress of Mathematicians, Edmund Landau listed four basic problems about prime numbers. These problems were characterised in his speech as "unattackable at the present state of mathematics" and are now known as Landau' ...
* Problems associated to Linnik's theorem
* New Mersenne conjecture: for any odd natural number
In mathematics, the natural numbers are those numbers used for counting (as in "there are ''six'' coins on the table") and ordering (as in "this is the ''third'' largest city in the country").
Numbers used for counting are called '' cardinal ...
, if any two of the three conditions or , is prime, and is prime are true, then the third condition is true.
* Polignac's conjecture: for all positive even numbers , there are infinitely many prime gap
A prime gap is the difference between two successive prime numbers. The ''n''-th prime gap, denoted ''g'n'' or ''g''(''p'n'') is the difference between the (''n'' + 1)-th and the
''n''-th prime numbers, i.e.
:g_n = p_ - p_n.\
W ...
s of size .
* Schinzel's hypothesis H that for every finite collection of nonconstant irreducible polynomial
In mathematics, an irreducible polynomial is, roughly speaking, a polynomial that cannot be factored into the product of two non-constant polynomials. The property of irreducibility depends on the nature of the coefficients that are accepted f ...
s over the integers with positive leading coefficients, either there are infinitely many positive integers for which are all primes
A prime number (or a prime) is a natural number greater than 1 that is not a product of two smaller natural numbers. A natural number greater than 1 that is not prime is called a composite number. For example, 5 is prime because the only ways ...
, or there is some fixed divisor which, for all , divides some .
* Selfridge's conjecture: is 78,557 the lowest Sierpiński number In number theory, a Sierpiński number is an odd natural number ''k'' such that k \times 2^n + 1 is composite for all natural numbers ''n''. In 1960, Wacław Sierpiński proved that there are infinitely many odd integers ''k'' which have this pro ...
?
* Twin prime conjecture
A twin prime is a prime number that is either 2 less or 2 more than another prime number—for example, either member of the twin prime pair (41, 43). In other words, a twin prime is a prime that has a prime gap of two. Sometimes the term ''twin pr ...
: there are infinitely many twin prime
A twin prime is a prime number that is either 2 less or 2 more than another prime number—for example, either member of the twin prime pair (41, 43). In other words, a twin prime is a prime that has a prime gap of two. Sometimes the term ''twin p ...
s.
* Does the converse of Wolstenholme's theorem hold for all natural numbers?
* Are all Euclid numbers square-free?
* Are all Fermat numbers square-free?
* Are all Mersenne number
In mathematics, a Mersenne prime is a prime number that is one less than a power of two. That is, it is a prime number of the form for some integer . They are named after Marin Mersenne, a French Minim friar, who studied them in the early 17t ...
s of prime index square-free?
* Are there any composite ''c'' satisfying 2''c'' − 1 ≡ 1 (mod ''c''2)?
* Are there any Wall–Sun–Sun primes?
* Are there any Wieferich primes in base 47?
* Are there infinitely many balanced prime In number theory, a balanced prime is a prime number with equal-sized prime gaps above and below it, so that it is equal to the arithmetic mean of the nearest primes above and below. Or to put it algebraically, given a prime number p_n, where is i ...
s?
* Are there infinitely many Carol primes?
* Are there infinitely many cluster primes?
* Are there infinitely many cousin prime
In number theory, cousin primes are prime numbers that differ by four. Compare this with twin primes, pairs of prime numbers that differ by two, and sexy primes, pairs of prime numbers that differ by six.
The cousin primes (sequences and in O ...
s?
* Are there infinitely many Cullen primes?
* Are there infinitely many Euclid primes?
* Are there infinitely many Fibonacci prime
A Fibonacci prime is a Fibonacci number that is prime, a type of integer sequence prime.
The first Fibonacci primes are :
: 2, 3, 5, 13, 89, 233, 1597, 28657, 514229, 433494437, 2971215073, ....
Known Fibonacci primes
It is not known wh ...
s?
* Are there infinitely many Kummer primes?
* Are there infinitely many Kynea primes?
* Are there infinitely many Lucas primes?
* Are there infinitely many Mersenne prime
In mathematics, a Mersenne prime is a prime number that is one less than a power of two. That is, it is a prime number of the form for some integer . They are named after Marin Mersenne, a French Minim friar, who studied them in the early 17 ...
s ( Lenstra–Pomerance–Wagstaff conjecture); equivalently, infinitely many even perfect number
In number theory, a perfect number is a positive integer that is equal to the sum of its positive divisors, excluding the number itself. For instance, 6 has divisors 1, 2 and 3 (excluding itself), and 1 + 2 + 3 = 6, so 6 is a perfect number.
T ...
s?
* Are there infinitely many Newman–Shanks–Williams primes?
* Are there infinitely many palindromic prime
In mathematics, a palindromic prime (sometimes called a palprime) is a prime number that is also a palindromic number. Palindromicity depends on the base of the number system and its notational conventions, while primality is independent of such ...
s to every base?
* Are there infinitely many Pell primes?
* Are there infinitely many Pierpont primes?
* Are there infinitely many prime quadruplet
In number theory, a prime quadruplet (sometimes called prime quadruple) is a set of four prime numbers of the form This represents the closest possible grouping of four primes larger than 3, and is the only prime constellation of length 4.
Prim ...
s?
* Are there infinitely many prime triplets?
* Are there infinitely many regular prime
In number theory, a regular prime is a special kind of prime number, defined by Ernst Kummer in 1850 to prove certain cases of Fermat's Last Theorem. Regular primes may be defined via the divisibility of either class numbers or of Bernoulli nu ...
s, and if so is their relative density ?
* Are there infinitely many sexy prime
In number theory, sexy primes are prime numbers that differ from each other by 6. For example, the numbers 5 and 11 are both sexy primes, because both are prime and .
The term "sexy prime" is a pun stemming from the Latin word for six: .
I ...
s?
* Are there infinitely many safe and Sophie Germain primes
In number theory, a prime number ''p'' is a if 2''p'' + 1 is also prime. The number 2''p'' + 1 associated with a Sophie Germain prime is called a . For example, 11 is a Sophie Germain prime and 2 × 11 + ...
?
* Are there infinitely many Wagstaff primes?
* Are there infinitely many Wieferich primes?
* Are there infinitely many Wilson prime
In number theory, a Wilson prime is a prime number p such that p^2 divides (p-1)!+1, where "!" denotes the factorial function; compare this with Wilson's theorem, which states that every prime p divides (p-1)!+1. Both are named for 18th-century E ...
s?
* Are there infinitely many Wolstenholme primes?
* Are there infinitely many Woodall primes?
* Can a prime ''p'' satisfy and simultaneously?
* Does every prime number appear in the Euclid–Mullin sequence?
* Find the smallest Skewes' number
In number theory, Skewes's number is any of several large numbers used by the South African mathematician Stanley Skewes as upper bounds for the smallest natural number x for which
:\pi(x) > \operatorname(x),
where is the prime-counting function ...
* For any given integer ''a'' > 0, are there infinitely many Lucas–Wieferich primes associated with the pair (''a'', −1)? (Specially, when ''a'' = 1, this is the Fibonacci-Wieferich primes, and when ''a'' = 2, this is the Pell-Wieferich primes)
* For any given integer ''a'' > 0, are there infinitely many primes ''p'' such that ''a''''p'' − 1 ≡ 1 (mod ''p''2)?
* For any given integer ''a'' which is not a square and does not equal to −1, are there infinitely many primes with ''a'' as a primitive root?
* For any given integer ''b'' which is not a perfect power and not of the form −4''k''4 for integer ''k'', are there infinitely many repunit
In recreational mathematics, a repunit is a number like 11, 111, or 1111 that contains only the digit 1 — a more specific type of repdigit. The term stands for repeated unit and was coined in 1966 by Albert H. Beiler in his book ''Recrea ...
primes to base ''b''?
* For any given integers , with and are there infinitely many primes of the form with integer ''n'' ≥ 1?
* Is every Fermat number composite for ?
* Is 509,203 the lowest Riesel number In mathematics, a Riesel number is an odd natural number ''k'' for which k\times2^n-1 is composite for all natural numbers ''n'' . In other words, when ''k'' is a Riesel number, all members of the following set are composite:
:\left\.
If the f ...
?
Set theory
Note: These conjectures are about models of Zermelo-Frankel set theory with choice
A choice is the range of different things from which a being can choose. The arrival at a choice may incorporate motivators and models. For example, a traveler might choose a route for a journey based on the preference of arriving at a given ...
, and may not be able to be expressed in models of other set theories such as the various constructive set theories or non-wellfounded set theory.
* ( Woodin) Does the generalized continuum hypothesis
In mathematics, the continuum hypothesis (abbreviated CH) is a hypothesis about the possible sizes of infinite sets. It states that
or equivalently, that
In Zermelo–Fraenkel set theory with the axiom of choice (ZFC), this is equivalent t ...
below a strongly compact cardinal imply the generalized continuum hypothesis
In mathematics, the continuum hypothesis (abbreviated CH) is a hypothesis about the possible sizes of infinite sets. It states that
or equivalently, that
In Zermelo–Fraenkel set theory with the axiom of choice (ZFC), this is equivalent t ...
everywhere?
* Does the generalized continuum hypothesis
In mathematics, the continuum hypothesis (abbreviated CH) is a hypothesis about the possible sizes of infinite sets. It states that
or equivalently, that
In Zermelo–Fraenkel set theory with the axiom of choice (ZFC), this is equivalent t ...
entail for every singular cardinal ?
* Does the generalized continuum hypothesis
In mathematics, the continuum hypothesis (abbreviated CH) is a hypothesis about the possible sizes of infinite sets. It states that
or equivalently, that
In Zermelo–Fraenkel set theory with the axiom of choice (ZFC), this is equivalent t ...
imply the existence of an ℵ2-Suslin tree?
* If ℵω is a strong limit cardinal, is (see Singular cardinals hypothesis)? The best bound, ℵω4, was obtained by Shelah using his PCF theory PCF theory is the name of a mathematical theory, introduced by Saharon , that deals with the cofinality of the ultraproducts of ordered sets. It gives strong upper bounds on the cardinalities of power sets of singular cardinals, and has many more ...
.
* The problem of finding the ultimate core model In set theory, the core model is a definable inner model of the universe of all sets. Even though set theorists refer to "the core model", it is not a uniquely identified mathematical object. Rather, it is a class of inner models that under the rig ...
, one that contains all large cardinals
In the mathematical field of set theory, a large cardinal property is a certain kind of property of transfinite cardinal numbers. Cardinals with such properties are, as the name suggests, generally very "large" (for example, bigger than the least � ...
.
* Woodin's Ω-conjecture: if there is a proper class
Proper may refer to:
Mathematics
* Proper map, in topology, a property of continuous function between topological spaces, if inverse images of compact subsets are compact
* Proper morphism, in algebraic geometry, an analogue of a proper map f ...
of Woodin cardinal In set theory, a Woodin cardinal (named for W. Hugh Woodin) is a cardinal number \lambda such that for all functions
:f : \lambda \to \lambda
there exists a cardinal \kappa < \lambda with
:
and an s, then Ω-logic satisfies an analogue of Gödel's completeness theorem.
* Does the consistency
In classical deductive logic, a consistent theory is one that does not lead to a logical contradiction. The lack of contradiction can be defined in either semantic or syntactic terms. The semantic definition states that a theory is consistent ...
of the existence of a strongly compact cardinal imply the consistent existence of a supercompact cardinal In set theory, a supercompact cardinal is a type of large cardinal. They display a variety of reflection properties.
Formal definition
If ''λ'' is any ordinal, ''κ'' is ''λ''-supercompact means that there exists an elementary ...
?
* Does there exist a Jónsson algebra on ℵω?
* Is OCA (the open coloring axiom The open coloring axiom (abbreviated OCA) is an axiom about coloring edges of a graph whose vertices are a subset of the real numbers: two different versions were introduced by and by .
Statement
Suppose that ''X'' is a subset of the reals, and e ...
) consistent with ?
* Without assuming the axiom of choice
In mathematics, the axiom of choice, or AC, is an axiom of set theory equivalent to the statement that ''a Cartesian product of a collection of non-empty sets is non-empty''. Informally put, the axiom of choice says that given any collection ...
, can a nontrivial elementary embedding ''V''→''V'' exist?
Topology
 * Baum–Connes conjecture: the assembly map is an
* Baum–Connes conjecture: the assembly map is an isomorphism
In mathematics, an isomorphism is a structure-preserving mapping between two structures of the same type that can be reversed by an inverse mapping. Two mathematical structures are isomorphic if an isomorphism exists between them. The word i ...
.
* Bing–Borsuk conjecture: every -dimensional homogeneous
Homogeneity and heterogeneity are concepts often used in the sciences and statistics relating to the uniformity of a substance or organism. A material or image that is homogeneous is uniform in composition or character (i.e. color, shape, siz ...
absolute neighborhood retract is a topological manifold In topology, a branch of mathematics, a topological manifold is a topological space that locally resembles real ''n''- dimensional Euclidean space. Topological manifolds are an important class of topological spaces, with applications throughout ma ...
.
* Borel conjecture: Aspherical space, aspherical closed manifolds are determined up to homeomorphism by their fundamental group
In the mathematical field of algebraic topology, the fundamental group of a topological space is the group of the equivalence classes under homotopy of the loops contained in the space. It records information about the basic shape, or holes, of ...
s.
* Halperin conjecture on rational Serre spectral sequences of certain fibrations.
* Hilbert–Smith conjecture: if a Locally compact space, locally compact topological group has a Continuous function, continuous, Group action#Types of actions, faithful group action on a topological manifold In topology, a branch of mathematics, a topological manifold is a topological space that locally resembles real ''n''- dimensional Euclidean space. Topological manifolds are an important class of topological spaces, with applications throughout ma ...
, then the group must be a Lie group.
* Mazur's conjectures
* Novikov conjecture on the Homotopy#Invariance, homotopy invariance of certain polynomials in the Pontryagin classes of a manifold
In mathematics, a manifold is a topological space that locally resembles Euclidean space near each point. More precisely, an n-dimensional manifold, or ''n-manifold'' for short, is a topological space with the property that each point has a ...
, arising from the fundamental group
In the mathematical field of algebraic topology, the fundamental group of a topological space is the group of the equivalence classes under homotopy of the loops contained in the space. It records information about the basic shape, or holes, of ...
.
* Quadrisecants of wild knots: it has been conjectured that wild knots always have infinitely many quadrisecants.
* Ravenel conjectures, Telescope conjecture: the last of Ravenel's conjectures in stable homotopy theory to be resolved.
* Unknotting problem: can unknots be recognized in Time complexity#Polynomial time, polynomial time?
* Volume conjecture relating quantum invariants of Knot (mathematics), knots to the hyperbolic geometry of their knot complements.
* Whitehead conjecture: every Connectedness, connected CW complex#Inductive construction of CW complexes, subcomplex of a two-dimensional Aspherical space, aspherical CW complex is aspherical.
* Zeeman conjecture: given a finite Contractible space, contractible two-dimensional CW complex , is the space Collapse (topology), collapsible?
Problems solved since 1995

Algebra
* Suita conjecture (Qi'an Guan and Xiangyu Zhou, 2015)
* Torsion conjecture (Loïc Merel, 1996)
* Carlitz–Wan conjecture (Hendrik Lenstra, 1995)
Analysis
* Kadison–Singer problem (Adam Marcus (mathematician), Adam Marcus, Daniel Spielman and Nikhil Srivastava, 2013)
Combinatorics
* Erdős sumset conjecture (Joel Moreira, Florian Richter, Donald Robertson, 2018)
* Simplicial sphere, McMullen's g-conjecture on the possible numbers of faces of different dimensions in a simplicial sphere (also Grünbaum conjecture, several conjectures of Kühnel) (Karim Adiprasito, 2018)
* Hirsch conjecture (Francisco Santos Leal, 2010)
* Stanley–Wilf conjecture (Gábor Tardos and Adam Marcus (mathematician), Adam Marcus, 2004) (and also the Alon–Friedgut conjecture)
* Kemnitz's conjecture (Christian Reiher, 2003, Carlos di Fiore, 2003)
* Cameron–Erdős conjecture (Ben J. Green, 2003, Alexander Sapozhenko, 2003)
Dynamical systems
* Zimmer's conjecture (Aaron Brown, David Fisher, and Sebastián Hurtado-Salazar, 2017)
* Painlevé conjecture (Jinxin Xue, 2014)
Game theory
* The angel problem (Various independent proofs, 2006)
Geometry
21st century
* Maximal rank conjecture (Eric Larson, 2018)
* Weibel's conjecture (Moritz Kerz, Florian Strunk, and Georg Tamme, 2018)
* Yau's conjecture (Antoine Song, 2018)
* Pentagonal tiling (Michaël Rao, 2017)
* Willmore conjecture (Fernando Codá Marques and André Neves, 2012)
* Erdős distinct distances problem (Larry Guth, Nets Katz, Nets Hawk Katz, 2011)
* Squaring the plane, Heterogeneous tiling conjecture (squaring the plane) (Frederick V. Henle and James M. Henle, 2008)
* Tameness conjecture (Ian Agol, 2004)
20th century
* Honeycomb conjecture (Thomas Callister Hales, 1999)
* Lange's conjecture (Montserrat Teixidor i Bigas and Barbara Russo, 1999)
* Bogomolov conjecture (Emmanuel Ullmo, 1998, Shou-Wu Zhang, 1998)
* Kepler conjecture (Samuel Ferguson, Thomas Callister Hales, 1998)
* Dodecahedral conjecture (Thomas Callister Hales, Sean McLaughlin, 1998)
Graph theory
* Blankenship–Oporowski conjecture on the book thickness of subdivisions (Vida Dujmović, David Eppstein, Robert Hickingbotham, Pat Morin, and David Wood (mathematician), David Wood, 2021)
*Graceful labeling, Ringel's conjecture on graceful labeling of trees (Richard Montgomery, Benny Sudakov, Alexey Pokrovskiy, 2020)
*Disproof of Hedetniemi's conjecture on the chromatic number of tensor products of graphs (Yaroslav Shitov, 2019)
* Kelmans–Seymour conjecture (Dawei He, Yan Wang, and Xingxing Yu, 2020)
* Goldberg–Seymour conjecture (Guantao Chen, Guangming Jing, and Wenan Zang, 2019)
* Babai's problem
Babai's problem is a problem in algebraic graph theory first proposed in 1979 by László Babai.
Babai's problem
Let G be a finite group, let \operatorname(G) be the set of all irreducible characters of G, let \Gamma=\operatorname(G,S) be the ...
(Alireza Abdollahi, Maysam Zallaghi, 2015)
* Alspach's conjecture (Darryn Bryant, Daniel Horsley, William Pettersson, 2014)
* Alon–Saks–Seymour conjecture (Hao Huang, Benny Sudakov, 2012)
* Read's conjecture, Read–Hoggar conjecture (June Huh, 2009)
* Scheinerman's conjecture (Jeremie Chalopin and Daniel Gonçalves, 2009)
* Erdős–Menger conjecture (Ron Aharoni, Eli Berger 2007)
* Road coloring conjecture (Avraham Trahtman, 2007)
* Robertson–Seymour theorem (Neil Robertson (mathematician), Neil Robertson, Paul Seymour (mathematician), Paul Seymour, 2004)
* Strong perfect graph conjecture (Maria Chudnovsky, Neil Robertson (mathematician), Neil Robertson, Paul Seymour (mathematician), Paul Seymour and Robin Thomas (mathematician), Robin Thomas, 2002)
* Toida's conjecture (Mikhail Muzychuk, Mikhail Klin, and Reinhard Pöschel, 2001)
* Harary's conjecture on the integral sum number of complete graphs (Zhibo Chen, 1996)
Group theory
* Hanna Neumann conjecture (Joel Friedman, 2011, Igor Mineyev, 2011)
* Density theorem for Kleinian groups, Density theorem (Hossein Namazi, Juan Souto, 2010)
* Full classification of finite simple groups (Koichiro Harada, Ronald Solomon, 2008)
Number theory
21st century
*Duffin–Schaeffer conjecture, Duffin-Schaeffer conjecture (Dimitris Koukoulopoulos, James Maynard (mathematician), James Maynard, 2019)
* Vinogradov's mean-value theorem#The conjectured form, Main conjecture in Vinogradov's mean-value theorem (Jean Bourgain, Ciprian Demeter, Larry Guth, 2015)
* Goldbach's weak conjecture (Harald Helfgott, 2013)
*Prime gap#Further results, Existence of bounded gaps between primes (Yitang Zhang, Polymath Project, Polymath8, James Maynard (mathematician), James Maynard, 2013)
* Sidon sequence, Sidon set problem (Javier Cilleruelo, Imre Z. Ruzsa, and Carlos Vinuesa, 2010)
* Serre's modularity conjecture (Chandrashekhar Khare and Jean-Pierre Wintenberger, 2008)
* Green–Tao theorem (Ben J. Green and Terence Tao, 2004)
* Mihăilescu's theorem, Catalan's conjecture (Preda Mihăilescu, 2002)
* Erdős–Graham problem (Ernest S. Croot III, 2000)
20th century
* Lafforgue's theorem (Laurent Lafforgue, 1998)
* Fermat's Last Theorem (Andrew Wiles and Richard Taylor (mathematician), Richard Taylor, 1995)
Ramsey theory
* Burr–Erdős conjecture (Choongbum Lee, 2017)
* Boolean Pythagorean triples problem (Marijn Heule, Oliver Kullmann, Victor W. Marek, 2016)
Theoretical computer science
*Decision tree model#Sensitivity conjecture, Sensitivity conjecture for Boolean functions (Hao Huang (mathematician), Hao Huang, 2019)
Topology
*Deciding whether the Conway knot is a slice knot (Lisa Piccirillo, 2020)
* Virtual Haken conjecture (Ian Agol, Daniel Groves, Jason Manning, 2012) (and by work of Daniel Wise (mathematician), Daniel Wise also virtually fibered conjecture)
* Hsiang–Lawson's conjecture (Simon Brendle, 2012)
* Ehrenpreis conjecture (Jeremy Kahn, Vladimir Markovic, 2011)
* Atiyah conjecture (Austin, 2009)
* Cobordism hypothesis (Jacob Lurie, 2008)
* Spherical space form conjecture (Grigori Perelman
Grigori Yakovlevich Perelman ( rus, links=no, Григорий Яковлевич Перельман, p=ɡrʲɪˈɡorʲɪj ˈjakəvlʲɪvʲɪtɕ pʲɪrʲɪlʲˈman, a=Ru-Grigori Yakovlevich Perelman.oga; born 13 June 1966) is a Russian mathemati ...
, 2006)
* Poincaré conjecture
In the mathematical field of geometric topology, the Poincaré conjecture (, , ) is a theorem about the characterization of the 3-sphere, which is the hypersphere that bounds the unit ball in four-dimensional space.
Originally conjectured b ...
(Grigori Perelman
Grigori Yakovlevich Perelman ( rus, links=no, Григорий Яковлевич Перельман, p=ɡrʲɪˈɡorʲɪj ˈjakəvlʲɪvʲɪtɕ pʲɪrʲɪlʲˈman, a=Ru-Grigori Yakovlevich Perelman.oga; born 13 June 1966) is a Russian mathemati ...
, 2002)Grigori Perelman
Grigori Yakovlevich Perelman ( rus, links=no, Григорий Яковлевич Перельман, p=ɡrʲɪˈɡorʲɪj ˈjakəvlʲɪvʲɪtɕ pʲɪrʲɪlʲˈman, a=Ru-Grigori Yakovlevich Perelman.oga; born 13 June 1966) is a Russian mathemati ...
,
Uncategorised
2010s
* Erdős discrepancy problem (Terence Tao, 2015)
* Umbral moonshine conjecture (John F. R. Duncan, Michael J. Griffin, Ken Ono, 2015)
* Anderson conjecture on the finite number of diffeomorphism classes of the collection of 4-manifolds satisfying certain properties (Jeff Cheeger, Aaron Naber, 2014)
* Gaussian correlation inequality (Thomas Royen, 2014)
* Beck's conjecture on discrepancies of set systems constructed from three permutations (Alantha Newman, Aleksandar Nikolov (computer scientist), Aleksandar Nikolov, 2011)
* Bloch–Kato conjecture (Vladimir Voevodsky, 2011) (and Quillen–Lichtenbaum conjecture and by work of Thomas Geisser (mathematician), Thomas Geisser and Marc Levine (mathematician), Marc Levine (2001) also Norm residue isomorphism theorem#Beilinson–Lichtenbaum conjecture, Beilinson–Lichtenbaum conjecture)
2000s
* Kauffman–Harary conjecture (Thomas Mattman, Pablo Solis, 2009)
* Surface subgroup conjecture (Jeremy Kahn, Vladimir Markovic, 2009)
* Normal scalar curvature conjecture and the Böttcher–Wenzel conjecture (Zhiqin Lu, 2007)
* Nirenberg–Treves conjecture (Nils Dencker, 2005)
* Peter Lax, Lax conjecture (Adrian Lewis (mathematician), Adrian Lewis, Pablo Parrilo, Motakuri Ramana, 2005)
* The Langlands–Shelstad fundamental lemma (Ngô Bảo Châu and Gérard Laumon, 2004)
* Milnor conjecture (Vladimir Voevodsky, 2003)
* Kirillov's conjecture (Ehud Baruch, 2003)
* Kouchnirenko’s conjecture (Bertrand Haas, 2002)
* n! conjecture, ''n''! conjecture (Mark Haiman, 2001) (and also Macdonald polynomials#The Macdonald positivity conjecture, Macdonald positivity conjecture)
* Kato's conjecture (Pascal Auscher, Steve Hofmann, Michael Lacey (mathematician), Michael Lacey, Alan Gaius Ramsay McIntosh, Alan McIntosh, and Philipp Tchamitchian, 2001)
* Deligne's conjecture on 1-motives (Luca Barbieri-Viale, Andreas Rosenschon, Morihiko Saito, 2001)
* Modularity theorem (Christophe Breuil, Brian Conrad, Fred Diamond, and Richard Taylor (mathematician), Richard Taylor, 2001)
* Erdős–Stewart conjecture (Florian Luca, 2001)
* Berry–Robbins problem (Michael Atiyah, 2000)[
]
See also
* List of conjectures
* List of unsolved problems in statistics
* List of unsolved problems in computer science
* List of unsolved problems in physics
* Lists of unsolved problems
* ''Open Problems in Mathematics''
* ''The Great Mathematical Problems''
*Scottish Book
References
Further reading
Books discussing problems solved since 1995
*
*
*
*
Books discussing unsolved problems
*
*
*
*
*
*
*
*
*
*
*
External links
24 Unsolved Problems and Rewards for them
List of links to unsolved problems in mathematics, prizes and research
Open Problem Garden
AIM Problem Lists
MathPro Press.
*
*
*
Unsolved Problems in Number Theory, Logic and Cryptography
The Open Problems Project (TOPP)
discrete and computational geometry problems
Kirby's list of unsolved problems in low-dimensional topology
Erdös' Problems on Graphs
Unsolved Problems in Virtual Knot Theory and Combinatorial Knot Theory
Open problems from the 12th International Conference on Fuzzy Set Theory and Its Applications
*
* Barry Simon'
15 Problems in Mathematical Physics
{{DEFAULTSORT:Unsolved problems in mathematics
Unsolved problems in mathematics,
Conjectures,
Lists of unsolved problems, Mathematics
Mathematics-related lists

 * Arnold–Givental conjecture and Arnold conjecture – relating symplectic geometry to Morse theory.
* Berry–Tabor conjecture in
* Arnold–Givental conjecture and Arnold conjecture – relating symplectic geometry to Morse theory.
* Berry–Tabor conjecture in  * The Hadwiger conjecture on covering ''n''-dimensional convex bodies with at most 2''n'' smaller copies
* Solving the
* The Hadwiger conjecture on covering ''n''-dimensional convex bodies with at most 2''n'' smaller copies
* Solving the  * Andrews–Curtis conjecture: every balanced
* Andrews–Curtis conjecture: every balanced  * ''n'' conjecture: a generalization of the ''abc'' conjecture to more than three integers.
** ''abc'' conjecture: for any , is true for only finitely many positive such that .
**
* ''n'' conjecture: a generalization of the ''abc'' conjecture to more than three integers.
** ''abc'' conjecture: for any , is true for only finitely many positive such that .
** 