Dipoles on:
[Wikipedia]
[Google]
[Amazon]
 In
In  A permanent magnet, such as a bar magnet, owes its magnetism to the intrinsic magnetic dipole moment of the electron. The two ends of a bar magnet are referred to as poles—not to be confused with monopoles, see
A permanent magnet, such as a bar magnet, owes its magnetism to the intrinsic magnetic dipole moment of the electron. The two ends of a bar magnet are referred to as poles—not to be confused with monopoles, see


 A ''physical dipole'' consists of two equal and opposite point charges: in the literal sense, two poles. Its field at large distances (i.e., distances large in comparison to the separation of the poles) depends almost entirely on the dipole moment as defined above. A ''point (electric) dipole'' is the limit obtained by letting the separation tend to 0 while keeping the dipole moment fixed. The field of a point dipole has a particularly simple form, and the order-1 term in the
A ''physical dipole'' consists of two equal and opposite point charges: in the literal sense, two poles. Its field at large distances (i.e., distances large in comparison to the separation of the poles) depends almost entirely on the dipole moment as defined above. A ''point (electric) dipole'' is the limit obtained by letting the separation tend to 0 while keeping the dipole moment fixed. The field of a point dipole has a particularly simple form, and the order-1 term in the
 Potassium bromide (KBr) has one of the highest dipole moments because it is an
Potassium bromide (KBr) has one of the highest dipole moments because it is an  The overall dipole moment of a molecule may be approximated as a vector sum of bond dipole moments. As a vector sum it depends on the relative orientation of the bonds, so that from the dipole moment information can be deduced about the molecular geometry.
For example, the zero dipole of CO2 implies that the two C=O bond dipole moments cancel so that the molecule must be linear. For H2O the O−H bond moments do not cancel because the molecule is bent. For ozone (O3) which is also a bent molecule, the bond dipole moments are not zero even though the O−O bonds are between similar atoms. This agrees with the Lewis structures for the resonance forms of ozone which show a positive charge on the central oxygen atom.
The overall dipole moment of a molecule may be approximated as a vector sum of bond dipole moments. As a vector sum it depends on the relative orientation of the bonds, so that from the dipole moment information can be deduced about the molecular geometry.
For example, the zero dipole of CO2 implies that the two C=O bond dipole moments cancel so that the molecule must be linear. For H2O the O−H bond moments do not cancel because the molecule is bent. For ozone (O3) which is also a bent molecule, the bond dipole moments are not zero even though the O−O bonds are between similar atoms. This agrees with the Lewis structures for the resonance forms of ozone which show a positive charge on the central oxygen atom.
 An example in organic chemistry of the role of geometry in determining dipole moment is the ''cis'' and ''trans'' isomers of
An example in organic chemistry of the role of geometry in determining dipole moment is the ''cis'' and ''trans'' isomers of
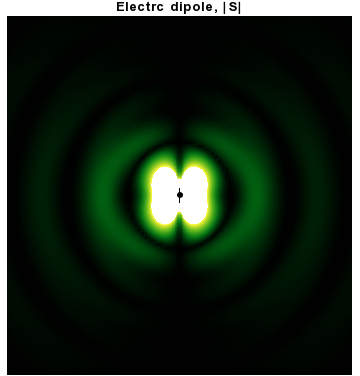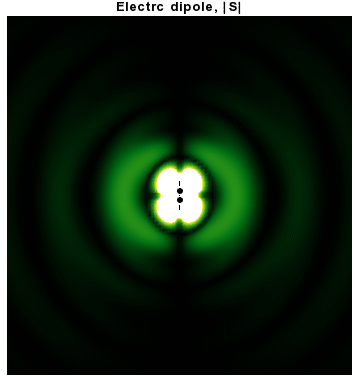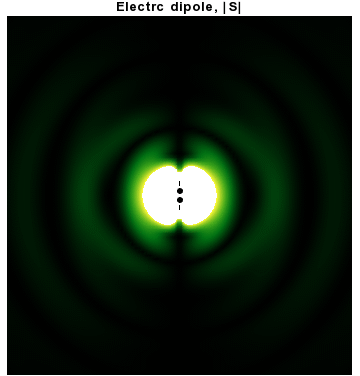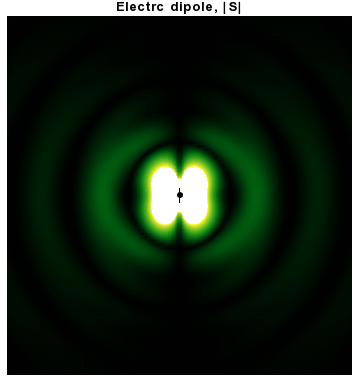 In addition to dipoles in electrostatics, it is also common to consider an electric or magnetic dipole that is oscillating in time. It is an extension, or a more physical next-step, to
In addition to dipoles in electrostatics, it is also common to consider an electric or magnetic dipole that is oscillating in time. It is an extension, or a more physical next-step, to
USGS Geomagnetism Program
a chapter from an online textbook
Electric Dipole Potential
by Stephen Wolfram an
Energy Density of a Magnetic Dipole
by Franz Krafft. Wolfram Demonstrations Project. Electromagnetism Potential theory
physics
Physics is the natural science that studies matter, its fundamental constituents, its motion and behavior through space and time, and the related entities of energy and force. "Physical science is that department of knowledge which r ...
, a dipole () is an electromagnetic phenomenon which occurs in two ways:
*An electric dipole deals with the separation of the positive and negative electric charge
Electric charge is the physical property of matter that causes charged matter to experience a force when placed in an electromagnetic field. Electric charge can be ''positive'' or ''negative'' (commonly carried by protons and electrons respe ...
s found in any electromagnetic system. A simple example of this system is a pair of charges of equal magnitude but opposite sign separated by some typically small distance. (A permanent electric dipole is called an electret.)
*A magnetic dipole is the closed circulation of an electric current
An electric current is a stream of charged particles, such as electrons or ions, moving through an electrical conductor or space. It is measured as the net rate of flow of electric charge through a surface or into a control volume. The moving pa ...
system. A simple example is a single loop of wire with constant current through it. A bar magnet
A magnet is a material or object that produces a magnetic field. This magnetic field is invisible but is responsible for the most notable property of a magnet: a force that pulls on other ferromagnetic materials, such as iron, steel, nickel, ...
is an example of a magnet with a permanent magnetic dipole moment
In electromagnetism, the magnetic moment is the magnetic strength and orientation of a magnet or other object that produces a magnetic field. Examples of objects that have magnetic moments include loops of electric current (such as electromagnets ...
.
Dipoles, whether electric or magnetic, can be characterized by their dipole moment, a vector quantity. For the simple electric dipole, the electric dipole moment
The electric dipole moment is a measure of the separation of positive and negative electrical charges within a system, that is, a measure of the system's overall polarity. The SI unit for electric dipole moment is the coulomb-meter (C⋅m). The ...
points from the negative charge towards the positive charge, and has a magnitude equal to the strength of each charge times the separation between the charges. (To be precise: for the definition of the dipole moment, one should always consider the "dipole limit", where, for example, the distance of the generating charges should ''converge'' to 0 while simultaneously, the charge strength should ''diverge'' to infinity in such a way that the product remains a positive constant.)
For the magnetic (dipole) current loop, the magnetic dipole moment
In electromagnetism, the magnetic moment is the magnetic strength and orientation of a magnet or other object that produces a magnetic field. Examples of objects that have magnetic moments include loops of electric current (such as electromagnets ...
points through the loop (according to the right hand grip rule), with a magnitude equal to the current in the loop times the area of the loop.
Similar to magnetic current loops, the electron
The electron ( or ) is a subatomic particle with a negative one elementary electric charge. Electrons belong to the first generation of the lepton particle family,
and are generally thought to be elementary particles because they have no kn ...
particle and some other fundamental particles have magnetic dipole moments, as an electron generates a magnetic field
A magnetic field is a vector field that describes the magnetic influence on moving electric charges, electric currents, and magnetic materials. A moving charge in a magnetic field experiences a force perpendicular to its own velocity and to ...
identical to that generated by a very small current loop. However, an electron's magnetic dipole moment is not due to a current loop, but to an intrinsic property of the electron. The electron may also have an ''electric'' dipole moment though such has yet to be observed (see electron electric dipole moment).
Classification Classification is a process related to categorization, the process in which ideas and objects are recognized, differentiated and understood.
Classification is the grouping of related facts into classes.
It may also refer to:
Business, organizat ...
below)—and may be labeled "north" and "south". In terms of the Earth's magnetic field, they are respectively "north-seeking" and "south-seeking" poles: if the magnet were freely suspended in the Earth's magnetic field, the north-seeking pole would point towards the north and the south-seeking pole would point towards the south. The dipole moment of the bar magnet points from its magnetic south
South is one of the cardinal directions or Points of the compass, compass points. The direction is the opposite of north and is perpendicular to both east and west.
Etymology
The word ''south'' comes from Old English ''sūþ'', from earlier Pro ...
to its magnetic north pole
The North Pole, also known as the Geographic North Pole or Terrestrial North Pole, is the point in the Northern Hemisphere where the Earth's axis of rotation meets its surface. It is called the True North Pole to distinguish from the Mag ...
. In a magnetic compass
A compass is a device that shows the cardinal directions used for navigation and geographic orientation. It commonly consists of a magnetized needle or other element, such as a compass card or compass rose, which can pivot to align itself with ...
, the north pole of a bar magnet points north. However, that means that Earth's geomagnetic north pole is the ''south'' pole (south-seeking pole) of its dipole moment and vice versa.
The only known mechanisms for the creation of magnetic dipoles are by current loops or quantum-mechanical spin
Spin or spinning most often refers to:
* Spinning (textiles), the creation of yarn or thread by twisting fibers together, traditionally by hand spinning
* Spin, the rotation of an object around a central axis
* Spin (propaganda), an intentionally b ...
since the existence of magnetic monopoles has never been experimentally demonstrated.
Classification
multipole expansion
A multipole expansion is a mathematical series representing a function that depends on angles—usually the two angles used in the spherical coordinate system (the polar and azimuthal angles) for three-dimensional Euclidean space, \R^3. Similarly ...
is precisely the point dipole field.
Although there are no known magnetic monopoles in nature, there are magnetic dipoles in the form of the quantum-mechanical spin
Spin or spinning most often refers to:
* Spinning (textiles), the creation of yarn or thread by twisting fibers together, traditionally by hand spinning
* Spin, the rotation of an object around a central axis
* Spin (propaganda), an intentionally b ...
associated with particles such as electron
The electron ( or ) is a subatomic particle with a negative one elementary electric charge. Electrons belong to the first generation of the lepton particle family,
and are generally thought to be elementary particles because they have no kn ...
s (although the accurate description of such effects falls outside of classical electromagnetism). A theoretical magnetic ''point dipole'' has a magnetic field of exactly the same form as the electric field of an electric point dipole. A very small current-carrying loop is approximately a magnetic point dipole; the magnetic dipole moment of such a loop is the product of the current flowing in the loop and the (vector) area of the loop.
Any configuration of charges or currents has a 'dipole moment', which describes the dipole whose field is the best approximation, at large distances, to that of the given configuration. This is simply one term in the multipole expansion when the total charge ("monopole moment") is 0—as it ''always'' is for the magnetic case, since there are no magnetic monopoles. The dipole term is the dominant one at large distances: Its field falls off in proportion to , as compared to for the next ( quadrupole) term and higher powers of for higher terms, or for the monopole term.
Molecular dipoles
Manymolecule
A molecule is a group of two or more atoms held together by attractive forces known as chemical bonds; depending on context, the term may or may not include ions which satisfy this criterion. In quantum physics, organic chemistry, and bioch ...
s have such dipole moments due to non-uniform distributions of positive and negative charges on the various atoms. Such is the case with polar compounds like hydrogen fluoride
Hydrogen fluoride (fluorane) is an inorganic compound with the chemical formula . This colorless gas or liquid is the principal industrial source of fluorine, often as an aqueous solution called hydrofluoric acid. It is an important feedstock i ...
(HF), where electron density is shared unequally between atoms. Therefore, a molecule's dipole is an electric dipole with an inherent electric field that should not be confused with a magnetic dipole, which generates a magnetic field.
The physical chemist Peter J. W. Debye
Peter Joseph William Debye (; ; March 24, 1884 – November 2, 1966) was a Dutch-American physicist and physical chemist, and Nobel laureate in Chemistry.
Biography
Early life
Born Petrus Josephus Wilhelmus Debije in Maastricht, Netherlands, D ...
was the first scientist to study molecular dipoles extensively, and, as a consequence, dipole moments are measured in the non- SI unit named ''debye
The debye (symbol: D) (; ) is a CGS unit (a non- SI metric unit) of electric dipole momentTwo equal and opposite charges separated by some distance constitute an electric dipole. This dipole possesses an electric dipole moment whose value is give ...
'' in his honor.
For molecules there are three types of dipoles:
; Permanent dipoles: These occur when two atoms in a molecule have substantially different electronegativity: One atom attracts electrons more than another, becoming more negative, while the other atom becomes more positive. A molecule with a permanent dipole moment is called a ''polar'' molecule. See dipole–dipole attraction
An intermolecular force (IMF) (or secondary force) is the force that mediates interaction between molecules, including the electromagnetic forces of attraction
or repulsion which act between atoms and other types of neighbouring particles, e.g. a ...
s.
; Instantaneous dipoles:These occur due to chance when electron
The electron ( or ) is a subatomic particle with a negative one elementary electric charge. Electrons belong to the first generation of the lepton particle family,
and are generally thought to be elementary particles because they have no kn ...
s happen to be more concentrated in one place than another in a molecule
A molecule is a group of two or more atoms held together by attractive forces known as chemical bonds; depending on context, the term may or may not include ions which satisfy this criterion. In quantum physics, organic chemistry, and bioch ...
, creating a temporary dipole. These dipoles are smaller in magnitude than permanent dipoles, but still play a large role in chemistry and biochemistry due to their prevalence. See instantaneous dipole.
; Induced dipoles: These can occur when one molecule with a permanent dipole repels another molecule's electrons, ''inducing'' a dipole moment in that molecule. A molecule is ''polarized'' when it carries an induced dipole. See induced-dipole attraction.
More generally, an induced dipole of ''any'' polarizable charge distribution ''ρ'' (remember that a molecule has a charge distribution) is caused by an electric field external to ''ρ''. This field may, for instance, originate from an ion or polar molecule in the vicinity of ''ρ'' or may be macroscopic (e.g., a molecule between the plates of a charged capacitor
A capacitor is a device that stores electrical energy in an electric field by virtue of accumulating electric charges on two close surfaces insulated from each other. It is a passive electronic component with two terminals.
The effect of ...
). The size of the induced dipole moment is equal to the product of the strength of the external field and the dipole polarizability of ''ρ''.
Dipole moment values can be obtained from measurement of the dielectric constant
The relative permittivity (in older texts, dielectric constant) is the permittivity of a material expressed as a ratio with the electric permittivity of a vacuum. A dielectric is an insulating material, and the dielectric constant of an insulat ...
. Some typical gas phase values in debye
The debye (symbol: D) (; ) is a CGS unit (a non- SI metric unit) of electric dipole momentTwo equal and opposite charges separated by some distance constitute an electric dipole. This dipole possesses an electric dipole moment whose value is give ...
units are:
* carbon dioxide
Carbon dioxide (chemical formula ) is a chemical compound made up of molecules that each have one carbon atom covalently double bonded to two oxygen atoms. It is found in the gas state at room temperature. In the air, carbon dioxide is transpar ...
: 0
* carbon monoxide
Carbon monoxide (chemical formula CO) is a colorless, poisonous, odorless, tasteless, flammable gas that is slightly less dense than air. Carbon monoxide consists of one carbon atom and one oxygen atom connected by a triple bond. It is the simple ...
: 0.112 D
* ozone
Ozone (), or trioxygen, is an inorganic molecule with the chemical formula . It is a pale blue gas with a distinctively pungent smell. It is an allotrope of oxygen that is much less stable than the diatomic allotrope , breaking down in the lo ...
: 0.53 D
* phosgene
Phosgene is the organic chemical compound with the formula COCl2. It is a toxic, colorless gas; in low concentrations, its musty odor resembles that of freshly cut hay or grass. Phosgene is a valued and important industrial building block, espe ...
: 1.17 D
* NH3 has a dipole moment of 1.42 D
* water vapor
(99.9839 °C)
, -
, Boiling point
,
, -
, specific gas constant
, 461.5 J/( kg·K)
, -
, Heat of vaporization
, 2.27 MJ/kg
, -
, Heat capacity
, 1.864 kJ/(kg·K)
Water vapor, water vapour or aqueous vapor is the gaseous pha ...
: 1.85 D
* hydrogen cyanide
Hydrogen cyanide, sometimes called prussic acid, is a chemical compound with the formula HCN and structure . It is a colorless, extremely poisonous, and flammable liquid that boils slightly above room temperature, at . HCN is produced on an ...
: 2.98 D
* cyanamide: 4.27 D
* potassium bromide
Potassium bromide ( K Br) is a salt, widely used as an anticonvulsant and a sedative in the late 19th and early 20th centuries, with over-the-counter use extending to 1975 in the US. Its action is due to the bromide ion (sodium bromide is equall ...
: 10.41 D
ionic compound
In chemistry, an ionic compound is a chemical compound composed of ions held together by electrostatic forces termed ionic bonding. The compound is neutral overall, but consists of positively charged ions called cations and negatively charged i ...
that exists as a molecule in the gas phase.
 An example in organic chemistry of the role of geometry in determining dipole moment is the ''cis'' and ''trans'' isomers of
An example in organic chemistry of the role of geometry in determining dipole moment is the ''cis'' and ''trans'' isomers of 1,2-dichloroethene
1,2-Dichloroethene, commonly called 1,2-dichloroethylene or 1,2-DCE, is the name for a pair of organochlorine compounds with the molecular formula CHCl. They are both colorless liquids with a sweet odor. It can exist as either of two geometric ...
. In the ''cis'' isomer the two polar C−Cl bonds are on the same side of the C=C double bond and the molecular dipole moment is 1.90 D. In the ''trans'' isomer, the dipole moment is zero because the two C−Cl bonds are on opposite sides of the C=C and cancel (and the two bond moments for the much less polar C−H bonds also cancel).
Another example of the role of molecular geometry is boron trifluoride
Boron trifluoride is the inorganic compound with the formula BF3. This pungent, colourless, and toxic gas forms white fumes in moist air. It is a useful Lewis acid and a versatile building block for other boron compounds.
Structure and bondin ...
, which has three polar bonds with a difference in electronegativity greater than the traditionally cited threshold of 1.7 for ionic bonding. However, due to the equilateral triangular distribution of the fluoride ions centered on and in the same plane as the boron cation, the symmetry of the molecule results in its dipole moment being zero.
Quantum mechanical dipole operator
Consider a collection of ''N'' particles with charges ''qi'' and position vectors r''i''. For instance, this collection may be a molecule consisting of electrons, all with charge −''e'', and nuclei with charge ''eZi'', where ''Zi'' is theatomic number
The atomic number or nuclear charge number (symbol ''Z'') of a chemical element is the charge number of an atomic nucleus. For ordinary nuclei, this is equal to the proton number (''n''p) or the number of protons found in the nucleus of every ...
of the ''i'' th nucleus.
The dipole observable (physical quantity) has the quantum mechanical dipole operator:
:
Notice that this definition is valid only for neutral atoms or molecules, i.e. total charge equal to zero. In the ionized case, we have
:
where is the center of mass of the molecule/group of particles.
Atomic dipoles
A non-degenerate (''S''-state) atom can have only a zero permanent dipole. This fact follows quantum mechanically from the inversion symmetry of atoms. All 3 components of the dipole operator are antisymmetric underinversion
Inversion or inversions may refer to:
Arts
* , a French gay magazine (1924/1925)
* ''Inversion'' (artwork), a 2005 temporary sculpture in Houston, Texas
* Inversion (music), a term with various meanings in music theory and musical set theory
* ...
with respect to the nucleus,
:
where is the dipole operator and is the inversion operator.
The permanent dipole moment of an atom in a non-degenerate state (see degenerate energy level) is given as the expectation (average) value of the dipole operator,
:
where is an ''S''-state, non-degenerate, wavefunction, which is symmetric or antisymmetric under inversion: . Since the product of the wavefunction (in the ket) and its complex conjugate (in the bra) is always symmetric under inversion and its inverse,
:
it follows that the expectation value changes sign under inversion. We used here the fact that , being a symmetry operator, is unitary
Unitary may refer to:
Mathematics
* Unitary divisor
* Unitary element
* Unitary group
* Unitary matrix
* Unitary morphism
* Unitary operator
* Unitary transformation
* Unitary representation
* Unitarity (physics)
* ''E''-unitary inverse semigroup ...
: and by definition the Hermitian adjoint may be moved from bra to ket and then becomes . Since the only quantity that is equal to minus itself is the zero, the expectation value vanishes,
:
In the case of open-shell atoms with degenerate energy levels, one could define a dipole moment by the aid of the first-order Stark effect
The Stark effect is the shifting and splitting of spectral lines of atoms and molecules due to the presence of an external electric field. It is the electric-field analogue of the Zeeman effect, where a spectral line is split into several compon ...
. This gives a non-vanishing dipole (by definition proportional to a non-vanishing first-order Stark shift) only if some of the wavefunctions belonging to the degenerate energies have opposite parity
Parity may refer to:
* Parity (computing)
** Parity bit in computing, sets the parity of data for the purpose of error detection
** Parity flag in computing, indicates if the number of set bits is odd or even in the binary representation of the r ...
; i.e., have different behavior under inversion. This is a rare occurrence, but happens for the excited H-atom, where 2s and 2p states are "accidentally" degenerate (see article Laplace–Runge–Lenz vector for the origin of this degeneracy) and have opposite parity (2s is even and 2p is odd).
Field of a static magnetic dipole
Magnitude
The far-field strength, ''B'', of a dipole magnetic field is given by : where :''B'' is the strength of the field, measured in teslas :''r'' is the distance from the center, measured in metres :''λ'' is the magnetic latitude (equal to 90° − ''θ'') where ''θ'' is the magnetic colatitude, measured inradian
The radian, denoted by the symbol rad, is the unit of angle in the International System of Units (SI) and is the standard unit of angular measure used in many areas of mathematics. The unit was formerly an SI supplementary unit (before that c ...
s or degree
Degree may refer to:
As a unit of measurement
* Degree (angle), a unit of angle measurement
** Degree of geographical latitude
** Degree of geographical longitude
* Degree symbol (°), a notation used in science, engineering, and mathematics
...
s from the dipole axisMagnetic colatitude is 0 along the dipole's axis and 90° in the plane perpendicular to its axis.
:''m'' is the dipole moment, measured in ampere
The ampere (, ; symbol: A), often shortened to amp,SI supports only the use of symbols and deprecates the use of abbreviations for units. is the unit of electric current in the International System of Units (SI). One ampere is equal to elect ...
-square metres or joule
The joule ( , ; symbol: J) is the unit of energy in the International System of Units (SI). It is equal to the amount of work done when a force of 1 newton displaces a mass through a distance of 1 metre in the direction of the force applied ...
s per tesla
:''μ''0 is the permeability of free space
The vacuum magnetic permeability (variously ''vacuum permeability'', ''permeability of free space'', ''permeability of vacuum''), also known as the magnetic constant, is the magnetic permeability in a classical vacuum. It is a physical constan ...
, measured in henries
The henry (symbol: H) is the unit of electrical inductance in the International System of Units (SI). If a current of 1 ampere flowing through a coil produces flux linkage of 1 weber turn, that coil has a self inductance of 1 henry. The unit ...
per metre.
Conversion to cylindrical coordinates is achieved using and
:
where ''ρ'' is the perpendicular distance from the ''z''-axis. Then,
:
Vector form
The field itself is a vector quantity: : where :B is the field :r is the vector from the position of the dipole to the position where the field is being measured :''r'' is the absolute value of r: the distance from the dipole :r̂ = is the unit vector parallel to r; :m is the (vector) dipole moment :''μ''0 is the permeability of free space : This is ''exactly'' the field of a point dipole, ''exactly'' the dipole term in the multipole expansion of an arbitrary field, and ''approximately'' the field of any dipole-like configuration at large distances.Magnetic vector potential
The vector potential A of a magnetic dipole is : with the same definitions as above.Field from an electric dipole
Theelectrostatic potential
Electrostatics is a branch of physics that studies electric charges at rest (static electricity).
Since classical times, it has been known that some materials, such as amber, attract lightweight particles after rubbing. The Greek word for amber ...
at position r due to an electric dipole at the origin is given by:
:
where p is the (vector) dipole moment, and ''є''0 is the permittivity of free space
Vacuum permittivity, commonly denoted (pronounced "epsilon nought" or "epsilon zero"), is the value of the absolute dielectric permittivity of classical vacuum. It may also be referred to as the permittivity of free space, the electric consta ...
.
This term appears as the second term in the multipole expansion
A multipole expansion is a mathematical series representing a function that depends on angles—usually the two angles used in the spherical coordinate system (the polar and azimuthal angles) for three-dimensional Euclidean space, \R^3. Similarly ...
of an arbitrary electrostatic potential Φ(r). If the source of Φ(r) is a dipole, as it is assumed here, this term is the only non-vanishing term in the multipole expansion of Φ(r). The electric field
An electric field (sometimes E-field) is the physical field that surrounds electrically charged particles and exerts force on all other charged particles in the field, either attracting or repelling them. It also refers to the physical field fo ...
from a dipole can be found from the gradient
In vector calculus, the gradient of a scalar-valued differentiable function of several variables is the vector field (or vector-valued function) \nabla f whose value at a point p is the "direction and rate of fastest increase". If the gradi ...
of this potential:
:
This is of the same form of the expression for the magnetic field of a point magnetic dipole, ignoring the delta function.
In a real electric dipole, however, the charges are physically separate and the electric field diverges or converges at the point charges.
This is different to the magnetic field of a real magnetic dipole which is continuous everywhere. The delta function represents the strong field pointing in the opposite direction between the point charges, which is often omitted since one is rarely interested in the field at the dipole's position.
For further discussions about the internal field of dipoles, see or Magnetic moment#Internal magnetic field of a dipole.
Torque on a dipole
Since the direction of anelectric field
An electric field (sometimes E-field) is the physical field that surrounds electrically charged particles and exerts force on all other charged particles in the field, either attracting or repelling them. It also refers to the physical field fo ...
is defined as the direction of the force on a positive charge, electric field lines point away from a positive charge and toward a negative charge.
When placed in a homogeneous electric or magnetic field
A magnetic field is a vector field that describes the magnetic influence on moving electric charges, electric currents, and magnetic materials. A moving charge in a magnetic field experiences a force perpendicular to its own velocity and to ...
, equal but opposite force
In physics, a force is an influence that can change the motion of an object. A force can cause an object with mass to change its velocity (e.g. moving from a state of rest), i.e., to accelerate. Force can also be described intuitively as a p ...
s arise on each side of the dipole creating a torque
In physics and mechanics, torque is the rotational equivalent of linear force. It is also referred to as the moment of force (also abbreviated to moment). It represents the capability of a force to produce change in the rotational motion of th ...
}:
:
for an electric dipole moment
The electric dipole moment is a measure of the separation of positive and negative electrical charges within a system, that is, a measure of the system's overall polarity. The SI unit for electric dipole moment is the coulomb-meter (C⋅m). The ...
p (in coulomb-meters), or
:
for a magnetic dipole moment
In electromagnetism, the magnetic moment is the magnetic strength and orientation of a magnet or other object that produces a magnetic field. Examples of objects that have magnetic moments include loops of electric current (such as electromagnets ...
m (in ampere-square meters).
The resulting torque will tend to align the dipole with the applied field, which in the case of an electric dipole, yields a potential energy of
:.
The energy of a magnetic dipole is similarly
:.
Dipole radiation
 In addition to dipoles in electrostatics, it is also common to consider an electric or magnetic dipole that is oscillating in time. It is an extension, or a more physical next-step, to
In addition to dipoles in electrostatics, it is also common to consider an electric or magnetic dipole that is oscillating in time. It is an extension, or a more physical next-step, to spherical wave
The (two-way) wave equation is a second-order linear partial differential equation for the description of waves or standing wave fields — as they occur in classical physics — such as mechanical waves (e.g. water waves, sound waves and seis ...
radiation.
In particular, consider a harmonically oscillating electric dipole, with angular frequency
In physics, angular frequency "''ω''" (also referred to by the terms angular speed, circular frequency, orbital frequency, radian frequency, and pulsatance) is a scalar measure of rotation rate. It refers to the angular displacement per unit tim ...
''ω'' and a dipole moment ''p''0 along the ẑ direction of the form
:
In vacuum, the exact field produced by this oscillating dipole can be derived using the retarded potential formulation as:
:
For ≫ 1, the far-field takes the simpler form of a radiating "spherical" wave, but with angular dependence embedded in the cross-product:David J. Griffiths
David Jeffrey Griffiths (born December 5, 1942) is an American physicist and educator. He worked at Reed College from 1978 through 2009, becoming the Howard Vollum Professor of Science before his retirement.
Biography
Griffiths is a graduate o ...
, Introduction to Electrodynamics, Prentice Hall, 1999, page 447
:
The time-averaged Poynting vector
In physics, the Poynting vector (or Umov–Poynting vector) represents the directional energy flux (the energy transfer per unit area per unit time) or '' power flow'' of an electromagnetic field. The SI unit of the Poynting vector is the watt ...
:
is not distributed isotropically, but concentrated around the directions lying perpendicular to the dipole moment, as a result of the non-spherical electric and magnetic waves. In fact, the spherical harmonic
In mathematics and physical science, spherical harmonics are special functions defined on the surface of a sphere. They are often employed in solving partial differential equations in many scientific fields.
Since the spherical harmonics form a ...
function (sin ''θ'') responsible for such toroidal angular distribution is precisely the ''l'' = 1 "p" wave.
The total time-average power radiated by the field can then be derived from the Poynting vector as
:
Notice that the dependence of the power on the fourth power of the frequency of the radiation is in accordance with the Rayleigh scattering, and the underlying effects why the sky consists of mainly blue colour.
A circular polarized dipole is described as a superposition of two linear dipoles.
See also
* Polarization density * Magnetic dipole models *Dipole model of the Earth's magnetic field
The dipole model of the Earth's magnetic field is a first order approximation of the rather complex true Earth's magnetic field. Due to effects of the interplanetary magnetic field (IMF), and the solar wind, the dipole model is particularly ina ...
* Electret
* Indian Ocean Dipole
The Indian Ocean Dipole (IOD), also known as the Indian Niño, is an irregular oscillation of sea surface temperatures in which the western Indian Ocean becomes alternately warmer (positive phase) and then colder (negative phase) than the eastern ...
and Subtropical Indian Ocean Dipole
The Subtropical Indian Ocean Dipole (SIOD) is featured by the oscillation of sea surface temperatures (SST) in which the southwest Indian Ocean i.e. south of Madagascar is warmer and then colder than the eastern part i.e. off Australia.Behera SK, ...
, two oceanographic phenomena
* Magnetic dipole-dipole interaction
Magnetism is the class of physical attributes that are mediated by a magnetic field, which refers to the capacity to induce attractive and repulsive phenomena in other entities. Electric currents and the magnetic moments of elementary particles ...
* Spin magnetic moment
* Monopole
* Solid harmonics In physics and mathematics, the solid harmonics are solutions of the Laplace equation in spherical polar coordinates, assumed to be (smooth) functions \mathbb^3 \to \mathbb. There are two kinds: the ''regular solid harmonics'' R^m_\ell(\mathbf), whi ...
* Axial multipole moments
Axial multipole moments are a series expansion of the electric potential of a charge distribution localized close to the origin along one Cartesian axis, denoted here as the ''z''-axis. However, the axial multipole expansion can also be applied ...
* Cylindrical multipole moments
* Spherical multipole moments
Spherical multipole moments are the coefficients in a series expansion
of a potential that varies inversely with the distance R to a source, ''i.e.'', as 1/''R''. Examples of such potentials are the electric potential, the magnetic potential a ...
* Laplace expansion
In linear algebra, the Laplace expansion, named after Pierre-Simon Laplace, also called cofactor expansion, is an expression of the determinant of an matrix as a weighted sum of minors, which are the determinants of some submatrices of . Spec ...
* Molecular solid
A molecular solid is a solid consisting of discrete molecules. The cohesive forces that bind the molecules together are van der Waals forces, dipole-dipole interactions, quadrupole interactions, π-π interactions, hydrogen bonding, halogen bon ...
* Magnetic moment#Internal magnetic field of a dipole
Notes
References
{{ReflistExternal links
USGS Geomagnetism Program
a chapter from an online textbook
Electric Dipole Potential
by Stephen Wolfram an
Energy Density of a Magnetic Dipole
by Franz Krafft. Wolfram Demonstrations Project. Electromagnetism Potential theory