decimal arithmetic on:
[Wikipedia]
[Google]
[Amazon]
The decimal
 Many
Many
 Most modern computer hardware and software systems commonly use a
Most modern computer hardware and software systems commonly use a
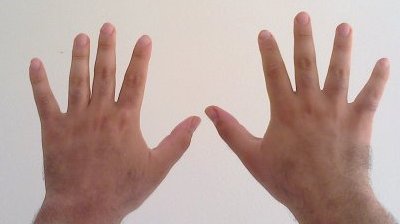
 Many ancient cultures calculated with numerals based on ten, sometimes argued due to human hands typically having ten fingers/digits. Standardized weights used in the Indus Valley civilization () were based on the ratios: 1/20, 1/10, 1/5, 1/2, 1, 2, 5, 10, 20, 50, 100, 200, and 500, while their standardized ruler – the ''Mohenjo-daro ruler'' – was divided into ten equal parts.
Many ancient cultures calculated with numerals based on ten, sometimes argued due to human hands typically having ten fingers/digits. Standardized weights used in the Indus Valley civilization () were based on the ratios: 1/20, 1/10, 1/5, 1/2, 1, 2, 5, 10, 20, 50, 100, 200, and 500, while their standardized ruler – the ''Mohenjo-daro ruler'' – was divided into ten equal parts. 
 Decimal fractions were first developed and used by the Chinese in the end of 4th century BCE, and then spread to the Middle East and from there to Europe. The written Chinese decimal fractions were non-positional. However, counting rod fractions were positional.
Decimal fractions were first developed and used by the Chinese in the end of 4th century BCE, and then spread to the Middle East and from there to Europe. The written Chinese decimal fractions were non-positional. However, counting rod fractions were positional.
Introduction to Old Norse
p. 293, gives number names that belong to this system. An expression cognate to 'one hundred and eighty' translates to 200, and the cognate to 'two hundred' translates to 240
Goodare
details the use of the long hundred in Scotland in the Middle Ages, giving examples such as calculations where the carry implies i C (i.e. one hundred) as 120, etc. That the general population were not alarmed to encounter such numbers suggests common enough use. It is also possible to avoid hundred-like numbers by using intermediate units, such as stones and pounds, rather than a long count of pounds. Goodare gives examples of numbers like vii score, where one avoids the hundred by using extended scores. There is also a paper by W.H. Stevenson, on 'Long Hundred and its uses in England'. * Many or all of the
numeral system
A numeral system (or system of numeration) is a writing system for expressing numbers; that is, a mathematical notation for representing numbers of a given set, using digits or other symbols in a consistent manner.
The same sequence of symbo ...
(also called the base-ten positional numeral system
Positional notation (or place-value notation, or positional numeral system) usually denotes the extension to any base of the Hindu–Arabic numeral system (or decimal system). More generally, a positional system is a numeral system in which th ...
and denary or decanary) is the standard system for denoting integer
An integer is the number zero (), a positive natural number (, , , etc.) or a negative integer with a minus sign ( −1, −2, −3, etc.). The negative numbers are the additive inverses of the corresponding positive numbers. In the languag ...
and non-integer number
A number is a mathematical object used to count, measure, and label. The original examples are the natural numbers 1, 2, 3, 4, and so forth. Numbers can be represented in language with number words. More universally, individual numbers c ...
s. It is the extension to non-integer numbers of the Hindu–Arabic numeral system
The Hindu–Arabic numeral system or Indo-Arabic numeral system Audun HolmeGeometry: Our Cultural Heritage 2000 (also called the Hindu numeral system or Arabic numeral system) is a positional decimal numeral system, and is the most common syste ...
. The way of denoting numbers in the decimal system is often referred to as ''decimal notation''.
A ''decimal numeral'' (also often just ''decimal'' or, less correctly, ''decimal number''), refers generally to the notation of a number in the decimal numeral system. Decimals may sometimes be identified by a decimal separator
A decimal separator is a symbol used to separate the integer part from the fractional part of a number written in decimal form (e.g., "." in 12.45). Different countries officially designate different symbols for use as the separator. The choi ...
(usually "." or "," as in or ). ''Decimal'' may also refer specifically to the digits after the decimal separator, such as in " is the approximation of to ''two decimals''". Zero-digits after a decimal separator serve the purpose of signifying the precision of a value.
The numbers that may be represented in the decimal system are the decimal fractions
The decimal numeral system (also called the base-ten positional numeral system and denary or decanary) is the standard system for denoting integer and non-integer numbers. It is the extension to non-integer numbers of the Hindu–Arabic numeral ...
. That is, fractions
A fraction (from la, fractus, "broken") represents a part of a whole or, more generally, any number of equal parts. When spoken in everyday English, a fraction describes how many parts of a certain size there are, for example, one-half, eight ...
of the form , where is an integer, and is a non-negative integer
In mathematics, the natural numbers are those numbers used for counting (as in "there are ''six'' coins on the table") and ordering (as in "this is the ''third'' largest city in the country").
Numbers used for counting are called '' cardinal ...
.
The decimal system has been extended to ''infinite decimals'' for representing any real number
In mathematics, a real number is a number that can be used to measure a ''continuous'' one-dimensional quantity such as a distance, duration or temperature. Here, ''continuous'' means that values can have arbitrarily small variations. Every ...
, by using an infinite sequence
In mathematics, a sequence is an enumerated collection of objects in which repetitions are allowed and order matters. Like a set, it contains members (also called ''elements'', or ''terms''). The number of elements (possibly infinite) is called t ...
of digits after the decimal separator (see decimal representation
A decimal representation of a non-negative real number is its expression as a sequence of symbols consisting of decimal digits traditionally written with a single separator:
r = b_k b_\ldots b_0.a_1a_2\ldots
Here is the decimal separator, i ...
). In this context, the decimal numerals with a finite number of non-zero digits after the decimal separator are sometimes called ''terminating decimals''. A ''repeating decimal
A repeating decimal or recurring decimal is decimal representation of a number whose digits are periodic (repeating its values at regular intervals) and the infinitely repeated portion is not zero. It can be shown that a number is rational if a ...
'' is an infinite decimal that, after some place, repeats indefinitely the same sequence of digits (e.g., ). An infinite decimal represents a rational number
In mathematics, a rational number is a number that can be expressed as the quotient or fraction of two integers, a numerator and a non-zero denominator . For example, is a rational number, as is every integer (e.g. ). The set of all rat ...
, the quotient
In arithmetic, a quotient (from lat, quotiens 'how many times', pronounced ) is a quantity produced by the division of two numbers. The quotient has widespread use throughout mathematics, and is commonly referred to as the integer part of a ...
of two integers, if and only if it is a repeating decimal or has a finite number of non-zero digits.
Origin
 Many
Many numeral system
A numeral system (or system of numeration) is a writing system for expressing numbers; that is, a mathematical notation for representing numbers of a given set, using digits or other symbols in a consistent manner.
The same sequence of symbo ...
s of ancient civilizations use ten and its powers for representing numbers, possibly because there are ten fingers on two hands and people started counting by using their fingers. Examples are firstly the Egyptian numerals
The system of ancient Egyptian numerals was used in Ancient Egypt from around 3000 BCE until the early first millennium CE. It was a system of numeration based on multiples of ten, often rounded off to the higher power, written in hieroglyphs. Th ...
, then the Brahmi numerals
The Brahmi numerals are a numeral system attested from the 3rd century BCE (somewhat later in the case of most of the tens). They are a non positional decimal system. They are the direct graphic ancestors of the modern Hindu–Arabic numeral s ...
, Greek numerals
Greek numerals, also known as Ionic, Ionian, Milesian, or Alexandrian numerals, are a system of writing numbers using the letters of the Greek alphabet. In modern Greece, they are still used for ordinal numbers and in contexts similar to tho ...
, Hebrew numerals, Roman numerals, and Chinese numerals
Chinese numerals are words and characters used to denote numbers in Chinese.
Today, speakers of Chinese use three written numeral systems: the system of Arabic numerals used worldwide, and two indigenous systems. The more familiar indigenous s ...
. Very large numbers were difficult to represent in these old numeral systems, and only the best mathematicians were able to multiply or divide large numbers. These difficulties were completely solved with the introduction of the Hindu–Arabic numeral system
The Hindu–Arabic numeral system or Indo-Arabic numeral system Audun HolmeGeometry: Our Cultural Heritage 2000 (also called the Hindu numeral system or Arabic numeral system) is a positional decimal numeral system, and is the most common syste ...
for representing integer
An integer is the number zero (), a positive natural number (, , , etc.) or a negative integer with a minus sign ( −1, −2, −3, etc.). The negative numbers are the additive inverses of the corresponding positive numbers. In the languag ...
s. This system has been extended to represent some non-integer numbers, called ''decimal fractions
The decimal numeral system (also called the base-ten positional numeral system and denary or decanary) is the standard system for denoting integer and non-integer numbers. It is the extension to non-integer numbers of the Hindu–Arabic numeral ...
'' or ''decimal numbers'', for forming the ''decimal numeral system''.
Decimal notation
For writing numbers, the decimal system uses tendecimal digit
A numerical digit (often shortened to just digit) is a single symbol used alone (such as "2") or in combinations (such as "25"), to represent numbers in a positional numeral system. The name "digit" comes from the fact that the ten digits (Latin ...
s, a decimal mark
A decimal separator is a symbol used to separate the integer part from the fractional part of a number written in decimal form (e.g., "." in 12.45). Different countries officially designate different symbols for use as the separator. The choi ...
, and, for negative numbers, a minus sign
The plus and minus signs, and , are mathematical symbols used to represent the notions of positive and negative, respectively. In addition, represents the operation of addition, which results in a sum, while represents subtraction, resul ...
"−". The decimal digits are 0, 1, 2, 3, 4, 5, 6, 7, 8, 9; the decimal separator
A decimal separator is a symbol used to separate the integer part from the fractional part of a number written in decimal form (e.g., "." in 12.45). Different countries officially designate different symbols for use as the separator. The choi ...
is the dot "" in many countries (mostly English-speaking), and a comma "" in other countries.
For representing a non-negative number
In mathematics, the sign of a real number is its property of being either positive, negative, or zero. Depending on local conventions, zero may be considered as being neither positive nor negative (having no sign or a unique third sign), or it ...
, a decimal numeral consists of
* either a (finite) sequence of digits (such as "2017"), where the entire sequence represents an integer,
*:
*or a decimal mark separating two sequences of digits (such as "20.70828")
::.
If , that is, if the first sequence contains at least two digits, it is generally assumed that the first digit is not zero. In some circumstances it may be useful to have one or more 0's on the left; this does not change the value represented by the decimal: for example, . Similarly, if the final digit on the right of the decimal mark is zero—that is, if —it may be removed; conversely, trailing zeros may be added after the decimal mark without changing the represented number; for example, and .
For representing a negative number, a minus sign is placed before .
The numeral represents the number
:.
The ''integer part
In mathematics and computer science, the floor function is the function that takes as input a real number , and gives as output the greatest integer less than or equal to , denoted or . Similarly, the ceiling function maps to the least int ...
'' or ''integral part'' of a decimal numeral is the integer written to the left of the decimal separator (see also truncation). For a non-negative decimal numeral, it is the largest integer that is not greater than the decimal. The part from the decimal separator to the right is the '' fractional part'', which equals the difference between the numeral and its integer part.
When the integral part of a numeral is zero, it may occur, typically in computing
Computing is any goal-oriented activity requiring, benefiting from, or creating computing machinery. It includes the study and experimentation of algorithmic processes, and development of both hardware and software. Computing has scientific, ...
, that the integer part is not written (for example, , instead of ). In normal writing, this is generally avoided, because of the risk of confusion between the decimal mark and other punctuation.
In brief, the contribution of each digit to the value of a number depends on its position in the numeral. That is, the decimal system is a positional numeral system
Positional notation (or place-value notation, or positional numeral system) usually denotes the extension to any base of the Hindu–Arabic numeral system (or decimal system). More generally, a positional system is a numeral system in which th ...
.
Decimal fractions
Decimal fractions (sometimes called decimal numbers, especially in contexts involving explicit fractions) are therational number
In mathematics, a rational number is a number that can be expressed as the quotient or fraction of two integers, a numerator and a non-zero denominator . For example, is a rational number, as is every integer (e.g. ). The set of all rat ...
s that may be expressed as a fraction whose denominator
A fraction (from la, fractus, "broken") represents a part of a whole or, more generally, any number of equal parts. When spoken in everyday English, a fraction describes how many parts of a certain size there are, for example, one-half, eight ...
is a power
Power most often refers to:
* Power (physics), meaning "rate of doing work"
** Engine power, the power put out by an engine
** Electric power
* Power (social and political), the ability to influence people or events
** Abusive power
Power may a ...
of ten. For example, the decimals represent the fractions , , , and , and are therefore decimal numbers.
More generally, a decimal with digits after the separator (a point or comma) represents the fraction with denominator , whose numerator is the integer obtained by removing the separator.
It follows that a number is a decimal fraction if and only if
In logic and related fields such as mathematics and philosophy, "if and only if" (shortened as "iff") is a biconditional logical connective between statements, where either both statements are true or both are false.
The connective is b ...
it has a finite decimal representation.
Expressed as a fully reduced fraction
An irreducible fraction (or fraction in lowest terms, simplest form or reduced fraction) is a fraction in which the numerator and denominator are integers that have no other common divisors than 1 (and −1, when negative numbers are considered). ...
, the decimal numbers are those whose denominator is a product of a power of 2 and a power of 5. Thus the smallest denominators of decimal numbers are
:
Real number approximation
Decimal numerals do not allow an exact representation for allreal number
In mathematics, a real number is a number that can be used to measure a ''continuous'' one-dimensional quantity such as a distance, duration or temperature. Here, ''continuous'' means that values can have arbitrarily small variations. Every ...
s, e.g. for the real number . Nevertheless, they allow approximating every real number with any desired accuracy, e.g., the decimal 3.14159 approximates the real , being less than 10−5 off; so decimals are widely used in science
Science is a systematic endeavor that Scientific method, builds and organizes knowledge in the form of Testability, testable explanations and predictions about the universe.
Science may be as old as the human species, and some of the earli ...
, engineering
Engineering is the use of scientific principles to design and build machines, structures, and other items, including bridges, tunnels, roads, vehicles, and buildings. The discipline of engineering encompasses a broad range of more speciali ...
and everyday life.
More precisely, for every real number and every positive integer , there are two decimals and with at most ' digits after the decimal mark such that and .
Numbers are very often obtained as the result of measurement. As measurements are subject to measurement uncertainty with a known upper bound
In mathematics, particularly in order theory, an upper bound or majorant of a subset of some preordered set is an element of that is greater than or equal to every element of .
Dually, a lower bound or minorant of is defined to be an eleme ...
, the result of a measurement is well-represented by a decimal with digits after the decimal mark, as soon as the absolute measurement error is bounded from above by . In practice, measurement results are often given with a certain number of digits after the decimal point, which indicate the error bounds. For example, although 0.080 and 0.08 denote the same number, the decimal numeral 0.080 suggests a measurement with an error less than 0.001, while the numeral 0.08 indicates an absolute error bounded by 0.01. In both cases, the true value of the measured quantity could be, for example, 0.0803 or 0.0796 (see also significant figures
Significant figures (also known as the significant digits, ''precision'' or ''resolution'') of a number in positional notation are digits in the number that are reliable and necessary to indicate the quantity of something.
If a number expres ...
).
Infinite decimal expansion
For areal number
In mathematics, a real number is a number that can be used to measure a ''continuous'' one-dimensional quantity such as a distance, duration or temperature. Here, ''continuous'' means that values can have arbitrarily small variations. Every ...
and an integer , let denote the (finite) decimal expansion of the greatest number that is not greater than ' that has exactly digits after the decimal mark. Let denote the last digit of . It is straightforward to see that may be obtained by appending to the right of . This way one has
:,
and the difference of and amounts to
:,
which is either 0, if , or gets arbitrarily small as ' tends to infinity. According to the definition of a limit
Limit or Limits may refer to:
Arts and media
* ''Limit'' (manga), a manga by Keiko Suenobu
* ''Limit'' (film), a South Korean film
* Limit (music), a way to characterize harmony
* "Limit" (song), a 2016 single by Luna Sea
* "Limits", a 2019 ...
, ' is the limit of when ' tends to infinity. This is written asor
: ,
which is called an infinite decimal expansion of '.
Conversely, for any integer and any sequence of digits the (infinite) expression is an ''infinite decimal expansion'' of a real number '. This expansion is unique if neither all are equal to 9 nor all are equal to 0 for ' large enough (for all ' greater than some natural number ).
If all for equal to 9 and , the limit of the sequence is the decimal fraction obtained by replacing the last digit that is not a 9, i.e.: , by , and replacing all subsequent 9s by 0s (see 0.999...).
Any such decimal fraction, i.e.: for , may be converted to its equivalent infinite decimal expansion by replacing by and replacing all subsequent 0s by 9s (see 0.999...).
In summary, every real number that is not a decimal fraction has a unique infinite decimal expansion. Each decimal fraction has exactly two infinite decimal expansions, one containing only 0s after some place, which is obtained by the above definition of , and the other containing only 9s after some place, which is obtained by defining as the greatest number that is ''less'' than , having exactly ' digits after the decimal mark.
Rational numbers
Long division
In arithmetic, long division is a standard division algorithm suitable for dividing multi-digit Hindu-Arabic numerals (Positional notation) that is simple enough to perform by hand. It breaks down a division problem into a series of easier steps ...
allows computing the infinite decimal expansion of a rational number
In mathematics, a rational number is a number that can be expressed as the quotient or fraction of two integers, a numerator and a non-zero denominator . For example, is a rational number, as is every integer (e.g. ). The set of all rat ...
. If the rational number is a decimal fraction
The decimal numeral system (also called the base-ten positional numeral system and denary or decanary) is the standard system for denoting integer and non-integer numbers. It is the extension to non-integer numbers of the Hindu–Arabic num ...
, the division stops eventually, producing a decimal numeral, which may be prolongated into an infinite expansion by adding infinitely many zeros. If the rational number is not a decimal fraction, the division may continue indefinitely. However, as all successive remainders are less than the divisor, there are only a finite number of possible remainders, and after some place, the same sequence of digits must be repeated indefinitely in the quotient. That is, one has a ''repeating decimal''. For example,
: = 0.012345679012... (with the group 012345679 indefinitely repeating).
The converse is also true: if, at some point in the decimal representation of a number, the same string of digits starts repeating indefinitely, the number is rational.
or, dividing both numerator and denominator by 6, .
Decimal computation
binary representation
A binary number is a number expressed in the base-2 numeral system or binary numeral system, a method of mathematical expression which uses only two symbols: typically "0" (zero) and "1" (one).
The base-2 numeral system is a positional notation ...
internally (although many early computers, such as the ENIAC
ENIAC (; Electronic Numerical Integrator and Computer) was the first programmable, electronic, general-purpose digital computer, completed in 1945. There were other computers that had these features, but the ENIAC had all of them in one pac ...
or the IBM 650, used decimal representation internally).
For external use by computer specialists, this binary representation is sometimes presented in the related octal
The octal numeral system, or oct for short, is the base-8 number system, and uses the digits 0 to 7. This is to say that 10octal represents eight and 100octal represents sixty-four. However, English, like most languages, uses a base-10 number ...
or hexadecimal systems.
For most purposes, however, binary values are converted to or from the equivalent decimal values for presentation to or input from humans; computer programs express literals in decimal by default. (123.1, for example, is written as such in a computer program, even though many computer languages are unable to encode that number precisely.)
Both computer hardware and software also use internal representations which are effectively decimal for storing decimal values and doing arithmetic. Often this arithmetic is done on data which are encoded using some variant of binary-coded decimal, especially in database implementations, but there are other decimal representations in use (including decimal floating point
Decimal floating-point (DFP) arithmetic refers to both a representation and operations on decimal floating-point numbers. Working directly with decimal (base-10) fractions can avoid the rounding errors that otherwise typically occur when convert ...
such as in newer revisions of the IEEE 754 Standard for Floating-Point Arithmetic).
Decimal arithmetic is used in computers so that decimal fractional results of adding (or subtracting) values with a fixed length of their fractional part always are computed to this same length of precision. This is especially important for financial calculations, e.g., requiring in their results integer multiples of the smallest currency unit for book keeping purposes. This is not possible in binary, because the negative powers of have no finite binary fractional representation; and is generally impossible for multiplication (or division). See Arbitrary-precision arithmetic
In computer science, arbitrary-precision arithmetic, also called bignum arithmetic, multiple-precision arithmetic, or sometimes infinite-precision arithmetic, indicates that calculations are performed on numbers whose digits of precision are li ...
for exact calculations.
History
 Many ancient cultures calculated with numerals based on ten, sometimes argued due to human hands typically having ten fingers/digits. Standardized weights used in the Indus Valley civilization () were based on the ratios: 1/20, 1/10, 1/5, 1/2, 1, 2, 5, 10, 20, 50, 100, 200, and 500, while their standardized ruler – the ''Mohenjo-daro ruler'' – was divided into ten equal parts.
Many ancient cultures calculated with numerals based on ten, sometimes argued due to human hands typically having ten fingers/digits. Standardized weights used in the Indus Valley civilization () were based on the ratios: 1/20, 1/10, 1/5, 1/2, 1, 2, 5, 10, 20, 50, 100, 200, and 500, while their standardized ruler – the ''Mohenjo-daro ruler'' – was divided into ten equal parts. Egyptian hieroglyphs
Egyptian hieroglyphs (, ) were the formal writing system used in Ancient Egypt, used for writing the Egyptian language. Hieroglyphs combined logographic, syllabic and alphabetic elements, with some 1,000 distinct characters.There were about 1, ...
, in evidence since around 3000 BCE, used a purely decimal system, as did the Cretan hieroglyphs
Cretan hieroglyphs are a hieroglyphic writing system used in early Bronze Age Crete, during the Minoan era. They predate Linear A by about a century, but the two writing systems continued to be used in parallel for most of their history. , the ...
() of the Minoans whose numerals are closely based on the Egyptian model. The decimal system was handed down to the consecutive Bronze Age cultures of Greece, including Linear A
Linear A is a writing system that was used by the Minoans of Crete from 1800 to 1450 BC to write the hypothesized Minoan language or languages. Linear A was the primary script used in palace and religious writings of the Minoan civi ...
(c. 18th century BCE−1450 BCE) and Linear B (c. 1375−1200 BCE) – the number system of classical Greece also used powers of ten, including, Roman numerals, an intermediate base of 5. Notably, the polymath Archimedes (c. 287–212 BCE) invented a decimal positional system in his Sand Reckoner
''The Sand Reckoner'' ( el, Ψαμμίτης, ''Psammites'') is a work by Archimedes, an Ancient Greek mathematician of the 3rd century BC, in which he set out to determine an upper bound for the number of grains of sand that fit into the unive ...
which was based on 108 and later led the German mathematician Carl Friedrich Gauss
Johann Carl Friedrich Gauss (; german: Gauß ; la, Carolus Fridericus Gauss; 30 April 177723 February 1855) was a German mathematician and physicist who made significant contributions to many fields in mathematics and science. Sometimes refer ...
to lament what heights science would have already reached in his days if Archimedes had fully realized the potential of his ingenious discovery. Hittite hieroglyphs (since 15th century BCE) were also strictly decimal.
Some non-mathematical ancient texts such as the Vedas
upright=1.2, The Vedas are ancient Sanskrit texts of Hinduism. Above: A page from the '' Atharvaveda''.
The Vedas (, , ) are a large body of religious texts originating in ancient India. Composed in Vedic Sanskrit, the texts constitute th ...
, dating back to 1700–900 BCE make use of decimals and mathematical decimal fractions.
The Egyptian hieratic numerals, the Greek alphabet numerals, the Hebrew alphabet numerals, the Roman numerals, the Chinese numerals and early Indian Brahmi numerals are all non-positional decimal systems, and required large numbers of symbols. For instance, Egyptian numerals used different symbols for 10, 20 to 90, 100, 200 to 900, 1000, 2000, 3000, 4000, to 10,000.
The world's earliest positional decimal system was the Chinese rod calculus Rod calculus or rod calculation was the mechanical method of algorithmic computation with counting rods in China from the Warring States to Ming dynasty before the counting rods were increasingly replaced by the more convenient and faster abacus. Ro ...
.
History of decimal fractions
 Decimal fractions were first developed and used by the Chinese in the end of 4th century BCE, and then spread to the Middle East and from there to Europe. The written Chinese decimal fractions were non-positional. However, counting rod fractions were positional.
Decimal fractions were first developed and used by the Chinese in the end of 4th century BCE, and then spread to the Middle East and from there to Europe. The written Chinese decimal fractions were non-positional. However, counting rod fractions were positional.Lam Lay Yong
Lam Lay Yong (maiden name Oon Lay Yong, ; born 1936) is a retired Professor of Mathematics.
Academic career
From 1988 to 1996 she was Professor at the Department of Mathematics, National University of Singapore (NUS). She graduated from the Uni ...
, "The Development of Hindu–Arabic and Traditional Chinese Arithmetic", ''Chinese Science'', 1996 p. 38, Kurt Vogel notation
Qin Jiushao
Qin Jiushao (, ca. 1202–1261), courtesy name Daogu (道古), was a Chinese mathematician, meteorologist, inventor, politician, and writer. He is credited for discovering Horner's method as well as inventing Tianchi basins, a type of rain gau ...
in his book Mathematical Treatise in Nine Sections
The ''Mathematical Treatise in Nine Sections'' () is a mathematical text written by Chinese Southern Song dynasty mathematician Qin Jiushao in the year 1247. The mathematical text has a wide range of topics and is taken from all aspects of th ...
(1247) denoted 0.96644 by
:::::
::::: , meaning
:::::
:::::096644
J. Lennart Berggren notes that positional decimal fractions appear for the first time in a book by the Arab mathematician Abu'l-Hasan al-Uqlidisi Abu'l Hasan Ahmad ibn Ibrahim Al-Uqlidisi ( ar, أبو الحسن أحمد بن ابراهيم الإقليدسي) was a Muslim Arab mathematician, who was active in Damascus and Baghdad. He wrote the earliest surviving book on the positional use ...
written in the 10th century. The Jewish mathematician Immanuel Bonfils Immanuel ben Jacob Bonfils (c. 1300 – 1377) was a French-Jewish mathematician and astronomer in medieval times who flourished from 1340 to 1377, a rabbi who was a pioneer of exponential calculus and is credited with inventing the system of decimal ...
used decimal fractions around 1350, anticipating Simon Stevin
Simon Stevin (; 1548–1620), sometimes called Stevinus, was a Flemish mathematician, scientist and music theorist. He made various contributions in many areas of science and engineering, both theoretical and practical. He also translated vario ...
, but did not develop any notation to represent them. The Persian mathematician Jamshīd al-Kāshī
Ghiyāth al-Dīn Jamshīd Masʿūd al-Kāshī (or al-Kāshānī) ( fa, غیاث الدین جمشید کاشانی ''Ghiyās-ud-dīn Jamshīd Kāshānī'') (c. 1380 Kashan, Iran – 22 June 1429 Samarkand, Transoxania) was a Persian astronomer ...
claimed to have discovered decimal fractions himself in the 15th century. Al Khwarizmi
Muḥammad ibn Mūsā al-Khwārizmī ( ar, محمد بن موسى الخوارزمي, Muḥammad ibn Musā al-Khwārazmi; ), or al-Khwarizmi, was a Persian polymath from Khwarazm, who produced vastly influential works in mathematics, astronom ...
introduced fraction to Islamic countries in the early 9th century; a Chinese author has alleged that his fraction presentation was an exact copy of traditional Chinese mathematical fraction from Sunzi Suanjing
''Sunzi Suanjing'' () was a mathematical treatise written during 3rd to 5th centuries AD which was listed as one of the Ten Computational Canons during the Tang dynasty. The specific identity of its author Sunzi (lit. "Master Sun") is still ...
. This form of fraction with numerator on top and denominator at bottom without a horizontal bar was also used by al-Uqlidisi and by al-Kāshī in his work "Arithmetic Key".
A forerunner of modern European decimal notation was introduced by Simon Stevin
Simon Stevin (; 1548–1620), sometimes called Stevinus, was a Flemish mathematician, scientist and music theorist. He made various contributions in many areas of science and engineering, both theoretical and practical. He also translated vario ...
in the 16th century.
John Napier
John Napier of Merchiston (; 1 February 1550 – 4 April 1617), nicknamed Marvellous Merchiston, was a Scottish landowner known as a mathematician, physicist, and astronomer. He was the 8th Laird of Merchiston. His Latinized name was Ioan ...
introduced using the period (.) to separate the integer part of a decimal number from the fractional part in his book on constructing tables of logarithms, published posthumously in 1620.
Natural languages
A method of expressing every possiblenatural number
In mathematics, the natural numbers are those numbers used for counting (as in "there are ''six'' coins on the table") and ordering (as in "this is the ''third'' largest city in the country").
Numbers used for counting are called ''cardinal ...
using a set of ten symbols emerged in India. Several Indian languages show a straightforward decimal system. Many Indo-Aryan and Dravidian languages
The Dravidian languages (or sometimes Dravidic) are a family of languages spoken by 250 million people, mainly in southern India, north-east Sri Lanka, and south-west Pakistan. Since the colonial era, there have been small but significant ...
have numbers between 10 and 20 expressed in a regular pattern of addition to 10.
The Hungarian language
Hungarian () is an Uralic language spoken in Hungary and parts of several neighbouring countries. It is the official language of Hungary and one of the 24 official languages of the European Union. Outside Hungary, it is also spoken by Hungarian ...
also uses a straightforward decimal system. All numbers between 10 and 20 are formed regularly (e.g. 11 is expressed as "tizenegy" literally "one on ten"), as with those between 20 and 100 (23 as "huszonhárom" = "three on twenty").
A straightforward decimal rank system with a word for each order (10 , 100 , 1000 , 10,000 ), and in which 11 is expressed as ''ten-one'' and 23 as ''two-ten-three'', and 89,345 is expressed as 8 (ten thousands) 9 (thousand) 3 (hundred) 4 (tens) 5 is found in Chinese
Chinese can refer to:
* Something related to China
* Chinese people, people of Chinese nationality, citizenship, and/or ethnicity
**''Zhonghua minzu'', the supra-ethnic concept of the Chinese nation
** List of ethnic groups in China, people of ...
, and in Vietnamese
Vietnamese may refer to:
* Something of, from, or related to Vietnam, a country in Southeast Asia
** A citizen of Vietnam. See Demographics of Vietnam.
* Vietnamese people, or Kinh people, a Southeast Asian ethnic group native to Vietnam
** Overse ...
with a few irregularities. Japanese
Japanese may refer to:
* Something from or related to Japan, an island country in East Asia
* Japanese language, spoken mainly in Japan
* Japanese people, the ethnic group that identifies with Japan through ancestry or culture
** Japanese diaspor ...
, Korean
Korean may refer to:
People and culture
* Koreans, ethnic group originating in the Korean Peninsula
* Korean cuisine
* Korean culture
* Korean language
**Korean alphabet, known as Hangul or Chosŏn'gŭl
**Korean dialects and the Jeju language
** ...
, and Thai have imported the Chinese decimal system. Many other languages with a decimal system have special words for the numbers between 10 and 20, and decades. For example, in English 11 is "eleven" not "ten-one" or "one-teen".
Incan languages such as Quechua
Quechua may refer to:
*Quechua people, several indigenous ethnic groups in South America, especially in Peru
*Quechuan languages, a Native South American language family spoken primarily in the Andes, derived from a common ancestral language
**So ...
and Aymara
Aymara may refer to:
Languages and people
* Aymaran languages, the second most widespread Andean language
** Aymara language, the main language within that family
** Central Aymara, the other surviving branch of the Aymara(n) family, which today ...
have an almost straightforward decimal system, in which 11 is expressed as ''ten with one'' and 23 as ''two-ten with three''.
Some psychologists suggest irregularities of the English names of numerals may hinder children's counting ability.
Other bases
Some cultures do, or did, use other bases of numbers. *Pre-Columbian
In the history of the Americas, the pre-Columbian era spans from the original settlement of North and South America in the Upper Paleolithic period through European colonization, which began with Christopher Columbus's voyage of 1492. Usually, ...
Mesoamerica
Mesoamerica is a historical region and cultural area in southern North America and most of Central America. It extends from approximately central Mexico through Belize, Guatemala, El Salvador, Honduras, Nicaragua, and northern Costa Rica ...
n cultures such as the Maya
Maya may refer to:
Civilizations
* Maya peoples, of southern Mexico and northern Central America
** Maya civilization, the historical civilization of the Maya peoples
** Maya language, the languages of the Maya peoples
* Maya (Ethiopia), a popul ...
used a base-20
vigesimal () or base-20 (base-score) numeral system is based on twenty (in the same way in which the decimal numeral system is based on ten). ''Vigesimal'' is derived from the Latin adjective '' vicesimus'', meaning 'twentieth'.
Places
In ...
system (perhaps based on using all twenty fingers and toe
Toes are the digits (fingers) of the foot of a tetrapod. Animal species such as cats that walk on their toes are described as being ''digitigrade''. Humans, and other animals that walk on the soles of their feet, are described as being ''plan ...
s).
* The Yuki language in California
California is a state in the Western United States, located along the Pacific Coast. With nearly 39.2million residents across a total area of approximately , it is the most populous U.S. state and the 3rd largest by area. It is also the m ...
and the Pamean languages in Mexico
Mexico (Spanish: México), officially the United Mexican States, is a country in the southern portion of North America. It is bordered to the north by the United States; to the south and west by the Pacific Ocean; to the southeast by Guatema ...
have octal
The octal numeral system, or oct for short, is the base-8 number system, and uses the digits 0 to 7. This is to say that 10octal represents eight and 100octal represents sixty-four. However, English, like most languages, uses a base-10 number ...
( base-8) systems because the speakers count using the spaces between their fingers rather than the fingers themselves.
* The existence of a non-decimal base in the earliest traces of the Germanic languages is attested by the presence of words and glosses meaning that the count is in decimal (cognates to "ten-count" or "tenty-wise"); such would be expected if normal counting is not decimal, and unusual if it were. Where this counting system is known, it is based on the "long hundred" = 120, and a "long thousand" of 1200. The descriptions like "long" only appear after the "small hundred" of 100 appeared with the Christians. Gordon'Introduction to Old Norse
p. 293, gives number names that belong to this system. An expression cognate to 'one hundred and eighty' translates to 200, and the cognate to 'two hundred' translates to 240
Goodare
details the use of the long hundred in Scotland in the Middle Ages, giving examples such as calculations where the carry implies i C (i.e. one hundred) as 120, etc. That the general population were not alarmed to encounter such numbers suggests common enough use. It is also possible to avoid hundred-like numbers by using intermediate units, such as stones and pounds, rather than a long count of pounds. Goodare gives examples of numbers like vii score, where one avoids the hundred by using extended scores. There is also a paper by W.H. Stevenson, on 'Long Hundred and its uses in England'. * Many or all of the
Chumashan languages
Chumashan was a family of languages that were spoken on the southern California coast by Native American Chumash people, from the Coastal plains and valleys of San Luis Obispo to Malibu, neighboring inland and Transverse Ranges valleys and ca ...
originally used a base-4 counting system, in which the names for numbers were structured according to multiples of 4 and 16.
* Many languages use quinary (base-5) number systems, including Gumatj, Nunggubuyu, Kuurn Kopan Noot and Saraveca
Saraveca is an extinct Arawakan language once spoken in Bolivia by the Sarave. It is saidEncyclopædia Britannica, "Numerals and numeral systems". to be the only language with a numeral system based exclusively on five, although quinary systems e ...
. Of these, Gumatj is the only true 5–25 language known, in which 25 is the higher group of 5.
* Some Nigeria
Nigeria ( ), , ig, Naìjíríyà, yo, Nàìjíríà, pcm, Naijá , ff, Naajeeriya, kcg, Naijeriya officially the Federal Republic of Nigeria, is a country in West Africa. It is situated between the Sahel to the north and the Gulf o ...
ns use duodecimal systems. So did some small communities in India and Nepal, as indicated by their languages.
* The Huli language
Huli is a Tari language spoken by the Huli people of the Hela Province of Papua New Guinea. It has a pentadecimal (base-15) numeral system: means 15, means 15×2 = 30, and means 15×15 = 225.
Huli has a pandanus language called (bush di ...
of Papua New Guinea
Papua New Guinea (abbreviated PNG; , ; tpi, Papua Niugini; ho, Papua Niu Gini), officially the Independent State of Papua New Guinea ( tpi, Independen Stet bilong Papua Niugini; ho, Independen Stet bilong Papua Niu Gini), is a country i ...
is reported to have base-15
There are many different numeral systems, that is, writing systems for expressing numbers.
By culture / time period
By type of notation
Numeral systems are classified here as to whether they use positional notation (also known as place-value ...
numbers. ''Ngui'' means 15, ''ngui ki'' means 15 × 2 = 30, and ''ngui ngui'' means 15 × 15 = 225.
* Umbu-Ungu
Kaugel (Gawigl) is one of the languages spoken in the Southern Highlands province of Papua New Guinea. Native speakers call the area on the Southern Highlands side of the Kaugel River from the Western Highlands province home.
Dialects are Aua ...
, also known as Kakoli, is reported to have base 24, base-24 numbers. ''Tokapu'' means 24, ''tokapu talu'' means 24 × 2 = 48, and ''tokapu tokapu'' means 24 × 24 = 576.
* Ngiti language, Ngiti is reported to have a base 32, base-32 number system with base-4 cycles.
* The Ndom language of Papua New Guinea
Papua New Guinea (abbreviated PNG; , ; tpi, Papua Niugini; ho, Papua Niu Gini), officially the Independent State of Papua New Guinea ( tpi, Independen Stet bilong Papua Niugini; ho, Independen Stet bilong Papua Niu Gini), is a country i ...
is reported to have base-6 numerals. ''Mer'' means 6, ''mer an thef'' means 6 × 2 = 12, ''nif'' means 36, and ''nif thef'' means 36×2 = 72.
See also
Notes
References
{{Authority control Elementary arithmetic Fractions (mathematics) Positional numeral systems