Coreolis Effect on:
[Wikipedia]
[Google]
[Amazon]
 In
In
 Italian scientist
Italian scientist
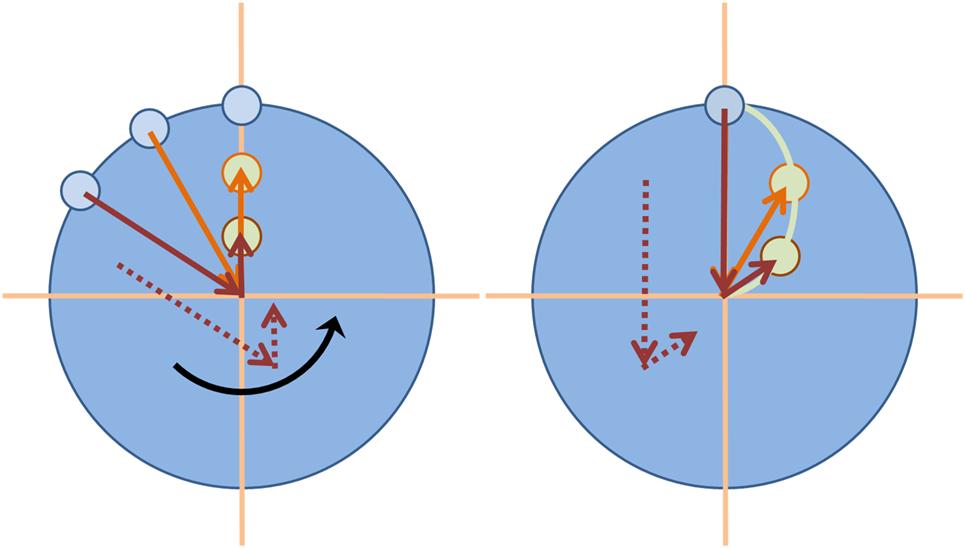 The figure illustrates a ball tossed from 12:00 o'clock toward the center of a counter-clockwise rotating carousel. On the left, the ball is seen by a stationary observer above the carousel, and the ball travels in a straight line to the center, while the ball-thrower rotates counter-clockwise with the carousel. On the right, the ball is seen by an observer rotating with the carousel, so the ball-thrower appears to stay at 12:00 o'clock. The figure shows how the trajectory of the ball as seen by the rotating observer can be constructed.
On the left, two arrows locate the ball relative to the ball-thrower. One of these arrows is from the thrower to the center of the carousel (providing the ball-thrower's line of sight), and the other points from the center of the carousel to the ball. (This arrow gets shorter as the ball approaches the center.) A shifted version of the two arrows is shown dotted.
On the right is shown this same dotted pair of arrows, but now the pair are rigidly rotated so the arrow corresponding to the line of sight of the ball-thrower toward the center of the carousel is aligned with 12:00 o'clock. The other arrow of the pair locates the ball relative to the center of the carousel, providing the position of the ball as seen by the rotating observer. By following this procedure for several positions, the trajectory in the rotating frame of reference is established as shown by the curved path in the right-hand panel.
The ball travels in the air, and there is no net force upon it. To the stationary observer, the ball follows a straight-line path, so there is no problem squaring this trajectory with zero net force. However, the rotating observer sees a ''curved'' path. Kinematics insists that a force (pushing to the ''right'' of the instantaneous direction of travel for a ''counter-clockwise'' rotation) must be present to cause this curvature, so the rotating observer is forced to invoke a combination of centrifugal and Coriolis forces to provide the net force required to cause the curved trajectory.
The figure illustrates a ball tossed from 12:00 o'clock toward the center of a counter-clockwise rotating carousel. On the left, the ball is seen by a stationary observer above the carousel, and the ball travels in a straight line to the center, while the ball-thrower rotates counter-clockwise with the carousel. On the right, the ball is seen by an observer rotating with the carousel, so the ball-thrower appears to stay at 12:00 o'clock. The figure shows how the trajectory of the ball as seen by the rotating observer can be constructed.
On the left, two arrows locate the ball relative to the ball-thrower. One of these arrows is from the thrower to the center of the carousel (providing the ball-thrower's line of sight), and the other points from the center of the carousel to the ball. (This arrow gets shorter as the ball approaches the center.) A shifted version of the two arrows is shown dotted.
On the right is shown this same dotted pair of arrows, but now the pair are rigidly rotated so the arrow corresponding to the line of sight of the ball-thrower toward the center of the carousel is aligned with 12:00 o'clock. The other arrow of the pair locates the ball relative to the center of the carousel, providing the position of the ball as seen by the rotating observer. By following this procedure for several positions, the trajectory in the rotating frame of reference is established as shown by the curved path in the right-hand panel.
The ball travels in the air, and there is no net force upon it. To the stationary observer, the ball follows a straight-line path, so there is no problem squaring this trajectory with zero net force. However, the rotating observer sees a ''curved'' path. Kinematics insists that a force (pushing to the ''right'' of the instantaneous direction of travel for a ''counter-clockwise'' rotation) must be present to cause this curvature, so the rotating observer is forced to invoke a combination of centrifugal and Coriolis forces to provide the net force required to cause the curved trajectory.
 The figure describes a more complex situation where the tossed ball on a turntable bounces off the edge of the carousel and then returns to the tosser, who catches the ball. The effect of Coriolis force on its trajectory is shown again as seen by two observers: an observer (referred to as the "camera") that rotates with the carousel, and an inertial observer. The figure shows a bird's-eye view based upon the same ball speed on forward and return paths. Within each circle, plotted dots show the same time points. In the left panel, from the camera's viewpoint at the center of rotation, the tosser (smiley face) and the rail both are at fixed locations, and the ball makes a very considerable arc on its travel toward the rail, and takes a more direct route on the way back. From the ball tosser's viewpoint, the ball seems to return more quickly than it went (because the tosser is rotating toward the ball on the return flight).
On the carousel, instead of tossing the ball straight at a rail to bounce back, the tosser must throw the ball toward the right of the target and the ball then seems to the camera to bear continuously to the left of its direction of travel to hit the rail (''left'' because the carousel is turning ''clockwise''). The ball appears to bear to the left from direction of travel on both inward and return trajectories. The curved path demands this observer to recognize a leftward net force on the ball. (This force is "fictitious" because it disappears for a stationary observer, as is discussed shortly.) For some angles of launch, a path has portions where the trajectory is approximately radial, and Coriolis force is primarily responsible for the apparent deflection of the ball (centrifugal force is radial from the center of rotation, and causes little deflection on these segments). When a path curves away from radial, however, centrifugal force contributes significantly to deflection.
The ball's path through the air is straight when viewed by observers standing on the ground (right panel). In the right panel (stationary observer), the ball tosser (smiley face) is at 12 o'clock and the rail the ball bounces from is at position 1. From the inertial viewer's standpoint, positions 1, 2, and 3 are occupied in sequence. At position 2, the ball strikes the rail, and at position 3, the ball returns to the tosser. Straight-line paths are followed because the ball is in free flight, so this observer requires that no net force is applied.
The figure describes a more complex situation where the tossed ball on a turntable bounces off the edge of the carousel and then returns to the tosser, who catches the ball. The effect of Coriolis force on its trajectory is shown again as seen by two observers: an observer (referred to as the "camera") that rotates with the carousel, and an inertial observer. The figure shows a bird's-eye view based upon the same ball speed on forward and return paths. Within each circle, plotted dots show the same time points. In the left panel, from the camera's viewpoint at the center of rotation, the tosser (smiley face) and the rail both are at fixed locations, and the ball makes a very considerable arc on its travel toward the rail, and takes a more direct route on the way back. From the ball tosser's viewpoint, the ball seems to return more quickly than it went (because the tosser is rotating toward the ball on the return flight).
On the carousel, instead of tossing the ball straight at a rail to bounce back, the tosser must throw the ball toward the right of the target and the ball then seems to the camera to bear continuously to the left of its direction of travel to hit the rail (''left'' because the carousel is turning ''clockwise''). The ball appears to bear to the left from direction of travel on both inward and return trajectories. The curved path demands this observer to recognize a leftward net force on the ball. (This force is "fictitious" because it disappears for a stationary observer, as is discussed shortly.) For some angles of launch, a path has portions where the trajectory is approximately radial, and Coriolis force is primarily responsible for the apparent deflection of the ball (centrifugal force is radial from the center of rotation, and causes little deflection on these segments). When a path curves away from radial, however, centrifugal force contributes significantly to deflection.
The ball's path through the air is straight when viewed by observers standing on the ground (right panel). In the right panel (stationary observer), the ball tosser (smiley face) is at 12 o'clock and the rail the ball bounces from is at position 1. From the inertial viewer's standpoint, positions 1, 2, and 3 are occupied in sequence. At position 2, the ball strikes the rail, and at position 3, the ball returns to the tosser. Straight-line paths are followed because the ball is in free flight, so this observer requires that no net force is applied.
 Consider a location with latitude ''φ'' on a sphere that is rotating around the north–south axis. A local coordinate system is set up with the ''x'' axis horizontally due east, the ''y'' axis horizontally due north and the ''z'' axis vertically upwards. The rotation vector, velocity of movement and Coriolis acceleration expressed in this local coordinate system (listing components in the order east (''e''), north (''n'') and upward (''u'')) are:
:
:
When considering atmospheric or oceanic dynamics, the vertical velocity is small, and the vertical component of the Coriolis acceleration () is small compared with the acceleration due to gravity (g, approximately near Earth's surface). For such cases, only the horizontal (east and north) components matter. The restriction of the above to the horizontal plane is (setting ''vu'' = 0):
:
where is called the Coriolis parameter.
By setting ''vn'' = 0, it can be seen immediately that (for positive φ and ω) a movement due east results in an acceleration due south. Similarly, setting ''ve'' = 0, it is seen that a movement due north results in an acceleration due east.
In general, observed horizontally, looking along the direction of the movement causing the acceleration, the acceleration always is turned 90° to the right (for positive φ) and of the same size regardless of the horizontal orientation.
As a different case, consider equatorial motion setting φ = 0°. In this case, Ω is parallel to the north or ''n''-axis, and:
:
Accordingly, an eastward motion (that is, in the same direction as the rotation of the sphere) provides an upward acceleration known as the
Consider a location with latitude ''φ'' on a sphere that is rotating around the north–south axis. A local coordinate system is set up with the ''x'' axis horizontally due east, the ''y'' axis horizontally due north and the ''z'' axis vertically upwards. The rotation vector, velocity of movement and Coriolis acceleration expressed in this local coordinate system (listing components in the order east (''e''), north (''n'') and upward (''u'')) are:
:
:
When considering atmospheric or oceanic dynamics, the vertical velocity is small, and the vertical component of the Coriolis acceleration () is small compared with the acceleration due to gravity (g, approximately near Earth's surface). For such cases, only the horizontal (east and north) components matter. The restriction of the above to the horizontal plane is (setting ''vu'' = 0):
:
where is called the Coriolis parameter.
By setting ''vn'' = 0, it can be seen immediately that (for positive φ and ω) a movement due east results in an acceleration due south. Similarly, setting ''ve'' = 0, it is seen that a movement due north results in an acceleration due east.
In general, observed horizontally, looking along the direction of the movement causing the acceleration, the acceleration always is turned 90° to the right (for positive φ) and of the same size regardless of the horizontal orientation.
As a different case, consider equatorial motion setting φ = 0°. In this case, Ω is parallel to the north or ''n''-axis, and:
:
Accordingly, an eastward motion (that is, in the same direction as the rotation of the sphere) provides an upward acceleration known as the


 Perhaps the most important impact of the Coriolis effect is in the large-scale dynamics of the oceans and the atmosphere. In meteorology and oceanography, it is convenient to postulate a rotating frame of reference wherein the Earth is stationary. In accommodation of that provisional postulation, the centrifugal and Coriolis forces are introduced. Their relative importance is determined by the applicable Rossby numbers.
Perhaps the most important impact of the Coriolis effect is in the large-scale dynamics of the oceans and the atmosphere. In meteorology and oceanography, it is convenient to postulate a rotating frame of reference wherein the Earth is stationary. In accommodation of that provisional postulation, the centrifugal and Coriolis forces are introduced. Their relative importance is determined by the applicable Rossby numbers.
 # The train travels toward the west: In that case, it moves against the direction of rotation. Therefore, on the Earth's rotating frame the Coriolis term is pointed inwards towards the axis of rotation (down). This additional force downwards should cause the train to be heavier while moving in that direction.If one looks at this train from the fixed non-rotating frame on top of the center of the Earth, at that speed it remains stationary as the Earth spins beneath it. Hence, the only force acting on it is
# The train travels toward the west: In that case, it moves against the direction of rotation. Therefore, on the Earth's rotating frame the Coriolis term is pointed inwards towards the axis of rotation (down). This additional force downwards should cause the train to be heavier while moving in that direction.If one looks at this train from the fixed non-rotating frame on top of the center of the Earth, at that speed it remains stationary as the Earth spins beneath it. Hence, the only force acting on it is  From the fixed inertial frame of reference above Earth, the train traveling east now rotates at twice the rate as when it was at rest—so the amount of centripetal force needed to cause that circular path increases leaving less force from gravity to act on the track. This is what the Coriolis term accounts for on the previous paragraph.As a final check one can imagine a frame of reference rotating along with the train. Such frame would be rotating at twice the angular velocity as Earth's rotating frame. The resulting
From the fixed inertial frame of reference above Earth, the train traveling east now rotates at twice the rate as when it was at rest—so the amount of centripetal force needed to cause that circular path increases leaving less force from gravity to act on the track. This is what the Coriolis term accounts for on the previous paragraph.As a final check one can imagine a frame of reference rotating along with the train. Such frame would be rotating at twice the angular velocity as Earth's rotating frame. The resulting
 The Coriolis force on a moving
The Coriolis force on a moving

 To demonstrate the Coriolis effect, a parabolic turntable can be used.
On a flat turntable, the inertia of a co-rotating object forces it off the edge. However, if the turntable surface has the correct
To demonstrate the Coriolis effect, a parabolic turntable can be used.
On a flat turntable, the inertia of a co-rotating object forces it off the edge. However, if the turntable surface has the correct
Geophysical fluid dynamics lab demonstration
John Marshall, Massachusetts Institute of Technology.For a java applet of the Coriolis effect on such a parabolic surface, se
School of Meteorology at the University of Oklahoma. Discs cut from cylinders of
Original book
Original article
Original article
Durran, D. R.
1993:
Is the Coriolis force really responsible for the inertial oscillation?
', Bull. Amer. Meteor. Soc., 74, pp. 2179–2184; Corrigenda. Bulletin of the American Meteorological Society, 75, p. 261 * Durran, D. R., and S. K. Domonkos, 1996:
An apparatus for demonstrating the inertial oscillation
', Bulletin of the American Meteorological Society, 77, pp. 557–559. * Marion, Jerry B. 1970, ''Classical Dynamics of Particles and Systems'', Academic Press. * Persson, A., 1998
How do we Understand the Coriolis Force?'' Bulletin of the American Meteorological Society 79, pp. 1373–1385. * Symon, Keith. 1971, ''Mechanics'', Addison–Wesley
Akira Kageyama & Mamoru Hyodo: ''Eulerian derivation of the Coriolis force''
James F. Price: ''A Coriolis tutorial''
Woods Hole Oceanographic Institute (2003) * . Elementary, non-mathematical; but well written.
1997: ''The Fontana History of the Mathematical Sciences''. Fontana, 817 pp. 710 pp. * Khrgian, A., 1970: ''Meteorology: A Historical Survey''. Vol. 1. Keter Press, 387 pp. * Kuhn, T. S., 1977: Energy conservation as an example of simultaneous discovery. ''The Essential Tension, Selected Studies in Scientific Tradition and Change'', University of Chicago Press, 66–104. * Kutzbach, G., 1979: ''The Thermal Theory of Cyclones. A History of Meteorological Thought in the Nineteenth Century''. Amer. Meteor. Soc., 254 pp.
The definition of the Coriolis effect from the Glossary of Meteorology
The Coriolis Effect — a conflict between common sense and mathematics
PDF-file. 20 pages. A general discussion by Anders Persson of various aspects of the coriolis effect, including Foucault's Pendulum and Taylor columns.
The coriolis effect in meteorology
PDF-file. 5 pages. A detailed explanation by Mats Rosengren of how the gravitational force and the rotation of the Earth affect the atmospheric motion over the Earth surface. 2 figures
from the About.com Weather Page
– from ScienceWorld
''Coriolis Effect and Drains''
An article from the NEWTON web site hosted by the
Catalog of Coriolis videos
''Coriolis Effect: A graphical animation''
a visual Earth animation with precise explanation
''An introduction to fluid dynamics''
SPINLab Educational Film explains the Coriolis effect with the aid of lab experiments
by Cecil Adams.
An article uncovering misinformation about the Coriolis effect. By Alistair B. Fraser, Emeritus Professor of Meteorology at
''The Coriolis Effect: A (Fairly) Simple Explanation''
an explanation for the layperson
Observe an animation of the Coriolis effect over Earth's surface
Animation clip
showing scenes as viewed from both an inertial frame and a rotating frame of reference, visualizing the Coriolis and centrifugal forces.
lets you control rotation speed, droplet speed and frame of reference to explore the Coriolis effect.
Rotating Co-ordinating Systems
transformation from inertial systems {{DEFAULTSORT:Coriolis Force Classical mechanics Force Atmospheric dynamics Physical phenomena Fictitious forces Rotation
 In
In physics
Physics is the natural science that studies matter, its fundamental constituents, its motion and behavior through space and time, and the related entities of energy and force. "Physical science is that department of knowledge which rel ...
, the Coriolis force is an inertial or fictitious force that acts on objects in motion within a frame of reference that rotates with respect to an inertial frame
In classical physics and special relativity, an inertial frame of reference (also called inertial reference frame, inertial frame, inertial space, or Galilean reference frame) is a frame of reference that is not undergoing any acceleration. ...
. In a reference frame with clockwise
Two-dimensional rotation can occur in two possible directions. Clockwise motion (abbreviated CW) proceeds in the same direction as a clock's hands: from the top to the right, then down and then to the left, and back up to the top. The opposite ...
rotation, the force acts to the left of the motion of the object. In one with anticlockwise (or counterclockwise) rotation, the force acts to the right. Deflection
Deflection or deflexion may refer to:
Board games
* Deflection (chess), a tactic that forces an opposing chess piece to leave a square
* Khet (game), formerly ''Deflexion'', an Egyptian-themed chess-like game using lasers
Mechanics
* Deflectio ...
of an object due to the Coriolis force is called the Coriolis effect. Though recognized previously by others, the mathematical expression for the Coriolis force appeared in an 1835 paper by French scientist Gaspard-Gustave de Coriolis, in connection with the theory of water wheel
A water wheel is a machine for converting the energy of flowing or falling water into useful forms of power, often in a watermill. A water wheel consists of a wheel (usually constructed from wood or metal), with a number of blades or buck ...
s. Early in the 20th century, the term ''Coriolis force'' began to be used in connection with meteorology
Meteorology is a branch of the atmospheric sciences (which include atmospheric chemistry and physics) with a major focus on weather forecasting. The study of meteorology dates back millennia, though significant progress in meteorology did no ...
.
Newton's laws of motion
Newton's laws of motion are three basic laws of classical mechanics that describe the relationship between the motion of an object and the forces acting on it. These laws can be paraphrased as follows:
# A body remains at rest, or in moti ...
describe the motion of an object in an inertial (non-accelerating) frame of reference. When Newton's laws are transformed to a rotating frame of reference, the Coriolis and centrifugal accelerations appear. When applied to objects with masses, the respective forces are proportional to their mass
Mass is an intrinsic property of a body. It was traditionally believed to be related to the quantity of matter in a physical body, until the discovery of the atom and particle physics. It was found that different atoms and different element ...
es. The magnitude of the Coriolis force is proportional to the rotation rate, and the magnitude of the centrifugal force is proportional to the square of the rotation rate. The Coriolis force acts in a direction perpendicular to two quantities: the angular velocity
In physics, angular velocity or rotational velocity ( or ), also known as angular frequency vector,(UP1) is a pseudovector representation of how fast the angular position or orientation of an object changes with time (i.e. how quickly an objec ...
of the rotating frame relative to the inertial frame and the velocity of the body relative to the rotating frame, and its magnitude is proportional to the object's speed in the rotating frame (more precisely, to the component of its velocity that is perpendicular to the axis of rotation). The centrifugal force acts outwards in the radial direction and is proportional to the distance of the body from the axis of the rotating frame. These additional forces are termed inertial forces, fictitious force
A fictitious force is a force that appears to act on a mass whose motion is described using a non-inertial frame of reference, such as a linearly accelerating or rotating reference frame.
It is related to Newton's second law of motion, which t ...
s, or ''pseudo forces''. By introducing these fictitious forces to a rotating frame of reference, Newton's laws of motion can be applied to the rotating system as though it were an inertial system; these forces are correction factors that are not required in a non-rotating system.
In popular (non-technical) usage of the term "Coriolis effect", the rotating reference frame implied is almost always the Earth
Earth is the third planet from the Sun and the only astronomical object known to harbor life. While large volumes of water can be found throughout the Solar System, only Earth sustains liquid surface water. About 71% of Earth's surf ...
. Because the Earth spins, Earth-bound observers need to account for the Coriolis force to correctly analyze the motion of objects. The Earth completes one rotation for each day/night cycle, so for motions of everyday objects the Coriolis force is usually quite small compared with other forces; its effects generally become noticeable only for motions occurring over large distances and long periods of time, such as large-scale movement of air in the atmosphere or water in the ocean; or where high precision is important, such as long-range artillery or missile trajectories. Such motions are constrained by the surface of the Earth, so only the horizontal component of the Coriolis force is generally important. This force causes moving objects on the surface of the Earth to be deflected to the right (with respect to the direction of travel) in the Northern Hemisphere and to the left in the Southern Hemisphere. The horizontal deflection effect is greater near the poles, since the effective rotation rate about a local vertical axis is largest there, and decreases to zero at the equator
The equator is a circle of latitude, about in circumference, that divides Earth into the Northern and Southern hemispheres. It is an imaginary line located at 0 degrees latitude, halfway between the North and South poles. The term can al ...
. Rather than flowing directly from areas of high pressure to low pressure, as they would in a non-rotating system, winds and currents tend to flow to the right of this direction north of the equator
The equator is a circle of latitude, about in circumference, that divides Earth into the Northern and Southern hemispheres. It is an imaginary line located at 0 degrees latitude, halfway between the North and South poles. The term can al ...
(anticlockwise) and to the left of this direction south of it (clockwise). This effect is responsible for the rotation and thus formation of cyclones
In meteorology, a cyclone () is a large air mass that rotates around a strong center of low atmospheric pressure, counterclockwise in the Northern Hemisphere and clockwise in the Southern Hemisphere as viewed from above (opposite to an ant ...
(see Coriolis effects in meteorology).
For an intuitive explanation of the origin of the Coriolis force, consider an object, constrained to follow the Earth's surface and moving northward in the Northern Hemisphere. Viewed from outer space, the object does not appear to go due north, but has an eastward motion (it rotates around toward the right along with the surface of the Earth). The further north it travels, the smaller the "radius of its parallel (latitude)" (the minimum distance from the surface point to the axis of rotation, which is in a plane orthogonal to the axis), and so the slower the eastward motion of its surface. As the object moves north, to higher latitudes, it has a tendency to maintain the eastward speed it started with (rather than slowing down to match the reduced eastward speed of local objects on the Earth's surface), so it veers east (i.e. to the right of its initial motion).
Though not obvious from this example, which considers northward motion, the horizontal deflection occurs equally for objects moving eastward or westward (or in any other direction). However, the theory that the effect determines the rotation of draining water in a typical size household bathtub, sink or toilet has been repeatedly disproven by modern-day scientists; the force is negligibly small compared to the many other influences on the rotation.
History
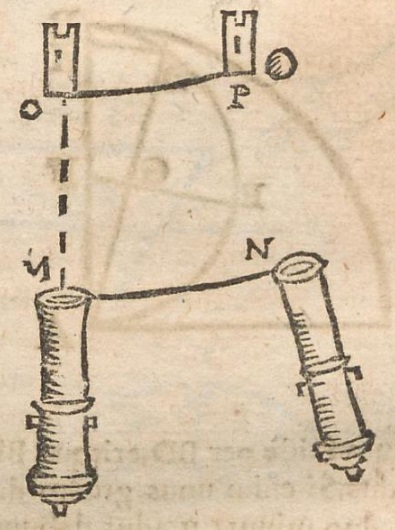
 Italian scientist
Italian scientist Giovanni Battista Riccioli
Giovanni Battista Riccioli, SJ (17 April 1598 – 25 June 1671) was an Italian astronomer and a Catholic priest in the Jesuit order. He is known, among other things, for his experiments with pendulums and with falling bodies, for his discussion ...
and his assistant Francesco Maria Grimaldi
Francesco Maria Grimaldi, SJ (2 April 1618 – 28 December 1663) was an Italian Jesuit priest, mathematician and physicist who taught at the Jesuit college in Bologna. He was born in Bologna to Paride Grimaldi and Anna Cattani.
Work
Betwee ...
described the effect in connection with artillery in the 1651 ''Almagestum Novum'', writing that rotation of the Earth should cause a cannonball fired to the north to deflect to the east. In 1674, Claude François Milliet Dechales
Claude François Milliet Dechales (1621 – 28 March 1678) was a French Jesuit priest and mathematician. He published a treatise on mathematics and a translation of the works of Euclid, though of lesser quality than that of Gilles Personne de Ro ...
described in his ''Cursus seu Mundus Mathematicus'' how the rotation of the Earth should cause a deflection in the trajectories of both falling bodies and projectiles aimed toward one of the planet's poles. Riccioli, Grimaldi, and Dechales all described the effect as part of an argument against the heliocentric system of Copernicus. In other words, they argued that the Earth's rotation should create the effect, and so failure to detect the effect was evidence for an immobile Earth. The Coriolis acceleration equation was derived by Euler in 1749, and the effect was described in the tidal equations of Pierre-Simon Laplace
Pierre-Simon, marquis de Laplace (; ; 23 March 1749 – 5 March 1827) was a French scholar and polymath whose work was important to the development of engineering, mathematics, statistics, physics, astronomy, and philosophy. He summarized ...
in 1778.
Gaspard-Gustave Coriolis
Gaspard-Gustave de Coriolis (; 21 May 1792 – 19 September 1843) was a French mathematician, mechanical engineer and scientist. He is best known for his work on the supplementary forces that are detected in a rotating frame of reference, le ...
published a paper in 1835 on the energy yield of machines with rotating parts, such as waterwheel
A water wheel is a machine for converting the energy of flowing or falling water into useful forms of power, often in a watermill. A water wheel consists of a wheel (usually constructed from wood or metal), with a number of blades or buc ...
s. That paper considered the supplementary forces that are detected in a rotating frame of reference. Coriolis divided these supplementary forces into two categories. The second category contained a force that arises from the cross product
In mathematics, the cross product or vector product (occasionally directed area product, to emphasize its geometric significance) is a binary operation on two vectors in a three-dimensional oriented Euclidean vector space (named here E), and i ...
of the angular velocity
In physics, angular velocity or rotational velocity ( or ), also known as angular frequency vector,(UP1) is a pseudovector representation of how fast the angular position or orientation of an object changes with time (i.e. how quickly an objec ...
of a coordinate system and the projection of a particle's velocity
Velocity is the directional speed of an object in motion as an indication of its rate of change in position as observed from a particular frame of reference and as measured by a particular standard of time (e.g. northbound). Velocity i ...
into a plane perpendicular
In elementary geometry, two geometric objects are perpendicular if they intersect at a right angle (90 degrees or π/2 radians). The condition of perpendicularity may be represented graphically using the ''perpendicular symbol'', ⟂. It can ...
to the system's axis of rotation. Coriolis referred to this force as the "compound centrifugal force" due to its analogies with the centrifugal force
In Newtonian mechanics, the centrifugal force is an inertial force (also called a "fictitious" or "pseudo" force) that appears to act on all objects when viewed in a rotating frame of reference. It is directed away from an axis which is parallel ...
already considered in category one. The effect was known in the early 20th century as the "acceleration
In mechanics, acceleration is the rate of change of the velocity of an object with respect to time. Accelerations are vector quantities (in that they have magnitude and direction). The orientation of an object's acceleration is given by ...
of Coriolis", and by 1920 as "Coriolis force".
In 1856, William Ferrel
William Ferrel (January 29, 1817 – September 18, 1891) was an American meteorologist who developed theories that explained the mid-latitude atmospheric circulation cell in detail, and it is after him that the Ferrel cell is named.
Biograph ...
proposed the existence of a circulation cell in the mid-latitudes with air being deflected by the Coriolis force to create the prevailing westerly winds.
The understanding of the kinematics of how exactly the rotation of the Earth affects airflow was partial at first. Late in the 19th century, the full extent of the large scale interaction of pressure-gradient force
In fluid mechanics, the pressure-gradient force is the force that results when there is a difference in pressure across a surface. In general, a pressure is a force per unit area, across a surface. A difference in pressure across a surface the ...
and deflecting force that in the end causes air masses to move along isobars was understood.
Formula
InNewtonian mechanics
Newton's laws of motion are three basic Scientific law, laws of classical mechanics that describe the relationship between the motion of an object and the forces acting on it. These laws can be paraphrased as follows:
# A body remains at re ...
, the equation of motion for an object in an inertial reference frame
In classical physics and special relativity, an inertial frame of reference (also called inertial reference frame, inertial frame, inertial space, or Galilean reference frame) is a frame of reference that is not undergoing any acceleratio ...
is
:
where is the vector sum of the physical forces acting on the object, is the mass of the object, and is the acceleration of the object relative to the inertial reference frame.
Transforming this equation to a reference frame rotating about a fixed axis through the origin with angular velocity
In physics, angular velocity or rotational velocity ( or ), also known as angular frequency vector,(UP1) is a pseudovector representation of how fast the angular position or orientation of an object changes with time (i.e. how quickly an objec ...
having variable rotation rate, the equation takes the form
:
where
* is the vector sum of the physical forces acting on the object
* is the angular velocity
In physics, angular velocity or rotational velocity ( or ), also known as angular frequency vector,(UP1) is a pseudovector representation of how fast the angular position or orientation of an object changes with time (i.e. how quickly an objec ...
, of the rotating reference frame relative to the inertial frame
* is the position vector of the object relative to the rotating reference frame
* is the velocity of the object relative to the rotating reference frame
* is the acceleration of the object relative to the rotating reference frame
The fictitious forces as they are perceived in the rotating frame act as additional forces that contribute to the apparent acceleration just like the real external forces.
The fictitious force terms of the equation are, reading from left to right:
* Euler force In classical mechanics, the Euler force is the fictitious tangential force
that appears when a non-uniformly rotating reference frame is used for analysis of motion and there is variation in the angular velocity of the reference frame's axes. The ...
,
* Coriolis force,
* centrifugal force
In Newtonian mechanics, the centrifugal force is an inertial force (also called a "fictitious" or "pseudo" force) that appears to act on all objects when viewed in a rotating frame of reference. It is directed away from an axis which is parallel ...
,
Notice the Euler and centrifugal forces depend on the position vector of the object, while the Coriolis force depends on the object's velocity as measured in the rotating reference frame. As expected, for a non-rotating inertial frame of reference
In classical physics and special relativity, an inertial frame of reference (also called inertial reference frame, inertial frame, inertial space, or Galilean reference frame) is a frame of reference that is not undergoing any acceleration. ...
the Coriolis force and all other fictitious forces disappear.
The forces also disappear for zero mass .
As the Coriolis force is proportional to a cross product
In mathematics, the cross product or vector product (occasionally directed area product, to emphasize its geometric significance) is a binary operation on two vectors in a three-dimensional oriented Euclidean vector space (named here E), and i ...
of two vectors, it is perpendicular to both vectors, in this case the object's velocity and the frame's rotation vector. It therefore follows that:
* if the velocity is parallel to the rotation axis, the Coriolis force is zero. For example, on Earth, this situation occurs for a body at the equator moving north or south relative to the Earth's surface.
* if the velocity is straight inward to the axis, the Coriolis force is in the direction of local rotation. For example, on Earth, this situation occurs for a body at the equator falling downward, as in the Dechales illustration above, where the falling ball travels further to the east than does the tower.
* if the velocity is straight outward from the axis, the Coriolis force is against the direction of local rotation. In the tower example, a ball launched upward would move toward the west.
* if the velocity is in the direction of rotation, the Coriolis force is outward from the axis. For example, on Earth, this situation occurs for a body at the equator moving east relative to Earth's surface. It would move upward as seen by an observer on the surface. This effect (see Eötvös effect below) was discussed by Galileo Galilei in 1632 and by Riccioli in 1651.
* if the velocity is against the direction of rotation, the Coriolis force is inward to the axis. For example, on Earth, this situation occurs for a body at the equator moving west, which would deflect downward as seen by an observer.
Length scales and the Rossby number
The time, space, and velocity scales are important in determining the importance of the Coriolis force. Whether rotation is important in a system can be determined by itsRossby number
The Rossby number (Ro), named for Carl-Gustav Arvid Rossby, is a dimensionless number used in describing fluid flow. The Rossby number is the ratio of inertial force to Coriolis force, terms , \mathbf \cdot \nabla \mathbf, \sim U^2 / L and \Omega ...
, which is the ratio of the velocity, ''U'', of a system to the product of the Coriolis parameter
The Coriolis frequency ''ƒ'', also called the Coriolis parameter or Coriolis coefficient, is equal to twice the rotation rate ''Ω'' of the Earth multiplied by the sine of the latitude \varphi.
:f = 2 \Omega \sin \varphi.\,
The rotation rate ...
, , and the length scale, ''L'', of the motion:
:
The Rossby number is the ratio of inertial to Coriolis forces. A small Rossby number indicates a system is strongly affected by Coriolis forces, and a large Rossby number indicates a system in which inertial forces dominate. For example, in tornadoes, the Rossby number is large, in low-pressure systems it is low, and in oceanic systems it is around 1. As a result, in tornadoes the Coriolis force is negligible, and balance is between pressure and centrifugal forces. In low-pressure systems, centrifugal force is negligible and balance is between Coriolis and pressure forces. In the oceans all three forces are comparable.
An atmospheric system moving at ''U'' = occupying a spatial distance of ''L'' = , has a Rossby number of approximately 0.1.
A baseball pitcher may throw the ball at ''U'' = for a distance of ''L'' = . The Rossby number in this case would be 32,000 ''(at latitude 31°47'46.382")''.
Baseball players don't care about which hemisphere they're playing in. However, an unguided missile obeys exactly the same physics as a baseball, but can travel far enough and be in the air long enough to experience the effect of Coriolis force. Long-range shells in the Northern Hemisphere landed close to, but to the right of, where they were aimed until this was noted. (Those fired in the Southern Hemisphere landed to the left.) In fact, it was this effect that first got the attention of Coriolis himself.
Simple cases
Tossed ball on a rotating carousel
Bounced ball
Applied to the Earth
The acceleration affecting the motion of air "sliding" over the Earth's surface is the horizontal component of the Coriolis term : This component is orthogonal to the velocity over the Earth surface and is given by the expression : where * is the spin rate of the Earth * is the latitude, positive in Northern Hemisphere and negative in the Southern Hemisphere In the Northern Hemisphere where the sign is positive this force/acceleration, as viewed from above, is to the right of the direction of motion, in the Southern Hemisphere where the sign is negative this force/acceleration is to the left of the direction of motionRotating sphere
Eötvös effect
The Eötvös effect is the change in measured Earth's gravity caused by the change in centrifugal acceleration resulting from eastbound or westbound velocity. When moving eastbound, the object's angular velocity is increased (in addition to Ear ...
, and an upward motion produces an acceleration due west.
Meteorology and oceanography


 Perhaps the most important impact of the Coriolis effect is in the large-scale dynamics of the oceans and the atmosphere. In meteorology and oceanography, it is convenient to postulate a rotating frame of reference wherein the Earth is stationary. In accommodation of that provisional postulation, the centrifugal and Coriolis forces are introduced. Their relative importance is determined by the applicable Rossby numbers.
Perhaps the most important impact of the Coriolis effect is in the large-scale dynamics of the oceans and the atmosphere. In meteorology and oceanography, it is convenient to postulate a rotating frame of reference wherein the Earth is stationary. In accommodation of that provisional postulation, the centrifugal and Coriolis forces are introduced. Their relative importance is determined by the applicable Rossby numbers. Tornado
A tornado is a violently rotating column of air that is in contact with both the surface of the Earth and a cumulonimbus cloud or, in rare cases, the base of a cumulus cloud. It is often referred to as a twister, whirlwind or cyclone, alt ...
es have high Rossby numbers, so, while tornado-associated centrifugal forces are quite substantial, Coriolis forces associated with tornadoes are for practical purposes negligible.
Because surface ocean currents are driven by the movement of wind over the water's surface, the Coriolis force also affects the movement of ocean currents and cyclones
In meteorology, a cyclone () is a large air mass that rotates around a strong center of low atmospheric pressure, counterclockwise in the Northern Hemisphere and clockwise in the Southern Hemisphere as viewed from above (opposite to an ant ...
as well. Many of the ocean's largest currents circulate around warm, high-pressure areas called gyre
In oceanography, a gyre () is any large system of circulating ocean currents, particularly those involved with large wind movements. Gyres are caused by the Coriolis effect; planetary vorticity, horizontal friction and vertical friction determin ...
s. Though the circulation is not as significant as that in the air, the deflection caused by the Coriolis effect is what creates the spiralling pattern in these gyres. The spiralling wind pattern helps the hurricane form. The stronger the force from the Coriolis effect, the faster the wind spins and picks up additional energy, increasing the strength of the hurricane.
Air within high-pressure systems rotates in a direction such that the Coriolis force is directed radially inwards, and nearly balanced by the outwardly radial pressure gradient. As a result, air travels clockwise around high pressure in the Northern Hemisphere and anticlockwise in the Southern Hemisphere. Air around low-pressure rotates in the opposite direction, so that the Coriolis force is directed radially outward and nearly balances an inwardly radial pressure gradient
In atmospheric science, the pressure gradient (typically of air but more generally of any fluid) is a physical quantity that describes in which direction and at what rate the pressure increases the most rapidly around a particular location. The ...
.
Flow around a low-pressure area
If a low-pressure area forms in the atmosphere, air tends to flow in towards it, but is deflected perpendicular to its velocity by the Coriolis force. A system of equilibrium can then establish itself creating circular movement, or a cyclonic flow. Because the Rossby number is low, the force balance is largely between thepressure-gradient force
In fluid mechanics, the pressure-gradient force is the force that results when there is a difference in pressure across a surface. In general, a pressure is a force per unit area, across a surface. A difference in pressure across a surface the ...
acting towards the low-pressure area and the Coriolis force acting away from the center of the low pressure.
Instead of flowing down the gradient, large scale motions in the atmosphere and ocean tend to occur perpendicular to the pressure gradient. This is known as geostrophic flow
A geostrophic current is an oceanic current in which the pressure gradient force is balanced by the Coriolis effect. The direction of geostrophic flow is parallel to the isobars, with the high pressure to the right of the flow in the Northern ...
. On a non-rotating planet, fluid would flow along the straightest possible line, quickly eliminating pressure gradients. The geostrophic balance is thus very different from the case of "inertial motions" (see below), which explains why mid-latitude cyclones are larger by an order of magnitude than inertial circle flow would be.
This pattern of deflection, and the direction of movement, is called Buys-Ballot's law. In the atmosphere, the pattern of flow is called a cyclone. In the Northern Hemisphere the direction of movement around a low-pressure area is anticlockwise. In the Southern Hemisphere, the direction of movement is clockwise because the rotational dynamics is a mirror image there. At high altitudes, outward-spreading air rotates in the opposite direction. Cyclones rarely form along the equator due to the weak Coriolis effect present in this region.
Inertial circles
An air or water mass moving with speed subject only to the Coriolis force travels in a circular trajectory called an ''inertial circle''. Since the force is directed at right angles to the motion of the particle, it moves with a constant speed around a circle whose radius is given by: : where is the Coriolis parameter , introduced above (where is the latitude). The time taken for the mass to complete a full circle is therefore . The Coriolis parameter typically has a mid-latitude value of about 10−4 s−1; hence for a typical atmospheric speed of , the radius is with a period of about 17 hours. For an ocean current with a typical speed of , the radius of an inertial circle is . These inertial circles are clockwise in the northern hemisphere (where trajectories are bent to the right) and anticlockwise in the southern hemisphere. If the rotating system is a parabolic turntable, then is constant and the trajectories are exact circles. On a rotating planet, varies with latitude and the paths of particles do not form exact circles. Since the parameter varies as the sine of the latitude, the radius of the oscillations associated with a given speed are smallest at the poles (latitude of ±90°), and increase toward the equator.Other terrestrial effects
The Coriolis effect strongly affects the large-scale oceanic andatmospheric circulation
Atmospheric circulation is the large-scale movement of air and together with ocean circulation is the means by which thermal energy is redistributed on the surface of the Earth. The Earth's atmospheric circulation varies from year to year, but ...
, leading to the formation of robust features like jet streams and western boundary current
Boundary currents are ocean currents with dynamics determined by the presence of a coastline, and fall into two distinct categories: western boundary currents and eastern boundary currents.
Eastern boundary currents
Eastern boundary currents are ...
s. Such features are in geostrophic balance, meaning that the Coriolis and ''pressure gradient'' forces balance each other. Coriolis acceleration is also responsible for the propagation of many types of waves in the ocean and atmosphere, including Rossby wave
Rossby waves, also known as planetary waves, are a type of inertial wave naturally occurring in rotating fluids. They were first identified by Sweden-born American meteorologist Carl-Gustaf Arvid Rossby. They are observed in the atmospheres and ...
s and Kelvin wave
A Kelvin wave is a wave in the ocean or atmosphere that balances the Earth's Coriolis force against a topographic boundary such as a coastline, or a waveguide such as the equator. A feature of a Kelvin wave is that it is non-dispersive, i.e., t ...
s. It is also instrumental in the so-called Ekman Ekman is a Swedish surname. Notable people with the surname include:
* Carl Gustaf Ekman (1872–1945), Swedish politician
*Carl Daniel Ekman (1845–1904), Swedish chemical engineer
* Erik Leonard Ekman (1883–1931), Swedish botanist
* Fam Ekman ...
dynamics in the ocean, and in the establishment of the large-scale ocean flow pattern called the Sverdrup balance.
Eötvös effect
The practical impact of the "Coriolis effect" is mostly caused by the horizontal acceleration component produced by horizontal motion. There are other components of the Coriolis effect. Westward-traveling objects are deflected downwards, while eastward-traveling objects are deflected upwards. This is known as theEötvös effect
The Eötvös effect is the change in measured Earth's gravity caused by the change in centrifugal acceleration resulting from eastbound or westbound velocity. When moving eastbound, the object's angular velocity is increased (in addition to Ear ...
. This aspect of the Coriolis effect is greatest near the equator. The force produced by the Eötvös effect is similar to the horizontal component, but the much larger vertical forces due to gravity and pressure suggest that it is unimportant in the hydrostatic equilibrium
In fluid mechanics, hydrostatic equilibrium (hydrostatic balance, hydrostasy) is the condition of a fluid or plastic solid at rest, which occurs when external forces, such as gravity, are balanced by a pressure-gradient force. In the planetar ...
. However, in the atmosphere, winds are associated with small deviations of pressure from the hydrostatic equilibrium. In the tropical atmosphere, the order of magnitude of the pressure deviations is so small that the contribution of the Eötvös effect to the pressure deviations is considerable.
In addition, objects traveling upwards (i.e. ''out'') or downwards (i.e. ''in'') are deflected to the west or east respectively. This effect is also the greatest near the equator. Since vertical movement is usually of limited extent and duration, the size of the effect is smaller and requires precise instruments to detect. For example, idealized numerical modeling studies suggest that this effect can directly affect tropical large-scale wind field by roughly 10% given long-duration (2 weeks or more) heating or cooling in the atmosphere. Moreover, in the case of large changes of momentum, such as a spacecraft being launched into orbit, the effect becomes significant. The fastest and most fuel-efficient path to orbit is a launch from the equator that curves to a directly eastward heading.
Intuitive example
Imagine a train that travels through afriction
Friction is the force resisting the relative motion of solid surfaces, fluid layers, and material elements sliding (motion), sliding against each other. There are several types of friction:
*Dry friction is a force that opposes the relative la ...
less railway line along the equator
The equator is a circle of latitude, about in circumference, that divides Earth into the Northern and Southern hemispheres. It is an imaginary line located at 0 degrees latitude, halfway between the North and South poles. The term can al ...
. Assume that, when in motion, it moves at the necessary speed to complete a trip around the world in one day (465 m/s). The Coriolis effect can be considered in three cases: when the train travels west, when it is at rest, and when it travels east. In each case, the Coriolis effect can be calculated from the rotating frame of reference
A rotating frame of reference is a special case of a non-inertial reference frame that is rotating relative to an inertial reference frame. An everyday example of a rotating reference frame is the surface of the Earth. (This article considers only ...
on Earth
Earth is the third planet from the Sun and the only astronomical object known to harbor life. While large volumes of water can be found throughout the Solar System, only Earth sustains liquid surface water. About 71% of Earth's surf ...
first, and then checked against a fixed inertial frame
In classical physics and special relativity, an inertial frame of reference (also called inertial reference frame, inertial frame, inertial space, or Galilean reference frame) is a frame of reference that is not undergoing any acceleration. ...
. The image below illustrates the three cases as viewed by an observer at rest in a (near) inertial frame from a fixed point above the North Pole
The North Pole, also known as the Geographic North Pole or Terrestrial North Pole, is the point in the Northern Hemisphere where the Earth's rotation, Earth's axis of rotation meets its surface. It is called the True North Pole to distingu ...
along the Earth's axis of rotation; the train is denoted by a few red pixels, fixed at the left side in the leftmost picture, moving in the others
 # The train travels toward the west: In that case, it moves against the direction of rotation. Therefore, on the Earth's rotating frame the Coriolis term is pointed inwards towards the axis of rotation (down). This additional force downwards should cause the train to be heavier while moving in that direction.If one looks at this train from the fixed non-rotating frame on top of the center of the Earth, at that speed it remains stationary as the Earth spins beneath it. Hence, the only force acting on it is
# The train travels toward the west: In that case, it moves against the direction of rotation. Therefore, on the Earth's rotating frame the Coriolis term is pointed inwards towards the axis of rotation (down). This additional force downwards should cause the train to be heavier while moving in that direction.If one looks at this train from the fixed non-rotating frame on top of the center of the Earth, at that speed it remains stationary as the Earth spins beneath it. Hence, the only force acting on it is gravity
In physics, gravity () is a fundamental interaction which causes mutual attraction between all things with mass or energy. Gravity is, by far, the weakest of the four fundamental interactions, approximately 1038 times weaker than the str ...
and the reaction from the track. This force is greater (by 0.34%) than the force that the passengers and the train experience when at rest (rotating along with Earth). This difference is what the Coriolis effect accounts for in the rotating frame of reference.
# The train comes to a stop: From the point of view on the Earth's rotating frame, the velocity of the train is zero, thus the Coriolis force is also zero and the train and its passengers recuperate their usual weight.From the fixed inertial frame of reference above Earth, the train now rotates along with the rest of the Earth. 0.34% of the force of gravity provides the centripetal force
A centripetal force (from Latin ''centrum'', "center" and ''petere'', "to seek") is a force that makes a body follow a curved path. Its direction is always orthogonal to the motion of the body and towards the fixed point of the instantaneous c ...
needed to achieve the circular motion on that frame of reference. The remaining force, as measured by a scale, makes the train and passengers "lighter" than in the previous case.
# The train travels east. In this case, because it moves in the direction of Earth's rotating frame, the Coriolis term is directed outward from the axis of rotation (up). This upward force makes the train seem lighter still than when at rest. From the fixed inertial frame of reference above Earth, the train traveling east now rotates at twice the rate as when it was at rest—so the amount of centripetal force needed to cause that circular path increases leaving less force from gravity to act on the track. This is what the Coriolis term accounts for on the previous paragraph.As a final check one can imagine a frame of reference rotating along with the train. Such frame would be rotating at twice the angular velocity as Earth's rotating frame. The resulting
From the fixed inertial frame of reference above Earth, the train traveling east now rotates at twice the rate as when it was at rest—so the amount of centripetal force needed to cause that circular path increases leaving less force from gravity to act on the track. This is what the Coriolis term accounts for on the previous paragraph.As a final check one can imagine a frame of reference rotating along with the train. Such frame would be rotating at twice the angular velocity as Earth's rotating frame. The resulting centrifugal force
In Newtonian mechanics, the centrifugal force is an inertial force (also called a "fictitious" or "pseudo" force) that appears to act on all objects when viewed in a rotating frame of reference. It is directed away from an axis which is parallel ...
component for that imaginary frame would be greater. Since the train and its passengers are at rest, that would be the only component in that frame explaining again why the train and the passengers are lighter than in the previous two cases.
This also explains why high-speed projectiles that travel west are deflected down, and those that travel east are deflected up. This vertical component of the Coriolis effect is called the Eötvös effect
The Eötvös effect is the change in measured Earth's gravity caused by the change in centrifugal acceleration resulting from eastbound or westbound velocity. When moving eastbound, the object's angular velocity is increased (in addition to Ear ...
.
The above example can be used to explain why the Eötvös effect starts diminishing when an object is traveling westward as its tangential speed increases above Earth's rotation (465 m/s). If the westward train in the above example increases speed, part of the force of gravity that pushes against the track accounts for the centripetal force needed to keep it in circular motion on the inertial frame. Once the train doubles its westward speed at that centripetal force becomes equal to the force the train experiences when it stops. From the inertial frame, in both cases it rotates at the same speed but in the opposite directions. Thus, the force is the same cancelling completely the Eötvös effect. Any object that moves westward at a speed above experiences an upward force instead. In the figure, the Eötvös effect is illustrated for a object on the train at different speeds. The parabolic shape is because the centripetal force
A centripetal force (from Latin ''centrum'', "center" and ''petere'', "to seek") is a force that makes a body follow a curved path. Its direction is always orthogonal to the motion of the body and towards the fixed point of the instantaneous c ...
is proportional to the square of the tangential speed. On the inertial frame, the bottom of the parabola is centered at the origin. The offset is because this argument uses the Earth's rotating frame of reference. The graph shows that the Eötvös effect is not symmetrical, and that the resulting downward force experienced by an object that travels west at high velocity is less than the resulting upward force when it travels east at the same speed.
Draining in bathtubs and toilets
Contrary to popular misconception, bathtubs, toilets, and other water receptacles do not drain in opposite directions in the Northern and Southern Hemispheres. This is because the magnitude of the Coriolis force is negligible at this scale. Forces determined by the initial conditions of the water (e.g. the geometry of the drain, the geometry of the receptacle, preexisting momentum of the water, etc.) are likely to be orders of magnitude greater than the Coriolis force and hence will determine the direction of water rotation, if any. For example, identical toilets flushed in both hemispheres drain in the same direction, and this direction is determined mostly by the shape of the toilet bowl. Under real-world conditions, the Coriolis force does not influence the direction of water flow perceptibly. Only if the water is so still that the effective rotation rate of the Earth is faster than that of the water relative to its container, and if externally applied torques (such as might be caused by flow over an uneven bottom surface) are small enough, the Coriolis effect may indeed determine the direction of the vortex. Without such careful preparation, the Coriolis effect will be much smaller than various other influences on drain direction such as any residual rotation of the water and the geometry of the container.Laboratory testing of draining water under atypical conditions
In 1962, Ascher Shapiro performed an experiment atMIT
The Massachusetts Institute of Technology (MIT) is a private land-grant research university in Cambridge, Massachusetts. Established in 1861, MIT has played a key role in the development of modern technology and science, and is one of the ...
to test the Coriolis force on a large basin of water, across, with a small wooden cross above the plug hole to display the direction of rotation, covering it and waiting for at least 24 hours for the water to settle. Under these precise laboratory conditions, he demonstrated the effect and consistent counterclockwise rotation.
He reported that,
Lloyd Trefethen reported clockwise rotation in the Southern Hemisphere at the University of Sydney in five tests with settling times of 18 h or more.
Ballistic trajectories
The Coriolis force is important inexternal ballistics
External ballistics or exterior ballistics is the part of ballistics that deals with the behavior of a projectile in flight. The projectile may be powered or un-powered, guided or unguided, spin or fin stabilized, flying through an atmosphere or ...
for calculating the trajectories of very long-range artillery
Artillery is a class of heavy military ranged weapons that launch munitions far beyond the range and power of infantry firearms. Early artillery development focused on the ability to breach defensive walls and fortifications during sieg ...
shells. The most famous historical example was the Paris gun
The Paris Gun (german: Paris-Geschütz / Pariser Kanone) was the name given to a type of German long-range siege gun, several of which were used to bombard Paris during World War I. They were in service from March to August 1918. When the guns w ...
, used by the Germans during World War I
World War I (28 July 1914 11 November 1918), often abbreviated as WWI, was List of wars and anthropogenic disasters by death toll, one of the deadliest global conflicts in history. Belligerents included much of Europe, the Russian Empire, ...
to bombard Paris
Paris () is the capital and most populous city of France, with an estimated population of 2,165,423 residents in 2019 in an area of more than 105 km² (41 sq mi), making it the 30th most densely populated city in the world in 2020. ...
from a range of about . The Coriolis force minutely changes the trajectory of a bullet, affecting accuracy at extremely long distances. It is adjusted for by accurate long-distance shooters, such as snipers. At the latitude of Sacramento
)
, image_map = Sacramento County California Incorporated and Unincorporated areas Sacramento Highlighted.svg
, mapsize = 250x200px
, map_caption = Location within Sacramento ...
, California, a northward shot would be deflected to the right. There is also a vertical component, explained in the Eötvös effect section above, which causes westward shots to hit low, and eastward shots to hit high.The claim is made that in the Falklands in WW I, the British failed to correct their sights for the southern hemisphere, and so missed their targets. For set up of the calculations, see Carlucci & Jacobson (2007), p. 225
The effects of the Coriolis force on ballistic trajectories should not be confused with the curvature of the paths of missiles, satellites, and similar objects when the paths are plotted on two-dimensional (flat) maps, such as the Mercator projection
The Mercator projection () is a cylindrical map projection presented by Flemish geographer and cartographer Gerardus Mercator in 1569. It became the standard map projection for navigation because it is unique in representing north as up and so ...
. The projections of the three-dimensional curved surface of the Earth to a two-dimensional surface (the map) necessarily results in distorted features. The apparent curvature of the path is a consequence of the sphericity of the Earth and would occur even in a non-rotating frame.
 The Coriolis force on a moving
The Coriolis force on a moving projectile
A projectile is an object that is propelled by the application of an external force and then moves freely under the influence of gravity and air resistance. Although any objects in motion through space are projectiles, they are commonly found i ...
depends on velocity components in all three directions, latitude
In geography, latitude is a coordinate that specifies the north– south position of a point on the surface of the Earth or another celestial body. Latitude is given as an angle that ranges from –90° at the south pole to 90° at the north po ...
, and azimuth
An azimuth (; from ar, اَلسُّمُوت, as-sumūt, the directions) is an angular measurement in a spherical coordinate system. More specifically, it is the horizontal angle from a cardinal direction, most commonly north.
Mathematicall ...
. The directions are typically downrange (the direction that the gun is initially pointing), vertical, and cross-range.
:
:
:
where
*, down-range acceleration.
*, vertical acceleration with positive indicating acceleration upward.
*, cross-range acceleration with positive indicating acceleration to the right.
*, down-range velocity.
*, vertical velocity with positive indicating upward.
*, cross-range velocity with positive indicating velocity to the right.
* = 0.00007292 rad/sec, angular velocity of the earth (based on a sidereal day
Sidereal time (as a unit also sidereal day or sidereal rotation period) (sidereal ) is a timekeeping system that astronomers use to locate celestial objects. Using sidereal time, it is possible to easily point a telescope to the proper coor ...
).
*, latitude with positive indicating Northern hemisphere.
*, azimuth measured clockwise from due North.
Visualization of the Coriolis effect

 To demonstrate the Coriolis effect, a parabolic turntable can be used.
On a flat turntable, the inertia of a co-rotating object forces it off the edge. However, if the turntable surface has the correct
To demonstrate the Coriolis effect, a parabolic turntable can be used.
On a flat turntable, the inertia of a co-rotating object forces it off the edge. However, if the turntable surface has the correct paraboloid
In geometry, a paraboloid is a quadric surface that has exactly one axis of symmetry and no center of symmetry. The term "paraboloid" is derived from parabola, which refers to a conic section that has a similar property of symmetry.
Every pla ...
(parabolic bowl) shape (see the figure) and rotates at the corresponding rate, the force components shown in the figure make the component of gravity tangential to the bowl surface exactly equal to the centripetal force necessary to keep the object rotating at its velocity and radius of curvature (assuming no friction). (See banked turn
A banked turn (or banking turn) is a turn or change of direction in which the vehicle banks or inclines, usually towards the inside of the turn. For a road or railroad this is usually due to the roadbed having a transverse down-slope towards the ...
.) This carefully contoured surface allows the Coriolis force to be displayed in isolation.When a container of fluid is rotating on a turntable, the surface of the fluid naturally assumes the correct parabolic shape. This fact may be exploited to make a parabolic turntable by using a fluid that sets after several hours, such as a synthetic resin
In polymer chemistry and materials science, resin is a solid or highly viscous substance of plant or synthetic origin that is typically convertible into polymers. Resins are usually mixtures of organic compounds. This article focuses on n ...
. For a video of the Coriolis effect on such a parabolic surface, seGeophysical fluid dynamics lab demonstration
John Marshall, Massachusetts Institute of Technology.For a java applet of the Coriolis effect on such a parabolic surface, se
School of Meteorology at the University of Oklahoma. Discs cut from cylinders of
dry ice
Dry ice is the solid form of carbon dioxide. It is commonly used for temporary refrigeration as CO2 does not have a liquid state at normal atmospheric pressure and sublimates directly from the solid state to the gas state. It is used primarily ...
can be used as pucks, moving around almost frictionlessly over the surface of the parabolic turntable, allowing effects of Coriolis on dynamic phenomena to show themselves. To get a view of the motions as seen from the reference frame rotating with the turntable, a video camera is attached to the turntable so as to co-rotate with the turntable, with results as shown in the figure. In the left panel of the figure, which is the viewpoint of a stationary observer, the gravitational force in the inertial frame pulling the object toward the center (bottom ) of the dish is proportional to the distance of the object from the center. A centripetal force of this form causes the elliptical motion. In the right panel, which shows the viewpoint of the rotating frame, the inward gravitational force in the rotating frame (the same force as in the inertial frame) is balanced by the outward centrifugal force (present only in the rotating frame). With these two forces balanced, in the rotating frame the only unbalanced force is Coriolis (also present only in the rotating frame), and the motion is an ''inertial circle
In physics, the Coriolis force is an inertial or fictitious force that acts on objects in motion within a frame of reference that rotates with respect to an inertial frame. In a reference frame with clockwise rotation, the force acts to the ...
''. Analysis and observation of circular motion in the rotating frame is a simplification compared with analysis and observation of elliptical motion in the inertial frame.
Because this reference frame rotates several times a minute rather than only once a day like the Earth, the Coriolis acceleration produced is many times larger and so easier to observe on small time and spatial scales than is the Coriolis acceleration caused by the rotation of the Earth.
In a manner of speaking, the Earth is analogous to such a turntable. The rotation has caused the planet to settle on a spheroid shape, such that the normal force, the gravitational force and the centrifugal force exactly balance each other on a "horizontal" surface. (See equatorial bulge
An equatorial bulge is a difference between the equatorial and polar diameters of a planet, due to the centrifugal force exerted by the rotation about the body's axis. A rotating body tends to form an oblate spheroid rather than a sphere.
On Ear ...
.)
The Coriolis effect caused by the rotation of the Earth can be seen indirectly through the motion of a Foucault pendulum
The Foucault pendulum or Foucault's pendulum is a simple device named after French physicist Léon Foucault, conceived as an experiment to demonstrate the Earth's rotation. A long and heavy pendulum suspended from the high roof above a circular a ...
.
Coriolis effects in other areas
Coriolis flow meter
A practical application of the Coriolis effect is the mass flow meter, an instrument that measures themass flow rate
In physics and engineering, mass flow rate is the mass of a substance which passes per unit of time. Its unit is kilogram per second in SI units, and slug per second or pound per second in US customary units. The common symbol is \dot (''� ...
and density
Density (volumetric mass density or specific mass) is the substance's mass per unit of volume. The symbol most often used for density is ''ρ'' (the lower case Greek letter rho), although the Latin letter ''D'' can also be used. Mathematicall ...
of a fluid flowing through a tube. The operating principle involves inducing a vibration of the tube through which the fluid passes. The vibration, though not completely circular, provides the rotating reference frame that gives rise to the Coriolis effect. While specific methods vary according to the design of the flow meter, sensors monitor and analyze changes in frequency, phase shift, and amplitude of the vibrating flow tubes. The changes observed represent the mass flow rate and density of the fluid.
Molecular physics
In polyatomic molecules, the molecule motion can be described by a rigid body rotation and internal vibration of atoms about their equilibrium position. As a result of the vibrations of the atoms, the atoms are in motion relative to the rotating coordinate system of the molecule. Coriolis effects are therefore present, and make the atoms move in a direction perpendicular to the original oscillations. This leads to a mixing in molecular spectra between the rotational and vibrational levels, from which Coriolis coupling constants can be determined.Gyroscopic precession
When an external torque is applied to a spinning gyroscope along an axis that is at right angles to the spin axis, the rim velocity that is associated with the spin becomes radially directed in relation to the external torque axis. This causes a ''torque-induced'' force to act on the rim in such a way as to tilt the gyroscope at right angles to the direction that the external torque would have tilted it. This tendency has the effect of keeping spinning bodies in their rotational frame.Insect flight
Flies ( Diptera) and some moths ( Lepidoptera) exploit the Coriolis effect in flight with specialized appendages and organs that relay information about theangular velocity
In physics, angular velocity or rotational velocity ( or ), also known as angular frequency vector,(UP1) is a pseudovector representation of how fast the angular position or orientation of an object changes with time (i.e. how quickly an objec ...
of their bodies.
Coriolis forces resulting from linear motion of these appendages are detected within the rotating frame of reference of the insects' bodies. In the case of flies, their specialized appendages are dumbbell shaped organs located just behind their wings called "halteres
''Halteres'' (; singular ''halter'' or ''haltere'') (from grc, ἁλτῆρες, weights held in the hands to give an impetus in leaping) are a pair of small club-shaped organs on the body of two orders of flying insects that provide infor ...
".
The fly's halteres oscillate in a plane at the same beat frequency as the main wings so that any body rotation results in lateral deviation of the halteres from their plane of motion.
In moths, their antennae are known to be responsible for the ''sensing'' of Coriolis forces in the similar manner as with the halteres in flies. In both flies and moths, a collection of mechanosensors at the base of the appendage are sensitive to deviations at the beat frequency, correlating to rotation in the pitch and roll planes, and at twice the beat frequency, correlating to rotation in the yaw plane.
Lagrangian point stability
In astronomy,Lagrangian point
In celestial mechanics, the Lagrange points (; also Lagrangian points or libration points) are points of equilibrium for small-mass objects under the influence of two massive orbiting bodies. Mathematically, this involves the solution of ...
s are five positions in the orbital plane of two large orbiting bodies where a small object affected only by gravity can maintain a stable position relative to the two large bodies. The first three Lagrangian points (L1, L2, L3) lie along the line connecting the two large bodies, while the last two points (L4 and L5) each form an equilateral triangle with the two large bodies. The L4 and L5 points, although they correspond to maxima of the effective potential
The effective potential (also known as effective potential energy) combines multiple, perhaps opposing, effects into a single potential. In its basic form, it is the sum of the 'opposing' centrifugal potential energy with the potential energy of a ...
in the coordinate frame that rotates with the two large bodies, are stable due to the Coriolis effect. The stability can result in orbits around just L4 or L5, known as tadpole orbit
In celestial mechanics, a horseshoe orbit is a type of co-orbital motion of a small orbiting body relative to a larger orbiting body. The osculating (instantaneous) orbital period of the smaller body remains very near that of the larger body, a ...
s, where trojans
Trojan or Trojans may refer to:
* Of or from the ancient city of Troy
* Trojan language, the language of the historical Trojans
Arts and entertainment Music
* ''Les Troyens'' ('The Trojans'), an opera by Berlioz, premiered part 1863, part 189 ...
can be found. It can also result in orbits that encircle L3, L4, and L5, known as horseshoe orbit
In celestial mechanics, a horseshoe orbit is a type of co-orbital motion of a small orbiting body relative to a larger orbiting body. The osculating (instantaneous) orbital period of the smaller body remains very near that of the larger body, a ...
s.
See also
*Analytical mechanics
In theoretical physics and mathematical physics, analytical mechanics, or theoretical mechanics is a collection of closely related alternative formulations of classical mechanics. It was developed by many scientists and mathematicians during the ...
* Applied mechanics
Applied mechanics is the branch of science concerned with the motion of any substance that can be experienced or perceived by humans without the help of instruments. In short, when mechanics concepts surpass being theoretical and are applied and e ...
* Classical mechanics
Classical mechanics is a physical theory describing the motion of macroscopic objects, from projectiles to parts of machinery, and astronomical objects, such as spacecraft, planets, stars, and galaxies. For objects governed by classical ...
* Dynamics (mechanics)
Dynamics is the branch of classical mechanics that is concerned with the study of forces and their effects on motion. Isaac Newton was the first to formulate the fundamental physical laws that govern dynamics in classical non-relativistic phys ...
* Earth's rotation
* Equatorial Rossby wave
* Frenet–Serret formulas
In differential geometry, the Frenet–Serret formulas describe the kinematic properties of a particle moving along a differentiable curve in three-dimensional Euclidean space \mathbb^, or the geometric properties of the curve itself irrespective ...
* Gyroscope
* Kinetics (physics)
In physics and engineering, kinetics is the branch of classical mechanics that is concerned with the relationship between the motion and its causes, specifically, forces and torques. Since the mid-20th century, the term " dynamics" (or "analytic ...
* Mechanics of planar particle motion
This article describes a particle in planar motionSee for example, , when observed from non-inertial reference frames.''Fictitious forces'' (also known as a ''pseudo forces'', ''inertial forces'' or ''d'Alembert forces''), exist for observers i ...
* Reactive centrifugal force
In classical mechanics, a reactive centrifugal force forms part of an action–reaction pair with a centripetal force.
In accordance with Newton's first law of motion, an object moves in a straight line in the absence of a net force acting on t ...
* Secondary flow
In fluid dynamics, flow can be decomposed into primary plus secondary flow, a relatively weaker flow pattern superimposed on the stronger primary flow pattern. The primary flow is often chosen to be an exact solution to simplified or approximated ...
* Statics
Statics is the branch of classical mechanics that is concerned with the analysis of force and torque (also called moment) acting on physical systems that do not experience an acceleration (''a''=0), but rather, are in static equilibrium with t ...
* Uniform circular motion
In physics, circular motion is a movement of an object along the circumference of a circle or rotation along a circular path. It can be uniform, with constant angular rate of rotation and constant speed, or non-uniform with a changing rate of rot ...
* Whirlpool
A whirlpool is a body of rotating water produced by opposing currents or a current running into an obstacle. Small whirlpools form when a bath or a sink is draining. More powerful ones formed in seas or oceans may be called maelstroms ( ). ''Vo ...
References
Further reading
Physics and meteorology
* Riccioli, G. B., 1651: ''Almagestum Novum'', Bologna, pp. 425–427Original book
n Latin
N, or n, is the fourteenth letter in the Latin alphabet, used in the modern English alphabet, the alphabets of other western European languages and others worldwide. Its name in English is ''en'' (pronounced ), plural ''ens''.
History
...
scanned images of complete pages.)
* Coriolis, G. G., 1832: "Mémoire sur le principe des forces vives dans les mouvements relatifs des machines." ''Journal de l'école Polytechnique'', Vol 13, pp. 268–302.Original article
n French
N, or n, is the fourteenth letter in the Latin alphabet, used in the modern English alphabet, the alphabets of other western European languages and others worldwide. Its name in English is ''en'' (pronounced ), plural ''ens''.
History
...
PDF file, 1.6 MB, scanned images of complete pages.)
* Coriolis, G. G., 1835: "Mémoire sur les équations du mouvement relatif des systèmes de corps." ''Journal de l'école Polytechnique'', Vol 15, pp. 142–154 Original article
n French
N, or n, is the fourteenth letter in the Latin alphabet, used in the modern English alphabet, the alphabets of other western European languages and others worldwide. Its name in English is ''en'' (pronounced ), plural ''ens''.
History
...
PDF file, 400 KB, scanned images of complete pages.)
* Gill, A. E. ''Atmosphere-Ocean dynamics'', Academic Press, 1982.
*
Durran, D. R.
1993:
Is the Coriolis force really responsible for the inertial oscillation?
', Bull. Amer. Meteor. Soc., 74, pp. 2179–2184; Corrigenda. Bulletin of the American Meteorological Society, 75, p. 261 * Durran, D. R., and S. K. Domonkos, 1996:
An apparatus for demonstrating the inertial oscillation
', Bulletin of the American Meteorological Society, 77, pp. 557–559. * Marion, Jerry B. 1970, ''Classical Dynamics of Particles and Systems'', Academic Press. * Persson, A., 1998
How do we Understand the Coriolis Force?'' Bulletin of the American Meteorological Society 79, pp. 1373–1385. * Symon, Keith. 1971, ''Mechanics'', Addison–Wesley
Akira Kageyama & Mamoru Hyodo: ''Eulerian derivation of the Coriolis force''
James F. Price: ''A Coriolis tutorial''
Woods Hole Oceanographic Institute (2003) * . Elementary, non-mathematical; but well written.
Historical
* Grattan-Guinness, I., Ed., 1994: ''Companion Encyclopedia of the History and Philosophy of the Mathematical Sciences''. Vols. I and II. Routledge, 1840 pp.1997: ''The Fontana History of the Mathematical Sciences''. Fontana, 817 pp. 710 pp. * Khrgian, A., 1970: ''Meteorology: A Historical Survey''. Vol. 1. Keter Press, 387 pp. * Kuhn, T. S., 1977: Energy conservation as an example of simultaneous discovery. ''The Essential Tension, Selected Studies in Scientific Tradition and Change'', University of Chicago Press, 66–104. * Kutzbach, G., 1979: ''The Thermal Theory of Cyclones. A History of Meteorological Thought in the Nineteenth Century''. Amer. Meteor. Soc., 254 pp.
External links
The definition of the Coriolis effect from the Glossary of Meteorology
The Coriolis Effect — a conflict between common sense and mathematics
PDF-file. 20 pages. A general discussion by Anders Persson of various aspects of the coriolis effect, including Foucault's Pendulum and Taylor columns.
The coriolis effect in meteorology
PDF-file. 5 pages. A detailed explanation by Mats Rosengren of how the gravitational force and the rotation of the Earth affect the atmospheric motion over the Earth surface. 2 figures
from the About.com Weather Page
– from ScienceWorld
''Coriolis Effect and Drains''
An article from the NEWTON web site hosted by the
Argonne National Laboratory
Argonne National Laboratory is a science and engineering research national laboratory operated by UChicago Argonne LLC for the United States Department of Energy. The facility is located in Lemont, Illinois, outside of Chicago, and is the lar ...
.
Catalog of Coriolis videos
''Coriolis Effect: A graphical animation''
a visual Earth animation with precise explanation
''An introduction to fluid dynamics''
SPINLab Educational Film explains the Coriolis effect with the aid of lab experiments
by Cecil Adams.
An article uncovering misinformation about the Coriolis effect. By Alistair B. Fraser, Emeritus Professor of Meteorology at
Pennsylvania State University
The Pennsylvania State University (Penn State or PSU) is a public state-related land-grant research university with campuses and facilities throughout Pennsylvania. Founded in 1855 as the Farmers' High School of Pennsylvania, Penn State becam ...
''The Coriolis Effect: A (Fairly) Simple Explanation''
an explanation for the layperson
Observe an animation of the Coriolis effect over Earth's surface
Animation clip
showing scenes as viewed from both an inertial frame and a rotating frame of reference, visualizing the Coriolis and centrifugal forces.
lets you control rotation speed, droplet speed and frame of reference to explore the Coriolis effect.
Rotating Co-ordinating Systems
transformation from inertial systems {{DEFAULTSORT:Coriolis Force Classical mechanics Force Atmospheric dynamics Physical phenomena Fictitious forces Rotation