Cantic Order-6 Cubic Honeycomb on:
[Wikipedia]
[Google]
[Amazon]
The order-6 cubic honeycomb is a paracompact
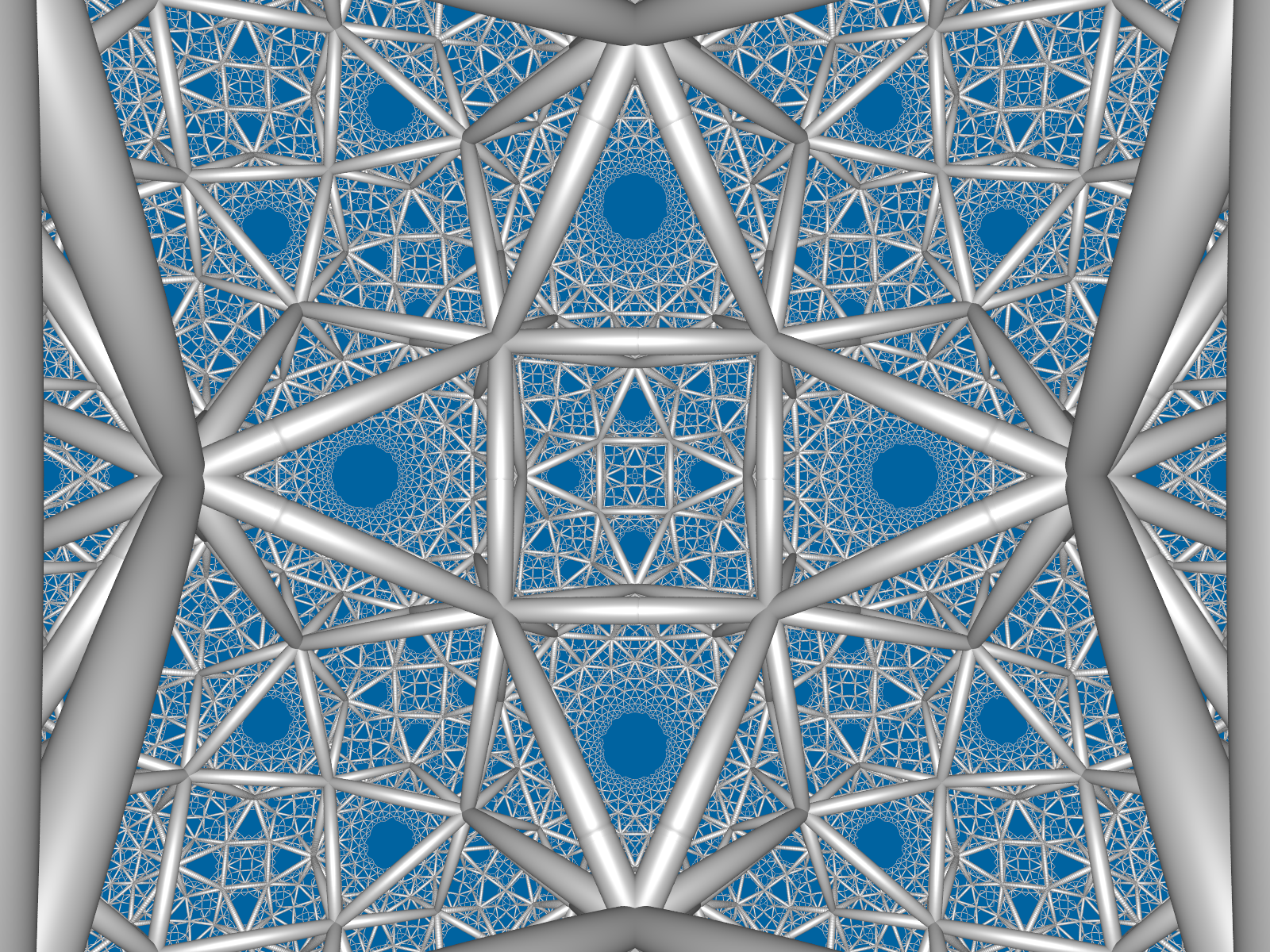It is similar to the 2D hyperbolic 
It is similar to the 2D hyperbolic 
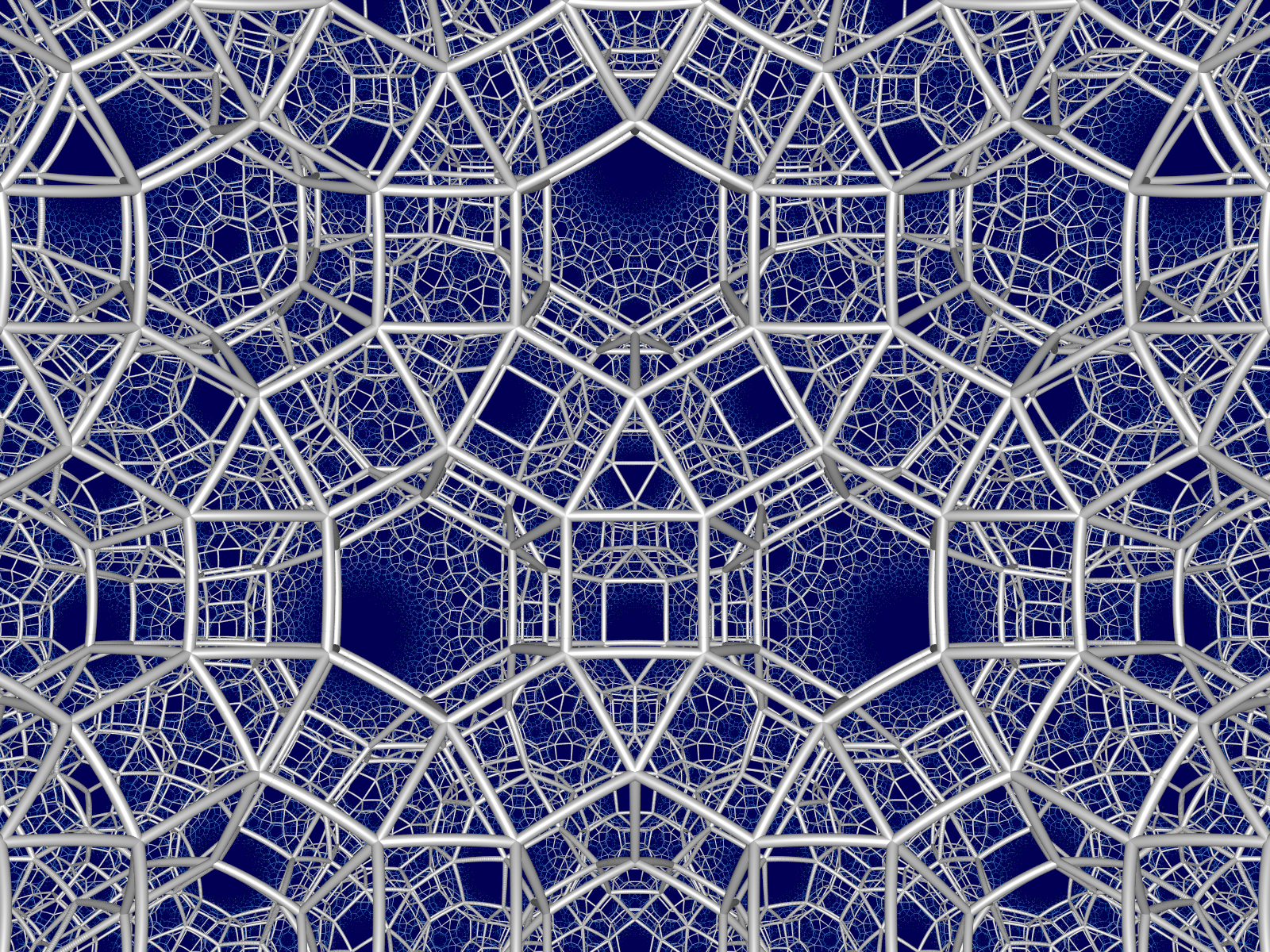
Regular Honeycombs in Hyperbolic Space
Table III * Jeffrey R. Weeks ''The Shape of Space, 2nd edition'' {{isbn, 0-8247-0709-5 (Chapter 16-17: Geometries on Three-manifolds I,II) * Norman Johnson ''Uniform Polytopes'', Manuscript ** N.W. Johnson: ''The Theory of Uniform Polytopes and Honeycombs'', Ph.D. Dissertation, University of Toronto, 1966 ** N.W. Johnson: ''Geometries and Transformations'', (2018) Chapter 13: Hyperbolic Coxeter groups Honeycombs (geometry)
regular
The term regular can mean normal or in accordance with rules. It may refer to:
People
* Moses Regular (born 1971), America football player
Arts, entertainment, and media Music
* "Regular" (Badfinger song)
* Regular tunings of stringed instrum ...
space-filling tessellation (or honeycomb) in hyperbolic 3-space. It is ''paracompact'' because it has vertex figures composed of an infinite number of facets, with all vertices as ideal points at infinity. With Schläfli symbol
In geometry, the Schläfli symbol is a notation of the form \ that defines regular polytopes and tessellations.
The Schläfli symbol is named after the 19th-century Swiss mathematician Ludwig Schläfli, who generalized Euclidean geometry to more ...
, the honeycomb has six ideal cubes meeting along each edge. Its vertex figure is an infinite triangular tiling. Its dual
Dual or Duals may refer to:
Paired/two things
* Dual (mathematics), a notion of paired concepts that mirror one another
** Dual (category theory), a formalization of mathematical duality
*** see more cases in :Duality theories
* Dual (grammatical ...
is the order-4 hexagonal tiling honeycomb
In the field of hyperbolic geometry, the order-4 hexagonal tiling honeycomb arises as one of 11 regular paracompact honeycombs in 3-dimensional hyperbolic space. It is ''paracompact'' because it has cells composed of an infinite number of faces. ...
.
Images
Symmetry
A half-symmetry construction of the order-6 cubic honeycomb exists as , with two alternating types (colors) of cubic cells. This construction has Coxeter-Dynkin diagram ↔ . Another lower-symmetry construction, ,3*,6 of index 6, exists with a non-simplex fundamental domain, with Coxeter-Dynkin diagram . This honeycomb contains that tile 2- hypercycle surfaces, similar to the paracompact order-3 apeirogonal tiling, : :
Related polytopes and honeycombs
The order-6 cubic honeycomb is a regular hyperbolic honeycomb in 3-space, and one of 11 which are paracompact. It has a related alternation honeycomb, represented by ↔ . This alternated form hashexagonal tiling
In geometry, the hexagonal tiling or hexagonal tessellation is a regular tiling of the Euclidean plane, in which exactly three hexagons meet at each vertex. It has Schläfli symbol of or (as a truncated triangular tiling).
English mathemat ...
and tetrahedron cells.
There are fifteen uniform honeycombs in the ,3,4 Coxeter group family, including the order-6 cubic honeycomb itself.
The order-6 cubic honeycomb is part of a sequence of regular polychora and honeycombs with cubic
Cubic may refer to:
Science and mathematics
* Cube (algebra), "cubic" measurement
* Cube, a three-dimensional solid object bounded by six square faces, facets or sides, with three meeting at each vertex
** Cubic crystal system, a crystal system w ...
cells
Cell most often refers to:
* Cell (biology), the functional basic unit of life
Cell may also refer to:
Locations
* Monastic cell, a small room, hut, or cave in which a religious recluse lives, alternatively the small precursor of a monastery w ...
.
It is also part of a sequence of honeycombs with triangular tiling vertex figures.
Rectified order-6 cubic honeycomb
The rectified order-6 cubic honeycomb, r, has cuboctahedral and triangular tiling facets, with a hexagonal prism vertex figure.
It is similar to the 2D hyperbolic tetraapeirogonal tiling
In geometry, the tetraapeirogonal tiling is a uniform tilings in hyperbolic plane, uniform tiling of the hyperbolic geometry, hyperbolic plane with a Schläfli symbol of r.
Uniform constructions
There are 3 lower symmetry uniform construction, one ...
, r, alternating apeirogonal and square faces:
:
Truncated order-6 cubic honeycomb
The truncated order-6 cubic honeycomb, t, has truncated cube and triangular tiling facets, with a hexagonal pyramid vertex figure.
It is similar to the 2D hyperbolic truncated infinite-order square tiling
In geometry, the truncated infinite-order square tiling is a uniform tiling of the hyperbolic plane. It has Schläfli symbol of t.
Uniform color
In (*∞44) symmetry this tiling has 3 colors. Bisecting the isosceles triangle domains can double th ...
, t, with apeirogonal and octagonal (truncated square) faces:
:
Bitruncated order-6 cubic honeycomb
The bitruncated order-6 cubic honeycomb is the same as the bitruncated order-4 hexagonal tiling honeycomb.Cantellated order-6 cubic honeycomb
The cantellated order-6 cubic honeycomb, rr, has rhombicuboctahedron, trihexagonal tiling, and hexagonal prism facets, with a wedge vertex figure.
Cantitruncated order-6 cubic honeycomb
The cantitruncated order-6 cubic honeycomb, tr, has truncated cuboctahedron,hexagonal tiling
In geometry, the hexagonal tiling or hexagonal tessellation is a regular tiling of the Euclidean plane, in which exactly three hexagons meet at each vertex. It has Schläfli symbol of or (as a truncated triangular tiling).
English mathemat ...
, and hexagonal prism facets, with a mirrored sphenoid vertex figure.
Runcinated order-6 cubic honeycomb
The runcinated order-6 cubic honeycomb is the same as theruncinated order-4 hexagonal tiling honeycomb
In the field of hyperbolic geometry, the order-4 hexagonal tiling honeycomb arises as one of 11 regular paracompact honeycombs in 3-dimensional hyperbolic space. It is ''paracompact'' because it has cells composed of an infinite number of faces. ...
.
Runcitruncated order-6 cubic honeycomb
The runcitruncated order-6 cubic honeycomb, rr, has truncated cube,rhombitrihexagonal tiling
In geometry, the rhombitrihexagonal tiling is a semiregular tiling of the Euclidean plane. There are one triangle, two squares, and one hexagon on each vertex. It has Schläfli symbol of rr.
John Conway calls it a rhombihexadeltille.Conway, 2008, ...
, hexagonal prism, and octagonal prism facets, with an isosceles-trapezoidal pyramid vertex figure.
Runcicantellated order-6 cubic honeycomb
The runcicantellated order-6 cubic honeycomb is the same as the runcitruncated order-4 hexagonal tiling honeycomb.Omnitruncated order-6 cubic honeycomb
The omnitruncated order-6 cubic honeycomb is the same as the omnitruncated order-4 hexagonal tiling honeycomb.Alternated order-6 cubic honeycomb
In three-dimensional hyperbolic geometry, the alternated order-6 hexagonal tiling honeycomb is a uniform compact space-filling tessellation (or honeycomb). As an alternation, withSchläfli symbol
In geometry, the Schläfli symbol is a notation of the form \ that defines regular polytopes and tessellations.
The Schläfli symbol is named after the 19th-century Swiss mathematician Ludwig Schläfli, who generalized Euclidean geometry to more ...
h and Coxeter-Dynkin diagram or , it can be considered a quasiregular honeycomb, alternating triangular tilings and tetrahedra around each vertex in a trihexagonal tiling vertex figure.
Symmetry
A half-symmetry construction from the form exists, with two alternating types (colors) of triangular tiling cells. This form has Coxeter-Dynkin diagram ↔ . Another lower-symmetry form of index 6, ,3*,6 exists with a non-simplex fundamental domain, with Coxeter-Dynkin diagram .Related honeycombs
The alternated order-6 cubic honeycomb is part of a series of quasiregular polychora and honeycombs. It also has 3 related forms: the cantic order-6 cubic honeycomb, h2, ; the runcic order-6 cubic honeycomb, h3, ; and the runcicantic order-6 cubic honeycomb, h2,3, .Cantic order-6 cubic honeycomb
The cantic order-6 cubic honeycomb is a uniform compact space-filling tessellation (or honeycomb) withSchläfli symbol
In geometry, the Schläfli symbol is a notation of the form \ that defines regular polytopes and tessellations.
The Schläfli symbol is named after the 19th-century Swiss mathematician Ludwig Schläfli, who generalized Euclidean geometry to more ...
h2. It is composed of truncated tetrahedron, trihexagonal tiling, and hexagonal tiling
In geometry, the hexagonal tiling or hexagonal tessellation is a regular tiling of the Euclidean plane, in which exactly three hexagons meet at each vertex. It has Schläfli symbol of or (as a truncated triangular tiling).
English mathemat ...
facets, with a rectangular pyramid vertex figure.
Runcic order-6 cubic honeycomb
The runcic order-6 cubic honeycomb is a uniform compact space-filling tessellation (or honeycomb) withSchläfli symbol
In geometry, the Schläfli symbol is a notation of the form \ that defines regular polytopes and tessellations.
The Schläfli symbol is named after the 19th-century Swiss mathematician Ludwig Schläfli, who generalized Euclidean geometry to more ...
h3. It is composed of tetrahedron, hexagonal tiling
In geometry, the hexagonal tiling or hexagonal tessellation is a regular tiling of the Euclidean plane, in which exactly three hexagons meet at each vertex. It has Schläfli symbol of or (as a truncated triangular tiling).
English mathemat ...
, and rhombitrihexagonal tiling
In geometry, the rhombitrihexagonal tiling is a semiregular tiling of the Euclidean plane. There are one triangle, two squares, and one hexagon on each vertex. It has Schläfli symbol of rr.
John Conway calls it a rhombihexadeltille.Conway, 2008, ...
facets, with a triangular cupola vertex figure.
Runcicantic order-6 cubic honeycomb
The runcicantic order-6 cubic honeycomb is a uniform compact space-filling tessellation (or honeycomb), withSchläfli symbol
In geometry, the Schläfli symbol is a notation of the form \ that defines regular polytopes and tessellations.
The Schläfli symbol is named after the 19th-century Swiss mathematician Ludwig Schläfli, who generalized Euclidean geometry to more ...
h2,3. It is composed of truncated hexagonal tiling, truncated trihexagonal tiling, and truncated tetrahedron facets, with a mirrored sphenoid vertex figure.
See also
*Convex uniform honeycombs in hyperbolic space
In hyperbolic geometry, a uniform honeycomb in hyperbolic space is a uniform tessellation of uniform polyhedral cells. In 3-dimensional hyperbolic space there are nine Coxeter group families of compact convex uniform honeycombs, generated as ...
* Regular tessellations of hyperbolic 3-space
* Paracompact uniform honeycombs
References
* Coxeter, '' Regular Polytopes'', 3rd. ed., Dover Publications, 1973. . (Tables I and II: Regular polytopes and honeycombs, pp. 294–296) * ''The Beauty of Geometry: Twelve Essays'' (1999), Dover Publications, , (Chapter 10Regular Honeycombs in Hyperbolic Space
Table III * Jeffrey R. Weeks ''The Shape of Space, 2nd edition'' {{isbn, 0-8247-0709-5 (Chapter 16-17: Geometries on Three-manifolds I,II) * Norman Johnson ''Uniform Polytopes'', Manuscript ** N.W. Johnson: ''The Theory of Uniform Polytopes and Honeycombs'', Ph.D. Dissertation, University of Toronto, 1966 ** N.W. Johnson: ''Geometries and Transformations'', (2018) Chapter 13: Hyperbolic Coxeter groups Honeycombs (geometry)