|
Ring Modulator
In electronics, ring modulation is a signal processing function, an implementation of frequency mixing, in which two signals are combined to yield an output signal. One signal, called the carrier, is typically a sine wave or another simple waveform; the other signal is typically more complicated and is called the input or the modulator signal. A ring modulator is an electronic device for ring modulation. A ring modulator may be used in music synthesizers and as an effects unit. The name derives from the fact that the analog circuit of diodes originally used to implement this technique takes the shape of a ring: a diode ring. The circuit is similar to a bridge rectifier, except that instead of the diodes facing left or right, they face clockwise or counterclockwise. Ring modulation is quite similar to amplitude modulation, with the difference that in the latter the modulator is shifted to be positive before being multiplied with the carrier, while in the former the uns ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Sideband
In radio communications, a sideband is a band of frequencies higher than or lower than the carrier frequency, that are the result of the modulation process. The sidebands carry the information transmitted by the radio signal. The sidebands comprise all the spectral components of the modulated signal except the carrier. The signal components above the carrier frequency constitute the upper sideband (USB), and those below the carrier frequency constitute the lower sideband (LSB). All forms of modulation produce sidebands. Sideband creation We can illustrate the creation of sidebands with one trigonometric identity: :\cos(A)\cdot \cos(B) \equiv \tfrac\cos(A+B) + \tfrac\cos(A-B) Adding \cos(A) to both sides: :\cos(A)\cdot +\cos(B)= \tfrac\cos(A+B) + \cos(A) + \tfrac\cos(A-B) Substituting (for instance) A \triangleq 1000\cdot t and B \triangleq 100\cdot t, where t represents time: :\underbrace_\cdot \underbrace_ = \underbrace_ + \underbrace_ + \u ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Combination Tone
A combination tone (also called resultant or subjective tone)Combination Tone , ''Britannica.com''. Accessed September 2015. is a psychoacoustic phenomenon of an additional tone or tones that are artificially perceived when two real tones are sounded at the same time. Their discovery is credited to the violinist (although he was not the first, see ) and so they are also called Tartini tones. There ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Frequency Domain
In physics, electronics, control systems engineering, and statistics, the frequency domain refers to the analysis of mathematical functions or signals with respect to frequency, rather than time. Put simply, a time-domain graph shows how a signal changes over time, whereas a frequency-domain graph shows how much of the signal lies within each given frequency band over a range of frequencies. A frequency-domain representation can also include information on the phase shift that must be applied to each sinusoid in order to be able to recombine the frequency components to recover the original time signal. A given function or signal can be converted between the time and frequency domains with a pair of mathematical operators called transforms. An example is the Fourier transform, which converts a time function into a complex valued sum or integral of sine waves of different frequencies, with amplitudes and phases, each of which represents a frequency component. The "spec ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Convolution
In mathematics (in particular, functional analysis), convolution is a mathematical operation on two functions ( and ) that produces a third function (f*g) that expresses how the shape of one is modified by the other. The term ''convolution'' refers to both the result function and to the process of computing it. It is defined as the integral of the product of the two functions after one is reflected about the y-axis and shifted. The choice of which function is reflected and shifted before the integral does not change the integral result (see commutativity). The integral is evaluated for all values of shift, producing the convolution function. Some features of convolution are similar to cross-correlation: for real-valued functions, of a continuous or discrete variable, convolution (f*g) differs from cross-correlation (f \star g) only in that either or is reflected about the y-axis in convolution; thus it is a cross-correlation of and , or and . For complex-valued fu ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Time Domain
Time domain refers to the analysis of mathematical functions, physical signals or time series of economic or environmental data, with respect to time. In the time domain, the signal or function's value is known for all real numbers, for the case of continuous time, or at various separate instants in the case of discrete time. An oscilloscope is a tool commonly used to visualize real-world signals in the time domain. A time-domain graph shows how a signal changes with time, whereas a frequency-domain graph shows how much of the signal lies within each given frequency band over a range of frequencies. Though most precisely referring to time in physics, the term ''time domain'' may occasionally informally refer to position in space when dealing with spatial frequencies, as a substitute for the more precise term ''spatial domain''. Origin of term The use of the contrasting terms ''time domain'' and '' frequency domain'' developed in U.S. communication engineering in the ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Trigonometric Identity
In trigonometry, trigonometric identities are equalities that involve trigonometric functions and are true for every value of the occurring variables for which both sides of the equality are defined. Geometrically, these are identities involving certain functions of one or more angles. They are distinct from triangle identities, which are identities potentially involving angles but also involving side lengths or other lengths of a triangle. These identities are useful whenever expressions involving trigonometric functions need to be simplified. An important application is the integration of non-trigonometric functions: a common technique involves first using the substitution rule with a trigonometric function, and then simplifying the resulting integral with a trigonometric identity. Pythagorean identities The basic relationship between the sine and cosine is given by the Pythagorean identity: :\sin^2\theta + \cos^2\theta = 1, where \sin^2 \theta means (\sin \theta)^2 ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
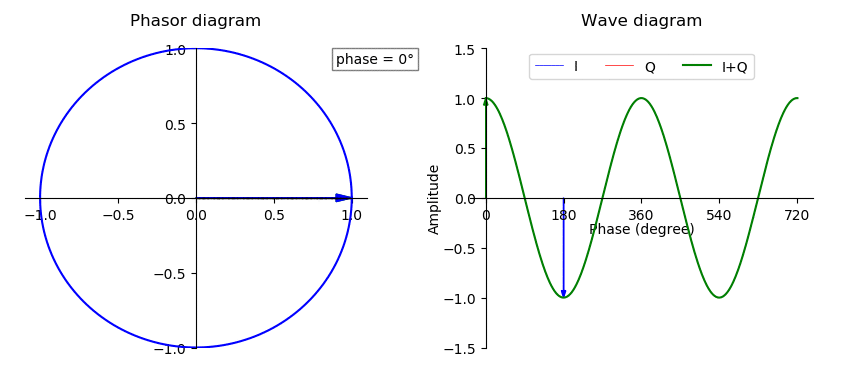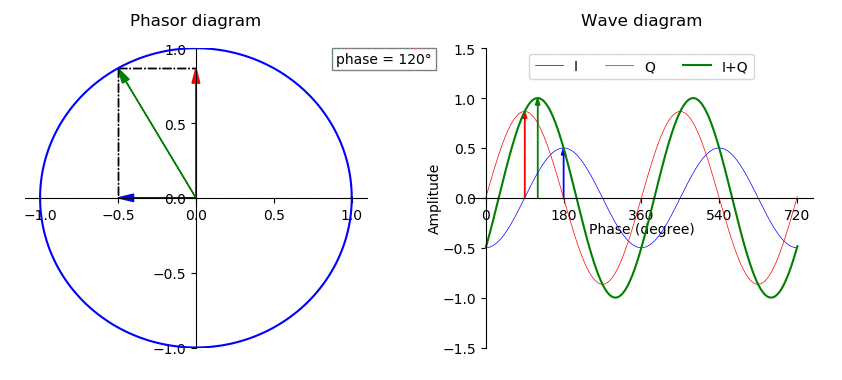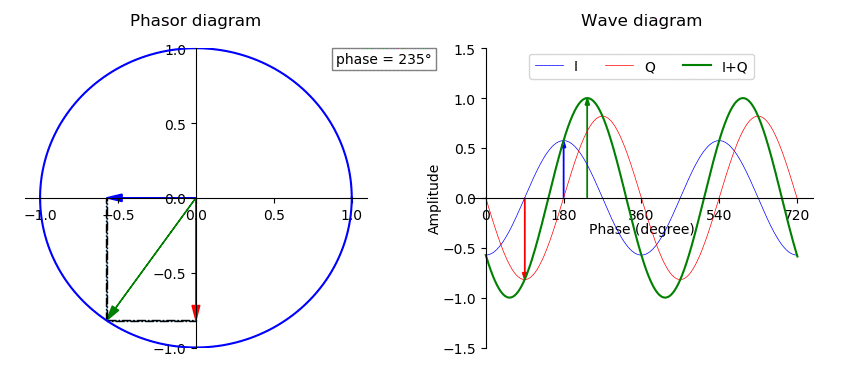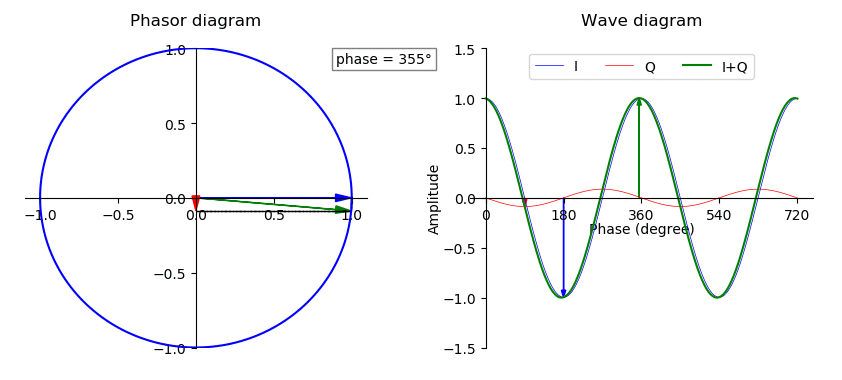
Phase Shift
In physics and mathematics, the phase of a periodic function F of some real variable t (such as time) is an angle-like quantity representing the fraction of the cycle covered up to t. It is denoted \phi(t) and expressed in such a scale that it varies by one full turn as the variable t goes through each period (and F(t) goes through each complete cycle). It may be measured in any angular unit such as degrees or radians, thus increasing by 360° or 2\pi as the variable t completes a full period. This convention is especially appropriate for a sinusoidal function, since its value at any argument t then can be expressed as \phi(t), the sine of the phase, multiplied by some factor (the amplitude of the sinusoid). (The cosine may be used instead of sine, depending on where one considers each period to start.) Usually, whole turns are ignored when expressing the phase; so that \phi(t) is also a periodic function, with the same period as F, that repeatedly scans the same range o ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Ring Modulation Two Forms Diode-clipping Or 'chopper' RM
Ring may refer to: * Ring (jewellery), a round band, usually made of metal, worn as ornamental jewelry * To make a sound with a bell, and the sound made by a bell :(hence) to initiate a telephone connection Arts, entertainment and media Film and literature * ''The Ring'' (franchise), a Japanese horror media franchise based on the novel series by Koji Suzuki ** ''Ring'' (novel series) *** ''Ring'' (Suzuki novel), 1991 ** ''Ring'' (film), or ''The Ring'', a 1998 Japanese horror film by Hideo Nakata *** ''The Ring'' (2002 film), an American horror film, remake of the 1998 Japanese film ** ''Ring'' (1995 film), a TV film ** ''Rings'' (2005 film), a short film by Jonathan Liebesman ** ''Rings'' (2017 film), an American horror film * ''Ring'' (Baxter novel), a 1994 science fiction novel * ''Ring'' (Alexis novel), a 2021 Canadian novel by André Alexis Gaming * ''Ring'' (video game), 1998 * Rings (''Sonic the Hedgehog''), a collectible in ''Sonic the Hedgehog'' games Music ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Frequency Mixer Mini Circuits ADE-1 Macro
Frequency is the number of occurrences of a repeating event per unit of time. It is also occasionally referred to as ''temporal frequency'' for clarity, and is distinct from ''angular frequency''. Frequency is measured in hertz (Hz) which is equal to one event per second. The period is the interval of time between events, so the period is the reciprocal of the frequency. For example, if a heart beats at a frequency of 120 times a minute (2 hertz), the period, —the interval at which the beats repeat—is half a second (60 seconds divided by 120 beats). Frequency is an important parameter used in science and engineering to specify the rate of oscillatory and vibratory phenomena, such as mechanical vibrations, audio signals (sound), radio waves, and light. Definitions and units For cyclical phenomena such as oscillations, waves, or for examples of simple harmonic motion, the term ''frequency'' is defined as the number of cycles or vibrations per unit of time. T ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Allen Strange
Allen Strange (June 26, 1943 – February 20, 2008http://www.kitsapsun.com/news/2008/feb/25/allen-strange-leader-in-electronic-music-dies/ ''Allen Strange, Leader in Electronic Music, Dies at 64'' By Rachel Pritchet, Kitsap Sun) was an American composer. He authored two books, ''Electronic Music: Systems, Techniques, and Controls'' (first published in the 1970s) and ''Programming and Meta-Programming the Electro-Organism''. He co-wrote ''The Contemporary Violin: Extended Performance Techniques'' with his wife, Patricia. Career Strange was born in Calexico, California. He studied composition with Donal Michalsky at the California State University, Fullerton. He received his MA in 1967. He later studied composition with Robert Erickson, Harry Partch, and Ken Gaburo, and electronic media with Pauline Oliveros at the University of California, San Diego during 1967–68 and 1970–71. In 1970, Strange became a professor of music and the director of the electronic music studios ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |

.gif)