|
Median Absolute Deviation
In statistics, the median absolute deviation (MAD) is a robust measure of the variability of a univariate sample of quantitative data. It can also refer to the population parameter that is estimated by the MAD calculated from a sample. For a univariate data set ''X''1, ''X''2, ..., ''Xn'', the MAD is defined as the median of the absolute deviations from the data's median \tilde=\operatorname(X) : : \operatorname = \operatorname( , X_i - \tilde, ) that is, starting with the residuals (deviations) from the data's median, the MAD is the median of their absolute values. Example Consider the data (1, 1, 2, 2, 4, 6, 9). It has a median value of 2. The absolute deviations about 2 are (1, 1, 0, 0, 2, 4, 7) which in turn have a median value of 1 (because the sorted absolute deviations are (0, 0, 1, 1, 2, 4, 7)). So the median absolute deviation for this data is 1. Uses The median absolute deviation is a measure of statistical dispersion. Moreover, the MAD is ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Probable Error
In statistics, probable error defines the half-range of an interval about a central point for the distribution, such that half of the values from the distribution will lie within the interval and half outside.Dodge, Y. (2006) ''The Oxford Dictionary of Statistical Terms'', OUP. Thus for a symmetric distribution it is equivalent to half the interquartile range, or the median absolute deviation. One such use of the term ''probable error'' in this sense is as the name for the scale parameter of the Cauchy distribution, which does not have a standard deviation. The probable error can also be expressed as a multiple of the standard deviation σ,Zwillinger, D.; Kokosa, S. (2000) ''CRC Standard Probability and Statistics Tables and Formulae'', Chapman & Hall/CRC. (Section 2.2.13) which requires that at least the second statistical moment of the distribution should exist, whereas the other definition does not. For a normal distribution this is \gamma = 0.6745 \times \sigma (see deta ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Geometric Median
In geometry, the geometric median of a discrete set of sample points in a Euclidean space is the point minimizing the sum of distances to the sample points. This generalizes the median, which has the property of minimizing the sum of distances for one-dimensional data, and provides a central tendency in higher dimensions. It is also known as the 1-median, spatial median, Euclidean minisum point, or Torricelli point. The geometric median is an important estimator of location in statistics, where it is also known as the ''L''1 estimator. It is also a standard problem in facility location, where it models the problem of locating a facility to minimize the cost of transportation. The special case of the problem for three points in the plane (that is, = 3 and = 2 in the definition below) is sometimes also known as Fermat's problem; it arises in the construction of minimal Steiner trees, and was originally posed as a problem by Pierre de Fermat and solved by Evangelista To ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Statistics
Statistics (from German: '' Statistik'', "description of a state, a country") is the discipline that concerns the collection, organization, analysis, interpretation, and presentation of data. In applying statistics to a scientific, industrial, or social problem, it is conventional to begin with a statistical population or a statistical model to be studied. Populations can be diverse groups of people or objects such as "all people living in a country" or "every atom composing a crystal". Statistics deals with every aspect of data, including the planning of data collection in terms of the design of surveys and experiments.Dodge, Y. (2006) ''The Oxford Dictionary of Statistical Terms'', Oxford University Press. When census data cannot be collected, statisticians collect data by developing specific experiment designs and survey samples. Representative sampling assures that inferences and conclusions can reasonably extend from the sample to the population as a whole. An ex ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Consistent Estimator
In statistics, a consistent estimator or asymptotically consistent estimator is an estimator—a rule for computing estimates of a parameter ''θ''0—having the property that as the number of data points used increases indefinitely, the resulting sequence of estimates converges in probability to ''θ''0. This means that the distributions of the estimates become more and more concentrated near the true value of the parameter being estimated, so that the probability of the estimator being arbitrarily close to ''θ''0 converges to one. In practice one constructs an estimator as a function of an available sample of size ''n'', and then imagines being able to keep collecting data and expanding the sample ''ad infinitum''. In this way one would obtain a sequence of estimates indexed by ''n'', and consistency is a property of what occurs as the sample size “grows to infinity”. If the sequence of estimates can be mathematically shown to converge in probability to the true value ' ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Percentile
In statistics, a ''k''-th percentile (percentile score or centile) is a score ''below which'' a given percentage ''k'' of scores in its frequency distribution falls (exclusive definition) or a score ''at or below which'' a given percentage falls (inclusive definition). For example, the 50th percentile (the median) is the score below which 50% of the scores in the distribution are found (by the "exclusive" definition), or at or below which 50% of the scores are found (by the "inclusive" definition). Percentiles are expressed in the same unit of measurement as the input scores; for example, if the scores refer to human weight, the corresponding percentiles will be expressed in kilograms or pounds. The percentile score and the ''percentile rank'' are related terms. The percentile rank of a score is the percentage of scores in its distribution that are less than it, an exclusive definition, and one that can be expressed with a single, simple formula. Percentile scores and percen ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Probability Distribution
In probability theory and statistics, a probability distribution is the mathematical function that gives the probabilities of occurrence of different possible outcomes for an experiment. It is a mathematical description of a random phenomenon in terms of its sample space and the probabilities of events (subsets of the sample space). For instance, if is used to denote the outcome of a coin toss ("the experiment"), then the probability distribution of would take the value 0.5 (1 in 2 or 1/2) for , and 0.5 for (assuming that the coin is fair). Examples of random phenomena include the weather conditions at some future date, the height of a randomly selected person, the fraction of male students in a school, the results of a survey to be conducted, etc. Introduction A probability distribution is a mathematical description of the probabilities of events, subsets of the sample space. The sample space, often denoted by \Omega, is the set of all possible outcomes of a ra ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Complex Number
In mathematics, a complex number is an element of a number system that extends the real numbers with a specific element denoted , called the imaginary unit and satisfying the equation i^= -1; every complex number can be expressed in the form a + bi, where and are real numbers. Because no real number satisfies the above equation, was called an imaginary number by René Descartes. For the complex number a+bi, is called the , and is called the . The set of complex numbers is denoted by either of the symbols \mathbb C or . Despite the historical nomenclature "imaginary", complex numbers are regarded in the mathematical sciences as just as "real" as the real numbers and are fundamental in many aspects of the scientific description of the natural world. Complex numbers allow solutions to all polynomial equations, even those that have no solutions in real numbers. More precisely, the fundamental theorem of algebra asserts that every non-constant polynomial equation with re ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Half-normal Distribution
In probability theory and statistics, the half-normal distribution is a special case of the folded normal distribution. Let X follow an ordinary normal distribution, N(0,\sigma^2). Then, Y=, X, follows a half-normal distribution. Thus, the half-normal distribution is a fold at the mean of an ordinary normal distribution with mean zero. Properties Using the \sigma parametrization of the normal distribution, the probability density function (PDF) of the half-normal is given by : f_Y(y; \sigma) = \frac\exp \left( -\frac \right) \quad y \geq 0, where E = \mu = \frac. Alternatively using a scaled precision (inverse of the variance) parametrization (to avoid issues if \sigma is near zero), obtained by setting \theta=\frac, the probability density function is given by : f_Y(y; \theta) = \frac\exp \left( -\frac \right) \quad y \geq 0, where E = \mu = \frac. The cumulative distribution function (CDF) is given by : F_Y(y; \sigma) = \int_0^y \frac\sqrt \, \exp \left( -\frac \rig ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
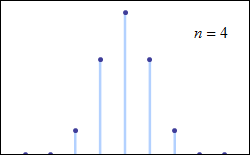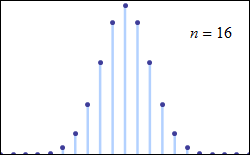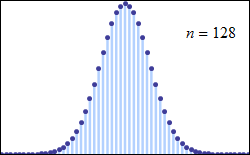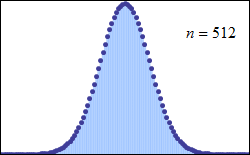
Standard Normal Distribution
In statistics, a normal distribution or Gaussian distribution is a type of continuous probability distribution for a real-valued random variable. The general form of its probability density function is : f(x) = \frac e^ The parameter \mu is the mean or expectation of the distribution (and also its median and mode), while the parameter \sigma is its standard deviation. The variance of the distribution is \sigma^2. A random variable with a Gaussian distribution is said to be normally distributed, and is called a normal deviate. Normal distributions are important in statistics and are often used in the natural and social sciences to represent real-valued random variables whose distributions are not known. Their importance is partly due to the central limit theorem. It states that, under some conditions, the average of many samples (observations) of a random variable with finite mean and variance is itself a random variable—whose distribution converges to a normal dist ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Cumulative Distribution Function
In probability theory and statistics, the cumulative distribution function (CDF) of a real-valued random variable X, or just distribution function of X, evaluated at x, is the probability that X will take a value less than or equal to x. Every probability distribution supported on the real numbers, discrete or "mixed" as well as continuous, is uniquely identified by an ''upwards continuous'' ''monotonic increasing'' cumulative distribution function F : \mathbb R \rightarrow ,1/math> satisfying \lim_F(x)=0 and \lim_F(x)=1. In the case of a scalar continuous distribution, it gives the area under the probability density function from minus infinity to x. Cumulative distribution functions are also used to specify the distribution of multivariate random variables. Definition The cumulative distribution function of a real-valued random variable X is the function given by where the right-hand side represents the probability that the random variable X takes on a value less ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Quantile Function
In probability and statistics, the quantile function, associated with a probability distribution of a random variable, specifies the value of the random variable such that the probability of the variable being less than or equal to that value equals the given probability. Intuitively, the quantile function associates with a range at and below a probability input the likelihood that a random variable is realized in that range for some probability distribution. It is also called the percentile function, percent-point function or inverse cumulative distribution function. Definition Strictly monotonic distribution function With reference to a continuous and strictly monotonic cumulative distribution function F_X\colon \mathbb \to ,1/math> of a random variable ''X'', the quantile function Q\colon , 1\to \mathbb returns a threshold value ''x'' below which random draws from the given c.d.f. would fall ''100*p'' percent of the time. In terms of the distribution function ''F'', the q ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Multiplicative Inverse
In mathematics, a multiplicative inverse or reciprocal for a number ''x'', denoted by 1/''x'' or ''x''−1, is a number which when multiplied by ''x'' yields the multiplicative identity, 1. The multiplicative inverse of a fraction ''a''/''b'' is ''b''/''a''. For the multiplicative inverse of a real number, divide 1 by the number. For example, the reciprocal of 5 is one fifth (1/5 or 0.2), and the reciprocal of 0.25 is 1 divided by 0.25, or 4. The reciprocal function, the function ''f''(''x'') that maps ''x'' to 1/''x'', is one of the simplest examples of a function which is its own inverse (an involution). Multiplying by a number is the same as dividing by its reciprocal and vice versa. For example, multiplication by 4/5 (or 0.8) will give the same result as division by 5/4 (or 1.25). Therefore, multiplication by a number followed by multiplication by its reciprocal yields the original number (since the product of the number and its reciprocal is 1). The term ''reciprocal' ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |