|
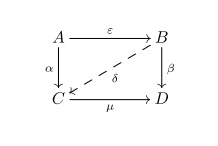
Monomorphisms
In the context of abstract algebra or universal algebra, a monomorphism is an injective homomorphism. A monomorphism from to is often denoted with the notation X\hookrightarrow Y. In the more general setting of category theory, a monomorphism (also called a monic morphism or a mono) is a left-cancellative morphism. That is, an arrow such that for all objects and all morphisms , : f \circ g_1 = f \circ g_2 \implies g_1 = g_2. Monomorphisms are a categorical generalization of injective functions (also called "one-to-one functions"); in some categories the notions coincide, but monomorphisms are more general, as in the examples below. The categorical dual of a monomorphism is an epimorphism, that is, a monomorphism in a category ''C'' is an epimorphism in the dual category ''C''op. Every section is a monomorphism, and every retraction is an epimorphism. Relation to invertibility Left-invertible morphisms are necessarily monic: if ''l'' is a left inverse for ''f'' (meani ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Monomorphism Scenarios
In the context of abstract algebra or universal algebra, a monomorphism is an injective homomorphism. A monomorphism from to is often denoted with the notation X\hookrightarrow Y. In the more general setting of category theory, a monomorphism (also called a monic morphism or a mono) is a left-cancellative morphism. That is, an arrow such that for all objects and all morphisms , : f \circ g_1 = f \circ g_2 \implies g_1 = g_2. Monomorphisms are a categorical generalization of injective functions (also called "one-to-one functions"); in some categories the notions coincide, but monomorphisms are more general, as in the examples below. The categorical dual of a monomorphism is an epimorphism, that is, a monomorphism in a category ''C'' is an epimorphism in the dual category ''C''op. Every section is a monomorphism, and every retraction is an epimorphism. Relation to invertibility Left-invertible morphisms are necessarily monic: if ''l'' is a left inverse for ''f'' (meaning ' ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Epimorphism
In category theory, an epimorphism (also called an epic morphism or, colloquially, an epi) is a morphism ''f'' : ''X'' → ''Y'' that is right-cancellative in the sense that, for all objects ''Z'' and all morphisms , : g_1 \circ f = g_2 \circ f \implies g_1 = g_2. Epimorphisms are categorical analogues of onto or surjective functions (and in the category of sets the concept corresponds exactly to the surjective functions), but they may not exactly coincide in all contexts; for example, the inclusion \mathbb\to\mathbb is a ring epimorphism. The dual of an epimorphism is a monomorphism (i.e. an epimorphism in a category ''C'' is a monomorphism in the dual category ''C''op). Many authors in abstract algebra and universal algebra define an epimorphism simply as an ''onto'' or surjective homomorphism. Every epimorphism in this algebraic sense is an epimorphism in the sense of category theory, but the converse is not true in all categories. In this article, the term "epimorphism" w ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Abelian Category
In mathematics, an abelian category is a category in which morphisms and objects can be added and in which kernels and cokernels exist and have desirable properties. The motivating prototypical example of an abelian category is the category of abelian groups, Ab. The theory originated in an effort to unify several cohomology theories by Alexander Grothendieck and independently in the slightly earlier work of David Buchsbaum. Abelian categories are very ''stable'' categories; for example they are regular and they satisfy the snake lemma. The class of abelian categories is closed under several categorical constructions, for example, the category of chain complexes of an abelian category, or the category of functors from a small category to an abelian category are abelian as well. These stability properties make them inevitable in homological algebra and beyond; the theory has major applications in algebraic geometry, cohomology and pure category theory. Abelian categories are na ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Epic Morphism
In category theory, an epimorphism (also called an epic morphism or, colloquially, an epi) is a morphism ''f'' : ''X'' → ''Y'' that is right-cancellative in the sense that, for all objects ''Z'' and all morphisms , : g_1 \circ f = g_2 \circ f \implies g_1 = g_2. Epimorphisms are categorical analogues of onto or surjective functions (and in the category of sets the concept corresponds exactly to the surjective functions), but they may not exactly coincide in all contexts; for example, the inclusion \mathbb\to\mathbb is a ring epimorphism. The dual of an epimorphism is a monomorphism (i.e. an epimorphism in a category ''C'' is a monomorphism in the dual category ''C''op). Many authors in abstract algebra and universal algebra define an epimorphism simply as an ''onto'' or surjective homomorphism. Every epimorphism in this algebraic sense is an epimorphism in the sense of category theory, but the converse is not true in all categories. In this article, the term "epimorphism" wi ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Morphism
In mathematics, particularly in category theory, a morphism is a structure-preserving map from one mathematical structure to another one of the same type. The notion of morphism recurs in much of contemporary mathematics. In set theory, morphisms are functions; in linear algebra, linear transformations; in group theory, group homomorphisms; in topology, continuous functions, and so on. In category theory, ''morphism'' is a broadly similar idea: the mathematical objects involved need not be sets, and the relationships between them may be something other than maps, although the morphisms between the objects of a given category have to behave similarly to maps in that they have to admit an associative operation similar to function composition. A morphism in category theory is an abstraction of a homomorphism. The study of morphisms and of the structures (called "objects") over which they are defined is central to category theory. Much of the terminology of morphisms, as well as the ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Homomorphism
In algebra, a homomorphism is a structure-preserving map between two algebraic structures of the same type (such as two groups, two rings, or two vector spaces). The word ''homomorphism'' comes from the Ancient Greek language: () meaning "same" and () meaning "form" or "shape". However, the word was apparently introduced to mathematics due to a (mis)translation of German meaning "similar" to meaning "same". The term "homomorphism" appeared as early as 1892, when it was attributed to the German mathematician Felix Klein (1849–1925). Homomorphisms of vector spaces are also called linear maps, and their study is the subject of linear algebra. The concept of homomorphism has been generalized, under the name of morphism, to many other structures that either do not have an underlying set, or are not algebraic. This generalization is the starting point of category theory. A homomorphism may also be an isomorphism, an endomorphism, an automorphism, etc. (see below). Each of th ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Section (category Theory)
In category theory, a branch of mathematics, a section is a right inverse of some morphism. Dually, a retraction is a left inverse of some morphism. In other words, if f: X\to Y and g: Y\to X are morphisms whose composition f \circ g: Y\to Y is the identity morphism on Y, then g is a section of f, and f is a retraction of g. Every section is a monomorphism (every morphism with a left inverse is left-cancellative), and every retraction is an epimorphism (every morphism with a right inverse is right-cancellative). In algebra, sections are also called split monomorphisms and retractions are also called split epimorphisms. In an abelian category, if f: X\to Y is a split epimorphism with split monomorphism g: Y\to X, then X is isomorphic to the direct sum of Y and the kernel of f. The synonym coretraction for section is sometimes seen in the literature, although rarely in recent work. Properties * A section that is also an epimorphism is an isomorphism. Dually a retraction that is al ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Retract (category Theory)
In category theory, a branch of mathematics, a section is a right inverse of some morphism. Dually, a retraction is a left inverse of some morphism. In other words, if f: X\to Y and g: Y\to X are morphisms whose composition f \circ g: Y\to Y is the identity morphism on Y, then g is a section of f, and f is a retraction of g. Every section is a monomorphism (every morphism with a left inverse is left-cancellative), and every retraction is an epimorphism (every morphism with a right inverse is right-cancellative). In algebra, sections are also called split monomorphisms and retractions are also called split epimorphisms. In an abelian category, if f: X\to Y is a split epimorphism with split monomorphism g: Y\to X, then X is isomorphic to the direct sum of Y and the kernel of f. The synonym coretraction for section is sometimes seen in the literature, although rarely in recent work. Properties * A section that is also an epimorphism is an isomorphism. Dually a retraction that is al ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Section (category Theory)
In category theory, a branch of mathematics, a section is a right inverse of some morphism. Dually, a retraction is a left inverse of some morphism. In other words, if f: X\to Y and g: Y\to X are morphisms whose composition f \circ g: Y\to Y is the identity morphism on Y, then g is a section of f, and f is a retraction of g. Every section is a monomorphism (every morphism with a left inverse is left-cancellative), and every retraction is an epimorphism (every morphism with a right inverse is right-cancellative). In algebra, sections are also called split monomorphisms and retractions are also called split epimorphisms. In an abelian category, if f: X\to Y is a split epimorphism with split monomorphism g: Y\to X, then X is isomorphic to the direct sum of Y and the kernel of f. The synonym coretraction for section is sometimes seen in the literature, although rarely in recent work. Properties * A section that is also an epimorphism is an isomorphism. Dually a retraction that is al ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Topos
In mathematics, a topos (, ; plural topoi or , or toposes) is a category that behaves like the category of sheaves of sets on a topological space (or more generally: on a site). Topoi behave much like the category of sets and possess a notion of localization; they are a direct generalization of point-set topology. The Grothendieck topoi find applications in algebraic geometry; the more general elementary topoi are used in logic. The mathematical field that studies topoi is called topos theory. Grothendieck topos (topos in geometry) Since the introduction of sheaves into mathematics in the 1940s, a major theme has been to study a space by studying sheaves on a space. This idea was expounded by Alexander Grothendieck by introducing the notion of a "topos". The main utility of this notion is in the abundance of situations in mathematics where topological heuristics are very effective, but an honest topological space is lacking; it is sometimes possible to find a topos formaliz ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Quotient Group
A quotient group or factor group is a mathematical group obtained by aggregating similar elements of a larger group using an equivalence relation that preserves some of the group structure (the rest of the structure is "factored" out). For example, the cyclic group of addition modulo ''n'' can be obtained from the group of integers under addition by identifying elements that differ by a multiple of n and defining a group structure that operates on each such class (known as a congruence class) as a single entity. It is part of the mathematical field known as group theory. For a congruence relation on a group, the equivalence class of the identity element is always a normal subgroup of the original group, and the other equivalence classes are precisely the cosets of that normal subgroup. The resulting quotient is written G\,/\,N, where G is the original group and N is the normal subgroup. (This is pronounced G\bmod N, where \mbox is short for modulo.) Much of the importance o ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Abelian Group
In mathematics, an abelian group, also called a commutative group, is a group in which the result of applying the group operation to two group elements does not depend on the order in which they are written. That is, the group operation is commutative. With addition as an operation, the integers and the real numbers form abelian groups, and the concept of an abelian group may be viewed as a generalization of these examples. Abelian groups are named after early 19th century mathematician Niels Henrik Abel. The concept of an abelian group underlies many fundamental algebraic structures, such as fields, rings, vector spaces, and algebras. The theory of abelian groups is generally simpler than that of their non-abelian counterparts, and finite abelian groups are very well understood and fully classified. Definition An abelian group is a set A, together with an operation \cdot that combines any two elements a and b of A to form another element of A, denoted a \cdot b. The symbo ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |