|
Entanglement-assisted Code
In the theory of quantum communication, the entanglement-assisted stabilizer formalism is a method for protecting quantum information with the help of entanglement shared between a sender and receiver before they transmit quantum data over a quantum communication channel. It extends the standard stabilizer formalism by including shared entanglement (Brun ''et al.'' 2006). The advantage of entanglement-assisted stabilizer codes is that the sender can exploit the error-correcting properties of an arbitrary set of Pauli operators. The sender's Pauli operators do not necessarily have to form an Abelian subgroup of the Pauli group \Pi^ over n qubits. The sender can make clever use of her shared ebits so that the global stabilizer is Abelian and thus forms a valid quantum error-correcting code. Definition We review the construction of an entanglement-assisted code (Brun ''et al.'' 2006). Suppose that there is a nonabelian subgroup \mathcal\subset\Pi^ of size n-k=2c+s. Applicati ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Quantum Communication
Quantum information science is an interdisciplinary field that seeks to understand the analysis, processing, and transmission of information using quantum mechanics principles. It combines the study of Information science with quantum mechanics, quantum effects in physics. It includes theoretical issues in computational models and more experimental topics in quantum physics, including what can and cannot be done with quantum information. The term quantum information theory is also used, but it fails to encompass experimental research, and can be confused with a subfield of quantum information science that addresses the processing of quantum information. Scientific and engineering studies To understand quantum teleportation, quantum entanglement and the manufacturing of quantum computer hardware requires a thorough understanding of quantum physics and engineering. Since 2010s, there has been remarkable progress in manufacturing quantum computers, with companies like Google and IBM in ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Commutativity
In mathematics, a binary operation is commutative if changing the order of the operands does not change the result. It is a fundamental property of many binary operations, and many mathematical proofs depend on it. Most familiar as the name of the property that says something like or , the property can also be used in more advanced settings. The name is needed because there are operations, such as division and subtraction, that do not have it (for example, ); such operations are ''not'' commutative, and so are referred to as ''noncommutative operations''. The idea that simple operations, such as the multiplication and addition of numbers, are commutative was for many years implicitly assumed. Thus, this property was not named until the 19th century, when mathematics started to become formalized. A similar property exists for binary relations; a binary relation is said to be symmetric if the relation applies regardless of the order of its operands; for example, equality is s ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Entanglement Assisted Capacity
In the theory of quantum communication, the entanglement-assisted classical capacity of a quantum channel is the highest rate at which classical information can be transmitted from a sender to receiver when they share an unlimited amount of noiseless entanglement. It is given by the quantum mutual information of the channel, which is the input-output quantum mutual information maximized over all pure bipartite quantum states with one system transmitted through the channel. This formula is the natural generalization of Shannon's noisy channel coding theorem, in the sense that this formula is equal to the capacity, and there is no need to regularize it. An additional feature that it shares with Shannon's formula is that a noiseless classical or quantum feedback channel cannot increase the entanglement-assisted classical capacity. The entanglement-assisted classical capacity theorem is proved in two parts: the direct coding theorem and the converse theorem. The direct coding the ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Quantum Channel
In quantum information theory, a quantum channel is a communication channel which can transmit quantum information, as well as classical information. An example of quantum information is the state of a qubit. An example of classical information is a text document transmitted over the Internet. More formally, quantum channels are completely positive (CP) trace-preserving maps between spaces of operators. In other words, a quantum channel is just a quantum operation viewed not merely as the reduced dynamics of a system but as a pipeline intended to carry quantum information. (Some authors use the term "quantum operation" to also include trace-decreasing maps while reserving "quantum channel" for strictly trace-preserving maps.) Memoryless quantum channel We will assume for the moment that all state spaces of the systems considered, classical or quantum, are finite-dimensional. The memoryless in the section title carries the same meaning as in classical information theory: the ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Nonabelian Group
In mathematics, and specifically in group theory, a non-abelian group, sometimes called a non-commutative group, is a group (''G'', ∗) in which there exists at least one pair of elements ''a'' and ''b'' of ''G'', such that ''a'' ∗ ''b'' ≠ ''b'' ∗ ''a''. This class of groups contrasts with the abelian groups. (In an abelian group, all pairs of group elements commute). Non-abelian groups are pervasive in mathematics and physics. One of the simplest examples of a non-abelian group is the dihedral group of order 6. It is the smallest finite non-abelian group. A common example from physics is the rotation group SO(3) in three dimensions (for example, rotating something 90 degrees along one axis and then 90 degrees along a different axis is not the same as doing them in reverse order). Both discrete groups and continuous groups may be non-abelian. Most of the interesting Lie groups are non-abelian, and these play an important role in gauge theory. ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Commuting
Commuting is periodically recurring travel between one's place of residence and place of work or study, where the traveler, referred to as a commuter, leaves the boundary of their home community. By extension, it can sometimes be any regular or often repeated travel between locations, even when not work-related. The modes of travel, time taken and distance traveled in commuting varies widely across the globe. Most people in least-developed countries continue to walk to work. The cheapest method of commuting after walking is usually by bicycle, so this is common in low-income countries, but is also increasingly practised by people in wealthier countries for environmental and health reasons. In middle-income countries, motorcycle commuting is very common. The next technology adopted as countries develop is more dependent on location: in more populous, older cities, especially in Eurasia mass transit (rail, bus, etc.) predominates, while in smaller, younger cities, and larg ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Eigenstate
In quantum physics, a quantum state is a mathematical entity that provides a probability distribution for the outcomes of each possible measurement on a system. Knowledge of the quantum state together with the rules for the system's evolution in time exhausts all that can be predicted about the system's behavior. A mixture of quantum states is again a quantum state. Quantum states that cannot be written as a mixture of other states are called pure quantum states, while all other states are called mixed quantum states. A pure quantum state can be represented by a ray in a Hilbert space over the complex numbers, while mixed states are represented by density matrices, which are positive semidefinite operators that act on Hilbert spaces. Pure states are also known as state vectors or wave functions, the latter term applying particularly when they are represented as functions of position or momentum. For example, when dealing with the energy spectrum of the electron in a hydrogen ato ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Anticommuting
In mathematics, anticommutativity is a specific property of some non- commutative mathematical operations. Swapping the position of two arguments of an antisymmetric operation yields a result which is the ''inverse'' of the result with unswapped arguments. The notion ''inverse'' refers to a group structure on the operation's codomain, possibly with another operation. Subtraction is an anticommutative operation because commuting the operands of gives for example, Another prominent example of an anticommutative operation is the Lie bracket. In mathematical physics, where symmetry is of central importance, these operations are mostly called antisymmetric operations, and are extended in an associative setting to cover more than two arguments. Definition If A, B are two abelian groups, a bilinear map f\colon A^2 \to B is anticommutative if for all x, y \in A we have :f(x, y) = - f(y, x). More generally, a multilinear map g : A^n \to B is anticommutative if for all x_1, \ ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
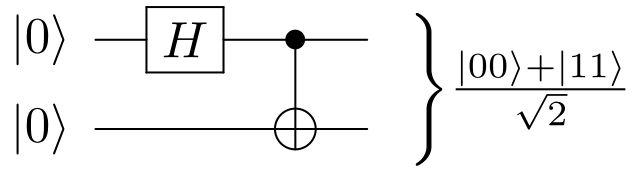
Bell State
The Bell states or EPR pairs are specific quantum states of two qubits that represent the simplest (and maximal) examples of quantum entanglement; conceptually, they fall under the study of quantum information science. The Bell states are a form of entangled and normalized basis vectors. This normalization implies that the overall probability of the particle being in one of the mentioned states is 1: \langle \Phi, \Phi \rangle = 1. Entanglement is a basis-independent result of superposition. Due to this superposition, measurement of the qubit will " collapse" it into one of its basis states with a given probability. Because of the entanglement, measurement of one qubit will "collapse" the other qubit to a state whose measurement will yield one of two possible values, where the value depends on which Bell state the two qubits are in initially. Bell states can be generalized to certain quantum states of multi-qubit systems, such as the GHZ state for 3 or more subsystems. Under ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Symplectic Geometry
Symplectic geometry is a branch of differential geometry and differential topology that studies symplectic manifolds; that is, differentiable manifolds equipped with a closed differential form, closed, nondegenerate form, nondegenerate differential form, 2-form. Symplectic geometry has its origins in the Hamiltonian mechanics, Hamiltonian formulation of classical mechanics where the phase space of certain classical systems takes on the structure of a symplectic manifold. The term "symplectic", introduced by Weyl, is a calque of "complex"; previously, the "symplectic group" had been called the "line complex group". "Complex" comes from the Latin ''com-plexus'', meaning "braided together" (co- + plexus), while symplectic comes from the corresponding Greek ''sym-plektikos'' (συμπλεκτικός); in both cases the stem comes from the Indo-European root wiktionary:Reconstruction:Proto-Indo-European/pleḱ-, *pleḱ- The name reflects the deep connections between complex and sym ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Stabilizer Formalism
The theory of quantum error correction plays a prominent role in the practical realization and engineering of quantum computing and quantum communication devices. The first quantum error-correcting codes are strikingly similar to classical block codes in their operation and performance. Quantum error-correcting codes restore a noisy, decohered quantum state to a pure quantum state. A stabilizer quantum error-correcting code appends ancilla qubits to qubits that we want to protect. A unitary encoding circuit rotates the global state into a subspace of a larger Hilbert space. This highly entangled, encoded state corrects for local noisy errors. A quantum error-correcting code makes quantum computation and quantum communication practical by providing a way for a sender and receiver to simulate a noiseless qubit channel given a noisy qubit channel whose noise conforms to a particular error model. The stabilizer theory of quantum error correction allows one to import some class ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Non-abelian Group
In mathematics, and specifically in group theory, a non-abelian group, sometimes called a non-commutative group, is a group (''G'', ∗) in which there exists at least one pair of elements ''a'' and ''b'' of ''G'', such that ''a'' ∗ ''b'' ≠ ''b'' ∗ ''a''. This class of groups contrasts with the abelian groups. (In an abelian group, all pairs of group elements commute). Non-abelian groups are pervasive in mathematics and physics. One of the simplest examples of a non-abelian group is the dihedral group of order 6. It is the smallest finite non-abelian group. A common example from physics is the rotation group SO(3) in three dimensions (for example, rotating something 90 degrees along one axis and then 90 degrees along a different axis is not the same as doing them in reverse order). Both discrete groups and continuous groups may be non-abelian. Most of the interesting Lie groups are non-abelian, and these play an important role in gauge theory. ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |