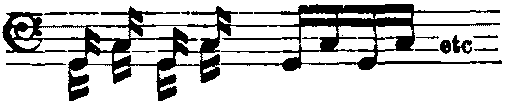|
Cross-beat
In music, a cross-beat or cross-rhythm is a specific form of polyrhythm. The term ''cross rhythm '' was introduced in 1934 by the musicologist Arthur Morris Jones (1889–1980). It refers to when the rhythmic conflict found in polyrhythms is the basis of an entire musical piece. Etymology The term "cross rhythm" was introduced in 1934 by the musicologist Arthur Morris Jones (1889–1980), who, with Klaus Wachsmann, took-up extended residence in Zambia and Uganda, respectively, as missionaries, educators, musicologists, and museologists. African music One main system African cross-rhythm is most prevalent within the greater Niger-Congo linguistic group, which dominates the continent south of the Sahara Desert. (Kubik, p. 58) Cross-rhythm was first identified as the basis of sub-Saharan rhythm by A.M. Jones. Later, the concept was more fully explained in the lectures of Ewe master drummer and scholar C.K. Ladzekpo, and in the writings of David Locke. Jones observes ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Cross-beat Escapement
An escapement is a mechanical linkage in mechanical watches and clocks that gives impulses to the timekeeping element and periodically releases the gear train to move forward, advancing the clock's hands. The impulse action transfers energy to the clock's timekeeping element (usually a pendulum or balance wheel) to replace the energy lost to friction during its cycle and keep the timekeeper oscillating. The escapement is driven by force from a coiled spring or a suspended weight, transmitted through the timepiece's gear train. Each swing of the pendulum or balance wheel releases a tooth of the escapement's ''escape wheel'', allowing the clock's gear train to advance or "escape" by a fixed amount. This regular periodic advancement moves the clock's hands forward at a steady rate. At the same time, the tooth gives the timekeeping element a push, before another tooth catches on the escapement's pallet, returning the escapement to its "locked" state. The sudden stopping of the esc ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Tonguing
Tonguing is a technique used with wind instruments to enunciate notes using the tongue on the palate or the reed or mouthpiece. A silent "tee" is made when the tongue strikes the reed or roof of the mouth causing a slight breach in the air flow through the instrument. If a more soft tone is desired, the syllable "da" (as in ''dou''ble) is preferred. The technique also works for whistling. Tonguing also refers to articulation, which is how a musician begins the note (punchy, legato, or a breath attack) and how the note is released (air release, tongued release, etc.) For wind players, articulation is commonly spoken of in terms of tonguing because the tongue is used to stop and allow air to flow in the mouth. Tonguing does not apply to non wind instruments, but articulation does apply to all instruments. An alteration called "double-tonguing" or "double-articulation" is used when the music being performed has many rapid notes in succession too fast for regular articulation. I ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Ostinato
In music, an ostinato (; derived from Italian word for ''stubborn'', compare English ''obstinate'') is a motif or phrase that persistently repeats in the same musical voice, frequently in the same pitch. Well-known ostinato-based pieces include classical compositions such as Ravel's '' Boléro'' and the ''Carol of the Bells'', and popular songs such as Donna Summer and Giorgio Moroder's "I Feel Love" (1977), Henry Mancini's theme from ''Peter Gunn'' (1959), The Who's "Baba O'Riley" (1971), and The Verve's " Bitter Sweet Symphony" (1997). Both ''ostinatos'' and ''ostinati'' are accepted English plural forms, the latter reflecting the word's Italian etymology. The repeating idea may be a rhythmic pattern, part of a tune, or a complete melody in itself. Kamien, Roger (1258). ''Music: An Appreciation'', p. 611. . Strictly speaking, ostinati should have exact repetition, but in common usage, the term covers repetition with variation and development, such as the alteration of an os ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Gyil
The balafon is a gourd-resonated xylophone, a type of struck idiophone. It is closely associated with the neighbouring Mandé, Senoufo and Gur peoples of West Africa, particularly the Guinean branch of the Mandinka ethnic group, but is now found across West Africa from Guinea to Mali. Its common name, ''balafon'', is likely a European coinage combining its Mandinka name ''bala'' with the word ''fôn'' 'to speak' or the Greek root ''phono''. History Believed to have been developed independently of the Southern African and South American instrument now called the marimba, oral histories of the balafon date it to at least the rise of the Mali Empire in the 12th century CE. Balafon is a Manding name, but variations exist across West Africa, including the ''balangi'' in Sierra Leone and the #Gyil, gyil of the Dagara, Lobi and Gurunsi from Ghana, Burkina Faso and Ivory Coast. Similar instruments are played in parts of Central Africa, with the ancient Kingdom of Kongo denoting ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Balafon
The balafon is a gourd-resonated xylophone, a type of struck idiophone. It is closely associated with the neighbouring Mandé, Senoufo and Gur peoples of West Africa, particularly the Guinean branch of the Mandinka ethnic group, but is now found across West Africa from Guinea to Mali. Its common name, ''balafon'', is likely a European coinage combining its Mandinka name ''bala'' with the word ''fôn'' 'to speak' or the Greek root ''phono''. History Believed to have been developed independently of the Southern African and South American instrument now called the marimba, oral histories of the balafon date it to at least the rise of the Mali Empire in the 12th century CE. Balafon is a Manding name, but variations exist across West Africa, including the ''balangi'' in Sierra Leone and the #Gyil, gyil of the Dagara, Lobi and Gurunsi from Ghana, Burkina Faso and Ivory Coast. Similar instruments are played in parts of Central Africa, with the ancient Kingdom of Kongo denoting ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Xylophone
The xylophone (; ) is a musical instrument in the percussion family that consists of wooden bars struck by mallets. Like the glockenspiel (which uses metal bars), the xylophone essentially consists of a set of tuned wooden keys arranged in the fashion of the keyboard of a piano. Each bar is an idiophone tuned to a pitch of a musical scale, whether pentatonic or heptatonic in the case of many African and Asian instruments, diatonic in many western children's instruments, or chromatic for orchestral use. The term ''xylophone'' may be used generally, to include all such instruments such as the marimba, balafon and even the semantron. However, in the orchestra, the term ''xylophone'' refers specifically to a chromatic instrument of somewhat higher pitch range and drier timbre than the marimba, and these two instruments should not be confused. A person who plays the xylophone is known as a ''xylophonist'' or simply a ''xylophone player''. The term is also popularly used to refer to ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Victor Kofi Agawu
Victor Kofi Agawu (born 28 September 1956) is a Ghanaian musicologist and music theorist. He often publishes as V. Kofi Agawu and specializes in musical semiotics and ethnomusicology. He is a Distinguished Professor at the Graduate Center, CUNY. Education Victor Kofi Agawu was born on 28 September 1956 in Hohoe, the Volta Region of Ghana. Agawu attended Presbyterian Boys' Secondary School at Legon (PRESEC) where he obtained his GCE Ordinary Level Certificate and went on to do this Advanced Level at Achimota School. He earned a bachelor's degree in music from the University of Reading in the United Kingdom in 1977, a master's degree in musical analysis from King's College London in 1978, and a Ph.D. in historical musicology from Stanford University in 1982. He is also certified by the Royal Academy of Music in the teaching of singing and by the Royal College of Music in musicianship and theory. Career Agawu has taught at Princeton University, Yale University, Cornell Univ ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Cycle (music)
Cycle has several meanings in the field of music. Acoustically, it refers to one complete vibration, the base unit of Hertz being one cycle per second. Theoretically, an interval cycle is a collection of pitch classes created by a sequence of identical intervals. Individual pieces that aggregate into larger works are considered cycles, for example, the movements of a suite, symphony, sonata, or string quartet. This definition can apply to everything from settings of the Mass or a song cycle to an opera cycle. Cycle also applies to the complete performance of an individual composer's work in one genre. Harmonic cycles—repeated sequences of a harmonic progression—are at the root of many musical genres, such as the twelve-bar blues. In compositions of this genre, the chord progression may be repeated indefinitely, with melodic and lyrical variation forming the musical interest. The form theme and variations is essentially of this type, but generally on a larger scale. Composition ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Cell (music)
The 1957 ''Encyclopédie Larousse''quoted in Nattiez, Jean-Jacques (1990). ''Music and Discourse: Toward a Semiology of Music'' (''Musicologie générale et sémiologue'', 1987). Translated by Carolyn Abbate (1990). . defines a cell in music as a "small rhythmic and melodic design that can be isolated, or can make up one part of a thematic context". The cell may be distinguished from the figure or motif: the 1958 ''Encyclopédie Fasquelle'' defines a cell as "the smallest indivisible unit", unlike the motif, which may be divisible into more than one cell. "A cell can be developed, independent of its context, as a melodic fragment, it can be used as a developmental motif. It can be the source for the whole structure of the work; in that case it is called a generative cell." A rhythmic cell is a cell without melodic connotations. It may be entirely percussive or applied to different melodic segments. History The term "cell" (German: ''Keim'') derives from organic music theorists ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Hemiola
In music, hemiola (also hemiolia) is the ratio 3:2. The equivalent Latin term is sesquialtera. In rhythm, ''hemiola'' refers to three beats of equal value in the time normally occupied by two beats. In pitch, ''hemiola'' refers to the interval of a perfect fifth. Etymology The word ''hemiola'' comes from the Greek adjective ἡμιόλιος, ''hemiolios'', meaning "containing one and a half," "half as much again," "in the ratio of one and a half to one (3:2), as in musical sounds." The words "hemiola" and "sesquialtera" both signify the ratio 3:2, and in music were first used to describe relations of pitch. Dividing the string of a monochord in this ratio produces the interval of a perfect fifth. Beginning in the 15th century, both words were also used to describe rhythmic relationships, specifically the substitution (usually through the use of coloration—red notes in place of black ones, or black in place of "white", hollow noteheads) of three Mensural notation#Proportions a ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Ratio
In mathematics, a ratio shows how many times one number contains another. For example, if there are eight oranges and six lemons in a bowl of fruit, then the ratio of oranges to lemons is eight to six (that is, 8:6, which is equivalent to the ratio 4:3). Similarly, the ratio of lemons to oranges is 6:8 (or 3:4) and the ratio of oranges to the total amount of fruit is 8:14 (or 4:7). The numbers in a ratio may be quantities of any kind, such as counts of people or objects, or such as measurements of lengths, weights, time, etc. In most contexts, both numbers are restricted to be Positive integer, positive. A ratio may be specified either by giving both constituting numbers, written as "''a'' to ''b''" or "''a'':''b''", or by giving just the value of their quotient Equal quotients correspond to equal ratios. Consequently, a ratio may be considered as an ordered pair of numbers, a Fraction (mathematics), fraction with the first number in the numerator and the second in the denom ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
