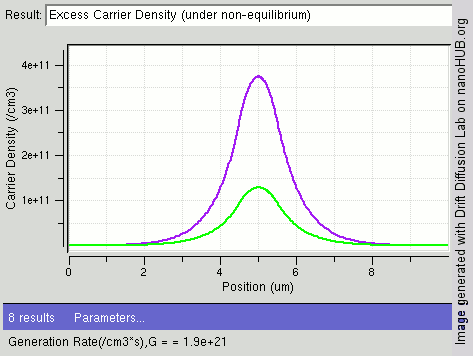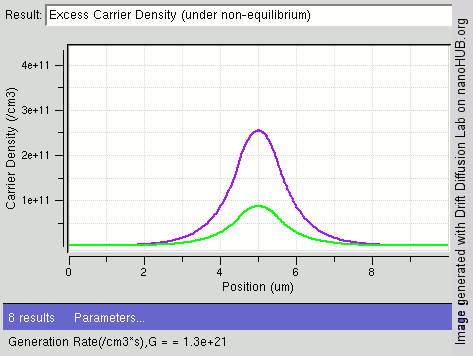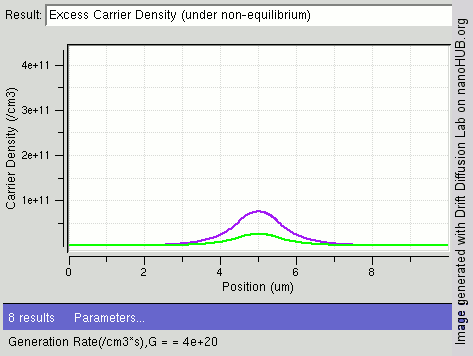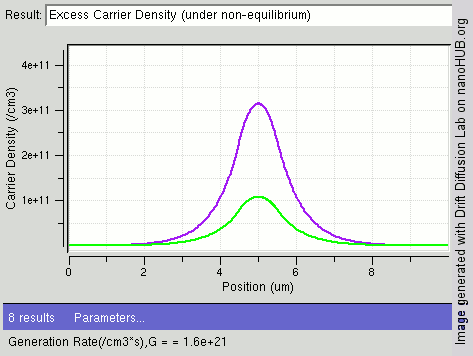
|
Adjoint Equation
An adjoint equation is a linear differential equation, usually derived from its primal equation using integration by parts. Gradient values with respect to a particular quantity of interest can be efficiently calculated by solving the adjoint equation. Methods based on solution of adjoint equations are used in wing shape optimization, Flow control (fluid), fluid flow control and uncertainty quantification. For example dX_t = a(X_t)dt + b(X_t)dW this is an Itō calculus, Itō stochastic differential equation. Now by using Euler scheme, we integrate the parts of this equation and get another equation, X_ = X_n + a \Delta t + \zeta b \sqrt, here \zeta is a random variable, later one is an adjoint equation. Example: Advection-Diffusion PDE Consider the following linear, scalar Convection–diffusion_equation, advection-diffusion equation for the primal solution u(\vec), in the domain \Omega with Dirichlet boundary conditions: : \begin \nabla \cdot \left(\vec u - \mu \nabla u \righ ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Linear Differential Equation
In mathematics, a linear differential equation is a differential equation that is defined by a linear polynomial in the unknown function and its derivatives, that is an equation of the form :a_0(x)y + a_1(x)y' + a_2(x)y'' \cdots + a_n(x)y^ = b(x) where and are arbitrary differentiable functions that do not need to be linear, and are the successive derivatives of an unknown function of the variable . Such an equation is an ordinary differential equation (ODE). A ''linear differential equation'' may also be a linear partial differential equation (PDE), if the unknown function depends on several variables, and the derivatives that appear in the equation are partial derivatives. A linear differential equation or a system of linear equations such that the associated homogeneous equations have constant coefficients may be solved by quadrature, which means that the solutions may be expressed in terms of integrals. This is also true for a linear equation of order one, with non-con ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Integration By Parts
In calculus, and more generally in mathematical analysis, integration by parts or partial integration is a process that finds the integral of a product of functions in terms of the integral of the product of their derivative and antiderivative. It is frequently used to transform the antiderivative of a product of functions into an antiderivative for which a solution can be more easily found. The rule can be thought of as an integral version of the product rule of differentiation. The integration by parts formula states: \begin \int_a^b u(x) v'(x) \, dx & = \Big (x) v(x)\Biga^b - \int_a^b u'(x) v(x) \, dx\\ & = u(b) v(b) - u(a) v(a) - \int_a^b u'(x) v(x) \, dx. \end Or, letting u = u(x) and du = u'(x) \,dx while v = v(x) and dv = v'(x) \, dx, the formula can be written more compactly: \int u \, dv \ =\ uv - \int v \, du. Mathematician Brook Taylor discovered integration by parts, first publishing the idea in 1715. More general formulations of integration by parts ex ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Wing Shape Optimization
Wing-shape optimization is a software implementation of shape optimization primarily used for aircraft design. This allows for engineers to produce more efficient and cheaper aircraft designs. History Shape optimization, as a software process and tool, first appeared as an algorithm in 1995 and as commercial software for the automotive industry by 1998, as noted by F. Muyl. Relative to the age of the automotive and aeronautical companies, this software is very new. The difficulty was not with the science behind the process, but rather the capabilities of computer hardware. In 1998, F. Muyl developed a compromise between exact accuracy and computational time to reduce drag of an automotive. GA phases are the standard genetic algorithm iterations and the BFGS phases are the approximated calculations designed to save time. However, he acknowledged that the computational time required on existing hardware, nearly two weeks for a moderate improvement on an oversimplified proof of co ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Flow Control (fluid)
Flow control is a major rapidly-evolving field of fluid dynamics. It implies a small change of a configuration serving an ideally large engineering benefit, like drag reduction, lift increase, mixing enhancement or noise reduction. This change may be accomplished by passive or active devices. Passive devices, like turbulators or roughness elements, are steady and require no energy by definition. Active control requires actuators that may be driven in a time-dependent manner and require energy. Examples are valves and plasma actuators. The actuation command may be pre-determined (open-loop control) or be dependent on sensors monitoring the flow state (closed-loop control). Active control Airplane wing performance has a substantial effect on not only the runway length, approach speed, climb rate, cargo capacity, and operation range but also the community noise and emission levels. The wing performance is often degraded by flow separation, which strongly depends on the aerodynamic de ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Uncertainty Quantification
Uncertainty quantification (UQ) is the science of quantitative characterization and reduction of uncertainties in both computational and real world applications. It tries to determine how likely certain outcomes are if some aspects of the system are not exactly known. An example would be to predict the acceleration of a human body in a head-on crash with another car: even if the speed was exactly known, small differences in the manufacturing of individual cars, how tightly every bolt has been tightened, etc., will lead to different results that can only be predicted in a statistical sense. Many problems in the natural sciences and engineering are also rife with sources of uncertainty. Computer experiments on computer simulations are the most common approach to study problems in uncertainty quantification. Sources Uncertainty can enter mathematical models and experimental measurements in various contexts. One way to categorize the sources of uncertainty is to consider: ; Paramet ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Itō Calculus
Itō may refer to: *Itō (surname), a Japanese surname *Itō, Shizuoka, Shizuoka Prefecture, Japan *Ito District, Wakayama Prefecture, Japan See also * Itô's lemma, used in stochastic calculus *Itoh–Tsujii inversion algorithm, in field theory *Itô calculus, an extension of calculus to stochastic processes, named after Kiyoshi Itô *Ito (other) *ITO (other) Ito may refer to: Places * Ito Island, an island of Milne Bay Province, Papua New Guinea * Ito Airport, an airport in the Democratic Republic of the Congo * Ito District, Wakayama, a district located in Wakayama Prefecture, Japan * Itō, Shizuo ..., for the three-letter acronym {{DEFAULTSORT:Ito es:Ito fr:Ito nl:Ito ja:いとう pt:Ito ru:Ито ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Convection–diffusion Equation
The convection–diffusion equation is a combination of the diffusion equation, diffusion and convection (advection equation, advection) equations, and describes physical phenomena where particles, energy, or other physical quantities are transferred inside a physical system due to two processes: diffusion and convection. Depending on context, the same equation can be called the advection–diffusion equation, drift velocity, drift–diffusion equation, or (generic) scalar transport equation. Equation General The general equation is \frac = \mathbf \cdot (D \mathbf c) - \mathbf \cdot (\mathbf c) + R where * is the variable of interest (species concentration for mass transfer, temperature for heat transfer), * is the diffusivity (also called diffusion coefficient), such as mass diffusivity for particle motion or thermal diffusivity for heat transport, * is the velocity field that the quantity is moving with. It is a function of time and space. For example, in advection, might be t ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Dirichlet Boundary Conditions
In the mathematical study of differential equations, the Dirichlet (or first-type) boundary condition is a type of boundary condition, named after Peter Gustav Lejeune Dirichlet (1805–1859). When imposed on an ordinary or a partial differential equation, it specifies the values that a solution needs to take along the boundary of the domain. In finite element method (FEM) analysis, ''essential'' or Dirichlet boundary condition is defined by weighted-integral form of a differential equation. The dependent unknown ''u in the same form as the weight function w'' appearing in the boundary expression is termed a ''primary variable'', and its specification constitutes the ''essential'' or Dirichlet boundary condition. The question of finding solutions to such equations is known as the Dirichlet problem. In applied sciences, a Dirichlet boundary condition may also be referred to as a fixed boundary condition. Examples ODE For an ordinary differential equation, for instance, y'' + y ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Weak Formulation
Weak formulations are important tools for the analysis of mathematical equations that permit the transfer of concepts of linear algebra to solve problems in other fields such as partial differential equations. In a weak formulation, equations or conditions are no longer required to hold absolutely (and this is not even well defined) and has instead weak solutions only with respect to certain "test vectors" or "test functions". In a strong formulation, the solution space is constructed such that these equations or conditions are already fulfilled. The Lax–Milgram theorem, named after Peter Lax and Arthur Milgram who proved it in 1954, provides weak formulations for certain systems on Hilbert spaces. General concept Let V be a Banach space, V' its dual space, A\colon V \to V', and f \in V'. Finding the solution u \in V of the equation Au = f is equivalent to finding u\in V such that, for all v \in V, uv) = f(v). Here, v is called a test vector or test function. To bring this ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Adjoint State Method
The adjoint state method is a numerical method for efficiently computing the gradient of a function or operator in a numerical optimization problem. It has applications in geophysics, seismic imaging, photonics and more recently in neural networks. The adjoint state space is chosen to simplify the physical interpretation of equation constraints.Plessix, R-E. "A review of the adjoint-state method for computing the gradient of a functional with geophysical applications." Geophysical Journal International,2006,167(2): 495-503free access on GJI website/ref> Adjoint state techniques allow the use of integration by parts, resulting in a form which explicitly contains the physically interesting quantity. An adjoint state equation is introduced, including a new unknown variable. The adjoint method formulates the gradient of a function towards its parameters in a constraint optimization form. By using the dual form of this constraint optimization problem, it can be used to calculat ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Costate Equations
The costate equation is related to the state equation used in optimal control. It is also referred to as auxiliary, adjoint, influence, or multiplier equation. It is stated as a vector of first order differential equations : \dot^(t)=-\frac where the right-hand side is the vector of partial derivatives of the negative of the Hamiltonian with respect to the state variables. Interpretation The costate variables \lambda(t) can be interpreted as Lagrange multipliers associated with the state equations. The state equations represent constraints of the minimization problem, and the costate variables represent the marginal cost of violating those constraints; in economic terms the costate variables are the shadow prices. Solution The state equation is subject to an initial condition and is solved forwards in time. The costate equation must satisfy a transversality condition and is solved backwards in time, from the final time towards the beginning. For more details see Pontryagin ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |