|
Wythoff's Construction
In geometry, a Wythoff construction, named after mathematician Willem Abraham Wythoff, is a method for constructing a uniform polyhedron or plane tiling. It is often referred to as Wythoff's kaleidoscope, kaleidoscopic construction. Construction process The method is based on the idea of tessellation, tiling a sphere, with spherical triangles – see Schwarz triangles. This construction arranges three mirrors at the sides of a triangle, like in a kaleidoscope. However, different from a kaleidoscope, the mirrors are not parallel, but intersect at a single point. They therefore enclose a spherical triangle on the surface of any sphere centered on that point and repeated reflections produce a multitude of copies of the triangle. If the angles of the spherical triangle are chosen appropriately, the triangles will tile the sphere, one or more times. If one places a vertex at a suitable point inside the spherical triangle enclosed by the mirrors, it is possible to ensure that the r ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Uniform Tiling 44-t12
A uniform is a variety of clothing worn by members of an organization while participating in that organization's activity. Modern uniforms are most often worn by armed forces and paramilitary organizations such as police, emergency services, security guards, in some workplaces and schools and by inmates in prisons. In some countries, some other officials also wear uniforms in their duties; such is the case of the Commissioned Corps of the United States Public Health Service or the French prefects. For some organizations, such as police, it may be illegal for non members to wear the uniform. Etymology From the Latin ''unus'', one, and ''forma'', form. Corporate and work uniforms Workers sometimes wear uniforms or corporate clothing of one nature or another. Workers required to wear a uniform may include retail workers, bank and post-office workers, public-security and health-care workers, blue-collar employees, personal trainers in health clubs, instructors in summer camp ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
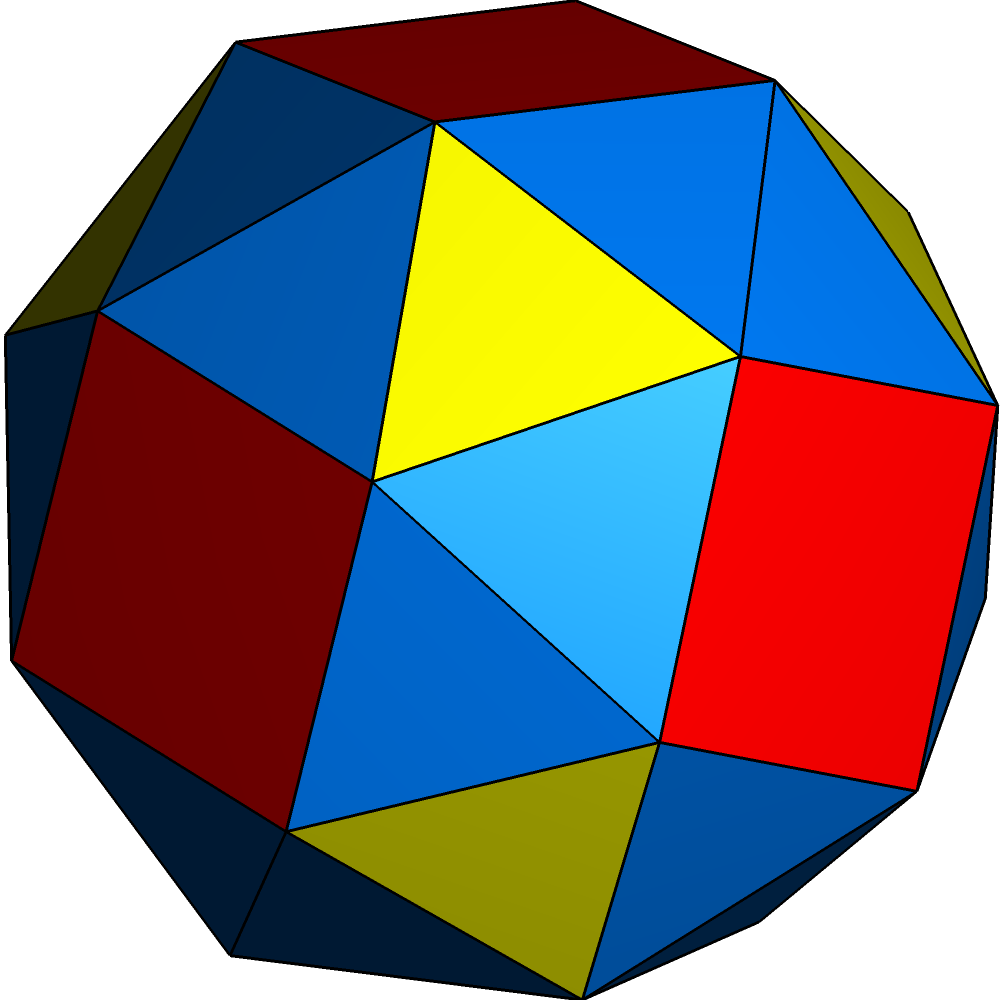
Elongated Triangular Tiling
In geometry, the elongated triangular tiling is a semiregular tiling of the Euclidean plane. There are three triangles and two squares on each vertex. It is named as a triangular tiling elongated by rows of squares, and given Schläfli symbol :e. Conway calls it a isosnub quadrille.Conway, 2008, p.288 table There are 3 regular and 8 semiregular tilings in the plane. This tiling is similar to the snub square tiling In geometry, the snub square tiling is a semiregular tiling of the Euclidean plane. There are three triangles and two squares on each vertex. Its Schläfli symbol is ''s''. Conway calls it a snub quadrille, constructed by a snub operation applie ... which also has 3 triangles and two squares on a vertex, but in a different order. Construction It is also the only convex uniform tiling that can not be created as a Wythoff construction. It can be constructed as alternate layers of apeirogonal prisms and apeirogonal antiprisms. Uniform colorings There is one un ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Tiling Semiregular 3-3-3-4-4 Elongated Triangular
{{disambiguation, surname ...
Tiling may refer to: *The physical act of laying tiles *Tessellations Computing *The compiler optimization of loop tiling *Tiled rendering, the process of subdividing an image by regular grid *Tiling window manager People *Heinrich Sylvester Theodor Tiling (1818–1871), physician and botanist *Reinhold Tiling (1893–1933), German rocket pioneer Other uses *Neuronal tiling *Tile drainage, an agriculture practice that removes excess water from soil *Tiling (crater), a small, undistinguished crater on the far side of the Moon See also *Brickwork *Packing (other) *Tiling puzzle Tiling puzzles are puzzles involving two-dimensional packing problems in which a number of flat shapes have to be assembled into a larger given shape without overlaps (and often without gaps). Some tiling puzzles ask you to dissect a given ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Dodecagonal Prism
In geometry, the dodecagonal prism is the tenth in an infinite set of prisms, formed by square sides and two regular dodecagon caps. If faces are all regular, it is a uniform polyhedron. Use It is used in the construction of two prismatic uniform honeycomb In geometry, a uniform honeycomb or uniform tessellation or infinite uniform polytope, is a vertex-transitive honeycomb made from uniform polytope facets. All of its vertices are identical and there is the same combination and arrangement of face ...s: The new British one pound (£1) coin, which entered circulation in March 2017, is shaped like a dodecagonal prism. Related polyhedra References External links * Prismatoid polyhedra Zonohedra {{Polyhedron-stub ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Hexagonal Antiprism
In geometry, the hexagonal antiprism is the 4th in an infinite set of antiprisms formed by an even-numbered sequence of triangle sides closed by two polygon caps. Antiprisms are similar to prisms except the bases are twisted relative to each other, and that the side faces are triangles, rather than quadrilaterals. In the case of a regular 6-sided base, one usually considers the case where its copy is twisted by an angle . Extra regularity is obtained by the line connecting the base centers being perpendicular to the base planes, making it a right antiprism. As faces, it has the two bases and, connecting those bases, isosceles triangles. If faces are all regular, it is a semiregular polyhedron. Crossed antiprism A crossed hexagonal antiprism is a star polyhedron, topologically identical to the convex ''hexagonal antiprism'' with the same vertex arrangement, but it can't be made uniform; the sides are isosceles triangles. Its vertex configuration is 3.3/2.3.6, with one triangl ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Hexagonal Antiprism
In geometry, the hexagonal antiprism is the 4th in an infinite set of antiprisms formed by an even-numbered sequence of triangle sides closed by two polygon caps. Antiprisms are similar to prisms except the bases are twisted relative to each other, and that the side faces are triangles, rather than quadrilaterals. In the case of a regular 6-sided base, one usually considers the case where its copy is twisted by an angle . Extra regularity is obtained by the line connecting the base centers being perpendicular to the base planes, making it a right antiprism. As faces, it has the two bases and, connecting those bases, isosceles triangles. If faces are all regular, it is a semiregular polyhedron. Crossed antiprism A crossed hexagonal antiprism is a star polyhedron, topologically identical to the convex ''hexagonal antiprism'' with the same vertex arrangement, but it can't be made uniform; the sides are isosceles triangles. Its vertex configuration is 3.3/2.3.6, with one triangl ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Snub (geometry)
In geometry, a snub is an operation applied to a polyhedron. The term originates from Kepler's names of two Archimedean solids, for the snub cube () and snub dodecahedron (). In general, snubs have chiral symmetry with two forms: with clockwise or counterclockwise orientation. By Kepler's names, a snub can be seen as an expansion of a regular polyhedron: moving the faces apart, twisting them about their centers, adding new polygons centered on the original vertices, and adding pairs of triangles fitting between the original edges. The terminology was generalized by Coxeter, with a slightly different definition, for a wider set of uniform polytopes. Conway snubs John Conway explored generalized polyhedron operators, defining what is now called Conway polyhedron notation, which can be applied to polyhedra and tilings. Conway calls Coxeter's operation a ''semi-snub''. In this notation, snub is defined by the dual and gyro operators, as ''s'' = ''dg'', and it is equiva ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Alternation (geometry)
In geometry, an alternation or ''partial truncation'', is an operation on a polygon, polyhedron, tiling, or higher dimensional polytope that removes alternate vertices.Coxeter, Regular polytopes, pp. 154–156 8.6 Partial truncation, or alternation Coxeter labels an ''alternation'' by a prefixed ''h'', standing for ''hemi'' or ''half''. Because alternation reduces all polygon faces to half as many sides, it can only be applied to polytopes with all even-sided faces. An alternated square face becomes a digon, and being degenerate, is usually reduced to a single edge. More generally any vertex-uniform polyhedron or tiling with a vertex configuration consisting of all even-numbered elements can be ''alternated''. For example, the alternation of a vertex figure with ''2a.2b.2c'' is ''a.3.b.3.c.3'' where the three is the number of elements in this vertex figure. A special case is square faces whose order divides in half into degenerate digons. So for example, the cube ''4.4.4'' i ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Uniform Polytope
In geometry, a uniform polytope of dimension three or higher is a vertex-transitive polytope bounded by uniform facets. The uniform polytopes in two dimensions are the regular polygons (the definition is different in 2 dimensions to exclude vertex-transitive even-sided polygons that alternate two different lengths of edges). This is a generalization of the older category of ''semiregular'' polytopes, but also includes the regular polytopes. Further, star regular faces and vertex figures ( star polygons) are allowed, which greatly expand the possible solutions. A strict definition requires uniform polytopes to be finite, while a more expansive definition allows uniform honeycombs (2-dimensional tilings and higher dimensional honeycombs) of Euclidean and hyperbolic space to be considered polytopes as well. Operations Nearly every uniform polytope can be generated by a Wythoff construction, and represented by a Coxeter diagram. Notable exceptions include the great dirhombic ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Octahedral Group
A regular octahedron has 24 rotational (or orientation-preserving) symmetries, and 48 symmetries altogether. These include transformations that combine a reflection and a rotation. A cube has the same set of symmetries, since it is the polyhedron that is dual to an octahedron. The group of orientation-preserving symmetries is ''S''4, the symmetric group or the group of permutations of four objects, since there is exactly one such symmetry for each permutation of the four diagonals of the cube. Details Chiral and full (or achiral) octahedral symmetry are the discrete point symmetries (or equivalently, symmetries on the sphere) with the largest symmetry groups compatible with translational symmetry. They are among the crystallographic point groups of the cubic crystal system. As the hyperoctahedral group of dimension 3 the full octahedral group is the wreath product S_2 \wr S_3 \simeq S_2^3 \rtimes S_3,and a natural way to identify its elements is as pairs (m, n) with m \ ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
