|
Walrasian Equilibrium
Competitive equilibrium (also called: Walrasian equilibrium) is a concept of economic equilibrium introduced by Kenneth Arrow and Gérard Debreu in 1951 appropriate for the analysis of commodity markets with flexible prices and many traders, and serving as the benchmark of efficiency in economic analysis. It relies crucially on the assumption of a competitive environment where each trader decides upon a quantity that is so small compared to the total quantity traded in the market that their individual transactions have no influence on the prices. Competitive markets are an ideal standard by which other market structures are evaluated. Definitions A competitive equilibrium (CE) consists of two elements: * A price function P. It takes as argument a vector representing a bundle of commodities, and returns a positive real number that represents its price. Usually the price function is linear - it is represented as a vector of prices, a price for each commodity type. * An allocation ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
|
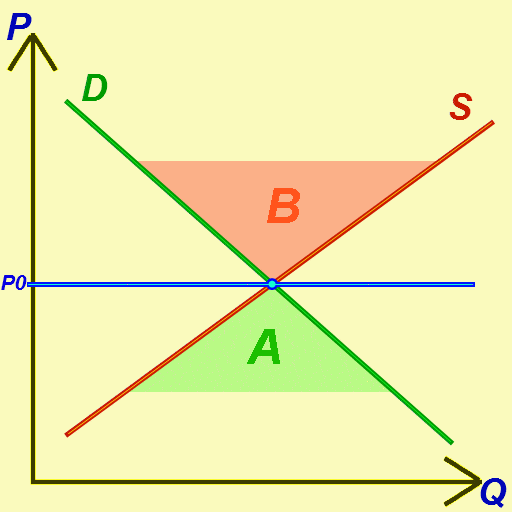 |
Economic Equilibrium
In economics, economic equilibrium is a situation in which economic forces such as supply and demand are balanced and in the absence of external influences the ( equilibrium) values of economic variables will not change. For example, in the standard text perfect competition, equilibrium occurs at the point at which quantity demanded and quantity supplied are equal. Market equilibrium in this case is a condition where a market price is established through competition such that the amount of goods or services sought by buyers is equal to the amount of goods or services produced by sellers. This price is often called the competitive price or market clearing price and will tend not to change unless demand or supply changes, and quantity is called the "competitive quantity" or market clearing quantity. But the concept of ''equilibrium'' in economics also applies to imperfectly competitive markets, where it takes the form of a Nash equilibrium. Understanding economic equilib ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
|
Finding An Equilibrium
Find, FIND or Finding may refer to: Computing * find (Unix), a command on UNIX platforms * find (Windows), a command on DOS/Windows platforms Books * ''The Find'' (2010), by Kathy Page * ''The Find'' (2014), by William Hope Hodgson Film and television * "The Find", an episode of '' Beyond Belief: Fact or Fiction'' * "The Find", an episode of reality TV show '' The Curse of Oak Island'' Music * ''Find'' (Hidden in Plain View EP), 2001 * ''Find'' (SS501 EP) * ''The Find'', a 2005 hip hop album by Ohmega Watts People * Áed Find (died 778), king of Dál Riata (modern-day Scotland) * Caittil Find, Norse-Gaelic warrior contingent leader * Cumméne Find (died 669), seventh abbot of Iona, Scotland Other uses * Find, in archaeology * Finding (jewelcrafting), jewellery components * Meteorite find, a found meteorite not observed to have fallen * Foundation for Innovative New Diagnostics, a not-for-profit organisation * Facial Images National Database See also * Discovery (obser ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
|
|
Fisher Market
Fisher market is an economic model attributed to Irving Fisher. It has the following ingredients: * A set of m divisible products with pre-specified supplies (usually normalized such that the supply of each good is 1). * A set of n buyers. * For each buyer i=1,\dots,n, there is a pre-specified monetary budget B_i. Each product j has a price p_j; the prices are determined by methods described below. The price of a ''bundle'' of products is the sum of the prices of the products in the bundle. A bundle is represented by a vector x = x_1,\dots,x_m, where x_j is the quantity of product j. So the price of a bundle x is p(x)=\sum_^m p_j\cdot x_j. A bundle is ''affordable'' for a buyer if the price of that bundle is at most the buyer's budget. I.e, a bundle x is affordable for buyer i if p(x)\leq B_i. Each buyer has a preference relation over bundles, which can be represented by a utility function. The utility function of buyer i is denoted by u_i. The ''demand set'' of a buyer is the se ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
|
|
Unit Demand
In economics, a unit demand agent is an agent who wants to buy a single item, which may be of one of different types. A typical example is a buyer who needs a new car. There are many different types of cars, but usually a buyer will choose only one of them, based on the quality and the price. If there are ''m'' different item-types, then a unit-demand valuation function is typically represented by ''m'' values v_1,\dots,v_m, with v_j representing the subjective value that the agent derives from item j. If the agent receives a set A of items, then his total utility is given by: :u(A)=\max_v_j since he enjoys the most valuable item from A and ignores the rest. Therefore, if the price of item j is p_j, then a unit-demand buyer will typically want to buy a single item – the item j for which the net utility v_j - p_j is maximized. Ordinal and cardinal definitions A unit-demand valuation is formally defined by: * For a preference relation: for every set B there is a subset A\subsete ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
|
|
Complementary Goods
In economics, a complementary good is a good whose appeal increases with the popularity of its complement. Technically, it displays a negative cross elasticity of demand and that demand for it increases when the price of another good decreases. If A is a complement to B, an increase in the price of A will result in a negative movement along the demand curve of A and cause the demand curve for B to shift inward; less of each good will be demanded. Conversely, a decrease in the price of A will result in a positive movement along the demand curve of A and cause the demand curve of B to shift outward; more of each good will be demanded. This is in contrast to a substitute good, whose demand decreases when its substitute's price decreases. When two goods are complements, they experience ''joint demand'' - the demand of one good is linked to the demand for another good. Therefore, if a higher quantity is demanded of one good, a higher quantity will also be demanded of the other, and '' ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
|
|
Substitute Good
In microeconomics, two goods are substitutes if the products could be used for the same purpose by the consumers. That is, a consumer perceives both goods as similar or comparable, so that having more of one good causes the consumer to desire less of the other good. Contrary to complementary goods and independent goods, substitute goods may replace each other in use due to changing economic conditions. An example of substitute goods is Coca-Cola and Pepsi; the interchangeable aspect of these goods is due to the similarity of the purpose they serve, i.e fulfilling customers' desire for a soft drink. These types of substitutes can be referred to as close substitutes. Definition Economic theory describes two goods as being close substitutes if three conditions hold: # products have the same or similar performance characteristics # products have the same or similar occasion for use and # products are sold in the same geographic area Performance characteristics describe what the pro ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
|
|
Independent Goods
Independent goods are goods that have a zero cross elasticity of demand. Changes in the price of one good will have no effect on the demand for an independent good. Thus independent goods are neither complements nor substitutes. For example, a person's demand for nails is usually independent of his or her demand for bread, since they are two unrelated types of goods. Note that this concept is subjective and depends on the consumer's personal utility function. A Cobb-Douglas utility function implies that goods are independent. For goods in quantities ''X''1 and ''X''2, prices ''p''1 and ''p''2, income ''m'', and utility function parameter ''a'', the utility function : u(X_1, X_2) = X_1^a X_2^, when optimized subject to the budget constraint that expenditure on the two goods cannot exceed income, gives rise to this demand function for good 1: X_1= am/p_1, which does not depend on ''p''2. See also * Consumer theory * Good (economics and accounting) In economics, goods are i ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
|
|
Examples
Example may refer to: * '' exempli gratia'' (e.g.), usually read out in English as "for example" * .example, reserved as a domain name that may not be installed as a top-level domain of the Internet ** example.com, example.net, example.org, example.edu, second-level domain names reserved for use in documentation as examples * HMS ''Example'' (P165), an Archer-class patrol and training vessel of the Royal Navy Arts * ''The Example'', a 1634 play by James Shirley * ''The Example'' (comics), a 2009 graphic novel by Tom Taylor and Colin Wilson * Example (musician), the British dance musician Elliot John Gleave (born 1982) * ''Example'' (album), a 1995 album by American rock band For Squirrels See also * * Exemplar (other), a prototype or model which others can use to understand a topic better * Exemplum, medieval collections of short stories to be told in sermons * Eixample The Eixample (; ) is a district of Barcelona between the old city (Ciutat Vella) and ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
|
|
Market Equilibrium Computation
Market equilibrium computation (also called competitive equilibrium computation or clearing-prices computation) is a computational problem in the intersection of economics and computer science. The input to this problem is a ''market'', consisting of a set of ''resources'' and a set of ''agents''. There are various kinds of markets, such as Fisher market and Arrow–Debreu market, with divisible or indivisible resources. The required output is a '' competitive equilibrium'', consisting of a ''price-vector'' (a price for each resource), and an ''allocation'' (a resource-bundle for each agent), such that each agent gets the best bundle possible (for him) given the budget, and the market ''clears'' (all resources are allocated). Market equilibrium computation is interesting due to the fact that a competitive equilibrium is always Pareto efficient. The special case of a Fisher market, in which all buyers have equal incomes, is particularly interesting, since in this setting a competit ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
|
|
Linear Utilities
In economics and consumer theory, a linear utility function is a function of the form: ::u(x_1,x_2,\dots,x_m) = w_1 x_1 + w_2 x_2 + \dots w_m x_m or, in vector form: ::u(\overrightarrow) = \overrightarrow \cdot \overrightarrow where: * m is the number of different goods in the economy. * \overrightarrow is a vector of size m that represents a bundle. The element x_i represents the amount of good i in the bundle. * \overrightarrow is a vector of size m that represents the subjective preferences of the consumer. The element w_i represents the relative value that the consumer assigns to good i. If w_i=0, this means that the consumer thinks that product i is totally worthless. The higher w_i is, the more valuable a unit of this product is for the consumer. A consumer with a linear utility function has the following properties: * The preferences are strictly monotone: having a larger quantity of even a single good strictly increases the utility. * The preferences are weakly convex, bu ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
|
|
Brouwer Fixed-point Theorem
Brouwer's fixed-point theorem is a fixed-point theorem in topology, named after L. E. J. (Bertus) Brouwer. It states that for any continuous function f mapping a compact convex set to itself there is a point x_0 such that f(x_0)=x_0. The simplest forms of Brouwer's theorem are for continuous functions f from a closed interval I in the real numbers to itself or from a closed disk D to itself. A more general form than the latter is for continuous functions from a convex compact subset K of Euclidean space to itself. Among hundreds of fixed-point theorems, Brouwer's is particularly well known, due in part to its use across numerous fields of mathematics. In its original field, this result is one of the key theorems characterizing the topology of Euclidean spaces, along with the Jordan curve theorem, the hairy ball theorem, the invariance of dimension and the Borsuk–Ulam theorem. This gives it a place among the fundamental theorems of topology. The theorem is also used for pr ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
|
|
Excess Demand Function
In microeconomics, excess demand is a phenomenon where the demand for goods and services exceeds that which the firms can produce. In microeconomics, an excess demand function is a function expressing excess demand for a product—the excess of quantity demanded over quantity supplied—in terms of the product's price and possibly other determinants. It is the product's demand function minus its supply function. In a pure exchange economy, the excess demand is the sum of all agents' demands minus the sum of all agents' initial endowments. A product's excess supply function is the negative of the excess demand function—it is the product's supply function minus its demand function. In most cases the first derivative of excess demand with respect to price is negative, meaning that a higher price leads to lower excess demand. The price of the product is said to be the equilibrium price if it is such that the value of the excess demand function is zero: that is, wh ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |