|
Uniform Convexity
In mathematics, uniformly convex spaces (or uniformly rotund spaces) are common examples of reflexive Banach spaces. The concept of uniform convexity was first introduced by James A. Clarkson in 1936. Definition A uniformly convex space is a normed vector space such that, for every 00 such that for any two vectors with \, x\, = 1 and \, y\, = 1, the condition :\, x-y\, \geq\varepsilon implies that: :\left\, \frac\right\, \leq 1-\delta. Intuitively, the center of a line segment inside the unit ball must lie deep inside the unit ball unless the segment is short. Properties * The unit sphere can be replaced with the closed unit ball in the definition. Namely, a normed vector space X is uniformly convex if and only if for every 00 so that, for any two vectors x and y in the closed unit ball (i.e. \, x\, \le 1 and \, y\, \le 1 ) with \, x-y\, \ge \varepsilon , one has \left\, \right\, \le 1-\delta (note that, given \varepsilon , the corresponding value of ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Mathematics
Mathematics is an area of knowledge that includes the topics of numbers, formulas and related structures, shapes and the spaces in which they are contained, and quantities and their changes. These topics are represented in modern mathematics with the major subdisciplines of number theory, algebra, geometry, and analysis, respectively. There is no general consensus among mathematicians about a common definition for their academic discipline. Most mathematical activity involves the discovery of properties of abstract objects and the use of pure reason to prove them. These objects consist of either abstractions from nature orin modern mathematicsentities that are stipulated to have certain properties, called axioms. A ''proof'' consists of a succession of applications of deductive rules to already established results. These results include previously proved theorems, axioms, andin case of abstraction from naturesome basic properties that are considered true starting points of ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Strictly Convex Space
In mathematics, a strictly convex space is a normed vector space (''X'', , , , , ) for which the closed unit ball is a strictly convex set. Put another way, a strictly convex space is one for which, given any two distinct points ''x'' and ''y'' on the unit sphere ∂''B'' (i.e. the boundary of the unit ball ''B'' of ''X''), the segment joining ''x'' and ''y'' meets ∂''B'' ''only'' at ''x'' and ''y''. Strict convexity is somewhere between an inner product space (all inner product spaces being strictly convex) and a general normed space in terms of structure. It also guarantees the uniqueness of a best approximation to an element in ''X'' (strictly convex) out of a convex subspace ''Y'', provided that such an approximation exists. If the normed space ''X'' is complete and satisfies the slightly stronger property of being uniformly convex (which implies strict convexity), then it is also reflexive by Milman-Pettis theorem. Properties The following properties are eq ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Joram Lindenstrauss
Joram Lindenstrauss ( he, יורם לינדנשטראוס) (October 28, 1936 – April 29, 2012) was an Israeli mathematician working in functional analysis. He was a professor of mathematics at the Einstein Institute of Mathematics. Biography Joram Lindenstrauss was born in Tel Aviv. He was the only child of a pair of lawyers who immigrated to Israel from Berlin. He began to study mathematics at the Hebrew University of Jerusalem in 1954 while serving in the army. He became a full-time student in 1956 and received his master's degree in 1959. In 1962 Lindenstrauss earned his Ph.D. from the Hebrew University (dissertation: ''Extension of Compact Operators'', advisors: Aryeh Dvoretzky, Branko Grünbaum). He worked as a postdoc at Yale University and the University of Washington in Seattle from 1962 - 1965. He was appointed senior lecturer at the Hebrew University in 1965, associate professor on 1967 and full professor in 1969. He became the Leon H. and Ada G. Miller Memorial ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Israel Journal Of Mathematics
'' Israel Journal of Mathematics'' is a peer-reviewed mathematics journal published by the Hebrew University of Jerusalem (Magnes Press). Founded in 1963, as a continuation of the ''Bulletin of the Research Council of Israel'' (Section F), the journal publishes articles on all areas of mathematics. The journal is indexed by ''Mathematical Reviews'' and Zentralblatt MATH. Its 2009 MCQ was 0.70, and its 2009 impact factor The impact factor (IF) or journal impact factor (JIF) of an academic journal is a scientometric index calculated by Clarivate that reflects the yearly mean number of citations of articles published in the last two years in a given journal, as i ... was 0.754. External links * Mathematics journals Publications established in 1963 English-language journals Bimonthly journals Hebrew University of Jerusalem {{math-journal-stub ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Uniformly Smooth Space
In mathematics, a uniformly smooth space is a normed vector space X satisfying the property that for every \epsilon>0 there exists \delta>0 such that if x,y\in X with \, x\, =1 and \, y\, \leq\delta then :\, x+y\, +\, x-y\, \le 2 + \epsilon\, y\, . The modulus of smoothness of a normed space ''X'' is the function ρ''X'' defined for every by the formula : \rho_X(t) = \sup \Bigl\. The triangle inequality yields that . The normed space ''X'' is uniformly smooth if and only if tends to 0 as ''t'' tends to 0. Properties * Every uniformly smooth Banach space is reflexive. * A Banach space X is uniformly smooth if and only if its continuous dual X^* is uniformly convex (and vice versa, via reflexivity). The moduli of convexity and smoothness are linked by ::\rho_(t) = \sup \, \quad t \ge 0, :and the maximal convex function majorated by the modulus of convexity δ''X'' is given by ::\tilde \delta_X(\varepsilon) = \sup \. :Furthermore, ::\delta_X(\varepsilon / 2) \ ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
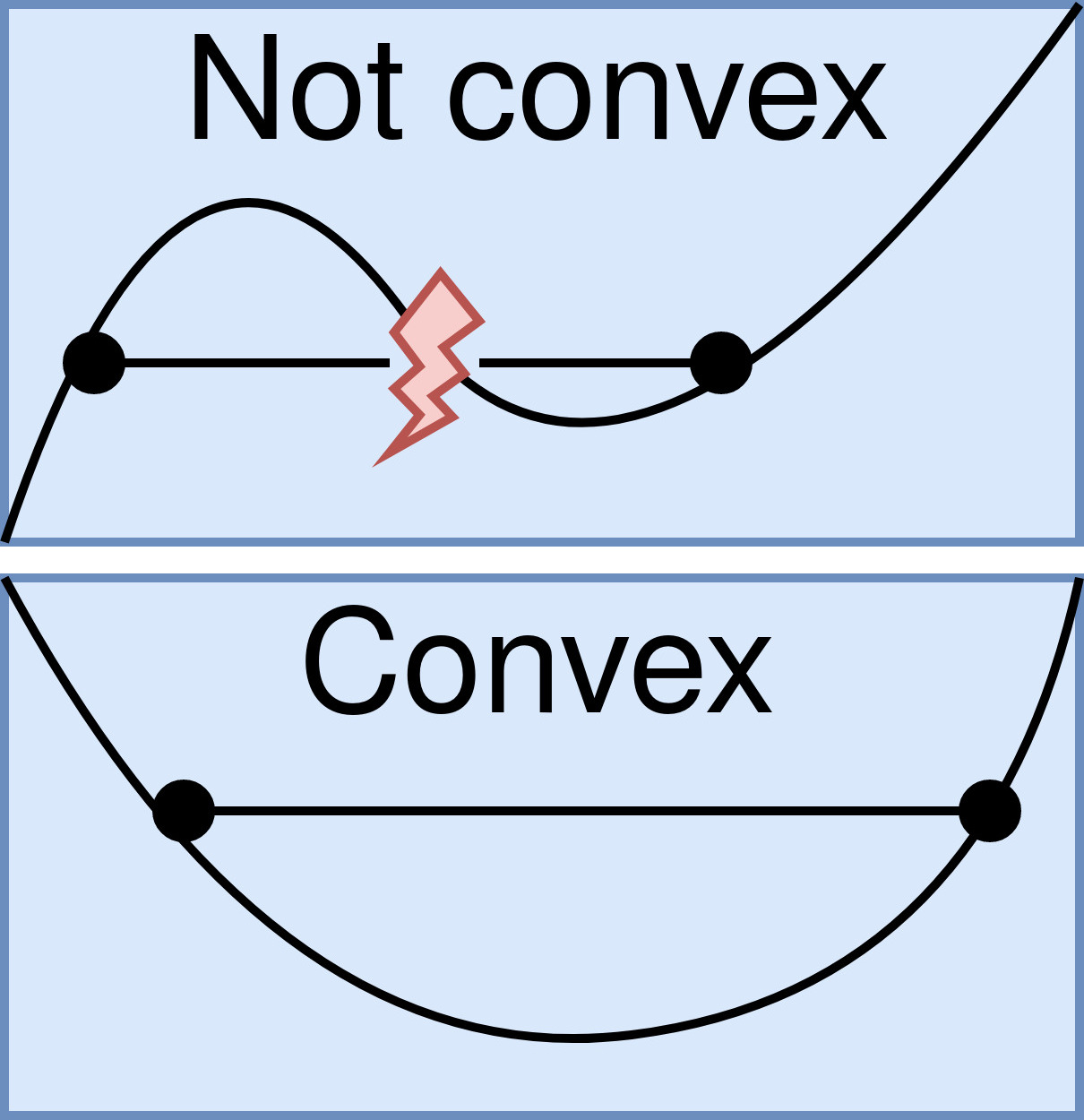
Convex Function
In mathematics, a real-valued function is called convex if the line segment between any two points on the graph of a function, graph of the function lies above the graph between the two points. Equivalently, a function is convex if its epigraph (mathematics), epigraph (the set of points on or above the graph of the function) is a convex set. A twice-differentiable function of a single variable is convex if and only if its second derivative is nonnegative on its entire domain. Well-known examples of convex functions of a single variable include the quadratic function x^2 and the exponential function e^x. In simple terms, a convex function refers to a function whose graph is shaped like a cup \cup, while a concave function's graph is shaped like a cap \cap. Convex functions play an important role in many areas of mathematics. They are especially important in the study of optimization problems where they are distinguished by a number of convenient properties. For instance, a st ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Modulus And Characteristic Of Convexity
In mathematics, the modulus of convexity and the characteristic of convexity are measures of "how convex" the unit ball in a Banach space is. In some sense, the modulus of convexity has the same relationship to the ''ε''-''δ'' definition of uniform convexity as the modulus of continuity does to the ''ε''-''δ'' definition of continuity. Definitions The modulus of convexity of a Banach space (''X'', , , ·, , ) is the function defined by :\delta (\varepsilon) = \inf \left\, where ''S'' denotes the unit sphere of (''X'', , , , , ). In the definition of ''δ''(''ε''), one can as well take the infimum over all vectors ''x'', ''y'' in ''X'' such that and . The characteristic of convexity of the space (''X'', , , , , ) is the number ''ε''0 defined by :\varepsilon_ = \sup \. These notions are implicit in the general study of uniform convexity by J. A. Clarkson (; this is the same paper containing the statements of Clark ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Lp Space
In mathematics, the spaces are function spaces defined using a natural generalization of the Norm (mathematics)#p-norm, -norm for finite-dimensional vector spaces. They are sometimes called Lebesgue spaces, named after Henri Lebesgue , although according to the Nicolas Bourbaki, Bourbaki group they were first introduced by Frigyes Riesz . spaces form an important class of Banach spaces in functional analysis, and of topological vector spaces. Because of their key role in the mathematical analysis of measure and probability spaces, Lebesgue spaces are used also in the theoretical discussion of problems in physics, statistics, economics, finance, engineering, and other disciplines. Applications Statistics In statistics, measures of central tendency and statistical dispersion, such as the mean, median, and standard deviation, are defined in terms of metrics, and measures of central tendency can be characterized as Central tendency#Solutions to variational problems, solutions to ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Hanner's Inequalities
In mathematics, Hanner's inequalities are results in the theory of ''L''''p'' spaces. Their proof was published in 1956 by Olof Hanner. They provide a simpler way of proving the uniform convexity of ''L''''p'' spaces for ''p'' ∈ (1, +∞) than the approach proposed by James A. Clarkson James Andrew Clarkson (7 February 1906 – 6 June 1970) was an American mathematician and professor of mathematics who specialized in number theory. He is known for proving inequalities in Hölder condition#Hölder spaces, Hölder spaces, and deri ... in 1936. Statement of the inequalities Let ''f'', ''g'' ∈ ''L''''p''(''E''), where ''E'' is any measure space. If ''p'' ∈ , 2 then :\, f+g\, _p^p + \, f-g\, _p^p \geq \big( \, f\, _p + \, g\, _p \big)^p + \big, \, f\, _p-\, g\, _p \big, ^p. The substitutions ''F'' = ''f'' + ''g'' and ''G'' = ''f'' − ''g'' yield the second of Hanner's inequalities: ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Triangle Inequality
In mathematics, the triangle inequality states that for any triangle, the sum of the lengths of any two sides must be greater than or equal to the length of the remaining side. This statement permits the inclusion of degenerate triangles, but some authors, especially those writing about elementary geometry, will exclude this possibility, thus leaving out the possibility of equality. If , , and are the lengths of the sides of the triangle, with no side being greater than , then the triangle inequality states that :z \leq x + y , with equality only in the degenerate case of a triangle with zero area. In Euclidean geometry and some other geometries, the triangle inequality is a theorem about distances, and it is written using vectors and vector lengths ( norms): :\, \mathbf x + \mathbf y\, \leq \, \mathbf x\, + \, \mathbf y\, , where the length of the third side has been replaced by the vector sum . When and are real numbers, they can be viewed as vectors in , and the trian ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Uniformly Smooth Space
In mathematics, a uniformly smooth space is a normed vector space X satisfying the property that for every \epsilon>0 there exists \delta>0 such that if x,y\in X with \, x\, =1 and \, y\, \leq\delta then :\, x+y\, +\, x-y\, \le 2 + \epsilon\, y\, . The modulus of smoothness of a normed space ''X'' is the function ρ''X'' defined for every by the formula : \rho_X(t) = \sup \Bigl\. The triangle inequality yields that . The normed space ''X'' is uniformly smooth if and only if tends to 0 as ''t'' tends to 0. Properties * Every uniformly smooth Banach space is reflexive. * A Banach space X is uniformly smooth if and only if its continuous dual X^* is uniformly convex (and vice versa, via reflexivity). The moduli of convexity and smoothness are linked by ::\rho_(t) = \sup \, \quad t \ge 0, :and the maximal convex function majorated by the modulus of convexity δ''X'' is given by ::\tilde \delta_X(\varepsilon) = \sup \. :Furthermore, ::\delta_X(\varepsilon / 2) \ ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Reflexive Space
In the area of mathematics known as functional analysis, a reflexive space is a locally convex topological vector space (TVS) for which the canonical evaluation map from X into its bidual (which is the strong dual of the strong dual of X) is an isomorphism of TVSs. Since a normable TVS is reflexive if and only if it is semi-reflexive, every normed space (and so in particular, every Banach space) X is reflexive if and only if the canonical evaluation map from X into its bidual is surjective; in this case the normed space is necessarily also a Banach space. In 1951, R. C. James discovered a Banach space, now known as James' space, that is reflexive but is nevertheless isometrically isomorphic to its bidual (any such isomorphism is thus necessarily the canonical evaluation map). Reflexive spaces play an important role in the general theory of locally convex TVSs and in the theory of Banach spaces in particular. Hilbert spaces are prominent examples of reflexive Banach spaces. ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
