|
Trihexagonal Tiling
In geometry, the trihexagonal tiling is one of 11 uniform tilings of the Euclidean plane by regular polygons. See in particular Theorem 2.1.3, p. 59 (classification of uniform tilings); Figure 2.1.5, p.63 (illustration of this tiling), Theorem 2.9.1, p. 103 (classification of colored tilings), Figure 2.9.2, p. 105 (illustration of colored tilings), Figure 2.5.3(d), p. 83 (topologically equivalent star tiling), and Exercise 4.1.3, p. 171 (topological equivalence of trihexagonal and two-triangle tilings). It consists of equilateral triangles and regular hexagons, arranged so that each hexagon is surrounded by triangles and vice versa. The name derives from the fact that it combines a regular hexagonal tiling and a regular triangular tiling. Two hexagons and two triangles alternate around each vertex, and its edges form an infinite arrangement of lines. Its dual is the rhombille tiling. This pattern, and its place in the classification of uniform tilings, was already known to Joh ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Geometry
Geometry (; ) is, with arithmetic, one of the oldest branches of mathematics. It is concerned with properties of space such as the distance, shape, size, and relative position of figures. A mathematician who works in the field of geometry is called a '' geometer''. Until the 19th century, geometry was almost exclusively devoted to Euclidean geometry, which includes the notions of point, line, plane, distance, angle, surface, and curve, as fundamental concepts. During the 19th century several discoveries enlarged dramatically the scope of geometry. One of the oldest such discoveries is Carl Friedrich Gauss' ("remarkable theorem") that asserts roughly that the Gaussian curvature of a surface is independent from any specific embedding in a Euclidean space. This implies that surfaces can be studied ''intrinsically'', that is, as stand-alone spaces, and has been expanded into the theory of manifolds and Riemannian geometry. Later in the 19th century, it appeared that geom ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Mineral
In geology and mineralogy, a mineral or mineral species is, broadly speaking, a solid chemical compound with a fairly well-defined chemical composition and a specific crystal structure that occurs naturally in pure form.John P. Rafferty, ed. (2011): Minerals'; p. 1. In the series ''Geology: Landforms, Minerals, and Rocks''. Rosen Publishing Group. The geological definition of mineral normally excludes compounds that occur only in living organisms. However, some minerals are often biogenic (such as calcite) or are organic compounds in the sense of chemistry (such as mellite). Moreover, living organisms often synthesize inorganic minerals (such as hydroxylapatite) that also occur in rocks. The concept of mineral is distinct from rock, which is any bulk solid geologic material that is relatively homogeneous at a large enough scale. A rock may consist of one type of mineral, or may be an aggregate of two or more different types of minerals, spacially segregated into disti ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
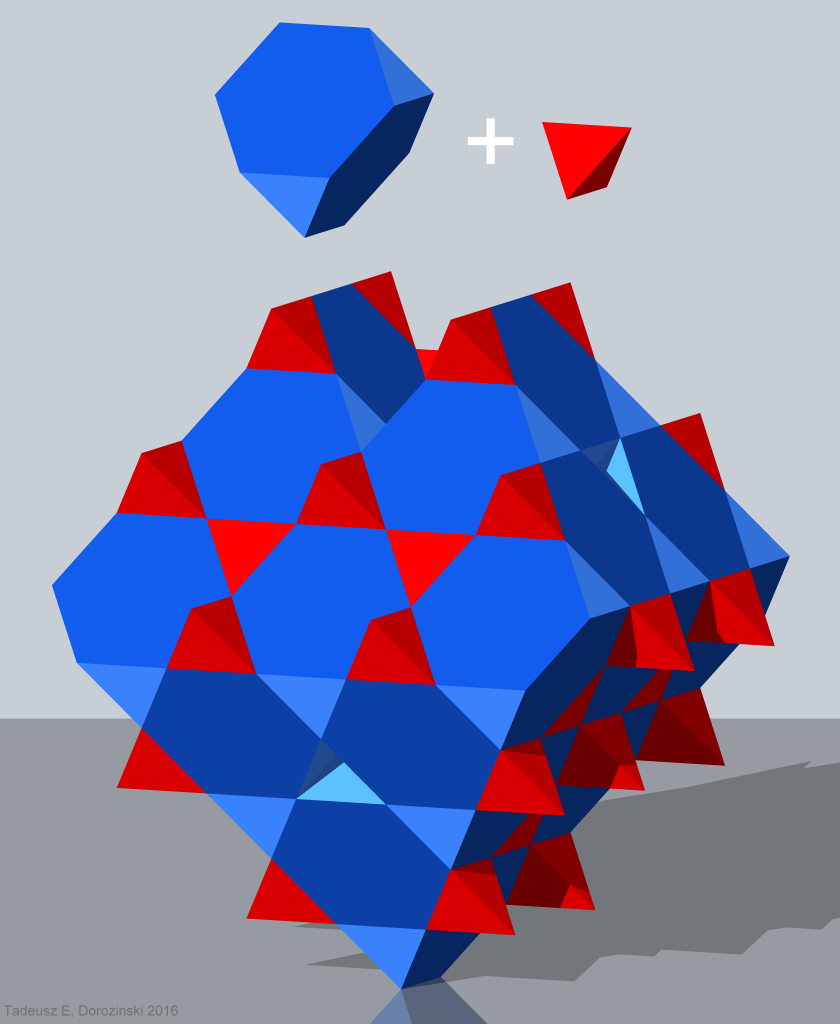
Trihexagonal Prismatic Honeycomb
The triangular prismatic honeycomb or triangular prismatic cellulation is a space-filling tessellation (or honeycomb) in Euclidean 3-space. It is composed entirely of triangular prisms. It is constructed from a triangular tiling extruded into prisms. It is one of 28 convex uniform honeycombs. It consists of 1 + 6 + 1 = 8 edges meeting at a vertex, There are 6 triangular prism cells meeting at an edge and faces are shared between 2 cells. Related honeycombs Hexagonal prismatic honeycomb The hexagonal prismatic honeycomb or hexagonal prismatic cellulation is a space-filling tessellation (or honeycomb) in Euclidean 3-space made up of hexagonal prisms. It is constructed from a hexagonal tiling extruded into prisms. It is one of 28 convex uniform honeycombs. This honeycomb can be alternated into the gyrated tetrahedral-octahedral honeycomb, with pairs of tetrahedra existing in the alternated gaps (instead of a triangular bipyramid). There are 1 + 3 + 1 = 5 edges meeting ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Truncated Tetrahedra
In geometry, the truncated tetrahedron is an Archimedean solid. It has 4 regular hexagonal faces, 4 equilateral triangle faces, 12 vertices and 18 edges (of two types). It can be constructed by truncating all 4 vertices of a regular tetrahedron at one third of the original edge length. A deeper truncation, removing a tetrahedron of half the original edge length from each vertex, is called rectification. The rectification of a tetrahedron produces an octahedron. A ''truncated tetrahedron'' is the Goldberg polyhedron containing triangular and hexagonal faces. A ''truncated tetrahedron'' can be called a cantic cube, with Coxeter diagram, , having half of the vertices of the cantellated cube (rhombicuboctahedron), . There are two dual positions of this construction, and combining them creates the uniform compound of two truncated tetrahedra. Area and volume The area ''A'' and the volume ''V'' of a truncated tetrahedron of edge length ''a'' are: :\begin A &= 7\sqrta^2 &&\approx 1 ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Tetrahedra
In geometry, a tetrahedron (plural: tetrahedra or tetrahedrons), also known as a triangular pyramid, is a polyhedron composed of four triangular faces, six straight edges, and four vertex corners. The tetrahedron is the simplest of all the ordinary convex polyhedra and the only one that has fewer than 5 faces. The tetrahedron is the three-dimensional case of the more general concept of a Euclidean simplex, and may thus also be called a 3-simplex. The tetrahedron is one kind of pyramid, which is a polyhedron with a flat polygon base and triangular faces connecting the base to a common point. In the case of a tetrahedron the base is a triangle (any of the four faces can be considered the base), so a tetrahedron is also known as a "triangular pyramid". Like all convex polyhedra, a tetrahedron can be folded from a single sheet of paper. It has two such nets. For any tetrahedron there exists a sphere (called the circumsphere) on which all four vertices lie, and another ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Quarter Cubic Honeycomb
The quarter cubic honeycomb, quarter cubic cellulation or bitruncated alternated cubic honeycomb is a space-filling tessellation (or honeycomb) in Euclidean 3-space. It is composed of tetrahedra and truncated tetrahedra in a ratio of 1:1. It is called "quarter-cubic" because its symmetry unit – the minimal block from which the pattern is developed by reflections – is four times that of the cubic honeycomb. It is vertex-transitive with 6 truncated tetrahedra and 2 tetrahedra around each vertex. It is one of the 28 convex uniform honeycombs. The faces of this honeycomb's cells form four families of parallel planes, each with a 3.6.3.6 tiling. Its vertex figure is an isosceles antiprism: two equilateral triangles joined by six isosceles triangles. John Horton Conway calls this honeycomb a truncated tetrahedrille, and its dual oblate cubille. The vertices and edges represent a Kagome lattice in three dimensions, which is the pyrochlore lattice. Construction The quart ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Lattice (group)
In geometry and group theory, a lattice in the real coordinate space \mathbb^n is an infinite set of points in this space with the properties that coordinate wise addition or subtraction of two points in the lattice produces another lattice point, that the lattice points are all separated by some minimum distance, and that every point in the space is within some maximum distance of a lattice point. Closure under addition and subtraction means that a lattice must be a subgroup of the additive group of the points in the space, and the requirements of minimum and maximum distance can be summarized by saying that a lattice is a Delone set. More abstractly, a lattice can be described as a free abelian group of dimension n which spans the vector space \mathbb^n. For any basis of \mathbb^n, the subgroup of all linear combinations with integer coefficients of the basis vectors forms a lattice, and every lattice can be formed from a basis in this way. A lattice may be viewed as a r ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Kôdi Husimi
Kōji Husimi (June 29, 1909 – May 8, 2008, ja, 伏見康治) was a Japanese theoretical physicist who served as the president of the Science Council of Japan.. Husimi trees in graph theory, the Husimi Q representation in quantum mechanics, and Husimi's theorem in the mathematics of paper folding are named after him. Education and career Husimi studied at the University of Tokyo, graduating in 1933. He spent a year there as an assistant, and then moved to Osaka University in 1934, where he soon began working with Seishi Kikuchi. At Osaka, he became Dean of the Faculty of Science. He moved to Nagoya University in 1961, and directed the plasma institute there. He retired in 1973, and became a professor emeritus of both Nagoya and Osaka. Contributions Physics A 1940 paper by Husimi introduced the Husimi Q representation in quantum mechanics. Husimi also gave the name to the kagome lattice, frequently used in statistical mechanics. Graph theory In the mathematical area ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Wallpaper Group
A wallpaper is a mathematical object covering a whole Euclidean plane by repeating a motif indefinitely, in manner that certain isometries keep the drawing unchanged. To a given wallpaper there corresponds a group of such congruent transformations, with function composition as the group operation. Thus, a wallpaper group (or plane symmetry group or plane crystallographic group) is in a mathematical classification of a two‑dimensional repetitive pattern, based on the symmetries in the pattern. Such patterns occur frequently in architecture and decorative art, especially in textiles, tessellations and tiles as well as wallpaper. What this page calls pattern Any periodic tiling can be seen as a wallpaper. More particularly, we can consider as a wallpaper a tiling by identical tiles edge‑to‑edge, necessarily periodic, and conceive from it a wallpaper by decorating in the same manner every tiling element, and eventually erase partly or entirely t ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Wallpaper Group
A wallpaper is a mathematical object covering a whole Euclidean plane by repeating a motif indefinitely, in manner that certain isometries keep the drawing unchanged. To a given wallpaper there corresponds a group of such congruent transformations, with function composition as the group operation. Thus, a wallpaper group (or plane symmetry group or plane crystallographic group) is in a mathematical classification of a two‑dimensional repetitive pattern, based on the symmetries in the pattern. Such patterns occur frequently in architecture and decorative art, especially in textiles, tessellations and tiles as well as wallpaper. What this page calls pattern Any periodic tiling can be seen as a wallpaper. More particularly, we can consider as a wallpaper a tiling by identical tiles edge‑to‑edge, necessarily periodic, and conceive from it a wallpaper by decorating in the same manner every tiling element, and eventually erase partly or entirely t ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Lath
A lath or slat is a thin, narrow strip of straight-grained wood used under roof shingles or tiles, on lath and plaster walls and ceilings to hold plaster, and in lattice and trellis work. ''Lath'' has expanded to mean any type of backing material for plaster. This includes metal wire mesh or expanded metal that is applied to a wood or metal framework as matrix over which stucco or plaster is applied, as well as wallboard products called gypsum or rock lath.Ching, Frank. ''A visual dictionary of architecture''. New York: Van Nostrand Reinhold, 1995. 198. Print. Historically, reed mat was also used as a lath material. One of the key elements of lath, whether wooden slats or wire mesh, are the openings or gaps that allow plaster or stucco to ooze behind and form a mechanical bond to the lath. This is not necessary for gypsum lath, which relies on a chemical bond. Etymology The word is recorded from the late 13th century and is likely derived from the Old English word *, ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |


.jpg)