|
Signal Averaging
Signal averaging is a signal processing technique applied in the time domain, intended to increase the strength of a signal relative to noise that is obscuring it. By averaging a set of replicate measurements, the signal-to-noise ratio (SNR) will be increased, ideally in proportion to the square root of the number of measurements. Deriving the SNR for averaged signals Assumed that * Signal s(t) is uncorrelated to noise, and noise z(t) is uncorrelated : E (t)z(t-\tau)= 0 = E (t)s(t-\tau)forall t,\tau. * Signal power P_=E ^2/math> is constant in the replicate measurements. * Noise is random, with a mean of zero and constant variance in the replicate measurements: E = 0 = \mu and 0 < E left(z-\mu\right)^2= E ^2= P_ = \sigma^2. * We (canonically) define Signal-to-Noise ratio as [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Signal Processing
Signal processing is an electrical engineering subfield that focuses on analyzing, modifying and synthesizing ''signals'', such as audio signal processing, sound, image processing, images, and scientific measurements. Signal processing techniques are used to optimize transmissions, Data storage, digital storage efficiency, correcting distorted signals, subjective video quality and to also detect or pinpoint components of interest in a measured signal. History According to Alan V. Oppenheim and Ronald W. Schafer, the principles of signal processing can be found in the classical numerical analysis techniques of the 17th century. They further state that the digital refinement of these techniques can be found in the digital control systems of the 1940s and 1950s. In 1948, Claude Shannon wrote the influential paper "A Mathematical Theory of Communication" which was published in the Bell System Technical Journal. The paper laid the groundwork for later development of information c ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Time Domain
Time domain refers to the analysis of mathematical functions, physical signals or time series of economic or environmental data, with respect to time. In the time domain, the signal or function's value is known for all real numbers, for the case of continuous time, or at various separate instants in the case of discrete time. An oscilloscope is a tool commonly used to visualize real-world signals in the time domain. A time-domain graph shows how a signal changes with time, whereas a frequency-domain graph shows how much of the signal lies within each given frequency band over a range of frequencies. Though most precisely referring to time in physics, the term ''time domain'' may occasionally informally refer to position in space when dealing with spatial frequencies, as a substitute for the more precise term ''spatial domain''. Origin of term The use of the contrasting terms ''time domain'' and ''frequency domain'' developed in U.S. communication engineering in the late 194 ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
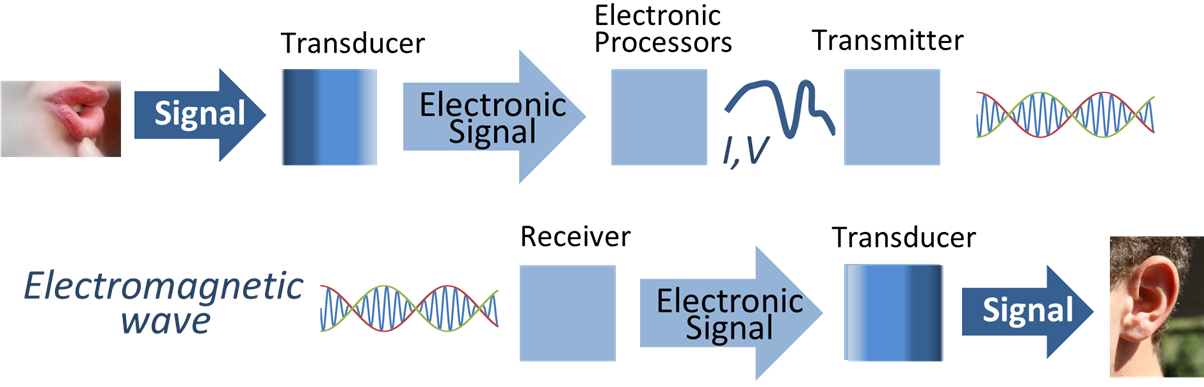
Signal (electronics)
In signal processing, a signal is a function that conveys information about a phenomenon. Any quantity that can vary over space or time can be used as a signal to share messages between observers. The ''IEEE Transactions on Signal Processing'' includes audio, video, speech, image, sonar, and radar as examples of signal. A signal may also be defined as observable change in a quantity over space or time (a time series), even if it does not carry information. In nature, signals can be actions done by an organism to alert other organisms, ranging from the release of plant chemicals to warn nearby plants of a predator, to sounds or motions made by animals to alert other animals of food. Signaling occurs in all organisms even at cellular levels, with cell signaling. Signaling theory, in evolutionary biology, proposes that a substantial driver for evolution is the ability of animals to communicate with each other by developing ways of signaling. In human engineering, signals are typi ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Noise
Noise is unwanted sound considered unpleasant, loud or disruptive to hearing. From a physics standpoint, there is no distinction between noise and desired sound, as both are vibrations through a medium, such as air or water. The difference arises when the brain receives and perceives a sound. Acoustic noise is any sound in the acoustic domain, either deliberate (e.g., music or speech) or unintended. In contrast, Noise (electronics), noise in electronics may not be audible to the human ear and may require instruments for detection. In audio engineering, noise can refer to the unwanted residual electronic noise signal that gives rise to acoustic noise heard as a Hiss (electromagnetic), hiss. This signal noise is commonly measured using A-weighting or ITU-R 468 noise weighting, ITU-R 468 weighting. In experimental sciences, noise can refer to any random fluctuations of data that hinders perception of a signal. Measurement Sound is measured based on the amplitude and frequency ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Replication (statistics)
In engineering, science, and statistics, replication is the repetition of an experimental condition so that the variability associated with the phenomenon can be estimated. ASTM, in standard E1847, defines replication as "... the repetition of the set of all the treatment combinations to be compared in an experiment. Each of the repetitions is called a ''replicate''." Replication is not the same as repeated measurements of the same item: they are dealt with differently in statistical experimental design and data analysis. For proper sampling, a process or batch of products should be in reasonable statistical control; inherent random variation is present but variation due to assignable (special) causes is not. Evaluation or testing of a single item does not allow for item-to-item variation and may not represent the batch or process. Replication is needed to account for this variation among items and treatments. Example As an example, consider a continuous process which produces ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Signal-to-noise Ratio
Signal-to-noise ratio (SNR or S/N) is a measure used in science and engineering that compares the level of a desired signal to the level of background noise. SNR is defined as the ratio of signal power to the noise power, often expressed in decibels. A ratio higher than 1:1 (greater than 0 dB) indicates more signal than noise. SNR, bandwidth, and channel capacity of a communication channel are connected by the Shannon–Hartley theorem. Definition Signal-to-noise ratio is defined as the ratio of the power of a signal (meaningful input) to the power of background noise (meaningless or unwanted input): : \mathrm = \frac, where is average power. Both signal and noise power must be measured at the same or equivalent points in a system, and within the same system bandwidth. Depending on whether the signal is a constant () or a random variable (), the signal-to-noise ratio for random noise becomes: : \mathrm = \frac where E refers to the expected value, i.e. in this case ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Mean
There are several kinds of mean in mathematics, especially in statistics. Each mean serves to summarize a given group of data, often to better understand the overall value (magnitude and sign) of a given data set. For a data set, the ''arithmetic mean'', also known as "arithmetic average", is a measure of central tendency of a finite set of numbers: specifically, the sum of the values divided by the number of values. The arithmetic mean of a set of numbers ''x''1, ''x''2, ..., x''n'' is typically denoted using an overhead bar, \bar. If the data set were based on a series of observations obtained by sampling from a statistical population, the arithmetic mean is the ''sample mean'' (\bar) to distinguish it from the mean, or expected value, of the underlying distribution, the ''population mean'' (denoted \mu or \mu_x).Underhill, L.G.; Bradfield d. (1998) ''Introstat'', Juta and Company Ltd.p. 181/ref> Outside probability and statistics, a wide range of other notions of mean are o ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Variance
In probability theory and statistics, variance is the expectation of the squared deviation of a random variable from its population mean or sample mean. Variance is a measure of dispersion, meaning it is a measure of how far a set of numbers is spread out from their average value. Variance has a central role in statistics, where some ideas that use it include descriptive statistics, statistical inference, hypothesis testing, goodness of fit, and Monte Carlo sampling. Variance is an important tool in the sciences, where statistical analysis of data is common. The variance is the square of the standard deviation, the second central moment of a distribution, and the covariance of the random variable with itself, and it is often represented by \sigma^2, s^2, \operatorname(X), V(X), or \mathbb(X). An advantage of variance as a measure of dispersion is that it is more amenable to algebraic manipulation than other measures of dispersion such as the expected absolute deviation; for e ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Oversampling
In signal processing, oversampling is the process of sampling a signal at a sampling frequency significantly higher than the Nyquist rate. Theoretically, a bandwidth-limited signal can be perfectly reconstructed if sampled at the Nyquist rate or above it. The Nyquist rate is defined as twice the bandwidth of the signal. Oversampling is capable of improving resolution and signal-to-noise ratio, and can be helpful in avoiding aliasing and phase distortion by relaxing anti-aliasing filter performance requirements. A signal is said to be oversampled by a factor of ''N'' if it is sampled at ''N'' times the Nyquist rate. Motivation There are three main reasons for performing oversampling: to improve anti-aliasing performance, to increase resolution and to reduce noise. Anti-aliasing Oversampling can make it easier to realize analog anti-aliasing filters. Without oversampling, it is very difficult to implement filters with the sharp cutoff necessary to maximize use of the available b ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Digital Signal Processing
Digital signal processing (DSP) is the use of digital processing, such as by computers or more specialized digital signal processors, to perform a wide variety of signal processing operations. The digital signals processed in this manner are a sequence of numbers that represent samples of a continuous variable in a domain such as time, space, or frequency. In digital electronics, a digital signal is represented as a pulse train, which is typically generated by the switching of a transistor. Digital signal processing and analog signal processing are subfields of signal processing. DSP applications include audio and speech processing, sonar, radar and other sensor array processing, spectral density estimation, statistical signal processing, digital image processing, data compression, video coding, audio coding, image compression, signal processing for telecommunications, control systems, biomedical engineering, and seismology, among others. DSP can involve linear or nonli ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
.gif)


