|
Source Set
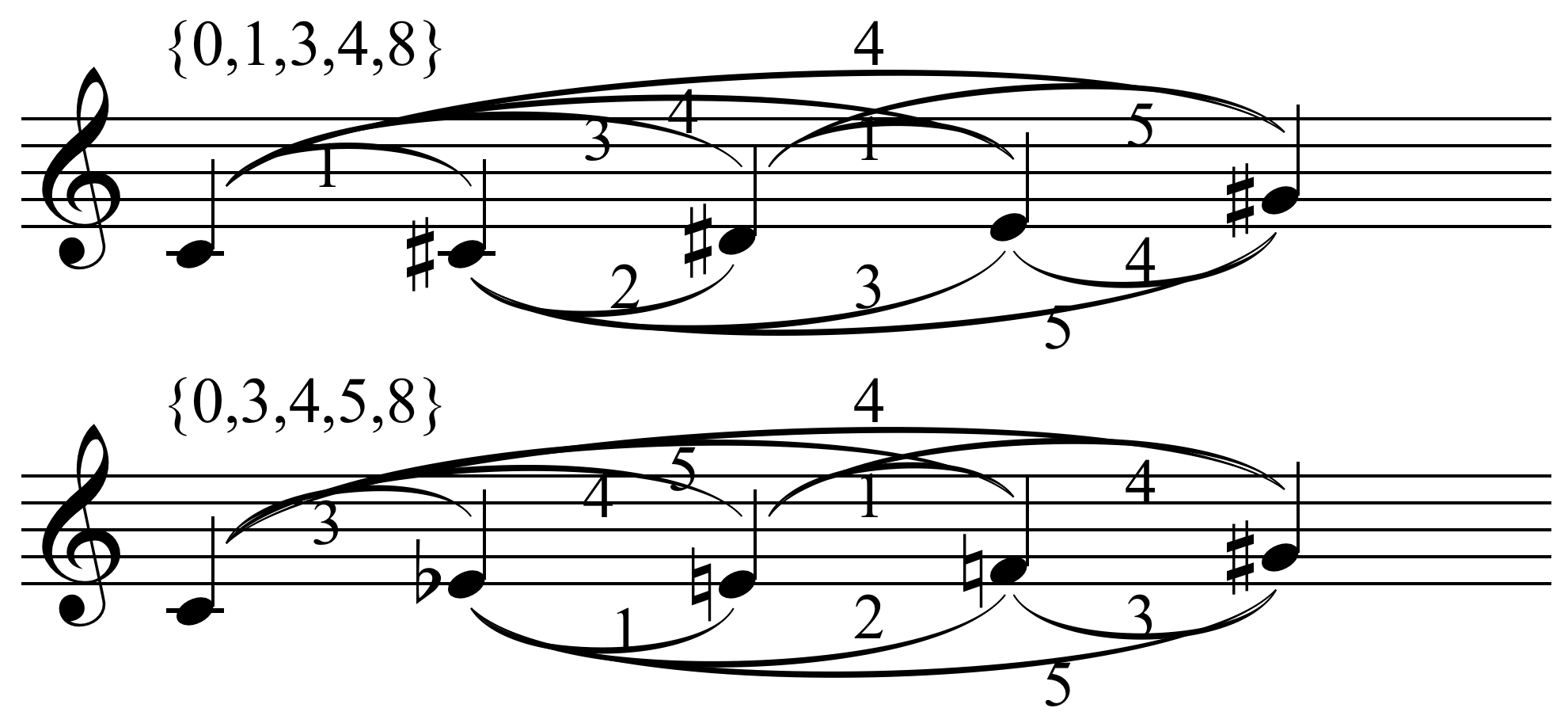
In music using the twelve tone technique, combinatoriality is a quality shared by twelve-tone tone rows whereby each section of a row and a proportionate number of its transformations combine to form aggregates (all twelve tones). Whittall, Arnold. 2008. ''The Cambridge Introduction to Serialism. Cambridge Introductions to Music'', p. 272. New York: Cambridge University Press. (hardback) (pbk). Much as the pitches of an aggregate created by a tone row do not need to occur simultaneously, the pitches of a combinatorially created aggregate need not occur simultaneously. Arnold Schoenberg, creator of the twelve-tone technique, often combined P-0/I-5 to create "two aggregates, between the first hexachords of each, and the second hexachords of each, respectively." Combinatoriality is a side effect of derived rows, where the initial segment or set may be combined with its transformations (T,R,I,RI) to create an entire row. "Derivation refers to a process whereby, for instance, the ini ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Music
Music is generally defined as the art of arranging sound to create some combination of form, harmony, melody, rhythm or otherwise expressive content. Exact definitions of music vary considerably around the world, though it is an aspect of all human societies, a cultural universal. While scholars agree that music is defined by a few specific elements, there is no consensus on their precise definitions. The creation of music is commonly divided into musical composition, musical improvisation, and musical performance, though the topic itself extends into academic disciplines, criticism, philosophy, and psychology. Music may be performed or improvised using a vast range of instruments, including the human voice. In some musical contexts, a performance or composition may be to some extent improvised. For instance, in Hindustani classical music, the performer plays spontaneously while following a partially defined structure and using characteristic motifs. In modal jazz ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Composition For Twelve Instruments
''Composition for Twelve Instruments'' (1948, rev. 1954) is a Serialism, serial music composition written by American composer Milton Babbitt for flute, oboe, clarinet, bassoon, horn, trumpet, harp, celesta, violin, viola, cello, and double bass. In it Babbitt for the first time employs a twelve-element duration series, duration set to serialize the rhythms as well as the pitches, predating Olivier Messiaen's (non-serial) "Quatre études de rythme#"Mode de valeurs et d'intensités", Mode de valeurs et d'intensités", but not the ''Turangalîla-Symphonie'' (1946–48), in which Messiaen used a duration series for the first time in the opening episode of the seventh movement, titled "Turangalîla II". (Babbitt had also earlier used a different kind of rhythmic series, and serial manipulation thereof, in his ''Three Compositions for Piano'' (1947) and ''Composition for Four Instruments'' (1948)). Babbitt's use of rhythm in ''Composition for Twelve Instruments'' was criticized by Peter ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Interval Vector
In musical set theory, an interval vector is an array of natural numbers which summarize the intervals present in a set of pitch classes. (That is, a set of pitches where octaves are disregarded.) Other names include: ic vector (or interval-class vector), PIC vector (or pitch-class interval vector) and APIC vector (or absolute pitch-class interval vector, which Michiel Schuijer states is more proper.) While primarily an analytic tool, interval vectors can also be useful for composers, as they quickly show the sound qualities that are created by different collections of pitch class. That is, sets with high concentrations of conventionally dissonant intervals (i.e., seconds and sevenths) sound more dissonant, while sets with higher numbers of conventionally consonant intervals (i.e., thirds and sixths) sound more consonant. While the actual perception of consonance and dissonance involves many contextual factors, such as register, an interval vector can nevertheless be a help ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Complement (set Theory)
In set theory, the complement of a set , often denoted by (or ), is the set of elements not in . When all sets in the universe, i.e. all sets under consideration, are considered to be members of a given set , the absolute complement of is the set of elements in that are not in . The relative complement of with respect to a set , also termed the set difference of and , written B \setminus A, is the set of elements in that are not in . Absolute complement Definition If is a set, then the absolute complement of (or simply the complement of ) is the set of elements not in (within a larger set that is implicitly defined). In other words, let be a set that contains all the elements under study; if there is no need to mention , either because it has been previously specified, or it is obvious and unique, then the absolute complement of is the relative complement of in : A^\complement = U \setminus A. Or formally: A^\complement = \. The absolute complement of is u ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Retrograde Inversion
Retrograde inversion is a musical term that literally means "backwards and upside down": "The inverse of the series is sounded in reverse order." Retrograde reverses the order of the motif's pitches: what was the first pitch becomes the last, and vice versa. This is a technique used in music, specifically in twelve-tone technique, where the inversion and retrograde techniques are performed on the same tone row successively, " e inversion of the prime series in reverse order from last pitch to first." Conventionally, inversion is carried out first, and the inverted form is then taken backward to form the retrograde inversion, so that the untransposed retrograde inversion ends with the pitch that began the prime form of the series. In his late twelve-tone works, however, Igor Stravinsky preferred the opposite order, so that his row charts use inverse retrograde (IR) forms for his source sets, instead of retrograde inversions (RI), although he sometimes labeled them RI in his sket ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Retrograde (music)
A melodic line that is the reverse of a previously or simultaneously stated line is said to be its retrograde or cancrizans ("walking backward", medieval Latin, from ''cancer'', crab). An exact retrograde includes both the pitches and rhythms in reverse. An even more exact retrograde reverses the physical contour of the notes themselves, though this is possible only in electronic music. Some composers choose to subject just the pitches of a musical line to retrograde, or just the rhythms. In twelve-tone music, reversal of the pitch classes alone—regardless of the melodic contour created by their registral placement—is regarded as a retrograde. In modal and tonal music In treatises Retrograde was not mentioned in theoretical treatises prior to 1500.Newes, p. 218. Nicola Vicentino (1555) discussed the difficulty in finding canonic imitation: "At times, the fugue or canon cannot be discovered through the systems mentioned above, either because of the impediment of rests ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Transposition (music)
In music, transposition refers to the process or operation of moving a collection of notes ( pitches or pitch classes) up or down in pitch by a constant interval. For example, one might transpose an entire piece of music into another key. Similarly, one might transpose a tone row or an unordered collection of pitches such as a chord so that it begins on another pitch. The transposition of a set ''A'' by ''n'' semitones is designated by ''T''''n''(''A''), representing the addition ( mod 12) of an integer ''n'' to each of the pitch class integers of the set ''A''. Thus the set (''A'') consisting of 0–1–2 transposed by 5 semitones is 5–6–7 (''T''5(''A'')) since , , and . Scalar transpositions In scalar transposition, every pitch in a collection is shifted up or down a fixed number of scale steps within some scale. The pitches remain in the same scale before and after the shift. This term covers both chromatic and diatonic transpositions as follows. Chromatic transpo ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Hexachordal Combinatoriality
In music using the twelve tone technique, combinatoriality is a quality shared by twelve-tone tone rows whereby each section of a row and a proportionate number of its transformations combine to form aggregates (all twelve tones). Whittall, Arnold. 2008. ''The Cambridge Introduction to Serialism. Cambridge Introductions to Music'', p. 272. New York: Cambridge University Press. (hardback) (pbk). Much as the pitches of an aggregate created by a tone row do not need to occur simultaneously, the pitches of a combinatorially created aggregate need not occur simultaneously. Arnold Schoenberg, creator of the twelve-tone technique, often combined P-0/I-5 to create "two aggregates, between the first hexachords of each, and the second hexachords of each, respectively." Combinatoriality is a side effect of derived rows, where the initial segment or set may be combined with its transformations (T,R,I,RI) to create an entire row. "Derivation refers to a process whereby, for instance, the ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Journal Of The American Musicological Society
The ''Journal of the American Musicological Society'' is a peer-reviewed academic journal and an official journal of the American Musicological Society. It is published by University of California Press The University of California Press, otherwise known as UC Press, is a publishing house associated with the University of California that engages in academic publishing. It was founded in 1893 to publish scholarly and scientific works by facult ... and covers all aspects of musicology. The ''Journal of the American Musicological Society'' has been published three times a year since 1948. It was preceded by the annual ''Bulletin of the American Musicological Society'' (1936–1947) and the annual ''Papers of the American Musicological Society'' (1936–1941). Online versions of the journal and its predecessors are available at JSTOR and the University of California Press. External links * {{Official website, 1=http://www.ucpressjournals.com/journal.asp?j=jams Publications e ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
René Leibowitz
René Leibowitz (; 17 February 1913 – 29 August 1972) was a Polish, later naturalised French, composer, conductor, music theorist and teacher. He was historically significant in promoting the music of the Second Viennese School in Paris after the Second World War, and teaching a new generation of serialist composers. Leibowitz remained firmly committed to the musical aesthetic of Arnold Schoenberg, and was to some extent sidelined among the French avant-garde in the 1950s, when, under the influence of Leibowitz's former student, Pierre Boulez and others, the music of Schoenberg's pupil Anton Webern was adopted as the orthodox model by younger composers. Although his compositional ideas remained strictly serialist, as a conductor Leibowitz had broad sympathies, performing works by composers as diverse as Gluck, Beethoven, Brahms, Offenbach and Ravel, and his repertory extended to include pieces by Gershwin, Puccini, Sullivan and Johann Strauss. Life and career Early years ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Milton Babbitt
Milton Byron Babbitt (May 10, 1916 – January 29, 2011) was an American composer, music theorist, mathematician, and teacher. He is particularly noted for his Serialism, serial and electronic music. Biography Babbitt was born in Philadelphia to Albert E. Babbitt and Sarah Potamkin, who were Jewish. He was raised in Jackson, Mississippi, and began studying the violin when he was four but soon switched to clarinet and saxophone. Early in his life he was attracted to jazz and theater music, and "played in every pit-orchestra that came to town". Babbitt was making his own arrangements of popular songs by age 7, "wrote a lot of pop tunes for school productions", and won a local songwriting contest when he was 13. A Jackson newspaper called Babbitt a "whiz kid" and noted "that he had perfect pitch and could add up his family’s grocery bills in his head. In his teens he became a great fan of jazz cornet player Bix Beiderbecke." Babbitt's father was a mathematician, and Babbitt inten ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
