|
Phase Synchronization
{{no footnotes, date=June 2017 Phase synchronization is the process by which two or more cyclic signals tend to oscillate with a repeating sequence of relative phase angles. Phase synchronisation is usually applied to two waveforms of the same frequency with identical phase angles with each cycle. However it can be applied if there is an integer relationship of frequency, such that the cyclic signals share a repeating sequence of phase angles over consecutive cycles. These integer relationships are called Arnold tongues which follow from bifurcation of the circle map. One example of phase synchronization of multiple oscillators can be seen in the behavior of Southeast Asian fireflies. At dusk, the flies begin to flash periodically with random phases and a gaussian distribution of native frequencies. As night falls, the flies, sensitive to one another's behavior, begin to synchronize their flashing. After some time all the fireflies within a given tree (or even larger area) wi ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Synchronization (other)
Synchronization is the coordination of events to operate a system in unison. Synchronization may also refer to: * Synchronization (alternating current), the process of matching the speed and frequency of a generator or other source to a running network * Synchronization (computer science), the synchronization of processes and data * Synchronization (Nazi Germany) or ''Gleichschaltung'', the process by which the Nazi Party established control over all aspects of German society * Synchronization rights A music synchronization license, or "sync" for short, is a music license granted by the holder of the copyright of a particular composition, allowing the licensee to synchronize ("sync") music with some kind of visual media output (film, televisi ..., also called "sync licensing", to provide copyright permission for music to be used in video, videogames, or other AV works See also * Sync (other) * Synchronization in telecommunications * {{Disambiguation ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
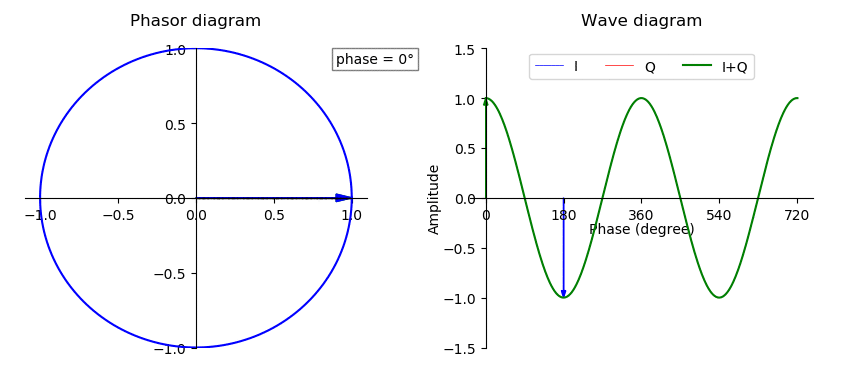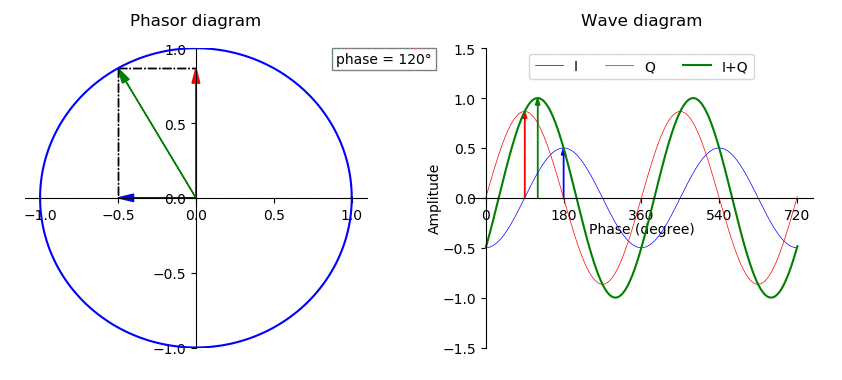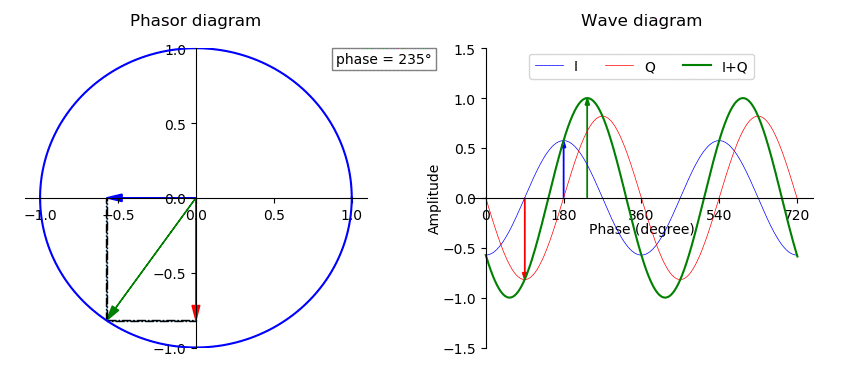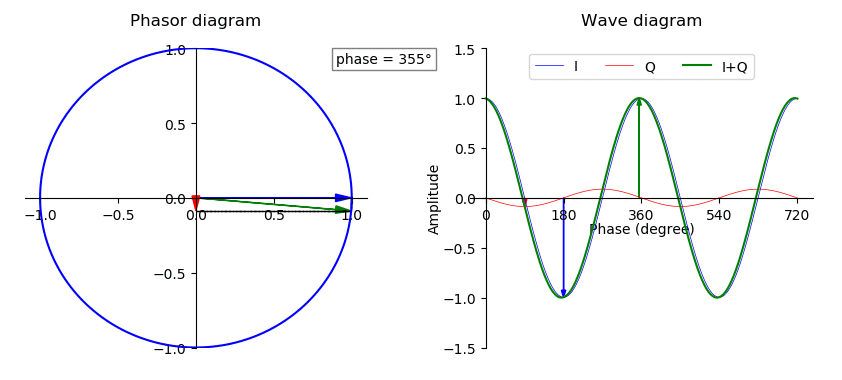
Phase (waves)
In physics and mathematics, the phase of a periodic function F of some real variable t (such as time) is an angle-like quantity representing the fraction of the cycle covered up to t. It is denoted \phi(t) and expressed in such a scale that it varies by one full turn as the variable t goes through each period (and F(t) goes through each complete cycle). It may be measured in any angular unit such as degrees or radians, thus increasing by 360° or 2\pi as the variable t completes a full period. This convention is especially appropriate for a sinusoidal function, since its value at any argument t then can be expressed as \phi(t), the sine of the phase, multiplied by some factor (the amplitude of the sinusoid). (The cosine may be used instead of sine, depending on where one considers each period to start.) Usually, whole turns are ignored when expressing the phase; so that \phi(t) is also a periodic function, with the same period as F, that repeatedly scans the same range ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Arnold Tongues
In mathematics, particularly in dynamical systems, Arnold tongues (named after Vladimir Arnold) Section 12 in page 78 has a figure showing Arnold tongues. are a pictorial phenomenon that occur when visualizing how the rotation number of a dynamical system, or other related invariant property thereof, changes according to two or more of its parameters. The regions of constant rotation number have been observed, for some dynamical systems, to form geometric shapes that resemble tongues, in which case they are called Arnold tongues. Arnold tongues are observed in a large variety of natural phenomena that involve oscillating quantities, such as concentration of enzymes and substrates in biological processes and cardiac electric waves. Sometimes the frequency of oscillation depends on, or is constrained (i.e., ''phase-locked'' or ''mode-locked'', in some contexts) based on some quantity, and it is often of interest to study this relation. For instance, the outset of a tumor triggers ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Fireflies
The Lampyridae are a family of elateroid beetles with more than 2,000 described species, many of which are light-emitting. They are soft-bodied beetles commonly called fireflies, lightning bugs, or glowworms for their conspicuous production of light, mainly during twilight, to attract mates. Light production in the Lampyridae is thought to have originated as an honest warning signal that the larvae were distasteful; this was co-opted in evolution as a mating signal in the adults. In a further development, female fireflies of the genus ''Photuris'' mimic the flash pattern of '' Photinus'' species to trap their males as prey. Fireflies are found in temperate and tropical climates. Many live in marshes or in wet, wooded areas where their larvae have abundant sources of food. While all known fireflies glow as larvae, only some species produce light in their adult stage, and the location of the light organ varies among species and between sexes of the same species. Fireflies h ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Oscillators
Oscillation is the repetitive or periodic variation, typically in time, of some measure about a central value (often a point of equilibrium) or between two or more different states. Familiar examples of oscillation include a swinging pendulum and alternating current. Oscillations can be used in physics to approximate complex interactions, such as those between atoms. Oscillations occur not only in mechanical systems but also in dynamic systems in virtually every area of science: for example the beating of the human heart (for circulation), business cycles in economics, predator–prey population cycles in ecology, geothermal geysers in geology, vibration of strings in guitar and other string instruments, periodic firing of nerve cells in the brain, and the periodic swelling of Cepheid variable stars in astronomy. The term ''vibration'' is precisely used to describe a mechanical oscillation. Oscillation, especially rapid oscillation, may be an undesirable phenomenon in pro ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Phase-locked Loop
A phase-locked loop or phase lock loop (PLL) is a control system that generates an output signal whose phase is related to the phase of an input signal. There are several different types; the simplest is an electronic circuit consisting of a variable frequency oscillator and a phase detector in a feedback loop. The oscillator's frequency and phase are controlled proportionally by an applied voltage, hence the term voltage-controlled oscillator (VCO). The oscillator generates a periodic signal of a specific frequency, and the phase detector compares the phase of that signal with the phase of the input periodic signal, to adjust the oscillator to keep the phases matched. Keeping the input and output phase in lockstep also implies keeping the input and output frequencies the same. Consequently, in addition to synchronizing signals, a phase-locked loop can track an input frequency, or it can generate a frequency that is a multiple of the input frequency. These properties are u ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Algebraic Connectivity
The algebraic connectivity (also known as Fiedler value or Fiedler eigenvalue after Miroslav Fiedler) of a graph ''G'' is the second-smallest eigenvalue (counting multiple eigenvalues separately) of the Laplacian matrix of ''G''. This eigenvalue is greater than 0 if and only if ''G'' is a connected graph. This is a corollary to the fact that the number of times 0 appears as an eigenvalue in the Laplacian is the number of connected components in the graph. The magnitude of this value reflects how well connected the overall graph is. It has been used in analyzing the robustness and synchronizability of networks. Properties The truncated icosahedron or Buckminsterfullerene graph has a traditional connectivity (graph theory)">connectivity of 3, and an algebraic connectivity of 0.243. The algebraic connectivity of undirected graphs with nonnegative weights, a(G)\geq0 with the inequality being strict if and only if G is connected. However, the algebraic connectivity can be negative f ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Coherence (physics)
In physics, two wave sources are coherent if their frequency and waveform are identical. Coherence is an ideal property of waves that enables stationary (i.e., temporally or spatially constant) interference. It contains several distinct concepts, which are limiting cases that never quite occur in reality but allow an understanding of the physics of waves, and has become a very important concept in quantum physics. More generally, coherence describes all properties of the correlation between physical quantities of a single wave, or between several waves or wave packets. Interference is the addition, in the mathematical sense, of wave functions. A single wave can interfere with itself, but this is still an addition of two waves (see Young's slits experiment). Constructive or destructive interference are limit cases, and two waves always interfere, even if the result of the addition is complicated or not remarkable. When interfering, two waves can add together to create a wave of ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Kuramoto Model
The Kuramoto model (or Kuramoto–Daido model), first proposed by , is a mathematical model used to describing synchronization. More specifically, it is a model for the behavior of a large set of coupled oscillators. Its formulation was motivated by the behavior of systems of chemical and biological oscillators, and it has found widespread applications in areas such as neuroscience and oscillating flame dynamics. Kuramoto was quite surprised when the behavior of some physical systems, namely coupled arrays of Josephson junctions, followed his model. The model makes several assumptions, including that there is weak coupling, that the oscillators are identical or nearly identical, and that interactions depend sinusoidally on the phase difference between each pair of objects. Definition In the most popular version of the Kuramoto model, each of the oscillators is considered to have its own intrinsic natural frequency \omega_i, and each is coupled equally to all other oscillato ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Synchronization (alternating Current)
In an alternating current electric power system, synchronization is the process of matching the frequency of a generator or other source to a running network. An AC generator cannot deliver power to an electrical grid unless it is running at the same frequency as the network. If two unconnected segments of a grid are to be connected to each other, they cannot exchange AC power until they are brought back into exact synchronization. A direct current (DC) generator can be connected to a power network by adjusting its open-circuit terminal voltage to match the network voltage, by either adjusting its speed or its field excitation. The exact engine speed is not critical. However, an AC generator must match both the amplitude and the timing of the network voltage, which requires both speed and excitation to be systematically controlled for synchronization. This extra complexity was one of the arguments against AC operation during the war of currents in the 1880s. In modern grids, synchro ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Jürgen Kurths
Jürgen Kurths (born 11 March 1953 in Arendsee/Altmark) is a German physicist and mathematician. He is senior advisor in the research department Complexity Sciences of the Potsdam Institute for Climate Impact Research, a Professor of Nonlinear Dynamics at the Institute of Physics at the Humboldt University, Berlin, and a 6th-century chair for Complex Systems Biology at the Institute for Complex Systems and Mathematical Biology at Kings College, Aberdeen University (UK). His research is mainly concerned with nonlinear physics and complex systems sciences and their applications to challenging problems in Earth system, physiology, systems biology and engineering. Biography Kurths studied mathematics at the University of Rostock and was awarded his PhD in 1983 at the GDR Academy of Sciences, followed by his installation in 1991 in theoretical physics at the University of Rostock. In 1991, in a special program of the Max-Planck-Society, he was selected as one of a few scientists f ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Wave Mechanics
Wave mechanics may refer to: * the mechanics of waves * the ''wave equation'' in quantum physics, see Schrödinger equation See also * Quantum mechanics * Wave equation The (two-way) wave equation is a second-order linear partial differential equation for the description of waves or standing wave fields — as they occur in classical physics — such as mechanical waves (e.g. water waves, sound waves and s ... {{Disambiguation ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |